In a survey, 33% of the respondents stated that they talk to the pots on the telephone A veterinarian believed this result to be too high, so he randomly selected 250 pet owners and discovered that 80 of them spoke to their pot on the telephone Does the veterinarian have a right to be skeptical? Use the a001 vel of Significance 5% of the population size, and the sample Because npo (1-P) 10. the sample size is the requirements for testing the hypothesis satisfied (Round to one decimal place as needed)
Answers
Therefore, the sample size of 250 is sufficient to test the hypothesis.
The hypothesis is that 33 percent of the population speaks to their pets on the phone. However, the veterinarian believed that the result was too high. As a result, he randomly sampled 250 pet owners and discovered that 80 of them spoke to their pot on the telephone.
The veterinarian is right to be skeptical. This is because a hypothesis has to be tested to see if it is true or false.
In this case, the veterinarian conducted a sample test to see if the hypothesis was true.
He found that only 32% of the people sampled spoke to their pets on the phone.
This is less than the hypothesis of 33%.
However, there is still a 5% probability that this result is due to chance.
The sample size is the requirements for testing the hypothesis satisfied. The sample size can be calculated as follows:
npo(1-p) = 10, where n is the sample size, p is the percentage of the population that speaks to their pets on the phone, and o is the margin of error.
In this case, we are given that p = 33%, and the margin of error is 5%.
Therefore, substituting these values into the formula: n x 0.33 x 0.67 ≤ 10.
Solving for n, we get n = 115.6.
To know more about hypothesis visit:
https://brainly.com/question/30821298
#SPJ11
Related Questions
TRUE OR FALSE: The alternative hypothesis states that there is no difference/no effect.
Answers
The answer is FALSE. The alternative hypothesis is a statement that there is a difference or an effect in the population being studied, and it contradicts the null hypothesis, which is a statement that there is no difference or no effect in the population being studied.
The alternative hypothesis is a statement that contradicts the null hypothesis and is generally the hypothesis that the researcher wants to support. It is a statement that there is a difference or an effect in the population being studied.
In hypothesis testing, the null hypothesis is a statement that there is no difference or no effect in the population being studied. The null hypothesis is typically the default hypothesis, which is assumed to be true unless evidence suggests otherwise. The alternative hypothesis, on the other hand, is a statement that there is a difference or an effect in the population being studied, and it is typically the hypothesis that the researcher wants to support.
For example, if a researcher wants to test the effectiveness of a new drug, the null hypothesis might be that the drug has no effect on the condition being treated, while the alternative hypothesis might be that the drug has a positive effect on the condition being treated.
In summary, the alternative hypothesis is a statement that there is a difference or an effect in the population being studied, and it contradicts the null hypothesis, which is a statement that there is no difference or no effect in the population being studied.
To know more about hypothesis:
https://brainly.com/question/14957391
#SPJ11
select the right answer

Answers
Answer:
Blurry po
Step-by-step explanation:
Pwede kunan nyu ulit
Isaiah is grounded and has to stay in his room all day. He made up a game where he throws
balled-up paper called a "trashball" into his trash can. The diameter of the top of the trash can
is 12 in. Isaiah wants the "trashball" to have a diameter that is the diameter of the top of
the trash can.
What should the diameter of Isaiah's
"trashball" be?
in.
d
12 in.
Answers
If Isaiah wants the diameter of the "trashball" to be equal to the diameter of the top of the trash can, then the diameter of the "trashball" should also be 12 inches.
WHAT IS DIAMETER IN 100 W0RDS ?
Diameter is a term used in geometry and mathematics that refers to the length of a straight line segment that passes through the center of a circle or a sphere, and whose endpoints lie on the circle or sphere's circumference.
In simpler terms, the diameter of air circle is the distance across the widest point of the circle, passing through its center. Similarly, the diameter of a sphere is the distance across the widest point of the sphere, passing through its center.
The diameter is a fundamental measurement in geometry and is used to calculate various other properties of circles and spheres, such as their radius, circumference, surface area, and volume. It is an important concept in many areas of science, technology, engineering, and mathematics.
The diameter of a sphere (such as a balled-up paper) is the distance across the widest point of the sphere, passing through the center. So if the diameter of the top of the trash can is 12 inches, then the diameter of the "trashball" should also be 12 inches in order for it to fit properly into the trash can.
To know more about diameter visit :-
https://brainly.com/question/23220731
#SPJ1
Answer: 4 is the diameter
Step-by-step explanation:
1/3 divided by 12=4
see attached photo, please help asap, it is due in 30 minutes !!!

Answers
Answer:
Answer is 3.398
Step-by-step explanation:
Solve by putting into calculator.
Mario works at Bluebonnet Ice Cream parior.He earns $87.50 every 7 hours of work.Rachel works at Guadalupe Music Store and earns $39.75 for every 3 hours of work.How does the amount Mario earns per hour compare with the amount Rachel earns per hour?
Answers
Answer:
63
Step-by-step explanation:
GIVING BRAINIEST IF CORRECT!!
Find the area of the shaded region.

Answers
Area of Rectangle 1 : 20cm^2
Area of Rectangle 2 : 6cm ^ 2
Area of the shaded region :
136 - (20+6)
136 - (26)
110 cm^2
Answer:
110 cm²
Step-by-step explanation:
17 · 8
136 cm²
4 · 1.5
6 cm²
5 · 4
20 cm²
20 cm² + 6 cm²
26 cm²
136 cm² - 26 cm²
110 cm²
a necklace is made from purple pyramids and yellow spheres in a 1 : 6 ratio
Answers
By simplifying the ratio and adding the fractions, we found that 1/7 of the beads are purple pyramids.
Ratios are used to compare two quantities or values. They are often expressed in the form of a fraction or as a percentage.
The given ratio of purple pyramids to yellow spheres is 1:6. This means that for every 1 purple pyramid, there are 6 yellow spheres. We can express this ratio as a fraction:
Purple pyramids : Yellow spheres = 1 : 6
This can be simplified by dividing both sides by 6:
1/6 Purple pyramids : 1 Yellow sphere = 1/6 : 1
Now, we can add the two fractions to get the total fraction of pyramids in the necklace:
1/6 + 1 = 7/6
This means that out of every 7 beads, 1 is a purple pyramid. We can express this as a fraction:
1/7 of the beads are purple pyramids.
So, the fraction of the beads that are pyramids is 1/7.
To know more about ratio here.
https://brainly.com/question/13419413
#SPJ4
Complete Question:
A necklace is made from purple pyramids and yellow spheres in a 1:6 ratio . What fraction of the beads are pyramids?
PLZ help now i need help n

Answers
Answer:
7
Step-by-step explanation:
hey there,
< Here's how you do subtraction in fractions!
Make sure you find a least common denominator. Here, it's given to you to be 15. Usually, you can just multiply the two denominators but then it would be quite large at times, so you kind of just have to guess.
How did they go from \(\frac{4}{5} x-\frac{1}{3} x\) to \(\frac{?}{15} x\)?
Well, they used a least common denominator. 5 was multiplied by 3 to create 15, and 3 was multiplied by 5. But when you multiply the bottoms, you have to multiply the tops of the fraction too (the numerators).
So 4/5x was multiplied by 3. Denominator turned out to be 15, and now the top is 4x3 = 12. So the fraction is 12/15.
1/3x, on the other hand, was multiplied by 5. Denominator was also 15 so they're the same, and top is 1x5 = 5. So the fraction is 5/15.
Now, let's put them together.
\(\frac{12}{15}x-\frac{5}{15} x\)
When subtracting x, the x just stays there, so if it was 10x-4x, the answer would've been 6x. So now let's subtract!
12-5 = 7, and the denominator stays the same.
The answer is \(\frac{7}{15} x\). So the numerator, or answer to your question, would be 7.
Hope this helped! Feel free to ask anything else.
Find the solution to this initial value problem. dy TU + 5 cot(5x) y = 3x³-1 csc(5x), y = 0 dx 10 Write the answer in the form y = f(x)
Answers
The solution to the initial value problem can be written in the form:
y(x) = (1/K)∫|sin(5x)|⁵ (3x³ - csc(5x)) dx
where K is a constant determined by the initial condition.
To solve the initial value problem and find the solution y(x), we can use the method of integrating factors.
Given: dy/dx + 5cot(5x)y = 3x³ - csc(5x), y = 0
Step 1: Recognize the linear first-order differential equation form
The given equation is in the form dy/dx + P(x)y = Q(x), where P(x) = 5cot(5x) and Q(x) = 3x³ - csc(5x).
Step 2: Determine the integrating factor
To find the integrating factor, we multiply the entire equation by the integrating factor, which is the exponential of the integral of P(x):
Integrating factor (IF) = e^{(∫ P(x) dx)}
In this case, P(x) = 5cot(5x), so we have:
IF = e^{(∫ 5cot(5x) dx)}
Step 3: Evaluate the integral in the integrating factor
∫ 5cot(5x) dx = 5∫cot(5x) dx = 5ln|sin(5x)| + C
Therefore, the integrating factor becomes:
IF = \(e^{(5ln|sin(5x)| + C)}\)
= \(e^C * e^{(5ln|sin(5x)|)}\)
= K|sin(5x)|⁵
where K =\(e^C\) is a constant.
Step 4: Multiply the original equation by the integrating factor
Multiplying the original equation by the integrating factor (K|sin(5x)|⁵), we have:
K|sin(5x)|⁵(dy/dx) + 5K|sin(5x)|⁵cot(5x)y = K|sin(5x)|⁵(3x³ - csc(5x))
Step 5: Simplify and integrate both sides
Using the product rule, the left side simplifies to:
(d/dx)(K|sin(5x)|⁵y) = K|sin(5x)|⁵(3x³ - csc(5x))
Integrating both sides with respect to x, we get:
∫(d/dx)(K|sin(5x)|⁵y) dx = ∫K|sin(5x)|⁵(3x³ - csc(5x)) dx
Integrating the left side:
K|sin(5x)|⁵y = ∫K|sin(5x)|⁵(3x³ - csc(5x)) dx
y = (1/K)∫|sin(5x)|⁵(3x³ - csc(5x)) dx
Step 6: Evaluate the integral
Evaluating the integral on the right side is a challenging task as it involves the integration of absolute values. The result will involve piecewise functions depending on the range of x. It is not possible to provide a simple explicit formula for y(x) in this case.
Therefore, the solution to the initial value problem can be written in the form: y(x) = (1/K)∫|sin(5x)|⁵(3x³ - csc(5x)) dx
where K is a constant determined by the initial condition.
To learn more about product rule visit:
brainly.com/question/29198114
#SPJ11
43) five friends were comparing the height of their dogs. the heights at the shoulders were 400mm, 370mm, 470mm, 330mm and 500mm. what is the standard deviation (nearest integer) of the heights of these dogs?: *
Answers
The standard deviation of the heights of these dogs, rounded to the nearest integer, is 63mm.
To find the standard deviation of the heights of these dogs, follow these steps:
1. Calculate the mean (average) height:
(400mm + 370mm + 470mm + 330mm + 500mm) / 5 = 2070mm / 5 = 414mm
2. Calculate the squared differences from the mean:
(400-414)^2 = 196
(370-414)^2 = 1936
(470-414)^2 = 3136
(330-414)^2 = 7056
(500-414)^2 = 7396
3. Calculate the mean of these squared differences:
(196 + 1936 + 3136 + 7056 + 7396) / 5 = 19720 / 5 = 3944
4. Find the square root of this mean:
√3944 ≈ 62.8
The standard deviation of the heights of these dogs, rounded to the nearest integer, is 63mm.
to learn more about standard deviation click here:
https://brainly.com/question/20553308
#SPJ11
The standard deviation of the heights of these dogs, rounded to the nearest integer, is 63m
To find the standard deviation of the heights of these dogs, follow these steps:
Calculate the mean (average) height:
(400mm + 370mm + 470mm + 330mm + 500mm) / 5 = 2070mm / 5 = 414mm
Subtract the mean from each height and square the result:
\((400mm - 414mm)^2 = (-14)^2 = 196\)
\((370mm - 414mm)^2 = (-44)^2 = 1936\)
\((470mm - 414mm)^2 = 56^2 = 3136\)
\((330mm - 414mm)^2 = (-84)^2 = 7056\)
\((500mm - 414mm)^2 = 86^2 = 7396\)
Find the mean of these squared differences:
(196 + 1936 + 3136 + 7056 + 7396) / 5 = 19720 / 5
= 3944
Take the square root of the mean of the squared differences to get the standard deviation:
√3944 ≈ 62.8
For similar question on deviation.
https://brainly.com/question/29800829
#SPJ11
The perimeter of a triangular park is (16x + 3) feet. Two of the sides are 5x - 1 and 2x + 5. What is the missing length?
Answers
Answer:
9x - 1
Step-by-step explanation:
A triangle is a three-sided polygon with three edges and three vertices. the sum of angles in a triangle is 180 degrees
Area of a triangle = 1/2 x base x height
the perimeter of a triangle = sum of the side lengths
missing length = perimeter - sum of the two sides
sum of the two sides = (5x -1) + (2x + 5) = 7x + 4
missing length = 16x + 3 - (7x + 4) = 9x-1
27 g^7 k^3 z^4 - 9 g^2 K^5 z
Answers
The subtraction of the expression 27 g^7 k^3 z^4 - 9 g^2 K^5 z is 18(g⁷k³z⁴ - g²k⁵z)
What are algebraic expressions?Algebraic expressions are described as expressions that are known to consist of terms, coefficients, constants, variables and factors.
They are also described as expressions composed of arithmetic operations, such as;
DivisionBracketParenthesesAdditionMultiplicationSubtractionIt is also important to note that index forms are mathematical representation of variables or values too large or small in more convenient forms.
From the information given, we have the expression;
27 g^7 k^3 z^4 - 9 g^2 K^5 z
Subtract the coefficient
18(g⁷k³z⁴ - g²k⁵z)
Hence, the value is 18(g⁷k³z⁴ - g²k⁵z)
Learn more about algebraic expressions here:
https://brainly.com/question/4344214
#SPJ1
Larry ran 4/5 of the way around the track before slowing down to a walk. What percent of the track did he run? *
Answers
Answer:
80%
Step-by-step explanation:
4 divided by 5 = 0.8
0.8 x 100 = 80%
The percentage Larry ran around the track = 80%
He ran 4/5 of the way around the track before slowing down for a walk.
This means he ran 4 parts of the whole 5 parts of the track. He walks the remaining 1 part around the track.
Therefore, the percentage he ran can be calculated below
% he ran the track = 4 / 5 of 100
% he ran the track = 4 / 5 × 100 = 400 / 5 = 80%
The percentage he ran around the track = 80%
Read more : https://brainly.com/question/2420956?referrer=searchResults
Apply the distributive property to create an equivalent expression 4(x-2+y)
Answers
Answer:
4 * x
4 * -2
4 * y
4x-8+4y
Step-by-step explanation:
Answer:
4x+4y-8
Step-by-step explanation:
(✿◡‿◡) <-- she wants Brainliest
Estimate the product by finding two numbers the exact answer is between 7×3481
Answers
The value of the numerical expression (7 x 3481) will be 24,367.
What is Algebra?Algebra is the study of algebraic expressions, while logic is the manipulation of those concepts.
The acronym PEMDAS stands for Parenthesis, Exponent, Multiplication, Division, Addition, and Subtraction. This rule is used to answer the problem correctly and precisely.
The numbers are given below.
7 and 3481
Then the product of the numbers 7 and 3481 will be given by putting a cross sign between them. Then we have
⇒ 7 x 3481
⇒ 24,367
The value of the numerical expression (7 x 3481) will be 24,367.
More about the Algebra link is given below.
https://brainly.com/question/953809
#SPJ1
In problems 9-12, a family of solutions of a differential equation is given. Find the value of the constant C so the solution satisfies the initial value condition. 9. y' 2x and y(3)=7. y x2 + C. ) 10. y'-3x2-5 and y(1)-2. y x3-5x + C. 11. y'-3y and y(0) 5. у Сезх. 12, y'-2y and y(0)-3, y Ce
Answers
As a result, the constant C has a value of -2. So, option (a) is the appropriate response.(9) Given y'(x) = 2x and y(x) = x^2 + C with y(3) = 7, we have the equation:
7 = 3^2 + C
Solve for C:
C = 7 - 9 = -2
10. Given y'(x) = -3x^2 - 5 and y(x) = x^3 - 5x + C with y(1) = -2, we have the equation:
-2 = 1^3 - 5(1) + C
Solve for C:
C = -2 - 1 + 5 = 2
11. Given y'(x) = -3y and y(x) = Ce^(-3x) with y(0) = 5, we have the equation:
5 = Ce^(-3 * 0)
Solve for C:
C = 5
12. Given y'(x) = -2y and y(x) = Ce^(2x) with y(0) = -3, we have the equation:
-3 = Ce^(2 * 0)
Solve for C:
C = -3
The given family of solutions of a differential equation are:y' = 2x and y(3) = 7.y = x² + CWe need to find the value of constant C so that the solution satisfies the initial value condition.Substitute x = 3 and y = 7 in the equation:y = x² + C7 = 3² + C7 = 9 + CC = -2Therefore, the value of constant C is -2. Hence option (a) is the correct answer.
To know more about equation click here
brainly.com/question/649785
#SPJ11
the following is a summary of a one-way between-subjects anova: f(2, 37) = 3.42, p < .05, η 2 = .12. how many pairwise comparisons need to be made for this anova result?
a.2
b.3
c.4
d.12
Answers
The pairwise comparisons that need to be made for this anova result will be b.3
How to calculate the valueIn order to determine the number of pairwise comparisons needed for a one-way between-subjects ANOVA with three groups, we can use the formula:
N = (k * (k-1)) / 2
Where N represents the number of pairwise comparisons and k represents the number of groups. In this case, k = 3.
Plugging in the values:
N = (3 * (3-1)) / 2
N = (3 * 2) / 2
N = 6 / 2
N = 3
Learn more about ANOVA on
https://brainly.com/question/25800044
#SPJ4
Jacob kept track of the number of hours that he spent playing soccer each week for several weeks he spent 3, 6, 7, 8, and 11 hours. What is the range of the data set?
Answers
Answer:
8
Step-by-step explanation:
The range of the data set is determined by the maximum - minimum.
The max value is 11
The min value is 3
11-3=8
The range of the data set is 8.
-10x + 3y = 5
x=y - 4
Answers
Answer:
You can use the second equation to substitute the value of x in the first equation and solve for y.
x = y - 4
-10(y - 4) + 3y = 5
-10y + 40 + 3y = 5
-7y = 35
y = 5
Now that you have the value of y, you can use the second equation to find the value of x.
x = y - 4
x = 5 - 4
x = 1
So the solution of the system of equations is x = 1 and y = 5
Step-by-step explanation:
You and your friends earn (14h+5c) dollars for washing h houses and c cars last month you washed 10 houses and your friend washed 28 cars who earned more money you,your friend, or neither
Answers
Calculate the density of benzene if 0.10L has a mass of 36.4g.
Answers
Answer:
\(d=0.364\ g/cm^3\)
Step-by-step explanation:
It is given that,
Mass of benzene, m = 0.1 L
Mass, m = 36.4 g
We know that, 1 L = 1000 cm³
0.1 L = 100 cm³
The density of an object is equal to its mass divided by its volume. So,
\(\rho=\dfrac{m}{V}\\\\=\dfrac{36.4\ g}{100\ cm^3}\\\\=0.364\ g/cm^3\)
So, the density of benzene is \(0.364\ g/cm^3\).
heeeeeeeeeeeeeeeeeeeeeeeeeeeeeelp

Answers
Answer:
(3,0)(0-10)
Step-by-step explanation:
check if it is helpful for u
The heights of adult males in the United States are approximately normally distributed. The mean height is 70 inches (5 feet 10 inches) and the standard deviation is 3 inches.
Use the table to estimate the probability that a randomly -selected male is between 67 and 45 inches tall. Express your answer as a decimal.
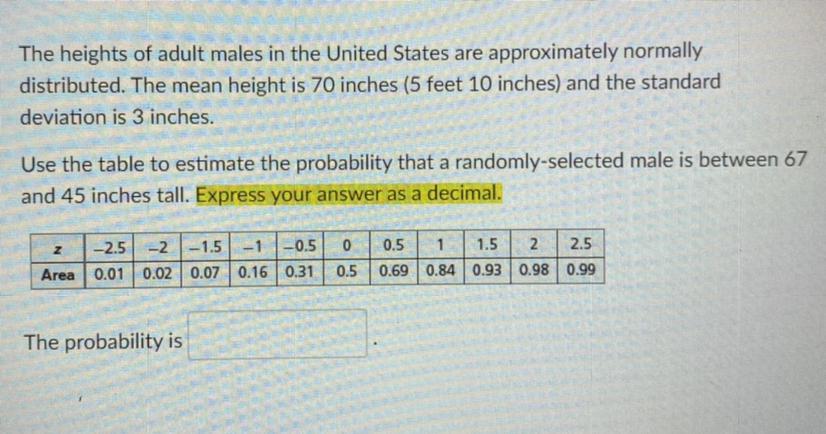
Answers
Using the normal distribution, it is found that the probability is 0.16.
Normal Probability DistributionIn a normal distribution with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
It measures how many standard deviations the measure is from the mean. After finding the z-score, we look at the z-score table and find the p-value associated with this z-score, which is the percentile of X.In this problem, the mean and the standard deviation are given by, respectively, \(\mu = 70, \sigma = 3\).
The proportion of students between 45 and 67 inches is the p-value of Z when X = 67 subtracted by the p-value of Z when X = 45, hence:
X = 67:
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{67 - 70}{3}\)
Z = -1
Z = -1 has a p-value of 0.16.
X = 45:
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{45 - 70}{3}\)
Z = -8.3
Z = -8.3 has a p-value of 0.
0.16 - 0 = 0.16
The probability is 0.16.
More can be learned about the normal distribution at https://brainly.com/question/24663213
Given that y varies directly as x when y = 8 and x=5.
(a) express y in terms of x, and hence
(b) find y when x=30
Answers
Answer:
(a) y = 1.6x
(b) y = 48
Step-by-step explanation:
(a) Since y varies directly as x,
y ∝ x
y = kx [where k = proportionality constant]
If y = 8 and x = 5
By substituting these values in the equation,
8 = 5k
k = \(\frac{8}{5}\)
k = 1.6
Therefore, equation will be,
y = 1.6x
(b) If x = 30, then we have to find the value of y.
By substituting the value of x in the equation,
y = 1.6 × 30
y = 48
find the value of x.

Answers
Answer:
74 I believe
180 - 32 = 148
148/2 = 74
Hope this helps!
Answer:
\(x + x + 32 = 180(sum \: of \: inside \: the \: triangle \: is180) \\ 2x = 180 - 32 \\ x = \frac{148}{2} \\ x = 74\)
Can someone help me slove for d

Answers
Answer:
D=23
Step-by-step explanation:
This is a 90 degree angle
90-39=51
51-5=46
46/2=23
Henry made himself a sandwich that was StartFraction 11 Over 12 EndFraction of a foot long. He put pepperoni on Three-fourths of his sandwich. What length of the sandwich did not have pepperoni on it?
Answers
Answer:
\(\bold{\dfrac{11}{48}\ ft}\)
Step-by-step explanation:
Given that:
Length of sandwich = \(\frac{11}{12}\) of a foot = \(\frac{11}{12}\) ft
Portion of sandwich on which there is pepperoni = \(\frac{3}{4}\) of the total length of sandwich
Portion of sandwich on which there is pepperoni = \(\frac{3}{4}\) \(\times \frac{11}{12}\) ft
To find:
The length of sandwich which does not have the pepperoni on it?
Solution:
First of all, let us find the length which has the pepperoni on it.
Length of sandwich on which there is pepperoni = \(\frac{3}{4}\) \(\times \frac{11}{12}\) ft = \(\frac{33}{48}\) ft
Length of sandwich on which there is no pepperoni can be found by subtracting the length on which there is pepperoni from the total length of sandwich.
Therefore, the answer is:
\(\dfrac{11}{12} - \dfrac{33}{48} = \dfrac{44-33}{48} = \bold{\dfrac{11}{48}\ ft}\)
Answer:
11/48
Step-by-step explanation:
i did this in 6th grade 6 years ago
A triangle has an area of 64 square feet. If the height of the triangle is 8 feet more than its base, x, what are its height and base?
Answers
Answer:
8 feet
Step-by-step explanation:
Given data
Area= 64 square feet
Base= x feet
Height= x+8 feet
The expression for the area
A= 1/2b*h
substitute
64= 1/2*x*(x+8)
64= 1/2*x^2+8x
64= (x^2+8x)/2
cross multiply
64*2= x^2+8x
128= x^2+8x
rearrange
x^2+8x-128= 0
using the quadratic formula
a=1
b=8
c=-128
x= -b±√b^2-4ac/2a
x= -1±√8^2-4*1*-128/2*1
simplifying
x= 8
x=-16
Hence the height of the base is
h= 8 feet
Solve the word problems
1) There are 45 red balloons, 85 blue balloons and
120 yellow balloons. How many red and blue
balloons are there altogether?
(Show your work)
Answers
Answer:
add the red and the blue balloons
45+85=130
3- Find all values of Z such that e² = 2+i√3
Answers
The values of Z such that e² = 2 + i√3 are Z = ln(2 + i√3) + 2πik, where k is an integer.
To find the values of Z, we can start by expressing 2 + i√3 in polar form. Let's denote it as re^(iθ), where r is the modulus and θ is the argument.
Given: 2 + i√3
To find r, we can use the modulus formula:
r = sqrt(a^2 + b^2)
= sqrt(2^2 + (√3)^2)
= sqrt(4 + 3)
= sqrt(7)
To find θ, we can use the argument formula:
θ = arctan(b/a)
= arctan(√3/2)
= π/3
So, we can express 2 + i√3 as sqrt(7)e^(iπ/3).
Now, we can find the values of Z by taking the natural logarithm (ln) of sqrt(7)e^(iπ/3) and adding 2πik, where k is an integer. This is due to the periodicity of the logarithmic function.
ln(sqrt(7)e^(iπ/3)) = ln(sqrt(7)) + i(π/3) + 2πik
Therefore, the values of Z are:
Z = ln(2 + i√3) + 2πik, where k is an integer.
The values of Z such that e² = 2 + i√3 are Z = ln(2 + i√3) + 2πik, where k is an integer.
To know more about polar form visit
https://brainly.com/question/30824428
#SPJ11