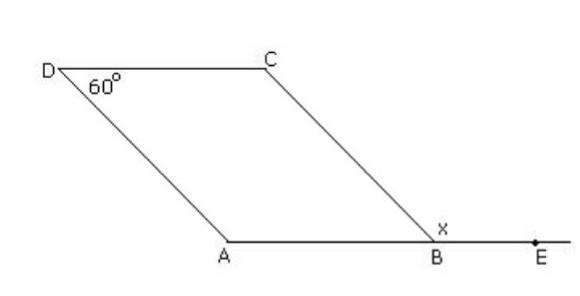
In the given figure, ABCD is a parallelogram. Determine the measure of x.​Please give full explanation.

Answer:

slope = - \(\frac{3}{2}\)

Step-by-step explanation:

calculate the slope m using the slope formula

m = \(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)

with (x₁, y₁ ) = (2, 1 ) and (x₂, y₂ ) = (8, - 8 ) ← 2 ordered pairs from the table

note that any 2 ordered pairs may be used to calculate m

m = \(\frac{-8-1}{8-2}\) = \(\frac{-9}{6}\) = - \(\frac{3}{2}\)

The correct solution to this problem is option e. 1/e. We can find it in the following manner.

The time constant of an RC circuit is the time in which the current decreases to 1/e (approximately 0.368) of its initial value.

This can be derived from the equation for the current in an RC circuit:

\(I=10 * e^({-t/RC} )\)

where I is current at time t, I0 is the initial current, R is the resistance, C is the capacitance, and e is the mathematical constant approximately equal to 2.71828.

To find the time constant, we set the exponent to -1:

\(e^({-t/RC} ) = 1 /e\)

Solving for t/RC, we get:

t/RC = 1

Therefore, the time constant is equal to RC, and the current decreases to 1/e of its initial value in one time constant.

So the answer is e. 1/e.

Learn more about RC circuits here brainly.com/question/2741777

#SPJ4

Answer:

\(\huge \fbox \pink {A}\huge \fbox \green {n}\huge \fbox \blue {s}\huge \fbox \red {w}\huge \fbox \purple {e}\huge \fbox \orange {r}\)

\(2(x + 2) - 5 = 3(x + 1) \\ 2x + 4 - 5 = 3x + 3 \\ 2x - 1 = 3x + 3 \\ - 1 - 3 = 3x - 2x \\ - 4 = x\)

Answer:

6.25 hours

Step-by-step explanation:

pages per hour

pages/hour

90/2.5 = 36

36 pages per hour

225 / 36 = 6.25 hours

Answer:

6.25 hours

Step-by-step explanation:

2 and half hours = 2.5 hours

Proportions:

90 pages  ⇔  2.5 hours

225 pages  ⇔  E hours

E = 225*2.5/90

E = 6.25 hours

6.25 hours = 6 and 1 quarter hours

Answer:

18 pints

Step-by-step explanation:

As we can see, three pint of yellow paint was mixed with four pint of red paint. Let x represent the number of yellow paint and let y represent the number of red paint. Therefore, the ratio of the two paints is:

x/y = 3/4

x = (3/4)y

To calculate the number of red paints (x) that is needed to be mixed with 24 pints of yellow paint, we use the equation and substitute y as 24. Hence:

x =  (3/4)y

x =  (3/4) * 24

x = 18 pints

Step-by-step explanation:

Here is how you do this problem:

we start off with the ratio of Darjeeling in the tea as 20% which is 1/5

every time we add (x) amount of Darjeeling, the total weight of the mixture would also increase by (x) amount

this idea can be represented by the expression

1 + x

---------- = 0.7

5 + x

I set this equal to 0.7 because our goal is to get it to 70% = 0.7

solving for x should get you to your answer

Answer:

8 1/3

Step-by-step explanation:

Answer:

56

Step-by-step explanation:

Answer:

B

Step-by-step explanation:

Because of the direction the line is going, we can automatically eliminate A and D.

The y-intercept is positive, so C is eliminated.

This leaves B as the answer. I hope this was helpful!

A NORMAL distribution is a symmetrical probability distribution with a bell-shaped curve. It is also called a Gaussian distribution or a normal curve. This distribution is often used in statistics to represent a large number of natural phenomena, such as the distribution of height, weight, or IQ scores in a population.

The first deviation of a NORMAL distribution includes 68.2% of the data. This means that if we were to take a random sample of data from a normally distributed population, about 68.2% of the data would be within one standard deviation of the mean.

The second deviation includes 95.4% of the data. This means that about 95.4% of the data would be within two standard deviations of the mean.

The third deviation includes 99.7% of the data. This means that about 99.7% of the data would be within three standard deviations of the mean.

It is important to note that while these percentages hold true for a NORMAL distribution, they may not apply to other types of distributions.

learn more about Gaussian distribution here: brainly.com/question/29734279

#SPJ11

Dividing two polynomials may produce another polynomial only when the divisor has a lower degree than the dividend. Otherwise, it will generally result in a rational function.

No, dividing two polynomials may not result in another polynomial. It can produce a rational function.

A polynomial is an algebraic expression consisting of terms with non-negative integer exponents. When two polynomials are divided, the result is not always a polynomial.

If the degree of the polynomial being divided (dividend) is higher than the degree of the polynomial dividing (divisor), then the result can be a polynomial with a lower degree. However, if the degree of the divisor is equal to or greater than the degree of the dividend, the result will generally be a rational function, which is a ratio of two polynomials.

A rational function has the form P(x)/Q(x), where P(x) and Q(x) are polynomials and Q(x) is not equal to zero. The division can introduce terms with negative or fractional exponents, making the result a rational function rather than a polynomial.

For more such questions on polynomial

https://brainly.com/question/1496352

#SPJ8

The standard error of the distribution of sample proportions for samples of size 1100 from a population with proportion 0.87 is approximately 0.0101.

The formula to calculate the standard error of the distribution of sample proportions is given by the equation:

Standard Error = √((p(1-p))/n)

where p is the population proportion and n is the sample size.

In this case, the population proportion is 0.87 and the sample size is 1100. Plugging in these values into the formula, we get:

Standard Error = √((0.87(1-0.87))/1100)

Simplifying further, we have:

Standard Error = √((0.87 * 0.13)/1100)

Standard Error = √(0.1131/1100)

Standard Error = √0.000102818

Standard Error ≈ 0.0101

So, the standard error of the distribution of sample proportions for samples of size 1100 from a population with proportion 0.87 is approximately 0.0101.

To learn more about sample size

https://brainly.com/question/32911129

#SPJ11

The value of the union of sets A and B is, P(A ∪ B) is 0.64.

The union of two sets means the total elements in both the sets combined.

Given: sets P(A) = 0.38, P(B) = 0.83, and  intersection P(A ∩ B) = 0.57

We need to find the union of P(A ∪ B).

P(A ∪ B) = P(A) + P(B) - P(A ∩ B)

Let us substitute the given values in the formula.

P(A ∪ B) = P(A) + P(B) - P(A ∩ B)

=0.38+0.83-0.57

=1.21-0.57

=0.64

Therefore, the value of union of sets P(A ∪ B) is 0.64.

#SPJ11

Let us know more about sets : https://brainly.com/question/30705181.

Answer:

7.01.

Step-by-step explanation:

We can use the Law of Cosines to solve for c:

c^2 = a^2 + b^2 - 2ab*cos(C)

We are given a = 7, m∠A = 50°, and m∠B = 60°. We can use the fact that the angles in a triangle add up to 180° to find m∠C:

m∠C = 180° - m∠A - m∠B

m∠C = 180° - 50° - 60°

m∠C = 70°

Now we can substitute the known values into the Law of Cosines and solve for c:

c^2 = 7^2 + b^2 - 27bcos(70°)

c^2 = 49 + b^2 - 14bcos(70°)

We don't know b, but we can solve for it using the given equation:

3/2 + b = 7/4

Subtracting 3/2 from both sides:

b = 7/4 - 3/2

b = 1/4

Now we can substitute b = 1/4 into the equation for c^2:

c^2 = 49 + (1/4)^2 - 14*(7/4)*(1/4)*cos(70°)

c^2 = 49.1709

c ≈ 7.01

Therefore, the length of side c is approximately 7.01.

1. The table of values for the function

f(x) = 30 - 2x between

x = 7 and

x = 27 is:x7 12 17 22 27f(x) 16 6 -4 -14 -20a. Using the right endpoint, we have the following Riemann sum:

R5 = Δx [f(12) + f(17) + f(22) + f(27) + f(20)]where

Δx = (27 - 7)/5 = 4. The sum is:

R5 = 4[(6) + (-4) + (-14) + (-20) + (16)]

= -32.

Therefore, the area between the curve and the x-axis on the interval [7,27] is approximately -32 square units.b. Using the left endpoint, we have the following Riemann sum:

L5 = Δx [f(7) + f(12) + f(17) + f(22) + f(27)]where

Δx = (27 - 7)/5

= 4. The sum is:

L5 = 4[(16) + (6) + (-4) + (-14) + (-20)]

= -88 Therefore, the area between the curve and the x-axis on the interval [7,27] is approximately -88 square units.2. The graph of the function

f(x) = x - x²

is shown below:To find the area above the curve and below the x-axis, we need to integrate the function from

x = 0 to

x = 1

f(x) = x - x²∫f(x)dx = ∫(x - x²)dx

= (x²/2) - (x³/3).

The area above the curve and below the x-axis is equal to ∫[0,1] f(x) dx:∫[0,1]

f(x) dx = [(1/2) - (1/3)] - [0 - 0]

= 1/6 Therefore, the area above the curve and below the x-axis is 1/6 square units.3. The population of a city that is growing at a continuous rate of 3.5% per year can be modeled by the function:

P(t) = 200,000e^(0.035t)

where t is the number of years since 2000.a. The population of the city as a function of the number of years since 2000 is given by the function:

P(t) = 200,000e^(0.035t)

To know more about values visit :

https://brainly.com/question/30145972

#SPJ11

Answer:

Step-by-step explanation:

Given,

Number of rainy days = 18

Number of cloudy days = 14

Number of sunny days = 29

Therefore,

Number of days either sunny or cloudy = 14 + 29 = 43

So,

Number of more days sunny or cloudy than rainy are

= 43 - 18 = 25

Hence,

Option C 25 is the correct answer.

Step-by-step explanation:

(-3y^2+24y)(-6y^2-3y)

open the bracket and collect the like terms

-3y^2-6y^2+24y-3y

-9y^2+21y

To ensure a zero cash flow in the portfolio, the writer needs to set up a portfolio that offsets the cash flow from selling the derivatives. The writer should have -3 underlying assets in the portfolio (meaning short sold assets)

The cash flow from selling 100 derivatives can be calculated as:

Cash Flow from selling derivatives = 100 * (Premium at time 0 - Expiry value)

Cash Flow from selling derivatives = 100 * (1.34 - 0.64) = 70

To offset this cash flow, the writer needs to include an equal and opposite cash flow from the underlying assets. Let's assume the writer buys ""x"" underlying assets.

Cash Flow from underlying assets = x * (Value at time 0 - Value at time 1)

Cash Flow from underlying assets = x * (36 - 57) = -21x

To make the cash flow from underlying assets equal to the cash flow from selling derivatives, we set up the equation:

-21x = 70

Solving this equation, we find:

x ≈ -3.33

Since the number of underlying assets must be an integer, we round -3.33 to the nearest integer, which is -3.

Therefore, the writer should have -3 underlying assets in the portfolio (meaning short sold assets).

To know more about derivative, click here: brainly.com/question/25324584

#SPJ11

Answer:

D. none of the above.

Step-by-step explanation:

Answer:

Distributive property.

Step-by-step explanation:

The 2 is being distributed to a and 5.

Answer:

Distributive Property

Step-by-step explanation:

You have to distribute the 2 to what’s in the parenthesis first.

Answer:

Answer: -1

Step-by-step explanation:

The Polynomial Remainder Theorem

It states that the remainder of the division of a polynomial f(x) by (x-r) is equal to f(r).

We have the polynomial:

\(f(x)=x^3+x^2+x+1\)

And we need to determine if x=1 and/or x=-1 are zeros of the polynomial.

Considering the polynomial remainder theorem, if we try any value for x, and the remainder is zero, then that value of x is a root or zero of the polynomial.

Find:

\(f(1)=1^3+1^2+1+1\)

f(1)=4

Thus, x=1 is not a zero of f(x)

Now, find:

\(f(-1)=(-1)^3+(-1)^2+(-1)+1\)

\(f(1)=-1+1-1+1=0\)

Thus, x=-1 is a zero of f(x)

Answer: -1

To find the vector v with the same direction as u and a length of 3, we can scale the vector u by a factor of 3 divided by its magnitude.

Given vector u = (1, 2, -1, 0) and the desired length of v as 3, we first need to calculate the magnitude (or length) of vector u.

The magnitude of a vector is computed using the formula:

∥u∥ = √(u1² + u2²+ u3² + u4²), where u₁, u₂, u₃, and u₄ are the components of vector u.

In this case, the magnitude of vector u is √(1² + 2² + (-1)² + 0²) = √6. To find vector v with the same direction as u and a length of 3, we scale u by the factor 3/√6.

This can be done by multiplying each component of u by the scaling factor.

Therefore, vector v = (3/√6) * (1, 2, -1, 0) = (√6/2, √6, -√6/2, 0).

Hence, vector v has the same direction as u and a length of 3.

Learn more about vector:

brainly.com/question/30958460

#SPJ11

Step-by-step explanation:

y/8=-10-5

y/8=-15

y=15×8

y=120

As a drop tester, you must drop cell phones from a predetermined height in the scenario to determine how much damage needs to be repaired. You discovered that the typical repair expense for 8 phones is $125.

You can run a hypothesis test to further analyse the data and determine the population of cell phones. Assume for the moment that the cost of repairing a cell phone has a normal distribution.The stages for performing a hypothesis test are as follows:

1. Identify the alternative hypothesis (Ha) and the null hypothesis (H0):

  - The population's mean repair cost is equal to a predetermined amount, such as $125 in the case of the null hypothesis (H0).

  - An alternative theory (Ha) The mean repair cost for the population is not equal.

learn more about predetermined here :

https://brainly.com/question/29829712

#SPJ11

Answer:c

Step-by-step explanation:

Hi there!

The ratio of the base to the height is 4:1, so:

12 cm : x cm

12/4 = 3, which is the scale. Thus, x = 3 cm.

Find the area using the equation A = b × h

A = 3 × 12 = 36 cm²

Answer:

All of the following proportions are equivalent except a/d=c/b.

a/b=c/d

b/a=d/c => a/b = c/d

a/d=c/b not equal to a/b = c/d

a/c=b/d => a/b = c/d

Step-by-step explanation:

Answer:

Step-by-step explanation:

y-intercept:5

slope:-½

linear equation slope intercept form:y=mx+b

therefore

y=-½x+5

our answer is D

Answer:

The radius of the sphere will be:

Step-by-step explanation:

Given that a sphere has the same volume as a cube with edge length 5.

\(V_c=V_s\)

\(\:a^3=\frac{4}{3}\pi r^3\)

\(\:r^3=\frac{3\:a^3}{4\pi }\)

\(\:\:r=\sqrt[3]{\frac{3\:a^3}{4\pi \:}\:\:}\)

substitute a = 5

\(r=\sqrt[3]{\frac{3\:\left(5\right)^3}{4\pi \:\:\:}\:\:}\)

\(r=3.1\) units

Therefore, the radius of the sphere will be: