Is –6 − 65 positive or negative?

Answer:

B. Jenny paid $322 for 23 T-shirts, which is a rate of $14 per T-shirt.

Step-by-step explanation:

To find the rate per shirt, divide the cost by the number of shirts:

322/14

= 14

So, she paid $322 at a rate of $14 per shirt.

Therefore, B is correct.

28.26 sq. Ft is the area of the table top

Answer:

\( {y}^{ - 1} = \sqrt{x - 16} \)

Step-by-step explanation:

Answer:

\(h(x)=-0.11(3)^{-x}\)

Step-by-step explanation:

Given function:

\(f(x)=0.11(3)^x\)

Reflection in the x-axis:

When reflecting a function in the x-axis, make the whole function negative:

\(\implies g(x)=-f(x)\)

\(\implies g(x)=-0.11(3)^x\)

Reflection in the y-axis:

When reflecting a function in the y-axis, make the x variable negative:

\(\implies h(x)=g(-x)\)

\(\implies h(x)=-0.11(3)^{-x}\)

Learn more about graph transformations here:

https://brainly.com/question/27845947

https://brainly.com/question/27962370

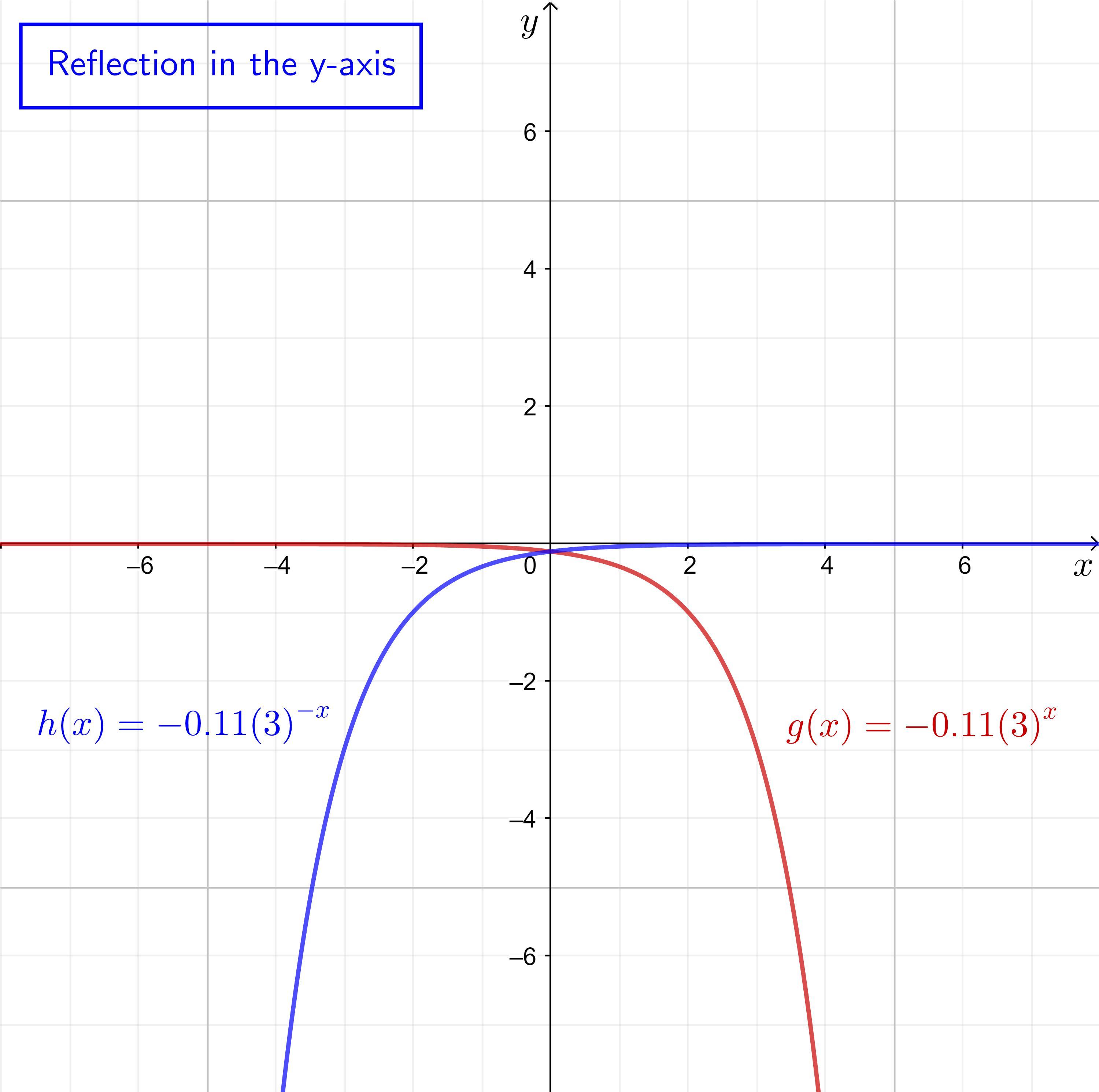
Answer:

the answer to this question is d

Step-by-step explanation:

h(x)=-0.11(3)^-x

We would expect to find the middle 68% of the average amounts of nicotine in a sample of size 41 to be between approximately 0.901 g and 0.991 g by using properties of the normal distribution.

To find the range in which you would expect to find the middle 68% of amounts of nicotine in these cigarettes, we can use the properties of the normal distribution.

Given:
Mean (μ) = 0.946 g
Standard deviation (σ) = 0.289 g

For a normal distribution, approximately 68% of the data falls within one standard deviation of the mean. Therefore, we can expect the middle 68% of amounts of nicotine to fall within the range:

μ ± σ

Substituting the given values:

0.946 ± 0.289

To calculate the range, we have:

Lower bound = 0.946 - 0.289 ≈ 0.657 g
Upper bound = 0.946 + 0.289 ≈ 1.235 g

Therefore, we can expect to find the middle 68% of amounts of nicotine in these cigarettes to be between approximately 0.657 g and 1.235 g.

Now, if we were to draw samples of size 41 from this population, the standard deviation of the sample mean (also known as the standard error) can be calculated as:

Standard error (SE) = σ / √n

where σ is the population standard deviation and n is the sample size.

Substituting the given values:

SE = 0.289 / √41 ≈ 0.045 g

To find the range in which we would expect to find the middle 68% of the sample means, we multiply the standard error by 1 to capture approximately 68% of the data, giving us:

Lower bound = μ - SE ≈ 0.946 - 0.045 ≈ 0.901 g
Upper bound = μ + SE ≈ 0.946 + 0.045 ≈ 0.991 g

Therefore, we would expect to find the middle 68% of the average amounts of nicotine in a sample of size 41 to be between approximately 0.901 g and 0.991 g.

To Learn more properties of the normal distribution go to:

https://brainly.com/question/18444740?referrer=searchResults

#SPJ11

The graph that shows the solution to the system of equations is attached below.

The solution to a system of linear equations is the point where the two graphs intersect. The coordinates of that point is the solution.

Given the system as:

y = 2/3x + 1

y = -2/3x - 1

Add both together to eliminate x

2y = 0

y = 0

Substitute y = 0 into y = 2/3x + 1 to find x

0 = 2/3x + 1

-1 = 2/3x

-3 = 2x

-3/2 = x

x = -3/2

The solution is: (-3/2, 0) = (-1.5, 0).

Therefore, the graph that shows the solution will intersect at (-1.5, 0), which is shown in the image attached below.

Learn more about the solution of a system of linear equations on:

https://brainly.com/question/14323743

#SPJ1

Answer:

c

Step-by-step explanation:

way simple

Answer:

area = 35 in²

Step-by-step explanation:

Answer:

10 is a real, rational, integer, whole and natural number,

5/6 is a rational number ,

24sqrt is irrational number,

0 is a real, rational, integer, whole and natural number ,

and

-81sqrt is a real, rational, integer, whole, and natural number.

Hope you have a nice day!

Mean of the data = 83.92  

The term "mean" refers to the average of the data and is derived by dividing the total number of data observations in the data by the total number of observations.

It is a data point that represents the average of all the data points in the collection. The most popular and commonly applied approach in statistics for determining the middle of a data collection is the mean.  

Here given data is

No of students(f\(_{i}\))         4        3       9         2       3        3          4  

Score (x\(_{i}\))                     70      75     80       85      90      95      100        

f\(_{i}\) x\(_{i}\)                          280       225    720    170     270    285    400      

The formula for the mean of the data is given by

=>  ∑ f\(_{i}\) x\(_{i}\) = 280 + 225 + 720 + 170 + 270 + 285 + 400 = 2350

=>  ∑f\(_{i}\)   = 4 + 3 + 9 + 2 + 3 + 3 + 4 = 28

=> Mean of the data = 2350/28 = 83.92  

Therefore,

The Mean of the data = 83.92

Learn more about Mean of data at

https://brainly.com/question/11163338

#SPJ1

The range of the functions f(x) = |x| 9 and g(x) = –6 then (f+g)(x) will be equal  to (f+g)(x)\(\geq\)3 for all the values of x.

Function, in mathematics, an expression, rule, or law that defines a relationship between one variable (the independent variable) and another variable (the dependent variable).

It is given that

\(f(x)=|x|+9\)

\(g(x)=-6\)

So now

\((f+g)(x)=|x|+9-6=|x|+5\)

So from the above equation, we can see that (f+g)(x) describes the range  (f+g)(x)\(\geq\)3

To know more about function follow

https://brainly.com/question/4025726

Answers 76:

Step-by-step explanation:

Answer:

rectangle C rectangle B rectangle A

Step-by-step explanation:

choose fourth is right

Answer:

your anwser is 1085

Step-by-step explanation:

For DNA testing the most popular and reliable way to collect samples is the oral buccal swab method.

What is DNA testing?

The Polymerase Chain Reaction (PCR) is the most used type of DNA analysis (PCR). Because PCR testing has improved the success rate of the analysis of old, deteriorated, or extremely tiny biological evidential materials, it has significantly advanced the area of forensic DNA testing.

Businesses compare their data from a database that might not yield conclusive findings. The majority of DNA testing businesses base their DNA accuracy checks on common genetic variants that can be identified in their database. As a result, if you employ various businesses, you can obtain different outcomes.

To learn more about DNA accuracy  visit:https://brainly.com/question/12459304

#SPJ1

The integers greater than 999 but not greater than 4000 is 375.

Integers are the collection of whole numbers and negative numbers. Similar to whole numbers, integers also does not include the fractional part. Thus, we can say, integers are numbers that can be positive, negative or zero, but cannot be a fraction. We can perform all the arithmetic operations, like addition, subtraction, multiplication and division, on integers. The examples of integers are, 1, 2, 5,8, -9, -12, etc. The symbol of integers is “Z“.

The smallest number in the series is 1000, a4-digit number.

The largest number in the series is 4000, a4-digit number

The left most digits (thousands place) of each of the 4 digit numbers other than 4000 can take one of the 3 values 1 or 2 or 3.

The next 3 digits (hundreds, tens and units place) can take any of the 5 values 0 or 1 or 2 or 3 or 4.

Hence, there are 3×5×5×5 or 375 numbers from 1000 to 3999.

For more such questions on integers:

https://brainly.com/question/7387118

#SPJ4

As per arithmetic progression the value of 15th term is 0.

An arithmetic progression, or A.P., is a sequence of numbers in which the difference between consecutive terms is constant.

To start, let's call the common difference "d" and the first term "a". We can use the formula for the nth term of an A.P., which is Tn = a + (n-1)d, where Tn is the nth term and n is the position of the term in the A.P.

Using this formula, we can find the 6th term and 9th term. For the 6th term, T6 = a + (6-1)d = a + 5d. For the 9th term, T9 = a + (9-1)d = a + 8d.

Now, using the information given, we can create an equation: 6(a + 5d) = 9(a + 8d). Expanding both sides and simplifying, we get:

6a + 30d = 9a + 72d

Subtracting 9a from both sides, we get:

-3a + 30d = 72d

Subtracting 30d from both sides, we get:

-3a = 42d

Dividing both sides by -3, we get:

a = -14d

Now that we have found the value of "a", we can use the formula for the nth term again to find the 15th term. T15 = a + (15-1)d = -14d + 14d = 0.

So, the 15th term of the A.P. is 0.

To know more about arithmetic progression here.

https://brainly.com/question/16947807

#SPJ4

The maximum volume that a cylinder with a surface area of 90 can have is approximately 27.05 cubic units

To solve this problem, we need to use the formulas for the surface area and volume of a cylinder

Surface area = 2πr^2 + 2πrh

Volume = πr^2h

where r is the radius of the base of the cylinder, h is the height of the cylinder, and π is approximately equal to 3.14159.

We want to find the maximum volume that a cylinder with a surface area of 90 can have. Let's first solve the surface area formula for h

90 = 2πr^2 + 2πrh

45 = πr^2 + πrh

45/π = r^2 + rh/π

Next, we can solve the volume formula for h

V = πr^2h

h = V/(πr^2)

Now we substitute this expression for h into the surface area formula

45/π = r^2 + r(V/(πr^2))

45/π = r^2 + V/r

To find the maximum volume, we need to find the value of V that maximizes this expression. We can do this by taking the derivative with respect to r and setting it equal to zero

d/dx (r^2 + V/r) = 2r - V/r^2 = 0

2r = V/r^2

r^3 = V/2

Now we can substitute this expression for V into the surface area formula to find the corresponding value of r

45/π = r^2 + r(V/(πr^2))

45/π = r^2 + r/2r^2

45/π = r^2 + 1/(2r)

45/π - 1/(2r) = r^2

r^2 = (45/π - 1/(2r))

r^4 = 45r/(π) - 1/4

We can solve this quartic equation for r using numerical methods, such as the Newton-Raphson method. Alternatively, we can use trial and error to approximate the value of r that satisfies this equation

V ≈ 27.05 cubic units

Learn more about volume here

brainly.com/question/1037971

#SPJ4

Answer:

Step-by-step explanation:

On the day of the canteen, 800 coupons were sold, the price of each coupon was RM 30 and RM 50 respectively. The amount of money earned from the sale of coupons was RM30000. How many copies of RM30 and RM50 coupons were sold?

Let:

RM 30 = x

RM 50 = y

x + y = 800 - - - (1)

30x + 50y = 30000 - - - (2)

From (1)

x = 800 - y

Put x = 800 - y in (2)

30(800 - y) + 50y = 30000

24000 - 30y + 50y = 30000

24000 + 20y = 30000

20y = 30000 - 24000

20y = 6000

y =

The solution to the radical equation is t = 2.

Here, we have,

The given radical equation is:

√(t + 2) + 6 = 4

To solve this equation, we can start by isolating the radical term on one side of the equation and then squaring both sides to eliminate the square root.

Step 1: Subtract 6 from both sides of the equation:

√(t + 2) = 4 - 6

√(t + 2) = -2

Step 2: Square both sides of the equation to eliminate the square root:

(√(t + 2))^2 = (-2)^2

t + 2 = 4

Step 3: Subtract 2 from both sides of the equation:

t + 2 - 2 = 4 - 2

t = 2

The solution to the radical equation is t = 2.

More can be learned about radical equations at

brainly.com/question/7133743

#SPJ4

Answer:

A- 200+25x

B- 200+25×4=300

Continued for question B: The input of 4 into "x" indicates how many cans of paint you have bought

Step-by-step explanation:

Answer:

Step-by-step explanation:

The slope is in the given line. It is the number in front of the x which is 3.

So far what you have is

y = 3x + b

Now use the point to find b

x = 2

y = 2

Substitute

y = 3*2 + b

2 = 6 + b

2 - 6 = b

b = - 4

y = 3x - 4

Answer:

18.42

Explanation:

15+3=18

0.4+0.02=0.42

18+0.42=18.42

Answer:

Step-by-step explanation:

You want the areas of an octagon and a square, each with a perimeter of 80 ft.

The side length of a square is 1/4 of its perimeter, so the square of interest has a side length of ...

  (80 ft)/4 = 20 ft

The area is the square of the side length, so the area of the square is ...

  A = s²

  A = (20 ft)² = 400 ft²

A regular octagon has 8 sides of equal length, so the side length is ...

  (80 ft)/8 = 10 ft

The area is found by the formula ...

  A = 2(1 +√2)s²

  A = 2(1 +√2)(10 ft)² ≈ 483 ft²

The area of the octagon is about 483 square feet; about 400 square feet for the square.

<95141404393>

Answer:

(See the attachment)

Step-by-step explanation:

None

Answer: Answer below

Step-by-step explanation:

let f(t) = a(b^t), with f(−1) = 1/2 and f(2) = 108. find the values of a and b.

let f(t) = a(b^t), with f(−1) = 1/2 and f(2) = 108. We have to find the values of a and b.The function f(t) = a(b^t) is of the form exponential functions.

Exponential functions: The exponential function is of the form y = ab^x. The base b must be greater than zero but cannot be equal to 1. The exponential function is a one-to-one function, which means that it passes the horizontal line test. Therefore, it has an inverse function.a. Finding the value of a. Using the value of f(−1) = 1/2, we get

a(b^−1) = 1/2

a/b = 1/2 .............(1)

Using the value of f(2) = 108, we get

a(b^2) = 108

a(b^2) = 2^2 * 3^3 ..........(2)

Dividing equation (2) by equation (1), we get

b^3 = 2^3 * 3^3

b = 2 * 3

b = 6

Plugging the value of b = 6 in equation (1), we get a/6 = 1/2 => a = 3

Therefore, the values of a and b are a = 3 and b = 6.

Learn more about Exponential functions: https://brainly.com/question/2456547

#SPJ11

Answer:

y= x + 5

Step-by-step explanation:

i took the test

The lifetime of meson according to laboratory clocks would be 14 μs.

In this question,

Meson decay, Δt = 2μs

Velocity, y = 0.99C, where C is the speed of the light

Time measurement depends on the reference frame of clock.

Time dilation, Δt' = y Δt

⇒  \(\frac{\Delta t }{\sqrt{1-\frac{v^{2} }{c^{2} } } }\)

⇒ \(\frac{2(10^{-6} ) }{\sqrt{1-\frac{(0.99c)^{2} }{c^{2} } } }\)

⇒ \(\frac{2(10^{-6} ) }{\sqrt{1-0.99^{2} } } }\)

⇒ \(\frac{2(10^{-6} ) }{\sqrt{1-0.9801 } } }\)

⇒ \(\frac{2(10^{-6} ) }{\sqrt{0.0199} } }\)

⇒ \(\frac{2(10^{-6} ) }{0.1410} } }\)

⇒ 14.18 × 10⁻⁶ ≈ 14 μs

Hence we can conclude that the lifetime of meson according to laboratory clocks would be 14 μs.

Learn more about lifetime at laboratory clocks here

https://brainly.com/question/23455167

#SPJ4

Porosity calculated as ratio of pore to total vol. Plot of porosity vs averaging length scale (edge of square used for averaging). Rep. elemental vol = vol of square used for averaging.

The porosity as a function of the averaging length scale can be calculated as follows:

Learn more about geometry :

https://brainly.com/question/10700399

#SPJ4