Jason plays a video game each weekend. He scored 100,000 more points on level 10 than he did on level 11. If he scored 785,354 points on level 10, what is his score on level 11?
Answers
Related Questions
40% of the children in a class are girls. If the number of girls 20, look for the total number of children in the class.
Answers
Answer:
50
Step-by-step explanation:
40% = 20
1% = \(\frac{20}{40}\) = 0.5
100% = 0.5 × 100 = 50
Answer:
No. of girls in class = 20
if 40% = 20 students then the rest 60% must be 30 students
No. of boys in class = 30
Total no. of students in class = 20+30
=50 students.
Step-by-step explanation:
Thanks you! Please mark me as brainliest.
find the volume of the region e bounded by the functions z=0 , z=1 and x^2 y^2 z^2=4
Answers
The volume of the region E is 2(\(2 - \sqrt(2)\)).
How to find the volume of the region e bounded by the functions?The region E is bounded by the plane z = 0, the plane z = 1, and the surface\(x^2y^2z^2 = 4\). To find its volume, we can use a triple integral over the region:
V = ∭E dV
Since the region is bounded by z = 0 and z = 1, we can integrate over z first and then over the region in the xy-plane:
V = ∫∫∫E dV = ∫∫R ∫\(0^1\) dz dA
where R is the region in the xy-plane defined by \(x^2y^2z^2 = 4\). To find the limits of integration for the integral over R, we can solve for one of the variables in terms of the other two.
For example, solving for z in terms of x and y gives:
z = 2/(xy)
Since z is between 0 and 1, we have:
0 ≤ z ≤ 1 ⇔ xy ≥ 2
So the region R is the set of points in the xy-plane where xy ≥ 2. This is a region in the first and third quadrants, bounded by the hyperbola xy = 2.
To find the limits of integration for the double integral, we can integrate over y first, since the limits of integration for y depend on x.
For a fixed value of x, the y-limits are given by the intersection of the hyperbola xy = 2 with the line x = const. This intersection occurs at y = 2/x, so the limits of integration for y are:
2/x ≤ y ≤ ∞
To find the limits of integration for x, we can note that the hyperbola xy = 2 is symmetric about the line y = x.
So we can integrate over the region where \(x \geq \sqrt(2)\) and then multiply the result by 2. Thus, the limits of integration for x are:
\(\sqrt(2)\) ≤ x ≤ ∞
Putting everything together, we have:
V =\(2\int \sqrt(2)\infty \int 2/x \infty \int 0^1\)dz dy dx
Integrating over z gives:
V = \(2\int \sqrt(2) \infty \int 2/x \infty z|0^1 dy dx = 2\int \sqrt(2)\infty \int 2/x \infty dy dx\)
Integrating over y gives:
\(V = 2\int \sqrt(2)\infty [y]2/x\infty dx = 2\int \sqrt(2)\infty (2/x - 2/\sqrt(2)) dx\)
\(= 4\int \sqrt(2)\infty (1/x - 1/\sqrt(2)) dx\)
= \(4(ln(x) - \sqrt(2) ln(x)|\sqrt(2)\infty)\)
= \(4(ln(\sqrt(2)) - \sqrt(2) ln(\sqrt(2))) = 4(1 - \sqrt(2)/2) = 2(2 - \sqrt(2))\)
Therefore, the volume of the region E is 2(\(2 - \sqrt(2)\)).
Learn more about volume of the region
brainly.com/question/15166233
#SPJ11
Janelle spots some dolphins in the ocean. Her father draws a diagram to show how far down they can go. How far is this relative to sea level? What would an altimeter read at this depth?
Answers
The diagram is missing, so i have attached it.
Answer:
-1000 ft
Step-by-step explanation:
From the diagram attached, we see that they went 1000 ft below the sea level.
Now an altimeter is used to measure altitude depth of an object relative to a fixed level which in this case the fixed level is the top of sea. Since the depth in this case is below sea level, it will be negative.
Thus,the altimeter will read -1000 ft

Answer:
The dolphins are shown 1,000 feet below sea level. The altimeter reading would be -1,000 feet.
Step-by-step explanation:
What additional information is needed to prove the triangles are congruent by side-angle-side?
Answers
The corresponding angles and sides of the two triangles need to be given in order to prove congruence by side-angle-side.
In order to prove congruence by side-angle-side, the corresponding angles and sides of the two triangles must be given. The sides must be proportional, which means that two sides of one triangle must be equal to two sides of the other triangle, and the angles between these two sides must also be equal. In other words, if two sides of one triangle are equal to two sides of the other triangle, and the angle between those two sides is also equal, then the two triangles are congruent. This can be expressed as “If two sides and the included angle of one triangle are equal to the corresponding sides and angle of a second triangle, then the two triangles are congruent.” In order to prove congruence by side-angle-side, all of the corresponding sides and angles must be given in order to show that they are equal.
Learn more about triangle here
https://brainly.com/question/2773823
#SPJ4
The additional information that is needed to prove the triangles are congruent by Side-Angle -Side us that the corresponding angles and sides of the two triangles need to be given .
What is Side-Angle-Side Congruence ?
The Two Triangles can be called as Congruent by Side-Angle-Side rule if any 2 sides and angle included between sides of one triangle is equivalent to corresponding 2 sides and angle between sides of second triangle .
In order to prove the Congruence by Side-Angle-Side, the corresponding angles and the sides of the 2 triangles must be given.
The sides must also be in proportional, that means that the two sides of one triangle must be equal to two sides of other triangle, and
the angles between these two sides of the triangle must be equal.
So , In order to prove a triangle congruent by Side-Angle-Side, all of the corresponding sides and angles must be given in order to prove that they are equal.
Learn more about Congruency here
https://brainly.com/question/29288158
#SPJ4
Calculate the VOLUME of the cone. (Round to the nearest tenth.)

Answers
The volume of the cone with a slant height of 8 cm and radius of 6 cm is approximately 199.5 cm³.
What is the volume of the cone?A cone is simply a 3-dimensional geometric shape with a flat base and a curved surface pointed towards the top.
The volume of a cone can be expressed as;
V = (1/3)πr² × √( l² - r² )
Where r is radius of the base, l is the slant height of the cone and π is constant pi.
From the diagram:
Radius r = 6cm
Slant height = 8 cm
Volume V = ?
Plug the given values into the above formula and solve for the volume:
V = (1/3)πr² × √( l² - r² )
V = (1/3)π × 6² × √( 8² - 6² )
V = (1/3)π × 36 × √( 64 - 36 )
V = (1/3)π × 36 × √28
V = 199.5 cm³
Therefore, the volume of the cone is 199.5 cm³.
Learn more on volume of cones here: brainly.com/question/1984638
#SPJ1
Please send an explanation.

Answers
==========================================================
Explanation:
The ratio of WX:XY is given to be 2:1. Let's double each part to get 4:2. Those two parts add to 4+2 = 6.
The ratio WY:YZ is 2:5. If we triple each part, then we get 6:15. The '6' here matches from the 6 we found earlier.
So the WX+XY = 4+2 = 6 matches with the WY = 6 in the second ratio. This means XY:YZ = 2:15
----------
Check out the diagram below. It represents a visual way to see how we got the answer. I'm using boxes to represent each smallest unit or part. Chaining together boxes forms the lines or curves. The WX portion is in blue consisting of 4 parts, XY is in green (2 parts), and YZ is in orange (15 parts).
The diagram shows that WX:XY = 4:2 = 2:1, and that WY:YZ = 6:15 = 2:5
Furthermore, it shows that XY:YZ = 2:15

Use the Ratio Test to determine whether the series is convergent or divergent. 8 (-7)" n² n=1 Identify an Evaluate the following limit. a lim n+ 1 n18 Since lim 318 n+1 an an ? 1, -Select---
Answers
The series 8 * (-7)^(n^2) n=1 is divergent according to the Ratio Test. The limit lim (n+1)/(n^18) as n approaches infinity is equal to 1.
To determine the convergence or divergence of the series 8 * (-7)^(n^2) n=1, we can use the Ratio Test. The Ratio Test states that if the limit of the absolute value of the ratio of consecutive terms in a series is less than 1, then the series is convergent.
If the limit is greater than 1 or equal to infinity, then the series is divergent.
Let's apply the Ratio Test to the given series:
a_n = 8 * (-7)^(n^2)
We calculate the ratio of consecutive terms:
|a_n+1 / a_n| = |8 * (-7)^((n+1)^2) / (8 * (-7)^(n^2))|
= |-7 * (-7)^(2n+1) / (-7)^(n^2)|
= 7 * |(-7)^(2n+1) / (-7)^(n^2)|
Simplifying the expression, we have:
|a_n+1 / a_n| = 7 * |(-7)^(2n+1 - n^2)| = 7 * |-7^(2n+1 - n^2)|
Now, let's evaluate the limit as n approaches infinity:
lim (n+1)/(n^18) = 1
Since the limit is equal to 1, according to the Ratio Test, the series 8 * (-7)^(n^2) n=1 is divergent.
Learn more about ratio test:
https://brainly.com/question/31700436
#SPJ11
Simplify.
8r(4 - p)
32r -p
32r - 8rp
32 - rp
4 - 8rp
helppppppppppppppppppppppppppppppppppppppppppppppppp
Answers
Answer:
Second option: 32r - 8rp
Step-by-step explanation:
When you expand 8r(4-p) you get 32r - 8rp.
Answer:
= 32r - 8rp
Step-by-step explanation:
Distributive property:
8r(4 - p)
= 32r - 8rp
Steps:
8r · 4 = 32r
8r · (-p) = -8rp
= 32r - 8rp
Is (–39, 42) a solution to the equation y = x + 81?
Answers
Answer:
Yes
Step-by-step explanation:
Yes because if you insert -39 for x and 42 for y then your equation is:
42 = -39 + 81
If you were to solve this equation it would be true.
Find solutions for your homework
science
physics
physics questions and answers
there is a bar with an uneven mass distribution.the length of the
Question: There Is A Bar With An Uneven Mass Distribution.The Length Of The
There is a bar with an uneven mass distribution.The length of the bar is l and the density is as follows.
λ = A cos(πx/2l)
x is the distance from the left end of the bar and 0 ≤ x ≤ l
(a) Find the mass M of this bar
(b) Find the position of the center of mass of this bar
Answers
a. the mass of the bar is M = 2A/π. b. the position of the center of mass of the bar is x_cm = (2 - 2/π) / π.
(a) To find the mass M of the bar, we need to integrate the density function λ(x) over the length of the bar.
The density function is given as:
λ = A cos(πx/2l)
To find the mass, we integrate λ(x) over the length of the bar:
M = ∫λ(x) dx
Using the given density function, we have:
M = ∫(A cos(πx/2l)) dx
Integrating, we get:
M = A ∫cos(πx/2l) dx
To evaluate this integral, we can use the substitution u = πx/2l, du = π/2l dx:
M = (2A/π) ∫cos(u) du
M = (2A/π) sin(u) + C
Substituting back u = πx/2l, we have:
M = (2A/π) sin(πx/2l) + C
Since we're interested in the mass of the entire bar from x = 0 to x = l, we evaluate M at these limits:
M = (2A/π) sin(π(l)/2l) - (2A/π) sin(π(0)/2l)
M = (2A/π) sin(π/2) - (2A/π) sin(0)
M = (2A/π) - 0
M = 2A/π
Therefore, the mass of the bar is M = 2A/π.
(b) To find the position of the center of mass of the bar, we need to calculate the average position of the mass distribution. We can do this by finding the weighted average of the positions along the bar.
The position x_cm of the center of mass is given by:
x_cm = (∫xλ(x) dx) / (∫λ(x) dx)
Using the given density function, we have:
x_cm = (∫x(A cos(πx/2l)) dx) / (∫(A cos(πx/2l)) dx)
To evaluate these integrals, we can use integration by parts. Let's denote u = x and dv = A cos(πx/2l) dx. Then du = dx and v = (2A/π) sin(πx/2l):
x_cm = [x(2A/π) sin(πx/2l)] - ∫[(2A/π) sin(πx/2l)] dx / (∫(A cos(πx/2l)) dx)
Simplifying the integrals, we have:
x_cm = [x(2A/π) sin(πx/2l)] - [(2A/π^2) cos(πx/2l)] / [A sin(πx/2l)]
Now, let's evaluate x_cm at the limits x = 0 to x = l:
x_cm = [l(2A/π) sin(πl/2l)] - [(2A/π^2) cos(πl/2l)] / [A sin(πl/2l)]
x_cm = [2A/π] sin(π/2) - [(2A/π^2) cos(π/2)] / [A sin(π/2)]
x_cm = [2A/π] - (2A/π^2) / A
x_cm = [2/π] - 2/π^2
x_cm = (2 - 2/π) / π
Therefore, the position of the center of mass of the bar is x_cm = (2 - 2/π) / π.
Learn more about mass here
https://brainly.com/question/19385703
#SPJ11
Which animal's heart beats at a rate of 65 beats per minute?
Answers
Answer:
A cow
Step-by-step explanation:
What is the slope-intercept form of the following linear equation: 8x - by = 48 ?
Answers
either roll or a 7 or 11 on the first roll, or match the point value on a subsequent roll. what's the likelihood of winning after one roll? explain your answer.
Answers
The likelihood of winning after one roll in a game is 1/6. This is because there are only two ways to win on the first roll: by rolling a 7 or an 11.
What is Probability?The possibility that an event will occur is gauged by probability. It is a mathematical idea that measures the probability or possibility that a specific event will occur. Probability is expressed as a number between 0 and 1, where 0 denotes that the event is highly unlikely to occur and 1 denotes that it will definitely happen. Making predictions and judgments based on the likelihood of an event occurring is a common use of probability in many disciplines, including statistics, economics, and gambling. The number of successful outcomes is divided by the total number of possible outcomes to determine probability. For example, if a coin is flipped and has a 50% chance of landing on heads, the probability of the coin falling on chiefs is 0.5.
How to solve?
Since there are 6 possible outcomes on a single roll of a pair of dice (1, 2, 3, 4, 5, 6), the likelihood of winning on the first roll is 1/6.
To learn more about probability, visit:
https://brainly.com/question/20164508
#SPJ4
What is the range of the function g(x) = |x – 12| – 2?
{y | y > –2}
{y | y > –2}
{y | y > 12}
{y | y > 12}
Answers
The range of the function g(x) = |x - 12| - 2 is {y | y > -2}, indicating that the function can take any value greater than -2.
To find the range of the function g(x) = |x - 12| - 2, we need to determine the set of all possible values that the function can take.
The absolute value function |x - 12| represents the distance between x and 12 on the number line. Since the absolute value always results in a non-negative value, the expression |x - 12| will always be greater than or equal to 0.
By subtracting 2 from |x - 12|, we shift the entire range downward by 2 units. This means that the minimum value of g(x) will be -2.
Therefore, the range of g(x) can be written as {y | y > -2}, which means that the function can take any value greater than -2. In other words, the range includes all real numbers greater than -2.
Visually, if we were to plot the graph of g(x), it would be a V-shaped graph with the vertex at (12, -2) and the arms extending upward infinitely. The function will never be less than -2 since we are subtracting 2 from the absolute value.
for similar questions on range of the function.
https://brainly.com/question/29017978
#SPJ8
A customer wants to purchase a sweater from a store.
- The original price of the sweater is $40.
- The sweater is on sale for 10% off the original price.
- The customer has a coupon for 25% off the sale price.
The customer claims that she can determine the final price of the sweater by taking 35% off the original price since 10%+25%=35%. Which of the following statements is true?
A) The customer’s claim is correct. The final price of the sweater will be $5.
B) The customer’s claim is correct. The final price of the sweater will be $26.
C) The customer’s claim is incorrect. The final price of the sweater will be $27.
D) The customer’s claim is incorrect. The final price of the sweater will be $30.
Answers
Answer:
400
Step-by-step explanation:
sale for 10% off the original price.
- The customer has a coupon for 25% off the sale price.
The customer claims that she can determine the final price of the sweater by taking 35% off the original price since 10%+25%=35%. Which of the following statements is true?
A) The customer’s claim is correct. The final price of the sweater will be $5.
B) The customer’s claim is correct. The final price of the sweater will be $26.
C) The customer’s claim is incorrect. The final price of the sweater will be $27.
D) The customer’s claim is incorrect. The final price of the sweater will be $30.
= 400
Answer:
C) The customer’s claim is incorrect. The final price of the sweater will be $27.
Find the value of x will give brainliest need answer soon

Answers
Answer:
\(\frac{135 - 5x}{2}= 45\\\\135 - 5x = 90 \\\\5x = 90-135\\\\5x = 45\\ x= 9\)
Option B
Work out the value of x . The diagram is not drawn to scale.
Answers
After calculating the angles, we know that the value of x is (C) 99°.
What are angles?An angle is formed when two straight lines or rays meet at a single terminal.
The place where two points converge is known as an angle's vertex.
The name "angel" comes from the Latin word "angulus," which means "corner."
Angles are also produced when two planes intersect.
These are what are known as dihedral angles.
So, according to the given figure of the diagram:
We can say that:
y + 116 = 180
y = 180 - 116
y = 64°
Now, calculate for x inside the given figure:
125 + 72 + 64 + x = 360
261 + x = 360
x = 360 - 261
x = 99°
Therefore, after calculating the angles, we know that the value of x is (C) 99°.
Know more about angles here:
https://brainly.com/question/29276964
#SPJ1

3 + 3t - 3t - 3 *
i need a help again algebra asap
Answers
\(\text{Hey there!}\)
\(\bold{3 + 3t - 3t - 3}\\\bold{GROUP/COMBINE\ your\ LIKE\ TERMS}\\\bold{(3t-3t)+(3-3)}\\\bold{3t-3t=0}\\\bold{3-3=0}\\\bold{0=0}}\\\boxed{\boxed{\bold{Answer: 0}}}\huge\checkmark\)
\(\text{Good luck on your assignment and enjoy your day!}\\\\\frak{Amphitrite1040:)}}\)
45/27=x/3 solve for x pls help
Answers
Answer:
x = 5
Step-by-step explanation:
hope this helps!!
Answer:
x=5
Step-by-step explanation:
5/3=45/27
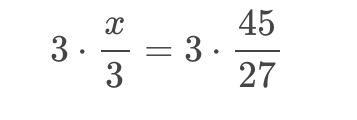
what is the probability that a person selected randomly from the survey group does not have a two-handed backhand swing, given that the person is male, or p(no two-handed backhand/male)?
Answers
The probability that a person selected randomly from the survey group does not have a two-handed backhand swing, given that the person is male is given as follows:
P(no two-handed backhand/male) = 0.81 = 81%.
How to obtain a probability?The probability of an event in the context of an experiment is obtained as the number of desired outcomes of the experiment divided by the number of total outcomes of the experiment.
In the context of this problem, these amounts are given as follows:
Desired: No two-handed backhanded swing and male.Total: Male.The number of males that have a two-handed backhand is of:
256 - 176 = 80.
Hence the number that does not have is of:
421 - 80 = 341.
Then the probability is of:
p = 341/421 = 0.81 = 81%.
Missing InformationThe problem is given by the image at the end of the answer.
More can be learned about probabilities at https://brainly.com/question/14398287
#SPJ1

FIND THE LENGTH AND WIDTH OF THE RECTANGLE

Answers
3w + 7 = length
Equation: 3w + 7 + w = 75
Combine like terms: 4w + 7 = 75
Subtract 7 to the other side: 4w = 68
Divide both sides by 4: w = 17
Width = 17
Length = 3(17) + 7, so 58
Answer:
w=7 5/8
l = 29.875
Step-by-step explanation:
We have to use system of equations here
Let l be length and w be width
l = 3w+7
w=l(1/3)-7
We can use the equation:
l+w+l+w = 75
2l+2w = 75
Now we substitute l for what we said above
2(3w+7)+2w=75
6w+14+2w=75
Combine like terms
8w+14=75
Subtract 14 on both sides
8w=61
w=7 5/8
We can use what we know about the relationship of the width and the length to find the length
7 5/8 * 3 + 7 = 29.875
l = 29.875
What are the first 10 Pythagorean triples?
Answers
The first 10 Pythagorean triples are: (3, 4, 5), (5, 12, 13), (8, 15, 17), (7, 24, 25), (20, 21, 29), (12, 35, 37), (9, 40, 41), (28, 45, 53), (11, 60, 61), and (16, 63, 65).
Pythagorean triples are sets of three positive integers that satisfy the Pythagorean theorem, which states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. The first 10 Pythagorean triples, listed in ascending order of their hypotenuse lengths, are (3, 4, 5), (5, 12, 13), (8, 15, 17), (7, 24, 25), (20, 21, 29), (12, 35, 37), (9, 40, 41), (28, 45, 53), (11, 60, 61), and (16, 63, 65). There are infinitely many Pythagorean triples, and they have important applications in mathematics, physics, and engineering.
Learn more about Pythagorean triples here: brainly.com/question/15190643
#SPJ4
jhhhhhhhhhhhhhhhhheeeeeeeeeeeeeeeeeeeeeeeeeelllllllllllllllllllllllppppppppppppppp

Answers
Answer:
pick D
Step-by-step explanation:
18 degrees
10% of 360 degrees is 36%
5% of 360 degrees is 18%
18°
It is an acute angle. Hope this helped
3075677532 yesyeysyeydsyeysys
Answers
Answer: What? What are you talking about?
y=2x-7 in point slope form
Answers
Answer:
This answer is y -7x.Hope you like answer
The point-slope form of the equation y=2x-7 will be y + 7 = 2(x - 0).
What is an equation of the line?An equation of the line is defined as a linear equation having a degree of one. The equation of the line contains two variables x and y. And the third parameter is the slope of the line which represents the elevation of the line.
Given that the equation of the line is y = 2x - 7. The point-slope form of the equation of the line will be written as:-
y = 2x - 7
y - (-7) = 2( x - 0 )
y + 7 = 2( x - 0 )
Therefore, the point-slope form of the equation y=2x-7 will be y + 7 = 2(x - 0).
To know more about an equation of the line follow
brainly.com/question/18831322
#SPJ5
Suppose that dollars in principal is invested for years at the given interest rates with continuous compounding. determine the amount that the investment is worth at the end of the given time period. p=1$2000, t=10yr
Answers
Amount after 10 years at 3%, 4% and 5.5% interest with principal $20000 is $26878.32, $29604.88 & $34162.88 respectively.
Although a part of your question is missing, you might be referring to this full question: Suppose that P dollars in principal are invested for years at the given interest rates with continuous compounding. Determine the amount that the investment is worth at the end of the given time period. p=$20000, t=10yr
a. 3% interest
b. 4% interest
c. 5.5% interest
P = $2000
T = 10 years
Formula to be used:
A = P(1+r/100)^n
A = total amount after n years
P = principal amount invested
r = rate of interest
n = number of years the money is invested
Solving for part a:
A = 20000(1+3/100)^10
= 20000*1.34
A = 26878.32
Solving for part b:
A = 20000(1+4/100)^10
= 20000*1.48
A = 29604.88
Solving for part c:
A = 20000(1+5.5/100)^10
= 20000*1.70
A = 34162.88
Learn more about compound interest:
https://brainly.com/question/14295570
#SPJ4
Students in a statistics class took their second test. The following are the scores they earned. Fill in the stem-and-leaf plot below use the tens place as the stem and the ones place as the leaf. Describe the shape of the distribution.
Answers
Data were collected for 1 quantitative variable(s). yes, It is appropriate to say that a stem and leaf plot for this type of data. The stem and leaf plot has right skewed shape curve.
From the above data that were collected for one quantitative variable. Yes, it is appropriate to say that to make a stem and leaf for this type of data and number of variables.
Stems | Leaves
5 | 2, 6, 1, 2, 4, 8, 0, 9, 7
6 | 7, 7, 5, 2, 0, 5, 8 , 8
7 | 8, 4, 7, 1 and 8
8 | 9 , 4, 8
9 | 8, 9
Also, the shape of the stem and leaf plot is right skewed curve.
To learn more about quantitative :
https://brainly.com/question/29787432
#SPJ4
If a cantaloupe that weighs 3.0 pounds costs $3.87, approximately how much will a 2.22 pound cantaloupe cost?
Answers
Answer:\(1.29 * 2.22 = 2.86\)
$2.86
Step-by-step explanation:
First we need to find the price per pound for the cantaloupe
\(3.87/3.0=1.29\)
So one pound of cantaloupe costs $1.29
Now multiply the price per pound by the weight of the second cantaloupe
\(1.29*2.22=2.86\)
So the price for a cantaloupe that weighs 2.22 lbs would be $2.86
Fill in the blank. When a data value is converted to a standardized scale representing the number of standard deviations the data value lies from the mean, we call the new value a _____ . When a data value is converted to a standardized scale representing the number of standard deviations the data value lies from the mean, we call the new value a ____.
Answers
When a data value is converted to a standardized scale representing the number of standard deviations the data value lies from the mean, we call the new value a Z-Score.
In statistics, each mathematical formula and each element has an internationally recognised name. This is to ensure that everyone in the world understands what every researcher has to say in every letter. A statistic that tells the number of standard deviations a data value is above or below the mean is called a z-score. And its formula is, Z = (X − μ)/σ
where, μ--> population mean,
σ --> population standard deviation and
x --> the raw-score.
Hence, when a data value is converted to a standardized scale, new value, Z-Score is representing the number of standard deviations the data value lies from the mean.
To learn more about Z-Score, refer:
https://brainly.com/question/25638875
#SPJ4
Which numbers are solutions to the inequality 2x – 1 > x 2? check all of the boxes that apply.
Answers
Solutions to the given inequality are numbers 4 and 5. Thus, the answer is (e) and (f).
To find the solution, first, we solve the inequality for x:
2x - 1 > x + 2
2x - x > 2 + 1
x > 3
Hence, the answer is any number that is greater than 3.
Therefore, the solutions are numbers 4 and 5.
To learn more about solving inequality:
https://brainly.com/question/11897796
The complete question is:
Which numbers are solutions to the inequality 2x - 1 > x + 2? Check all of the boxes that apply.
(a) 0
(b) 1
(c) 2
(d) 3
(e) 4
(f) 5