Lisa wants to arrange the numbers below in order from LEAST to GREATEST. 1/5, -0.3, 0.01,-1/-4 Which of these represents her list?
Answers
-0.3, 0.01, 1/5, -1/-4
Answer:-.3 , .01 , 1/5 , -1/-4
Step-by-step explanation:
Related Questions
In a basketball game, Alana scores twice as many points as Taylor. Taylor scores four points fewer than Nancy, and Nancy scores three
times as many points as Molly. If Molly scores 6 points, how many points did Alana score?
Determine your answer by first determining how many points each other player scored:
1. Nancy scores
2. Taylor sco res
3. Alana scores
points.
points.
points.
Answers
1. Molly scores 6 points.
2. Nancy scores three times as many points as Molly, so Nancy scores 3 * 6 = 18 points.
3. Taylor scores four points fewer than Nancy, so Taylor scores 18 - 4 = 14 points.
4. Alana scores twice as many points as Taylor, so Alana scores 2 * 14 = 28 points.
Therefore, Alana scored 28 points in the basketball game.
Let Vi = x ℝ − 1 i ≤ x ≤ 1 i = − 1 i , 1 i for each positive integer i. Find each of the following. (Enter your answers using interval notation.) (a) ∪4i = 1Vi = (b) ∩4i = 1Vi = (c) Are V1, V2, V3, mutually disjoint? Explain. a.) Yes, because the intersection of the sets V1, V2, V3, ... is empty. b.) Yes, because no two of the sets V1, V2, V3, ... have any elements in common. c.) Yes, because the union of the sets V1, V2, V3, ... is empty. d.) No, because no two of the sets V1, V2, V3, ... are disjoint. e.) No, because the sets V1, V2, V3, ... are disjoint. (d) ∪ni = 1Vi = (e) ∩ni = 1Vi = (f) ∪[infinity]i = 1Vi = (g) ∩[infinity]i = 1Vi =
Answers
(a) The union of V1, V2, V3, and V4 is the set {x}.
(b) The intersection of V1, V2, V3, and V4 is the interval [-1, x].
(c) No, V1, V2, and V3 are not mutually disjoint.
(d) The union of all Vi for positive integer i from 1 to n is the interval [-1, x].
(e) The intersection of all Vi for positive integer i from 1 to n is the interval [-1, x].
(f) The union of an infinite number of Vi is the interval [-1, x].
(g) The intersection of an infinite number of Vi is the interval [-1, x].
We have,
Let's solve each part of the question:
(a) ∪ (i = 1 to 4) Vi:
We have V1 = [-1, x], V2 = [-1, x], V3 = [-1, x], and V4 = [-1, x].
To find the union, we need to consider the maximum range for x across all intervals.
The maximum range for x is [x, x] = {x}.
Therefore, ∪(i = 1 to 4) Vi = {x}.
(b) ∩ (i = 1 to 4) Vi:
To find the intersection, we need to consider the minimum range for x across all intervals.
The minimum range for x is [-1, x].
Therefore, ∩ (i = 1 to 4) Vi = [-1, x].
(c) Are V1, V2, V3 mutually disjoint?
No, V1, V2, and V3 are not mutually disjoint.
Since their intervals overlap, they share common elements.
(d) ∪ (i = 1 to n) Vi:
In this case, n is a positive integer.
The union of all Vi from i = 1 to n will be the maximum range for x across all intervals.
The maximum range for x is [-1, x].
Therefore, ∪ni=1 Vi = [-1, x].
(e) ∩ (i = 1 to n) Vi:
In this case, n is a positive integer.
The intersection of all Vi from i = 1 to n will be the minimum range for x across all intervals.
The minimum range for x is [-1, x].
Therefore, ∩ (i = 1 to n) Vi = [-1, x].
(f) ∪ (i = 1 to ∞) Vi:
When we take the union of an infinite number of intervals, we consider the maximum range for x across all intervals.
The maximum range for x is [-1, x].
Therefore, ∪ (i = 1 to ∞) Vi = [-1, x].
(g) ∩ (i = 1 to ∞) Vi:
When we take the intersection of an infinite number of intervals, we consider the minimum range for x across all intervals.
The minimum range for x is [-1, x].
Therefore, ∩ (i = 1 to ∞) Vi = [-1, x].
Thus,
(a) The union of V1, V2, V3, and V4 is the set {x}.
(b) The intersection of V1, V2, V3, and V4 is the interval [-1, x].
(c) No, V1, V2, and V3 are not mutually disjoint.
(d) The union of all Vi for positive integer i from 1 to n is the interval [-1, x].
(e) The intersection of all Vi for positive integer i from 1 to n is the interval [-1, x].
(f) The union of an infinite number of Vi is the interval [-1, x].
(g) The intersection of an infinite number of Vi is the interval [-1, x].
Learn more about sets here:
https://brainly.com/question/8053622
#SPJ12
a spinner is spun and a number cube is rolled. what is the probability that a blue will be spun on the spinner and a four will be rolled on the number cube?
Answers
Answer:1/24
Step-by-step explanation:A spinner has 4 sections and a number cube has 6 sides. the probability of getting blue on the spinner is 1/4 and the probability of getting a 4 on a number cube is 1/6. So 1/6×1/4=1/24
Factorize:
6 + 13mn + 7m² n²
explanation
Answers
Answer:
1(6+13mn+7m^2n^2)
Step-by-step explanation:
The coefficients and variables in this equation have a common factor of 1, therefore the equation will go back to it's original state if you use 1 as the common factor.
I hope this helps and sorry if am wrong
Differentiate the function with respect to x
f(x)= x^4/ x^2- 4
Show work
Answers
Answer:
Step-by-step explanation:
\(f(x)=\frac{u}{v} ,u~ and~ v~ functions~ of~ x\\f'(x)=\frac{v ~u'-u~v' }{v^2} \\f(x)=\frac{x^4}{x^2-4} \\f'(x)=\frac{(x^2-4)*4x^3-x^4(2x)}{(x^2-4)^2} \\=\frac{2x^3(2x^2-8-x^2) }{(x^2-4)^2} \\=\frac{2x^3(x^2-8)}{(x^2-4)^2}\)
Point B is the midpoint of AC. If AB=10 and AC=2x-4, find x
Answers
x=12. The average midpoint of AC is located halfway between the two endpoints, so AB must be equal to half of AC. Therefore, AC=2x-4 and AB=10, resulting in 2x-4=10, which yields x=12.
The midpoint of a line segment is located at the exact center of the line segment, so the two sides of the line segment must be equal in length. In this case, Point B is the midpoint of AC, which means that AB must be equal to half of AC. To find x, we can set up the equation 2x-4=10, which gives us x=12. This means that AC=2(12)-4=20-4=16, and since we know that AB=10, we can confirm that the midpoint of AC is located between the two endpoints. Therefore, Point B is the midpoint of AC and x=12.
The midpoint of a line segment is an important concept in geometry because it allows us to find the midpoint of a line segment without having to measure the entire length of the line. By setting up a simple equation and solving for x, we can easily find the midpoint of AC. Furthermore, this equation can be used to find the midpoint of any line segment, allowing us to quickly and accurately measure distances.
Learn more about average here
https://brainly.com/question/24057012
#SPJ4
Plz help will give 100 points

Answers
Answer: i think is d not sure tho
Step-by-step explanation:
Shawn saw a frisbee on sale for $15.99. It was originally priced at $19.99. What is the approximate percent discount on the frisbee?
Answers
Butt Koko, this is the solution:
Original price = 19.99
Sale price = 15.99
Discount = 19.99 - 15.99 = 4.00
We can use Direct Rule of Three to calculate the percent of discount, this way:
Price Percent
19.99 100
4.00 x
___________________
4 * 100 = 19.99 * x
400 = 19.99x
x = 400/19.99
x = 20.01
The approximate percent discount on the frisbee is 20%
Larry's age four years ago is a quarter of Kelly's current age.
Kelly was born 8 years before Larry. How old will Larry be two
years from now?
Answers
Larry's age two years from now = 8 + 2 = 10
In two years, Larry will turn 10 years old.
Let's break down the information given step by step to find Larry's current age and determine how old he will be two years from now.
"Kelly is now a quarter Larry's age from four years ago."
Let's assume Larry's age four years ago was L. And Kelly's current age is K.
According to the given information, we can write the equation:
L - 4 = (1/4)K
"Kelly was born 8 years before Larry."
This means that the age difference between Larry and Kelly is 8 years.
K = L + 8
We can solve this system of equations to find the current ages of Larry and Kelly.
When the second equation is used in place of the first equation:
L - 4 = (1/4)(L + 8)
Multiplying both sides of the equation by 4 to eliminate the fraction:
4L - 16 = L + 8
Subtracting L from both sides of the equation:
3L - 16 = 8
Add 16 to both sides of the equation:
3L = 24
Dividing both sides of the equation by 3:
L = 8
Now that we know Larry's current age is 8, we can calculate his age two years from now:
Larry's age two years from now = 8 + 2 = 10
Therefore, Larry will be 10 years old two years from now.
For such more questions on Larry's Future Age
https://brainly.com/question/22802205
#SPJ8
3. Suppose the curve x = t³ - 9t, y = t + 3 for 1 ≤ t ≤ 2 is rotated about the x-axis. Set up (but do not evaluate) the integral for the surface area that is generated.
Answers
The integral for the surface area generated by rotating the curve x = t³ - 9t, y = t + 3 for 1 ≤ t ≤ 2 about the x-axis can be set up as follows.
First, we divide the interval [1, 2] into small subintervals. Each subinterval is represented by Δt. For each Δt, we consider a small segment of the curve and approximate it as a straight line segment.
We then rotate this line segment about the x-axis to form a small section of the surface. The surface area of each small section is given by 2πyΔs, where y is the height of the line segment and Δs is the length of the arc.
By summing up the contributions of all the small sections, we can set up the integral for the total surface area.
To explain further, we can consider a small subinterval [t, t + Δt]. The corresponding line segment can be approximated by connecting the points (t, t + 3) and (t + Δt, t + Δt + 3).
The height of this line segment is given by the difference in the y-coordinates, which is Δy = Δt.
The length of the arc can be approximated as Δs ≈ √(Δx)² + (Δy)², where Δx is the difference in the x-coordinates, given by Δx = (t + Δt)³ - 9(t + Δt) - (t³ - 9t).
We then multiply the surface area of each small section by 2π to account for the rotation around the x-axis. Finally, we integrate over the interval [1, 2] to obtain the total surface area.
Learn ore about integral here: brainly.com/question/31433890
#SPJ11
The integral for the surface area generated by rotating the curve x = t³ - 9t, y = t + 3 for 1 ≤ t ≤ 2 about the x-axis can be set up as follows. Δx = (t + Δt)³ - 9(t + Δt) - (t³ - 9t).
First, we divide the interval [1, 2] into small subintervals. Each subinterval is represented by Δt. For each Δt, we consider a small segment of the curve and approximate it as a straight line segment.
We then rotate this line segment about the x-axis to form a small section of the surface. The surface area of each small section is given by 2πyΔs, where y is the height of the line segment and Δs is the length of the arc.
By summing up the contributions of all the small sections, we can set up the integral for the total surface area.
To explain further, we can consider a small subinterval [t, t + Δt]. The corresponding line segment can be approximated by connecting the points (t, t + 3) and (t + Δt, t + Δt + 3).
The height of this line segment is given by the difference in the y-coordinates, which is Δy = Δt.
The length of the arc can be approximated as Δs ≈ √(Δx)² + (Δy)², where Δx is the difference in the x-coordinates, given by Δx = (t + Δt)³ - 9(t + Δt) - (t³ - 9t).
We then multiply the surface area of each small section by 2π to account for the rotation around the x-axis. Finally, we integrate over the interval [1, 2] to obtain the total surface area.
Learn ore about integral here: brainly.com/question/31433890
#SPJ11
use cylindrical coordinates. evaluate x2 dv, e where e is the solid that lies within the cylinder x2 y2 = 4, above the plane z = 0, and below the cone z2 = 36x2 36y2.
Answers
Using cylindrical coordinates ∫∫∫ (r^3cos^2θ) dz dr dθ, where r ranges from 0 to 2, θ ranges from 0 to 2π, and z ranges from 0 to √(36r^2).
To evaluate the integral ∫∫∫ x^2 dV over the solid e, using cylindrical coordinates, we need to express the integral in terms of cylindrical coordinates and determine the appropriate bounds for the variables.
In cylindrical coordinates, the solid e can be defined as follows:
Radius: r ranges from 0 to 2 (from x^2 + y^2 = 4, taking the square root).
Angle: θ ranges from 0 to 2π (full revolution around the z-axis).
Height: z ranges from 0 to the height of the cone, which is determined by z^2 = 36x^2 + 36y^2.
To convert the integral, we need to express x^2 in terms of cylindrical coordinates:
x^2 = (rcosθ)^2 = r^2cos^2θ
The integral in cylindrical coordinates becomes:
∫∫∫ (r^2cos^2θ) r dz dr dθ
Now we can determine the bounds for the variables:
r ranges from 0 to 2.
θ ranges from 0 to 2π.
z ranges from 0 to the height of the cone, which can be determined by setting z^2 = 36r^2.
Substituting the bounds and integrating, we can evaluate the integral to find the desired result.
To know more about cylindrical coordinates refer here:
https://brainly.com/question/30394340
#SPJ11
word problem for 2/3 multiply 3 equals c
Answers
Answer:2
Step-by-step explanation:
ASAPPPP!!! CAN U START FROM SECTION A TO SECTION B!!!!????
LISTEN HERE I NEED SOMEONE TO WORKOUT ALL OF THESE CUZ IF I DONT FINISH IT BY TOMZ I GET A DETENTION !!

Answers
Answer: I just did the ones that did not already have an answer
Section A:
7. 1.5 hours
9. 480 meters
10. 10 km
Section B:
1. 40 mph
2. 160 miles
3. 168 miles
4. 5.2 mph
5. 26.25 km/h
How to solve right triangles with no sides or angles given.
Answers
Pls help me!!!!!!!!!!!

Answers
Answer:
1) y-4+1(x-5)
Step-by-step explanation:
With point-slope form, you just have to substitue the information that is given to you into the generic formula.
y-y1= m(x-x1)
so the y1 is the y value of the point that is given to you and x1 is the x value of the point that is given to you
m is the slope (also given)
and x and y just stay the same; x &y
What is the distance between (4, – 7) and (4, 2)?
2
4
5
9
Answers
Answer:
9
Step-by-step explanation:
2-9= -7 so the distance is 9
Answer:
7 units
Step-by-step explanation:
if ST = 10, what is RU
Answers
What does St mean or what’s the meaning of that
f(x)=1/3x+2 what x is equal to 6
Answers
Answer: To find the value of F(x) when x = 6, we can substitute x = 6 into the formula for F(x) and simplify:
F(x) = (1/3)x + 2
F(6) = (1/3)(6) + 2 [Substitute x = 6]
F(6) = 2 + 2 [Simplify]
F(6) = 4 [Simplify further]
Therefore, F(6) = 4 when F(x) = (1/3)x + 2.
Step-by-step explanation:
In the figure, m/3= (3x+15)° and mZ8= (x+100)°. Find the value of x. Then calculate the measure of Z4.

Answers
Value of x = 42.5 ° and measure of angle 4 = 37.5 ° .
measure of angle 3 = measure of angle 8
( 3 x + 15 ) ° = ( x + 100 ) °
2 x = 85
x = 42.5 °
measure of angle 3 + measure of angle 4 = 180 °
measure of angle 3 = ( 3 x + 15 ) °
= ( 3 * 42.5 ) + 15
= 142.5 °
Hence , measure of angle 4 = 180 - 142.5 ° = 37.5 ° .
To learn more on transversal follow link :
https://brainly.com/question/26961508
#SPJ9
need help plz its do in 5 min

Answers
Answer:
Answer is 120
Step-by-step explanation:
180*(6-2)/6
120
Write the equation of the line fully simplified slope-intercept form.
HELP ME PLEASE

Answers
The equation of line in slope intercept form is y = -0.5x -3.
What is slope intercept form?
The graph of the equation y = mx + b is a line with slope m and y-intercept b. The slope-intercept method is used to depict the linear equation, where m and b are real numbers.
We are given a line on the graph.
Taking two points as (2, -4) and (-2, -2), we get the slope as
⇒m = \(\frac{-2 +4 }{-2-2}\)
⇒m = \(\frac{2 }{-4}\)
⇒m = \(\frac{-1 }{2}\)
⇒m = -0.5
Similarly,
b = -3
We know that slope intercept form is given by
y = mx + b
On substituting, we get
y = -0.5x -3
Hence, the equation of line in slope intercept form is y = -0.5x -3.
Learn more about slope-intercept form from the given link
https://brainly.com/question/1884491
#SPJ1
PLS PLS i need step by step please and undefined numbers to be shown please THANK YOU!

Answers
1)The expression 4x^2-16x+12/x^2-9 is undefined when the denominator, x^2-9, equals zero because division by zero is undefined.
x^2-9 equals zero when x equals 3 or x equals -3. Therefore, the expression is undefined at x = 3 and x = -3. In all other cases, the expression is defined.
2) The given expression is:
(5-2x)/(x+2) + x^2/(x^2-4) - 5
To simplify this expression, we need to first find the LCD (least common denominator) of the two fractions. The denominator of the first fraction is x+2, and the denominator of the second fraction is x^2-4, which can be factored as (x+2)(x-2). So the LCD is (x+2)(x-2). Now we can rewrite the expression with this common denominator:
[(5-2x)(x-2) + x^2(x+2) - 5(x+2)(x-2)] / [(x+2)(x-2)]
Expanding the brackets and simplifying, we get:
(-x^3 - 3x^2 - 3x + 5) / [(x+2)(x-2)]
This expression is undefined when the denominator, (x+2)(x-2), equals zero because division by zero is undefined.
(x+2)(x-2) equals zero when x equals -2 or x equals 2. Therefore, the expression is undefined at x = -2 and x = 2. In all other cases, the expression is defined.
#SPJ1
the sum of 4 and the second power of b is equal to 104. write as an equation
Answers
Answer:
4 + b² = 104
Hope this helps. :)
Answer:
b^{2} + 4 = 104.
Step-by-step explanation:
The sum of 4 and the second power of b basically means 4 + b^2.
b^2 + 4 = 104
\(b^{2} + 4 = 104\)
b^2 = 100
b = plus or minus 10.
Hope this helps!
PLEASE HELP ITS DUE SOON! 40 points and i'll mark you as brainliest!! Also explain how you got the answer!!!

Answers
Answer: 6, 4.5
Step-by-step explanation:
The graph shows the amount of rain that falls over time. Does the rain fall at a constant or variable rate? How much rain falls per hour?
thanks!

Answers
Answer: yes, it does fall at a constant rate. for each hour there is one inch of rainfall.
Step-by-step explanation:
if the line goes the the origin (0,0) then that means that it is a constant rate. if you look at the chart, there is number on every other line. This means that there is an odd number on the other lines. If you then go up the line for 1 hour, then you will meet at lines intersecting to get 1 inch of rainfall per hour
please help help help meee

Answers
Answer:
Kaylaa do you use desmos graphing calculator? it's an online graphsing tool and would make this very easy for you. Maybe you are not allowed to use a calculator?
Step-by-step explanation:
with out a calculator it's a long math process, 1st because each of the equations are equal to Y, we can set each of them equal to each other. like this
\(\sqrt{x-8}\) + 3 = - \((x-5)^{2}\) + 18
\(\sqrt{x-8}\) = - \((x-5)^{2}\) +18 -3
\(\sqrt{x-8}\) = - \((x-5)^{2}\) + 15
\(\sqrt{x-8}\) = -[ (x-5)*(x-5) ] + 15
\(\sqrt{x-8}\) = -[ \(x^{2}\) - 10x + 25] +15
\(\sqrt{x-8}\) = -\(x^{2}\) + 10x -25 +15
\(\sqrt{x-8}\) = -\(x^{2}\) + 10x -10
- \(\sqrt{x-8}\) = \(x^{2}\) - 10x +10
(- \(\sqrt{x-8}\))^2 = ( \(x^{2}\)- 10x +10)^2
this turns into a 4 order polynomial. I used a solver to find x
x = 8.75884.... approx
so the question asks what is the point at the nearest 1000th, which is 8.759
then they ask what is the point at the nearest 100th, which is
8.76
I'm attaching the graph, if it helps :P

HELP!!! I don’t understand this question!
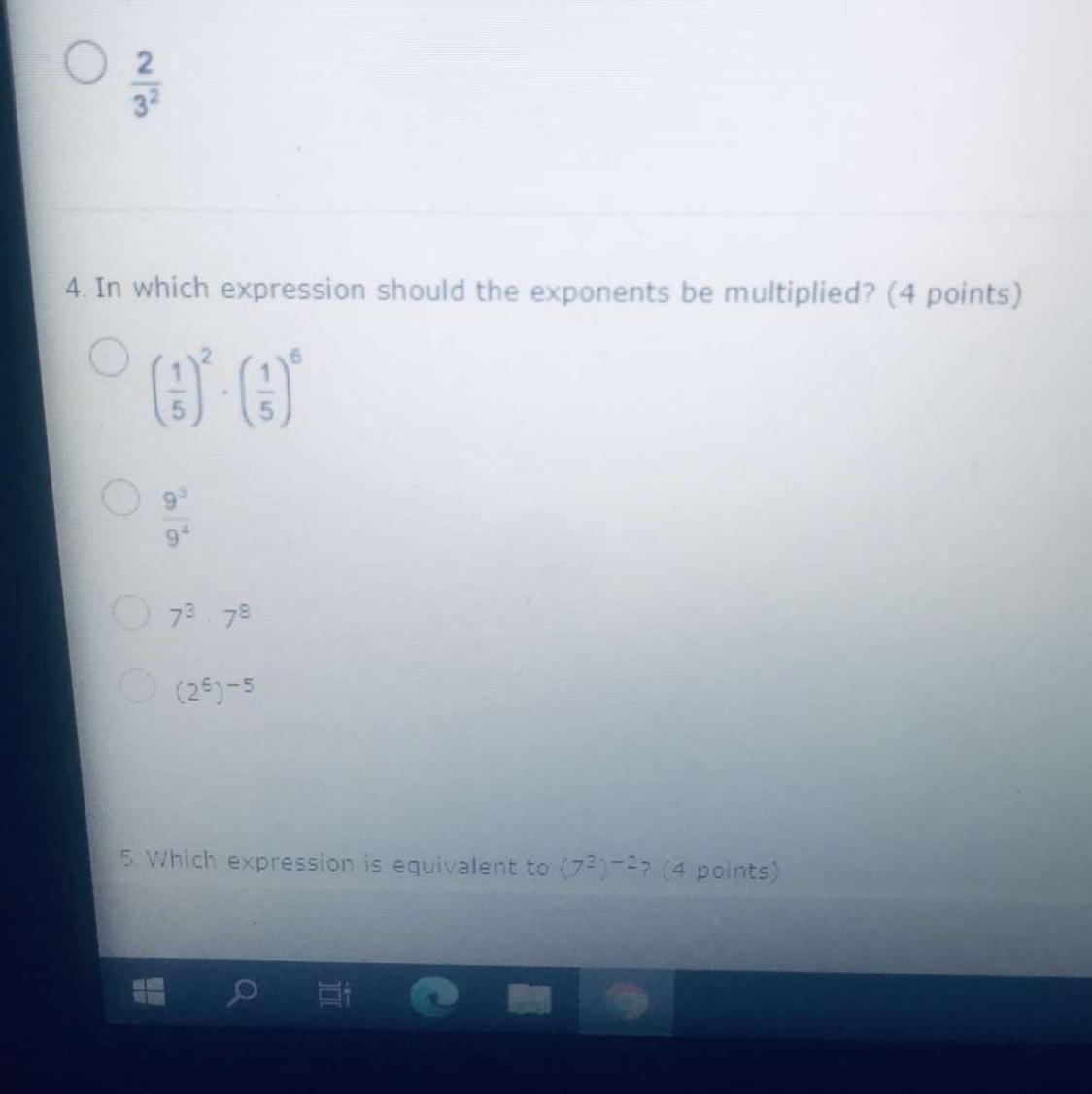
Answers
Answer:
Step-by-step explanation:
(2^6)^-5 is the expression that should be multiplied
Find the measures of a positive angle and a negative angle that are coterminal with each given angle
0=110
Answers
Answer:
Why was Mrs Hallette unhappy when people asked about her son?
Step-by-step explanation:
Ping lives at the corner of 3rd street and 6th avenue. ari lives at the corner of 21st street and 18th avenue. there is a gym the distance from ping's home to ari's home. where is the gym?
Answers
The distance between ping's home to aris's home will be 1 street and 12th avenue.
What is the arithmetic operator?Arithmetic operators are four basic mathematical operations in which summation, subtraction, division, and multiplication involve.,
Summation = addition of two or more numbers or variable
For example = 2 + 8 + 9
Subtraction = Minus of any two or more numbers with each other called subtraction.
For example = 4 - 8
Division = divide any two numbers or variable called division.
For example 4/8
Multiplication = to multiply any two or more numbers or variables called multiplication.
For example 5 × 7.
Given,
Ping house ⇒ 3rd street and 6th avenue
Ari's home ⇒ 21st street and 18th avenue
So, the distance between them
21 - 3 = 18 street
18 - 6 = 12 avenue.
Hence "The distance between ping's home to aris's home will be 1 street and 12th avenue".
To learn more about the arithmetic operators,
brainly.com/question/25834626
#SPJ4
Assignment Scoring Your last submission is used for your score. 1. + -/1 points SPreCalc7 3.7.033. My Notes Solve the inequality. (Enter your answer using interval notation.) 72 - 72 > 1 X-1 X Need Help? Read It Talk to a Tutor 2. + -/1 points SPreCalc7 3.7.037. My Notes Find all values of x for which the graph of Flies above the graph of g. (Enter your answer using interval notation.) f(x) = x2; 9(x) = 2x + 48 Need Help? Read It Talk to a Tutor 3. -/1 points SPreCalc7 3.7.041. My Notes Find the domain of the given function. (Enter your answer using interval notation.) f(x) = 72 + x - x2
Answers
domain of the function is (-∞, ∞)
When answering questions on the Brainly platform, it is important to always be factually accurate, professional, and friendly. In addition, you should be concise and provide a step-by-step explanation in your answer. Irrelevant parts of the question should be ignored, and the following terms should be used in your answer.To solve the inequality 72 - 72 > 1 X-1 X, we need to simplify the inequality as shown below:72 - 72 > 1 X-1 X0 > X - 1Since we want to get X alone on one side of the inequality, we need to add 1 to both sides:0 + 1 > X - 1 + 1X > 0Thus, the solution to the inequality 72 - 72 > 1 X-1 X is (0, ∞).To find all values of x for which the graph of f(x) = x² flies above the graph of g(x) = 2x + 48, we need to solve the inequality:f(x) > g(x)x² > 2x + 48We can rearrange this inequality as follows:x² - 2x > 48Now, we need to factor the left-hand side of the inequality:x(x - 2) > 48The inequality will be satisfied if x > 0 and x - 2 > 0 (i.e. x > 2), so the solution to the inequality is x > 2.The domain of the given function f(x) = 72 + x - x² is all real numbers, since there are no restrictions on the input value x. Therefore, the domain of the function is (-∞, ∞).
Learn more about Function
brainly.com/question/12431044
#SPJ4