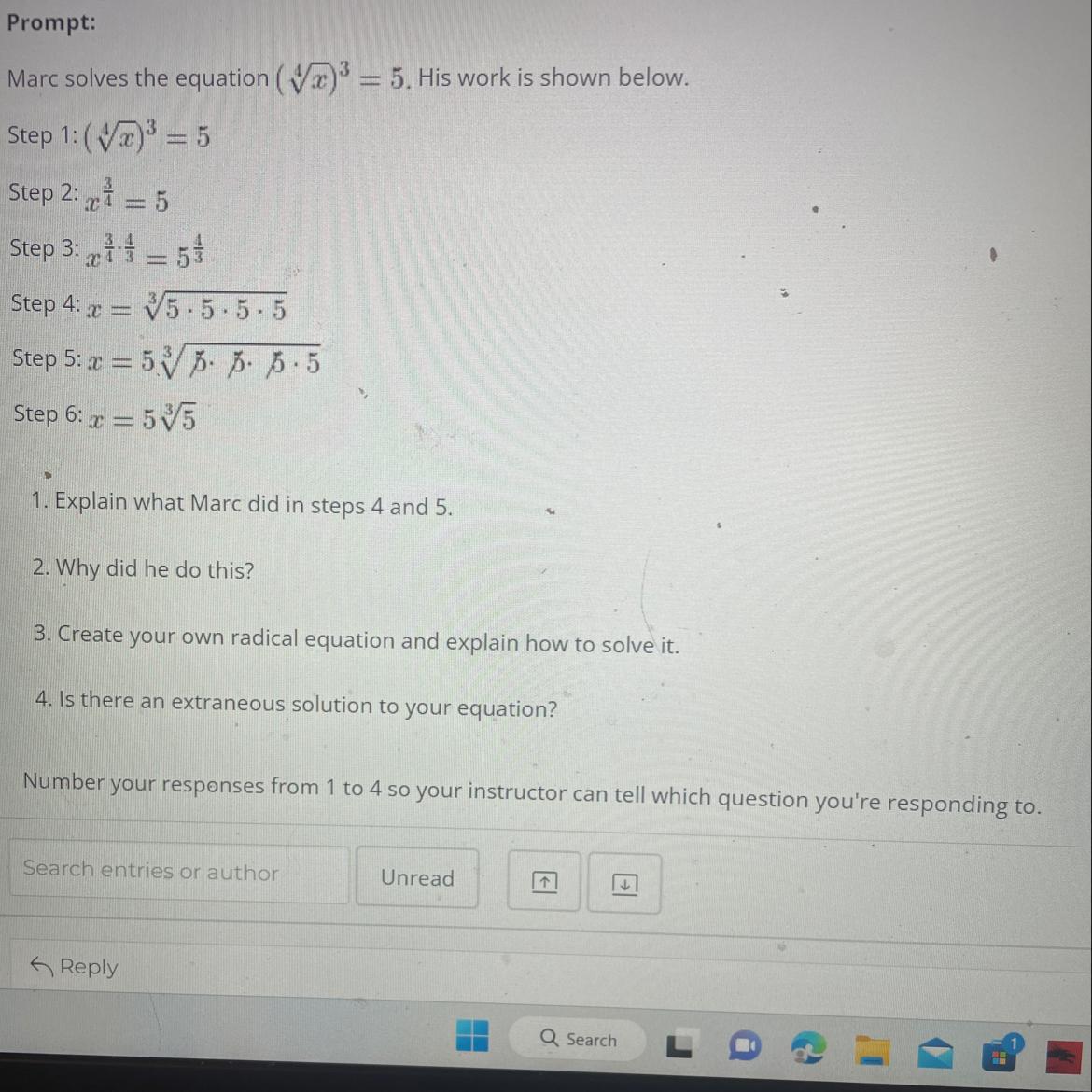
Answers
Answer:
23: 21 - 30
48: 41 - 50
39: 31 - 40
25: 21 - 30
45: 41 - 50
10: 1 - 10
16: 11 - 20
32: 31 - 40
62: 61 - 70
39: 31 - 40
48: 41 - 50
18: 11 - 20
19: 11 - 20
54: 51 - 60
12: 11 - 20
4: 1 - 10
Hope this helps :D
Related Questions
What is the volume of the cone shown below?
A. 1344 cu. units
B. 504 cu. units
C. 4032 cu. units
D. 2016 cu. units

Answers
Work Shown:
r = 8 = radius
h = 63 = height
V = volume of the cone
\(V = \frac{1}{3}\pi*r^2*h\\\\V = \frac{1}{3}\pi*8^2*63\\\\V = \left(\frac{1}{3}*8^2*63\right)\pi\\\\V = \left(\frac{1}{3}*63*8^2\right)\pi\\\\V = \left(21*64\right)\pi\\\\V = 1344\pi\\\\\)
Side note: You could write the symbol π as "pi" without quotes.
please quick as possible

Answers
Answer:
3
Step-by-step explanation:
6x (horizontal totals) + 12 x(vertical totals) = 54
18x = 54
x = 3
HOPE THIS HELPS!!!
Mrs. Wright is planning her best friend's baby shower. She calculates that the food will cost $9 per guest, and she has already spent $43 on decorations.
Answers
Based on the questions, 13 guests are attending her best friend’s baby shower.
How did we get 13 guests as a result?Firstly, we need to write down the numbers that are already stated in the question.
The food will cost around $9 per guests. She spent $43 on decoration for the event. Then, she can afford to spend $160 for the event.43 + 9 × х = 160
Secondly, move the x to continue counting.
9x = 160 – 43
Thirdly, calculate the numbers above.
9x = 117
Then, move the X to get the answer.
X = \(\frac{117}{9}\)
Lastly, try to finish the division.
X = 13
In conclusion, the result shows that 13 guests attend Mrs. Wright’s best friend's baby shower.
The above question is about the algebraic word problem.
A word problem is a math question written in a short story scenario. Usually, it is a simple short story that is easy to understand.
Learn more about Algebra word problem:
https://brainly.com/question/21405634
#SPJ4
In a run chart, the variable being measured is typically placed on what axis?
(A) X axis
(B) Y axis
(C) Either axis
(D) Neither axis;
Answers
Which word is associated with multiplication when computing probabilities? Choose the correct answer below Disjoint O And O Not
Answers
When we calculate probabilities involving one event AND another event occurring, we multiply their probabilities.
According to me , independent is the word which is associated with multiplication when computing probabilities . Because when the events are independent then we multiply the probabilities .
According to the multiplication rule of probability, the probability of occurrence of both the events A and B is equal to the product of the probability of B occurring and the conditional probability that event A occurring given that event B occurs.
The probability of the union of two events is equal to the sum of individual probabilities. The union of two set contains all the elements of previous sets. The union is denoted by ∪. The equation for the students earnings will be expressed as P(A∪B). The occurrence of event A changes the probability of B then the events are dependent. If the probability of two events happening together is zero then the events are mutually exclusive.
Therefore,
When we calculate probabilities involving one event AND another event occurring, we multiply their probabilities.
To learn more about Probability visit :
brainly.com/question/13490624
#SPJ4
1,945x46 multiplying multiplication
Answers
Answer:
89,470
Step-by-step explanation:
when running a half marathon (13.1 miles), it took noah 8 minutes to run from mile marker 1 to mile marker 2, and 19 minutes to run from mile marker 2 to mile marker 4. how long did it take noah to run from mile marker 1 to mile marker 4?
Answers
It took Noah 27 minutes to run from mile marker 1 to mile marker 2, by basic algebra.
What is basic algebra?Numbers, variables, constants, expressions, equations, linear equations, and quadratic equations are all part of the basics of algebra.Additionally, the algebraic expressions contain the fundamental arithmetic operations of addition, subtraction, multiplication, and division.Now,
Given:
Time taken to run from mile marker 1 to mile marker 2 = 8 minutesTime taken to run from mile marker 2 to mile marker 4 = 19 minutesTo find: Total time taken to run from mile marker 1 to mile marker 4.
Finding:
By basic algebra, we can say that:
The total time taken = Time taken to run from mile marker 1 to mile marker 2 + Time taken to run from mile marker 2 to mile marker 4
=> Total time taken = 8 minutes + 19 minutes = 27 minutes.
Hence, It took Noah 27 minutes to run from mile marker 1 to mile marker 2, by basic algebra.
To learn more about basic algebra, refer to the link: brainly.com/question/723406
#SPJ4
Jose had 83.8% in math class. He did not do well on his last test and his grade changed by -1.9%. What is his new grade?
Answers
Answer:
81.9
Step-by-step explanation:
simple math pls mark brainlist
I need help on this problem

Answers
Answer:
a
Step-by-step explanation:
a
. Express the balance sheets in common-size percents. (Do not round intermediate calculations and round your final percentage answers to 1 decimal place.)
Answers
Full question attached
Answer attached
Answer and Step-by-step explanation:
We are asked to find the percentage of each asset or liability in total assets and liabilities. To find percentage of each asset or liability in the balance sheet, we divide the asset or liability's value(from each year in the balance sheet) by total assets and liabilities for that year and multiply by 100:


Mean=1. 3kg and standard daviation=5. 6kg. If 16 male college students are randomly selected find the probability that their mean weight gain during freshman year is between
0 kg
and
3 kg
The probability is (Round to four decimal places as needed
Answers
The probability that their mean weight gain during freshman year is between 0 kg and 3 kg is 0.7207, rounded to four decimal places.
To solve this problem, we can use the central limit theorem, which states that the sample mean of a large enough sample taken from a population with any distribution approaches a normal distribution.
Let X be the weight gain of a male college student during freshman year. Then X follows a normal distribution with mean μ = 1.3 kg and standard deviation σ/√n = 5.6kg/√16 = 1.4 kg (since we have a sample size of 16).
Let Y be the sample mean weight gain of 16 male college students during freshman year. Then Y also follows a normal distribution with mean μ = 1.3 kg and standard deviation σ_Y = σ/√n = 1.4 kg.
To find the probability that their mean weight gain during freshman year is between 0 kg and 3 kg, we need to standardize the values using the z-score formula:
z = (x - μ) / σ_Y
For x = 0 kg:
z1 = (0 - 1.3) / 1.4 = -0.93
For x = 3 kg:
z2 = (3 - 1.3) / 1.4 = 1.21
Using a standard normal distribution table or calculator, we can find the area under the curve between z1 and z2:
P(-0.93 < Z < 1.21) = 0.7207
Therefore, the probability that their mean weight gain during freshman year is between 0 kg and 3 kg is 0.7207, rounded to four decimal places.
Learn more about probability from
https://brainly.com/question/30390037
#SPJ11
I need help with this problem please!
Answers
Answer: uh don't know
Step-by-step explanation: look it up
List the sides of ΔJKL in order from least to greatest if m∠J=(2x+6)°, m∠K=(10x-11)°, and m∠L=(3x-10)°.
Answers
The sides of the triangle JKL from least to greatest would be
JK,
KL, and
JL.
How to list the sides of the triangle JKLTo find the order of the sides of ΔJKL from least to greatest, we need to use the angle-side relationship in triangles
In a triangle, the largest angle is always opposite the longest side, and the smallest angle is opposite the shortest side.
The sum of the angles in a triangle is always 180°, so:
m∠J + m∠K + m∠L = 180
(2x + 6) + (10x - 11) + (3x - 10) = 180
15x - 15 = 180
15x = 195
x = 13
Now we can find the measures of all three angles:
m∠J = (2x + 6)° = 32°
m∠K = (10x - 11)° = 119°
m∠L = (3x - 10)° = 29°
Next, we can use the angle-side relationship to find the order of the sides:
Side opposite the smallest angle: JK
Side opposite the largest angle: KL
So, the sides of ΔJKL in order from least to greatest are JK, JL, KL.
Learn more about angle-side relationship at:
https://brainly.com/question/18980866
#SPJ1
NEED HELP ASAP! will rate 5 stars!!

Answers
Answer:
The first one is the answer
Step-by-step explanation:
This is just a review!!!!!!! help!!!!!!!!!! Question is on image below.

Answers
Answer:
I don't know but use this app on your phone called mw and it should help
Step-by-step explanation:
find the average rate of change of the car's position on the interval . include units on your answer.
Answers
The average rate of change of the car's position on the interval is ∆P/∆t.
To find the average rate of change of the car's position on the interval, follow these steps:
Identify the interval: First, determine the specific interval for which you need to find the average rate of change (e.g.,
between times t1 and t2).
Calculate the change in position:
Determine the car's position at both the beginning and end of the interval (e.g., positions P1 and P2).
Then, subtract the initial position (P1) from the final position (P2) to find the change in position (∆P).
Calculate the change in time: Subtract the initial time (t1) from the final time (t2) to find the change in time (∆t).
Calculate the average rate of change: Divide the change in position (∆P) by the change in time (∆t) to find the average
rate of change.
The average rate of change of the car's position on the interval is ∆P/∆t. Include units in your answer (e.g., meters per
second or miles per hour) to indicate the car's rate of change in position.
for such more question on average
https://brainly.com/question/20118982
#SPJ11
Ned is looking over his credit scores. His Experian score is 690, his Equifax score is 691, and his TransUnion score is 651. What is his mean credit score? (Round to the nearest whole point, if applicable.) a. 677 b. 671 c. 665 d. 690
Not on the quiz, so this isn't urgent. Thank you for helping :}}
Answers
Answer:
a. 677
Step-by-step explanation:
Add the three scores, 690+ 691+651 then divide by 3
equals to 677.3 so by rounding to the nearest whole point it’s 677.
The mean credit score of Ned is 677. The correct answer would be option (A).
What is arithmetic mean?The arithmetic mean is defined as the ratio of the sum of observations to the total number of observations. It can be referred to as the average of a specific set of data or the arithmetic mean.
The formula for the mean of a given set of data is as follows:
Mean = Sum of Observations/Total number of observations
The mean credit score of Ned can be calculated by adding all three credit scores and dividing the sum by 3.
So, (690 + 691 + 651) / 3
= 2032 / 3
= 677.33
Rounding to the nearest whole point, we get
= 677
Therefore, the mean credit score of Ned is 677.
Learn more about Arithmetic means here:
brainly.com/question/13000783
#SPJ6
What is
\( {4}^{ - 3}\)
as a fraction?
Answers
Step-by-step explanation:
\( {4}^{ - 3} \)
\( = ( \frac{1}{4} )^{3} \)
I have double check this
suppose a large shipment of laser printers contained 14% defectives. if a sample of size 411 is selected, what is the probability that the sample proportion will differ from the population proportion by greater than 4%? round your answer to four decimal places.
Answers
The probability that the sample proportion will differ from the population proportion by greater than 4% is 0.990.
According to the Central limit theorem, if from an unknown population large samples of sizes n > 30, are selected and the sample proportion for each sample is computed then the sampling distribution of sample proportion follows a Normal distribution.
The mean of this sampling distribution of sample proportion is:
µ = p
The standard deviation of this sampling distribution of sample proportion is:
σ = √p(1-p)/n
The information provided is:
p = 0.14
n = 41
As the sample size is large, i.e n = 411 > 30. the central limit theorem can be used to approximate the sampling distribution of sampling proportion.
Compute the values of P(p^ - p >0.04) as follows:
P(p^ - p < 0.04) = P(p^-p/σ > 0.04/√0.14(1-0.14)/411
= P(Z>2.33)
= 0.990
Thus the probability that the sample proportion will differ from the population proportion by greater than 4% is 0.990
Learn more about Normal distribution here:
brainly.com/question/25638875
#SPJ4
Miss Vickers is planning a field trip for her class. She puts her students' names in a hat and pulls out 10 names and asks the students whether they prefer the zoo, the aquarium, or the nature center. What sampling method does she use
Answers
Answer: hmmm
Step-by-step explanation:
A data set has a mean of 177 and a standard deviation of 20. Compute the coefficient of variation.
Answers
A data set has a mean of 177 and a standard deviation of 20. 11.3% is the coefficient of variance for this collection of data.
The ratio of a data set's standard deviation to its mean, stated as a percentage, is represented by a coefficient of variation (CV), a dimensional measure of variability. It is a practical tool for contrasting the relative variance of two or more data groups with various means or measurement units.
We divide the usual level deviation by the mean, multiply the result by 100, and that number is the coefficient of variation. The coefficient in variation can be computed as follows in this situation: Using the formula CV = (standard deviation / mean) x 100, (20 / 177) x 100, and 11.3%
A low coefficient for variation means that the mean is a good indicator of the data and that the set of data has low relative variability. On the other hand, a high coefficient for variation shows substantial relative variability, which could point to the need for additional research or alternate metrics of central tendency.
Learn more about variance here:
https://brainly.com/question/30044695
#SPJ4
whats 13/12 as a mixed number?
Answers
The required solution in mixed form is,
⇒ 1 1/12
We have to given that,
To change the fraction 13/12 into mixed number.
We know that,
Mixed fraction is written as,
a b/c
Where, a, b and c are whole number.
Here, Fraction is,
13 / 12
After divide,
1 1/12
Therefore, The required solution in mixed form is,
⇒ 1 1/12
Learn more about the fraction visit:
https://brainly.com/question/5454147
#SPJ6
15. If 3x + 5y = 11 and xy = 2, find the value of: 9x2 + 25y2
Answers
The problem provides the information:
3 x + 5 y = 11
and x y = 2
Asking to find the value of 9 x^2 + 25 y^2
so we proceed to recall what the square of a binimial of the following form is:
\((3x+5y)^2=(3x)^2+2(3x\cdot5y)+(5y)^2=9x^2+30xy+25y^2_{}\)So, we see that the square of the quantity: (3 x + 5 y) which from the information given equals 11^2 = 121, must equal the expression:
9 x^2 + 25 y^2 + 30 x y.
then we write the following equation making both parts equal:
\(\begin{gathered} 11^2=9x^2+25y^2+30xy \\ 121=9x^2+25y^2+30xy \\ 121=9x^2+25y^2+30(2) \\ 121-60=9x^2+25y^2 \\ 61=9x^2+25y^2 \end{gathered}\)Therefore the value of 9 x^2 + 25 y^2 = 61
Let A for A = 6. 6 -2 -2 6 -1 The eigenvalues for A are λ = 3,6 and 8. Find a basis for the eigenspace -1 {B} Orthogonally diagonalize A; that is, find matrices P and D such that A = PDP-1 (b) A basis for the eigenspace for λ = 3 is and a basis for the eigenspace for A = 8 is {[]} where the columns of P are orthonormal and D is diagonal.
Answers
For the eigenspace -1 {B}, the basis vectors are [-1 2 0]T and [-1 0 1]T. The matrix A can be orthogonally diagonalized as A = PDP-1 where P is the orthonormal matrix whose columns are the eigenvectors of A, and D is the diagonal matrix containing the eigenvalues of A.
The eigenspace of the matrix A is the set of all eigenvectors associated with a particular eigenvalue. Given the matrix A = 6 6 -2 -2 6 -1 with eigenvalues λ = 3, 6, and 8. The eigenvectors of A are obtained by solving the equation (A - λI)x = 0, where λ is the eigenvalue and I is the identity matrix.
For λ = 3, we have (A - 3I)x = 0. Substituting A and I, we get the matrix equation:
3x1 - 6x2 - 2x3 = 0
-2x1 + 3x2 - 2x3 = 0
-2x1 - 2x2 + 3x3 = 0
Solving this system of equations, we get two linearly independent solutions, which form a basis for the eigenspace of λ = 3. These basis vectors are given by [-1 2 0]T and [-1 0 1]T. For λ = 8, we have (A - 8I)x = 0. Substituting A and I, we get the matrix equation:
-2x1 + 6x2 - 2x3 = 0
-2x1 - 2x2 - 2x3 = 0
-2x1 - 2x2 - 9x3 = 0
Solving this system of equations, we get one linearly independent solution, which forms a basis for the eigenspace of λ = 8. This basis vector is given by [0 1 4/3]T. To orthogonally diagonalize A, we need to find an orthonormal basis for the eigenspace of A. The orthonormal basis can be obtained by normalizing the basis vectors obtained above.
The orthonormal basis for λ = 3 is given by
v1 = [-1/√2 1/√2 0]T
v2 = [-1/√6 0 1/√3]T
The orthonormal basis for λ = 8 is given by
v3 = [0 1/√2 1/√2]T
The orthonormal matrix P is then given by
P = [v1 v2 v3]
The diagonal matrix D is given by
D = diag(3, 6, 8)
Finally, we can compute the orthogonally diagonalized matrix A as
A = PDP-1
where P-1 is the inverse of P, which can be computed as the transpose of P since P is an orthonormal matrix.
To know more about orthonormal matrix refer here:
https://brainly.com/question/31419241
#SPJ11
Who prepares the questions for grade 7 math worksheets?
Answers
The questions for grade 7 math worksheets are typically prepared by math teachers, curriculum specialists, and educational publishers.
These professionals use their knowledge of math concepts and pedagogy to create questions that are appropriate for the grade level and aligned with educational standards.
Additionally, they may use feedback from students and other teachers to refine the questions and ensure they are effective for teaching and learning. It is important for the questions to be clear, accurate, and challenging in order to help students develop their math skills and prepare for more advanced concepts.
To know more about pedagogy click on below link:
https://brainly.com/question/30400049#
#SPJ11
Answer is actually 1420
Answers
Answer:
1420
Step-by-step explanation:
its in the question
**PLS SOMEONE HELP!! I WOULD APPRECIATE IT AND ILL GIVE BRAINLIEST:)**

Answers
Answer:
x = 3
Step-by-step explanation:
First, you should put what is shown into an equation, which would be:
x + x + x + x + x + 2 = 17 or 5x + 2 = 17
Next, you subtract by two on both sides o the equation to isolate the variable x:
5x = 15
Now, you can divide by five on both sides to find the actual value of x:
x = 3
Hope this helped somewhat! :)
**25 POINTS**
Which of the following lists of ordered pairs is a function?

Answers
Answer: A
Step-by-step explanation: A function is when every domain (x value) corresponds to one range (y value) so that it passes the vertical line test
g suppose the acme drug company what is the probability that the percent difference of -.13 or less is seen if the true difference is 0
Answers
To conclude, the probability of the Acme Drug Company seeing a percent difference of -.13 or less if the true difference is 0 is quite low and is equal to 0.0934.
The probability that the Acme Drug Company would see a percent difference of -.13 or less if the true difference is 0 is quite low. This is because a difference of -.13 is a very small percentage in comparison to a true difference of 0.
Mathematically, the probability of this happening would be equal to the area under the standard normal distribution curve for values between -0.13 and 0. In other words, the probability that the Acme Drug Company would see a percent difference of -.13 or less if the true difference is 0 is equal to the area from the left tail of the standard normal distribution curve up to the mean (0) of the curve.
Using a standard normal distribution calculator, we can see that the probability of the Acme Drug Company seeing a percent difference of -.13 or less is 0.0934. This probability is extremely low and it is not likely that the Acme Drug Company would experience such a small percent difference.
for such more questions on probability
https://brainly.com/question/13604758
#SPJ11
combine/distribute
1. 1 - 4a - 5
2. -k + 3k
3. -3(n+9)
4. 3(8-4v)-v
Answers
Answer:
1.)-4a-4
2.) 2k
3.)-3n-27
4.)24-13v
Step-by-step explanation:
