Margo places 6 pennies and 4 nickels in a jar. For the experiment, she selects a penny, replaces it, and selects another penny. If Margo repeats the experiment 100 times, how many times can she expect to select two pennies?
Answers
Answer: She is expected to select 2 pennies 36 times.
Step-by-step explanation:
In jar , we have
6 pennies and 4 nickels
Total coins = 10
The probability of getting two pennies ( with replacement) =
\(\dfrac{\text{Number if ways to get 2 pennies}}{\text{Total ways to select 2 coins}}\\\\=\dfrac{6\times6}{10\times10} \ \ [\text{independent events]}\\\\=\dfrac{36}{100}\)
If Margo repeats the experiment 100 times, then expected number of times to select two pennies = \(\dfrac{36}{100}\times100=36\)
She is expected to select 2 pennies 36 times.
We will see that Margo can expect to randomly select two pennies 36 times.
Probability of selecting two pennies at random.
The probability of selecting a penny at random is equal to the quotient between the number of pennies in the jar and the total number of coins in the jar, this is:
p = 6/10
And doing that two times, needs that probability two times, so the probability of selecting two pennies is:
p = (6/10)*(6/10) = 36/100
How many times she can expect to select 2 pennies in 100 attempts?Here we just need to multiply the above probability by 100, we will get:
(36/100)*100 = 36
So she can expect to get two pennies 36 times.
If you want to learn more about probability, you can read:
https://brainly.com/question/251701
Related Questions
are
equivalent to 192 ounces?
How many pounds
24
pounds.
192 ounces is equivalent to
Answers
Answer:
12 lbs
Step-by-step explanation:
There are 16 ounces in a pound
192 ounces * 1 pound / 16 ounces = 12 pounds
Step-by-step explanation:
1 pound = 16 ounces
24 pound = 16 x 24 ounces = 384 ounces
1 ounce = 1/16 pounds
192 ounces = 192/16 pounds = 12 pounds
12 miles below sea level
Answers
Answer:
12 miles below sea level
Step-by-step explanation:
Define the event b as the event that a black horse wins. assuming that in the original experiment, the a priori probabilities of any particular horse winning is equal, verify that:_____.
Answers
The probability of event B, denoting the event that a black horse wins, is given by P(B) = M/N, where M represents the number of black horses and N represents the total number of horses in the race.
Assuming that in the original experiment, the a priori probabilities of any particular horse winning are equal, we can verify that the probability of event B, which is defined as the event that a black horse wins, can be determined as follows:
Let's assume there are N total horses participating in the race, and each horse has an equal chance of winning. Since there are N horses in total, the probability of any specific horse winning is 1/N.
Now, let's consider the number of black horses participating in the race. Let's assume there are M black horses among the N total horses. Therefore, the probability of any specific horse being black is M/N.
To calculate the probability of event B, which is the event of a black horse winning, we need to determine the number of favorable outcomes (black horse winning) and divide it by the total number of possible outcomes (any horse winning).
The number of favorable outcomes is M, as there are M black horses in the race.
The total number of possible outcomes is N since there are N horses in the race.
Therefore, the probability of event B, denoted as P(B), can be calculated as:
P(B) = M/N
Since we assumed that the a priori probabilities of any particular horse winning are equal, this implies that the a priori probabilities of a specific horse being black are also equal. Thus, the probability of event B, which is the event of a black horse winning, is equal to the ratio of the number of black horses (M) to the total number of horses (N).
In conclusion, the probability of event B, denoting the event that a black horse wins, is given by P(B) = M/N, where M represents the number of black horses and N represents the total number of horses in the race.
Learn more about probability here
https://brainly.com/question/25839839
#SPJ11
there are 7 red pens in jamals desk drawer. there are 3 more black pens than red pens. there are also 3 more blue pens than red pens. how many pens are there in all
Answers
Answer:
27 pens
Step-by-step explanation:
red pen=7
3more black pen than red pen=3+7=10 black pen
3 more blue pen than red pen=3+7=10 blue pen
T=redpen + blackpen +blue pen
T=7+10+10
T=27 pen
Please help with this problem thanks

Answers
1.5 x 10
-15^2 +120
=-105
1. Perform the following transformations on LMK: R180°L(2,6), M(8,8), K(7,4)What are the vertices of L', M', nad K?

Answers
In order to make the given transformation (rotation of 180°) you consider thatsuch transformation demands:
T(x,y) => (-x,-y)
Then, when you apply the previous transformation to the points L(2,6), M(8,8) and K(7,4), you obtain:
T[L(2,6)] => L'(-2,-6)
T[M(8,8)] => M'(-8,-8)
T[K(7,4)] => K'(-7,-4)
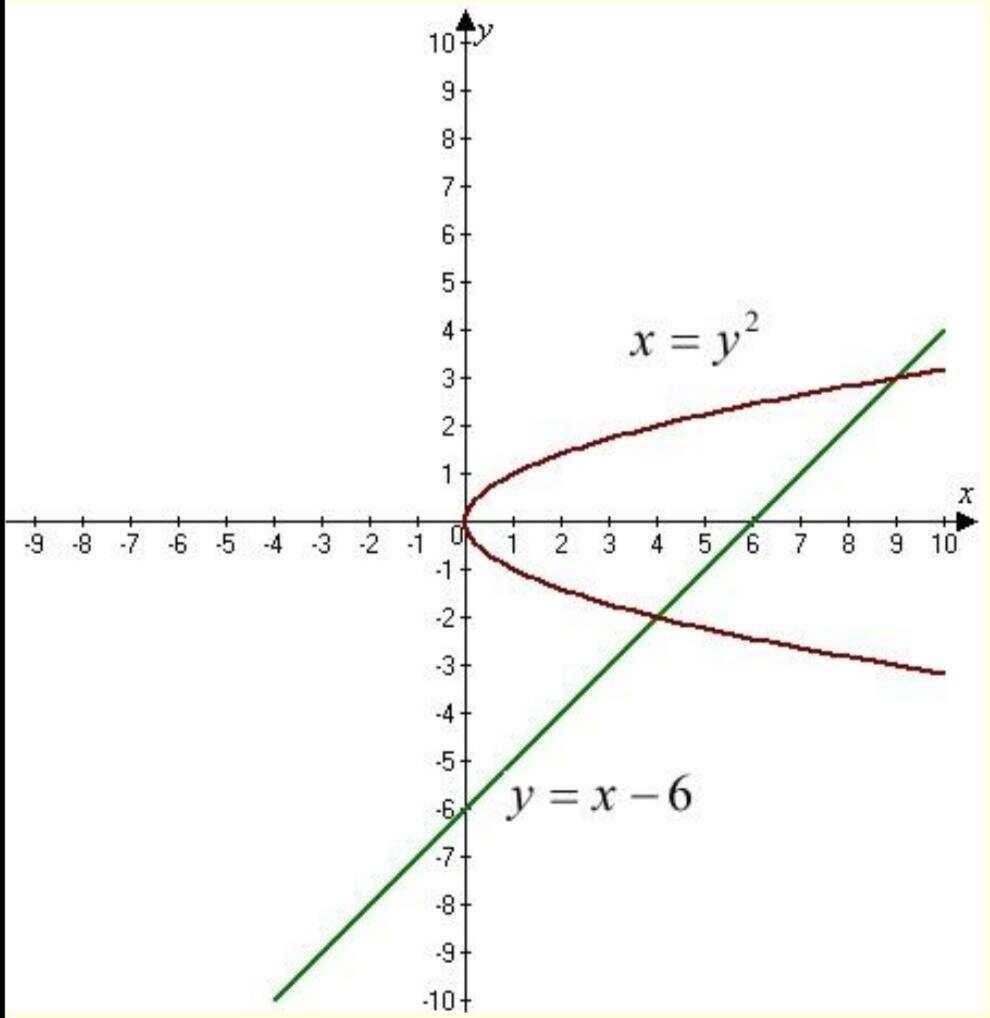
Set up iterated integrals for both orders of integration. Then evaluate the double integral using the easier order.
y dA, D is bounded by y = x − 6; x = y2
D
Answers
The value of the double integral using the easier order, ydA bounded by y = x − 6; x = y² is 125/12.
The double integral, indicated by ', is mostly used to calculate the surface area of a two-dimensional figure. By using double integration, we may quickly determine the area of a rectangular region. If we understand simple integration, we can easily tackle double integration difficulties. Hence, first and foremost, we will go over some fundamental integration guidelines.
Given, the double integral ∫∫yA and the region y = x-6 and x = y²
y = x-6
x = y²
y² = y +6
y² - y - 6 = 0
y² - 3y +2y - 6 = 0
(y-3) (y+2) = 0
y = 3 and y = -2
\(\int\int\limits_\triangle {y} \, dA\\ \\\)
= \(\int\limits^3_2 {y(y+6-y^2)} \, dx \\\\\int\limits^3_2 {(y^2+6y-y^3)} \, dx \\\\(\frac{y^3}{3} + 3y^2-\frac{y^4}{4} )_-_2^3\\\\\frac{63}{4} -\frac{16}{3} \\\\\frac{125}{12}\)
The value for the double integral is 125/12.
Integration is an important aspect of calculus, and there are many different forms of integrations, such as basic integration, double integration, and triple integration. We often utilise integral calculus to determine the area and volume on a very big scale that simple formulae or calculations cannot.
Learn more about Double Integral:
https://brainly.com/question/19053586
#SPJ4
Use spherical coordinates to find the volume of the solid. Solid inside x2 + y2 + z2 = 9, outside z = sqrt x2 + y2, and above the xy-plane
Answers
To determine the volume of the solid, use spherical coordinates. The formula to use when converting to spherical coordinates is:
r = √(x^2 + y^2 + z^2)θ = tan-1(y/x)ϕ = tan-1(√(x^2 + y^2)/z)
For the solid, we have that:
\(x^2 + y^2 + z^2 = 9, z = √(x^2 + y^2)\)
, and the solid is above the xy-plane.
To find the limits of integration in spherical coordinates, we note that the solid is symmetric with respect to the xy-plane. As a result, the limits for ϕ will be 0 to π/2. The limits for θ will be 0 to 2π since the solid is circularly symmetric around the z-axis.To determine the limits for r, we will need to solve the equation z = √(x^2 + y^2) in terms of r.
Since z > 0 and the solid is above the xy-plane, we have that:z = √(x^2 + y^2) = r cos(ϕ)Substituting this expression into the equation x^2 + y^2 + z^2 = 9 gives:r^2 cos^2(ϕ) + r^2 sin^2(ϕ) = 9r^2 = 9/cos^2(ϕ)The limits for r will be from 0 to 3/cos(ϕ).The volume of the solid is given by the triple integral:V = ∫∫∫ r^2 sin(ϕ) dr dϕ dθ where the limits of integration are:r: 0 to 3/cos(ϕ)ϕ: 0 to π/2θ: 0 to 2π\(r = √(x^2 + y^2 + z^2)θ = tan-1(y/x)ϕ = tan-1(√(x^2 + y^2)/z)\)
To know more about limits of integration visit :
https://brainly.com/question/31994684
#SPJ11
Test 52,320 for divisibility by 2, 3, 5, 9, or 10
Answers
Answer: try 2
Step-by-step explanation:
Because u could divide then
Find the maximum rate of change of f at the given point and the direction in which it occurs.
f(s,t)= te^(st), (0,2)
Answers
The maximum rate of change of function f at the given point occurs in the direction of the gradient vector.
How to find maximum rate of change?To find the maximum rate of change of a function f at a given point, we need to compute the gradient of the function and evaluate it at that point. The gradient represents the direction of steepest ascent, and its magnitude indicates the maximum rate of change.
By finding the partial derivatives of f with respect to each variable and evaluating them at the given point, we obtain the components of the gradient vector. The maximum rate of change occurs in the direction of this gradient vector. Without the specific function and point, it is not possible to determine the exact maximum rate of change or its direction.
Learn more about rate of change
brainly.com/question/29181688
#SPJ11
Find the domain of the function. (Enter your answer using interval notation.) 3x³-3 f(x)= x² + 3x - 18 Need Help? Read It Viewing Saved Work Revert to Last Response 8. [-/2 Points] DETAILS SCALCCC4 1.1.044. Find the domain of the function. (If you need to use or , enter -INFINITY or INFINITY.) 1(2)-{9-11 #1752 ifr>2 18 Need Help? Read I Wh 9. [-/2 Points] DETAILS SCALCCC4 1.1.046. Find the domain of the function. (If you need to use or, enter -INFINITY or INFINITY.) f(x) = z+3 ifz<-1 -2r if r $1 -2 if : > 1 18 Need Help? Readi
Answers
Domain of the function f(x)= 3x³-3x²+3x - 18 is (-∞, ∞).
The term "domain" in mathematics means the set of all possible inputs for which a function provides a valid output.
In order to find the domain of a function, you need to consider the value of the variables that the function depends on and make sure that the function is defined for all possible values of those variables.
The given function is:
f(x)= 3x³-3x²+3x - 18
To find the domain of the function, we need to make sure that the function is defined for all possible values of x.
The given function is a polynomial function.
Polynomial functions are defined for all values of x. Hence, the domain of the given function is (-∞, ∞).
To know more about infinity visit:
https://brainly.com/question/22443880
#SPJ11
A new graph is formed from y = 5x by changing the slope to 9 and y-intercept to 8. Which statement about the new relationship is true?
The new graph has a steeper slope and a y-intercept of 13.
The new graph has a steeper slope and y-intercept of 8.
The new graph has a less steep slope and a y-intercept of 13
The new graph has a less steep slope and a y-intercept of 8
Answers
Answer:
The new graph has a steeper slope and a y-intercept of 8.
Step-by-step explanation:
From Analytical Geometry, we define a straight line by following first order polynomial (linear function):
\(y = m\cdot x + b\) (1)
Where:
\(x\) - Independent variable.
\(y\) - Dependent variable.
\(b\) - y-Intercept.
\(m\) - Slope.
And the slope represents the change in dependent variable (\(\Delta y\)) divided by the change in independent variable (\(\Delta x\)). That is:
\(m = \frac{\Delta y}{\Delta x}\)
If \(m_{1} > 0\) and \(m_{2} > m_{1}\), then the new line is steeper with respect to the original line.
Let \(y = 5\cdot x\), its slope and y-intercept are 5 and 0, respectively. If slope is changed into 9 and y-intercept becomes 8, then the new graph has a steeper slope and a y-intercept of 8.
What is the gradient of the linear function, X-3Y=6
Answers
Answer:
x=6+3y
Step-by-step explanation:
x subject of the formula
What is the value of x in the figure below? In this diagram, AABD~ ACAD.
12
X
27
Answers
Step-by-step explanation:
Based on the information given in the diagram, we know that AABD and ACAD are similar triangles, which means their corresponding sides are proportional.
Let's denote the length of AD as x. According to the similarity of the triangles, we can set up the following proportion:
AB/AC = AD/AD
Since AD is common to both triangles and has a length of x, the proportion simplifies to:
AB/AC = 1
We are given that AB = 12, so we can substitute that value into the proportion:
12/AC = 1
To solve for AC, we can cross-multiply:
12 = AC
Therefore, the value of x in the figure is 12.
Find the maximum value of f(x, y, z) = 5xy + 5xz + 5yz – xyz subject to the constraint g(x, y, z) = x + y + z = 1, for x>0, y > 0, and z > 0.
Answers
We can solve this problem using the method of Lagrange multipliers. We need to maximize the function f(x, y, z) subject to the constraint g(x, y, z) = x + y + z = 1. We can set up the Lagrangian function L(x, y, z, λ) as follows:
L(x, y, z, λ) = f(x, y, z) - λg(x, y, z)
= 5xy + 5xz + 5yz - xyz - λ(x + y + z - 1)
To find the critical points of L, we need to take partial derivatives of L with respect to x, y, z, and λ, and set them equal to zero:
∂L/∂x = 5y + 5z - yz - λ = 0
∂L/∂y = 5x + 5z - xz - λ = 0
∂L/∂z = 5x + 5y - xy - λ = 0
∂L/∂λ = x + y + z - 1 = 0
From the first three equations, we can solve for x, y, and z in terms of λ:
x = (λ - 5y - 5z)/(5 - yz)
y = (λ - 5x - 5z)/(5 - xz)
z = (λ - 5x - 5y)/(5 - xy)
Substituting these expressions into the constraint equation x + y + z = 1, we get:
(λ - 5y - 5z)/(5 - yz) + (λ - 5x - 5z)/(5 - xz) + (λ - 5x - 5y)/(5 - xy) = 1
Simplifying this equation, we get:
λ(3xyz - 5(xy + xz + yz)) = -125
Since x, y, and z are positive, we know that 3xyz > 0, so we can divide both sides by 3xyz to get:
λ = -125/(5(xy + xz + yz))
Substituting this expression for λ back into the equations for x, y, and z, we get:
x = 5/3
y = 5/3
z = 1/3
We can check that these values satisfy the constraint equation x + y + z = 1 and that they correspond to a maximum of f(x, y, z) by computing the second partial derivatives of L and evaluating them at the critical point:
∂²L/∂x² = -yz, ∂²L/∂y² = -xz, ∂²L/∂z² = -xy, ∂²L/∂x∂y = 5 - z, ∂²L/∂x∂z = 5 - y, ∂²L/∂y∂z = 5 - x
The determinant of the Hessian matrix of L evaluated at the critical point is:
∂²L/∂x²(∂²L/∂y²)(∂²L/∂z²) + 2∂²L/∂x∂y(∂²L/∂x∂z)(∂²L/∂y∂z) - (∂²L/∂x²)(∂²L/∂y∂z)²
Learn more about derivatives here :brainly.com/question/30365299
#SPJ11
is the standard deviation of the numbers x, y, and z equal to the standard deviation of 10, 15, and 20 ?
Answers
This matches the placement of 10, 15, and 20 and hence the standard deviation will be the same in the two cases.
What is the standard deviation?
The standard deviation in statistics is a measure of the amount of variation or dispersion in a set of values. A low standard deviation indicates that the values are close to the set's mean, whereas a high standard deviation indicates that the values are spread out over a wider range.
Standard deviation determines how far numbers deviate from the mean. The Standard deviation of the two sets will be the same if the numbers from the respective means are placed in the same order.
This is what 10, 15, and 20 will be on a number line
10_ _ _ _15 _ _ _ _20
(15 is the mean and 10 and 20 are 5 steps away from the mean)
i) Z - X = 10
This is what Z and X will be on the number line
X _ _ _ _ _ Z
ii) Z - Y = 5
This is what Z and Y will on the number line.
Y_ _ _ _ _ Z
Together, their relative placement on the number line:
X _ _ _ _ _ Y _ _ _ _ _ Z
This matches the placement of 10, 15, and 20 and hence the standard deviation will be the same in the two cases.
To learn more about the area of the standard deviation visit,
https://brainly.com/question/475676
#SPJ1
Joe buys a sandwich from a deli for $8. He leaves a 15% tip. What is the total amount he paid for his lunch?
Answers
Answer:
9.20
Step-by-step explanation:
8.00 x 15% = 1.20
Here is an equation: 2x+4y=80 use the equation to help you complete the table.
Which equation represents the same relationship?

Answers
Answer:
When x = 6, y is 17
When x = 12, y is 14
The equation of the line is D
Step-by-step explanation:
2x + 4y = 80 Subtract 2x from both sides
2x - 2x + 4y = 80 - 2x
4y = 80 - 2x Divide both sides by 4
y = 20 - 2/4 x
y = 20 - 1/2x
Substitute x with 6
y = 20 - 1/2(6)
y = 20 - 3
y = 17
Substitute x with 12
y = 20 - 1/2(12)
y = 20 - 6
y = 14
find the critical numbers of the function on the interval ( 0 , 2 π ) . (enter your answers as a comma-separated list. if an answer does not exist, enter dne.) g ( θ ) = 32 θ − 8 tan θ
Answers
The critical numbers of the function \(\(g(\theta)\)\) on the interval \(\((0, 2\pi)\)\) are \(\(\frac{\pi}{3}\)\) and \(\(\frac{5\pi}{3}\)\).
To obtain the critical numbers of the function \(\(g(\theta) = 32\theta - 8\tan(\theta)\)\) on the interval \(\((0, 2\pi)\)\), we need to obtain the values of \(\(\theta\)\) where the derivative of \(\(g(\theta)\)\) is either zero or does not exist.
First, let's obtain the derivative of \(\(g(\theta)\)\):
\(\(g'(\theta) = 32 - 8\sec^2(\theta)\)\)
To obtain the critical numbers, we set \(\(g'(\theta)\)\) equal to zero and solve for \(\(\theta\)\):
\(\(32 - 8\sec^2(\theta) = 0\)\)
Dividing both sides by 8:
\(\(\sec^2(\theta) = 4\)\)
Taking the square root:
\(\(\sec(\theta) = \pm 2\)\)
Since \(\(\sec(\theta)\)\) is the reciprocal of \(\(\cos(\theta)\)\), we can rewrite the equation as:
\(\(\cos(\theta) = \pm \frac{1}{2}\)\)
To obtain the values of \(\(\theta\)\) that satisfy this equation, we consider the unit circle and identify the angles where the cosine function is equal to \(\(\frac{1}{2}\) (positive)\) or \(\(-\frac{1}{2}\) (negative)\).
For positive \(\(\frac{1}{2}\)\), the corresponding angles on the unit circle are \(\(\frac{\pi}{3}\)\) and \(\(\frac{5\pi}{3}\)\).
For negative \(\(-\frac{1}{2}\)\), the corresponding angles on the unit circle are \(\(\frac{2\pi}{3}\)\) and \(\(\frac{4\pi}{3}\)\)
However, we need to ensure that these angles fall within the provided interval \(\((0, 2\pi)\)\).
The angles \(\(\frac{\pi}{3}\)\) and \(\(\frac{5\pi}{3}\)\) satisfy this condition, while \(\(\frac{2\pi}{3}\)\) and \(\(\frac{4\pi}{3}\)\) do not. Hence, the critical numbers are \(\(\frac{\pi}{3}\)\) and \(\(\frac{5\pi}{3}\)\).
To know more about critical numbers refer here:
https://brainly.com/question/29743892#
#SPJ11
helppppppp 58 points

Answers
Answer:
19 sq cm
Step-by-step explanation:
2x2=4
2x3=6
1x3=3
2x3=6
6+6+4+3=19
If a place you like is renting for $800, and you had one other renter with whom you split the rent expense, how much does your GROSS INCOME need to be?
a.
$800
b.
$700
c.
$600
d.
$1,200
e.
$400
Answers
Answer:
How much should I spend on rent and utilities?
We calculate your monthly rent at 25% of your monthly net income (after monthly debt). This allows you to have enough cash left for other expenses and savings. Utilities are in general 2.5% of your monthly net income, and so in this case, you should expect to spend $0 or more per month on utilities.
Step-by-step explanation:
Answer:
the correct answer is e.$400
Which of the following is NOT a voluntary response sample? Choose the correct answer below O A. A survey is taken at a mall by asking passersby if they will fill out the survey O B. A radio station asks for call-in responses to a question concerning city recycling OC. A local dentist asks her patients to fill out a questionnaire and mail it back to determine the quality of the care received during an office visit OD. Quiz scores from a college level statistics course are analyzed to determine student progress State whether the data described below are discrete or continuous, and explain why. The number of donations a charity receives each month Choose the correct answer below. O A. The data are discrete because the data can only take on specific values. O B. The data are continuous because the data can take on any value in an interval. O C. The data are continuous because the data can only take on specific values. D. The data are discrete because the data can take on any value in an interval. O
Answers
The correct answer for the first question is D. The data are discrete because the data can take on any value in an interval.
Quiz scores from a college level statistics course are analyzed to determine student progress. This is not a voluntary response sample because the students taking the quiz are required to participate, and their scores are collected for the purpose of analyzing their progress.
For the second question, the data described, which is the number of donations a charity receives each month, is discrete. Discrete data can only take on specific values and cannot be divided into smaller, meaningful intervals. In this case, the number of donations can only be whole numbers, such as 0, 1, 2, and so on. It cannot take on any value in an interval or be represented by fractions or decimals. Therefore, the data is discrete.
It is important to distinguish between discrete and continuous data when analyzing and interpreting data, as different statistical methods and techniques are used for each type. Discrete data is usually counted or measured in whole numbers, while continuous data can take on any value within a given range or interval.
Know more about discrete here,
https://brainly.com/question/30565766
#SPJ11
Write an equivalent expression to 2x-1/2 - 4x + 4/3
Answers
Answer:
-4x+5/3
Step-by-step explanation:
-2x+5/6
multiply by 2
-4x+5/3
you can also check by plugging in a number for x and see if the answer is the same.
One week Grace spent 16% of her
weekly wage on a new bookshelf
that cost $184. What is her weekly
wage?
Answers
Answer:
$1150 will be the weekly wage
Step-by-step explanation:
184 = 16%*x
184 = 0.16*x
184/0.16 = x
x = $1150
Here, "x" is her weekly wage.
The product of the ages (in days) of two newborn babies Simran and Jessie in two dayswill be 48 more than the product of their ages today. How old are the babies if Jessie is 2days older than Simran?
Answers
Given that:
- The product of the ages (in days) of babies Simran and Jessie in two days
will be 48 more than the product of their ages today.
- Jessie is 2 days older than Simran.
Let be "j" Jessie's age (in days) and "s" Simran's age (in days).
You can set up the following System of Equations using the data given in the exercise:
\(\begin{cases}(j+2)(s+2)=js+{48} \\ j={s+2}\end{cases}\)You can use the Substitution Method to solve the system:
1. Substitute the second equation into the first one:
\((s+2+2)(s+2)=(s+2)s+48\)2. Solve for "s":
\((s+4)(s+2)=s^2+2s+48\)\((s)(s)+(s)(2)+(4)(s)+(4)(2)=s^2+2s+48\)\(s^2+2s+4s+8=s^2+2s+48\)\(\begin{gathered} 4s=48-8 \\ \\ s=\frac{40}{4} \\ \\ s=10 \end{gathered}\)3. Substitute "s" into the second original equation and evaluate:
\(\begin{gathered} j=10+2 \\ j=12 \end{gathered}\)Hence, the answer is: Jessie is 12 days old and Simran is 10 days old.
1.1 Discuss how interactions involving dummy variables, impact on the results and interpretation of a regression model. Use your own example. 1.2 State the problems of using the linear probability model. In addition, briefly explain how some of these problems can be remedied 1.3 Critically assess the goodness-of-fit measures of logit models.
Answers
Interactions involving dummy variables can provide insights into the different effects of independent variables across categories.
1. Dummy variables are binary variables that represent categorical variables in a regression analysis. When interactions are included between dummy variables and other independent variables, it allows for differential effects of the independent variables based on the different levels of the categorical variable.
For example, let's consider a regression model to predict income based on education level and gender. We can include an interaction term between education level (represented by dummy variables for different levels) and gender. This interaction term allows us to examine whether the effect of education level on income differs between males and females. It helps capture any gender-specific differences in the relationship between education and income.
1.2 The linear probability model (LPM) is a common approach to estimate the probability of an event occurring using a linear regression framework. However, it has several problems:
1. The predicted probabilities from the LPM can fall outside the [0, 1] range: Since the LPM does not impose any restrictions on the predicted probabilities, they can sometimes exceed the valid probability range. This violates the assumption of probabilities being bounded between 0 and 1.
2. Heteroscedasticity: The LPM assumes constant error variance across the range of the predictors. However, in practice, the variability of the error term may change with different levels of the predictors, resulting in heteroscedasticity. This violates the assumption of homoscedasticity.
3. Non-linearity: The LPM assumes a linear relationship between the predictors and the probability of the event. However, this may not always be the case, and using a linear model can result in misspecification.
To remedy these problems, an alternative to the LPM is to use logistic regression or probit regression models. These models explicitly model the probability of an event occurring and address the issues mentioned above. They provide predicted probabilities that fall within the valid range of 0 to 1, account for heteroscedasticity, and allow for non-linear relationships between the predictors and the probability of the event.
Learn more about LPM here:
https://brainly.com/question/30890632
#SPJ4
solve for x multiple choice question

Answers
Answer:
12
Step-by-step explanation:
Using the side splitter theorem, \(\frac{x}{32}=\frac{9}{24} \implies x=12\).
The value of x is 12, option (B) is correct.
What is triangle proportionality theorem?A line parallel to one side of a triangle splits the other two sides of the triangle proportionally if the line also crosses the other two sides.
Given that,
Two lines are parallel to each other,
And length of sides are,
32 , x , 24 and 9.
By angle proportionality theorem,
32 / x = 24 / 9
x = (32 × 9) / 24
x = (4 × 9) / 3
x = 4 × 3
x = 12
The required length of x is 12.
Hence, option (B) is correct.
To know more about Triangle proportionality theorem on:
https://brainly.com/question/11807262
#SPJ1
if you answer some of them ill appreciate it ( you don't have to answer all of them )

Answers
Answer:
1) 23, 3, 13
2) 7, 13, 18
3) 9, 6, 77
4) 39, 8, 46
5) 13, 1, 32
6) 45, 9, 54
7) 14, 45, 30
8) 25, 10, 39
Step-by-step explanation:
Left to Right
please someone help me no one willl

Answers
Answer:
2/36 (5.556%)
I believe so correct me if I am wrong
. Importance of hydrologic cycle The role of water is central to most natural processes - Transport - Weathering, contaminant transport - Energy balance - transport of heat, high heat capacity - Greenhouse gas - 80% of the atmospheric greenhouse effect is caused by water vapor - Life - for most terrestrial life forms, water determines where they may live; man is exception
Answers
The hydrologic cycle, also known as the water cycle, plays a crucial role in the Earth's natural processes. It involves the continuous movement of water between the Earth's surface, atmosphere, and underground reservoirs.
The importance of the hydrologic cycle can be understood by considering its various functions:
Transport: The hydrologic cycle facilitates the transport of water across the Earth's surface, including rivers, lakes, and oceans. This movement of water is vital for the distribution of nutrients, sediments, and organic matter, which are essential for the functioning of ecosystems.
Weathering and Contaminant Transport: Water plays a significant role in weathering processes, such as erosion and dissolution of rocks and minerals. It also acts as a carrier for contaminants, pollutants, and nutrients, influencing their transport through the environment.
Energy Balance: Water has a high heat capacity, which means it can absorb and store large amounts of heat energy. This property helps regulate the Earth's temperature and climate by transporting heat through evaporation, condensation, and precipitation.
Greenhouse Gas: Water vapor is a major greenhouse gas that contributes to the Earth's natural greenhouse effect. It absorbs and re-emits thermal radiation, trapping heat in the atmosphere. Approximately 80% of the atmospheric greenhouse effect is attributed to water vapor.
Life: Water is vital for supporting life on Earth. It provides a habitat for numerous organisms and serves as a medium for various biological processes. Terrestrial life forms, including plants, animals, and humans, rely on water availability for their survival, growth, and reproduction.
It is important to note that while water is critical for most terrestrial life forms, human beings have developed technologies and systems that allow them to inhabit regions with limited water availability. However, water still remains a fundamental resource for human societies, and the hydrologic cycle plays a crucial role in ensuring its availability and sustainability.
To know more about hydrologic cycle click here:
https://brainly.com/question/13729546
#SPJ4