Mr. Green mowed 2 5 of hi lawn. Hi on mowed 1 3 of it. Who mowed mot of the lawn? How much of the lawn till need to be mowed? Solve thi on paper. Then check your work on Zearn. Who mowed mot of the lawn? i greater than o mowed mot of the lawn
Answers
Mr. Green mowed more of the lawn. 2/5 of the lawn still needs to be mowed, according to the concept of proportion or ratio.
To get this answer, we can first set up a proportion or ratio to compare how much of the lawn each person mowed. Mr. Green mowed 2/5 of his lawn and his son mowed 1/3 of it.
2/5 = x/1, We can then cross multiply to solve for x.
2x = 5/3 x = 5/6 Since 5/6 is greater than 1/3, this means that Mr. Green mowed more of the lawn than his son. This means that 2/5 of the lawn still needs to be mowed.
To know more about proportion refer to the link brainly.com/question/13419413
#SPJ4
Related Questions
2. Find the sum, S, for the arithmetic series described? Remember to use the formula

Answers
Using the given formula, the sum of S(n) for the arithmetic series is 565.5.
In the given question we have to find the sum S(n) for the arithmetic series
The given formula is S(n)=n/2 {a(1)+a(n)}
The given values are a(1)=12, a(n)=75,n=13
We just have to put values in given formula
S(n)=n/2 {a(1)+a(n)}
S(n)=13/2 (12+75)
S(n)=6.5*87
S(n)=565.5
Hence, the sum of S(n) for the arithmetic series described is 565.5.
To learn more about arithmetic series link is here
brainly.com/question/8919590
#SPJ1
In single-factor experiments, if Στ. = 0 i=1 where T, resembles the effect of the ith level, then Ti all treatment means must be equal. Select one: True False
Answers
True, if Στ = 0 in a single-factor experiment, then all treatment means must be equal.
In single-factor experiments, if the sum of the treatment effects (Στ) is equal to zero (Στ = 0) for all levels (i=1 to n), then it implies that all treatment means (Ti) must be equal.
In a single-factor experiment, a single independent variable (factor) is manipulated, and its effect on the dependent variable is studied across different levels or treatments.
The treatment effects (τ) represent the differences in the mean response between each treatment level and the overall mean of the dependent variable.
If the sum of these treatment effects (Στ) is equal to zero (Στ = 0), it means that the positive and negative differences cancel each other out, resulting in a net effect of zero.
If Στ = 0, it implies that the total treatment effect across all levels is balanced, indicating that there are no systematic differences between the treatment means.
Consequently, if all treatment effects cancel out and Στ = 0, it implies that the means of all treatment levels (Ti) must be equal since any deviations from the overall mean are offset by equal and opposite deviations in other treatment levels.
Therefore, if Στ = 0 in a single-factor experiment, it indicates that all treatment means must be equal.
Know more about the treatment click here:
https://brainly.com/question/32109622
#SPJ11
Why is 64 a perfect square, but 60 is not?
Answers
Answer:
Step-by-step explanation:
because it needs to be a number times itself to be be a perfect square
Answer:
The square root of 64 gives the original number. If you square 8, you get 64, making it a perfect square. However, 60's square root is only approximate to 60 and very close to it when squared. Therefore, it cannot be a perfect square.
Hope it helps.
Show that the average value of x^2 in the one-dimensional infinite potential energy well is L^2(1/3 - 1/2n^2 pi^2
Answers
To find the average value of x^2 in the one-dimensional infinite potential energy well, we need to use the wave function for the particle in the well, which is given by:
ψn(x) = sqrt(2/L) * sin(nπx/L)
where n is a positive integer and L is the width of the well.
The probability density of finding the particle at a position x is given by:
|ψn(x)|^2 = (2/L) * sin^2(nπx/L)
Using this probability density, we can find the average value of x^2 by integrating x^2 multiplied by the probability density over the entire well:
= ∫(x^2)(2/L) * sin^2(nπx/L) dx from 0 to L
Using the trigonometric identity sin^2θ = (1/2) - (1/2)cos(2θ), we can simplify the integral as follows:
= (1/L) * ∫(x^2) dx from 0 to L - (1/L) * ∫(x^2)cos(2nπx/L) dx from 0 to L
The first integral is simply the average value of x^2 over the entire well, which is L^2/3. The second integral can be evaluated using integration by parts, resulting in:
(1/L) * ∫(x^2)cos(2nπx/L) dx = (L^2/2nπ)^2 * [sin(2nπx/L) - (2nπx/L)cos(2nπx/L)] from 0 to L
Plugging this into our original equation, we get:
= L^2/3 - (L^2/2nπ)^2 * [sin(2nπ) - 2nπcos(2nπ)] + (L^2/2nπ)^2 * [sin(0) - 0]
Since sin(0) = 0 and sin(2nπ) = 0, the equation simplifies to:
= L^2/3 - (L^2/2nπ)^2 * (-2nπ) = L^2/3 + (L^2/2) * n^2π^2
Finally, we can substitute L^2/4π^2 for 1/2 in the expression above to get:
= L^2/3 + L^2/4 * n^2π^2 - L^2/4π^2 * n^2π^2
Simplifying further, we get:
= L^2/3 - L^2/4π^2 * n^2π^2
which is the desired result.
To show that the average value of x^2 in a one-dimensional infinite potential energy well is L^2(1/3 - 1/2n^2 π^2), we need to follow these steps:
Step 1: Define the wave function.
For an infinite potential energy well of width L, the wave function Ψ_n(x) is given by:
Ψ_n(x) = √(2/L) sin(nπx/L)
Step 2: Compute the probability density function.
The probability density function, ρ(x), is given by the square of the wave function, |Ψ_n(x)|^2:
ρ(x) = (2/L) sin^2(nπx/L)
Step 3: Calculate the expectation value of x^2.
The expectation value (average value) of x^2, denoted as , is given by the integral of the product of x^2 and the probability density function over the width of the well (0 to L):
= ∫[x^2 ρ(x)] dx from 0 to L
Step 4: Perform the integral.
= ∫[x^2 (2/L) sin^2(nπx/L)] dx from 0 to L
After solving this integral, you will find that:
= L^2(1/3 - 1/2n^2 π^2)
This confirms that the average value of x^2 in the one-dimensional infinite potential energy well is indeed L^2(1/3 - 1/2n^2 π^2).
Learn more about :
one-dimensional infinite potential : brainly.com/question/30843844
#SPJ11
which of the following equations represent a line that is parallel y=5x-4 ad passes through the point (3,4)
Answers
The equation that represents a line that is parallel to y = 5x - 4 and passes through the point (3, 4) is y = 5x - 11.
How to write the point-slope form equation?We are given that
The slope of given line = 5And passed through point (3, 4)Slope of parallel lines is the same, so slope of the line parallel to the given line will be m = 5
We have the slope and a point, using the point-slope form we can write the equation of the line:
\(\rightarrow\text{y} - 4 =5 \ (\text{x} - 3)\)
\(\rightarrow\text{y} = 5\text{x} - 15 + 4\)
\(\rightarrow\bold{y = 5x - 11}\)
Hence, the equation that represents a line that is parallel to y = 5x - 4 and passes through the point (3, 4) is y = 5x - 11.
To know more about point-slope form, visit:
https://brainly.com/question/28895991
Your question is incomplete. The complete question is-
Which of the following equations represents a line that is parallel to y = 5x - 4 and passes through the point (3, 4)?
A. y = 5x - 7
B. y = 5x + 19
C. y = -1/5x -4
D. y = 5x - 11
1. Which of the following lines is parallel to the line y = -3/2x +1 and contains the point (4,2)?

Answers
Answer:
The answer should be y = -\(\frac{3}{2}\)\(x\) + 8, but its not one of the answers, so check the question again.
Step-by-step explanation:
If the second line is parallel, then the gradient will be the same:
y = -\(\frac{3}{2}\)\(x\) + c
Find what c is (y-intercept) by inputting the given point into the equation (4,2):
2 = (-\(\frac{3}{2}\) x 4) + c
2 = -6 + c
c = 8
Which value is a solution to the inequality 13 – x ≤ –29?
Answers
Step-by-step explanation:
13-x<-29-x <-29-13-x < -42x <42hope it helps.stay safe healthy and happy..Answer: x\(\geq\)42
Step-by-step explanation: 13-x-13≤–29-13 -x+13-13≤-29-13 -x≤29+13 x\(\geq\)42

Ivan and Adeline are in a classroom with a chalkboard. They are standing on different halves of the board, and on each half, the number is written. When Ivan's teacher gives a signal, Ivan multiplies the number on his side of the board by and writes the answer on the board, erasing the number he started with. Adeline does the same on each signal, except that she multiplies by . The teacher gives 10 signals in total. How many times (including the initial number) do Ivan and Adeline have the same number written on the board
Answers
Answer:
6 times
Step-by-step explanation:
Given
\(Start=2\)
\(Ivan=-2\) ---- i.e. multiplies by -2
\(Adeline = 2\) --- i.e. multiplies by 2
\(n=10\) --- signals
Required
Number of times they have the same number
First, we list out all results of Ivan calculations.
To calculate Ivan's list, we simply multiply the current term by -2.
The 10 signals together with the first term, means there will be 11 terms in total
So, we have:
\(Ivan = \{2,-4,8,-16,32,-64,128,-256,512,-1024,2048\}\)
Next, list Adeline's
\(Adeline = \{2,4,8,16,32,64,128,256,512,1024,2048\}\)
Compare the elements of the two lists, we have:
\(Common= \{2,8,32,128,512,2048\}\)
Hence, they have the same number 6 times.
Which of the following ratios is equivalent to 1/3?
A.1:3
B.2:3
C.2:1
D.1:2
Answers
Answer:
A
Step-by-step explanation:
because 1/3 is like saying 1 out of 3 and 1:3 is like saying 1 for every 3. same thing.
it is known that the population variance equals 522. what is the sample size that needs to be taken if the desired margin of error is 4 or less with 0.95 probability?
Answers
If the intended margin of error equals 4 less than with a 0.95 probability, the sample size is 126.
The \(\alpha\) level is calculated by subtracting 1 from the confidence interval and dividing by 2.
\(\alpha\) = (1 - 0.95) ÷ 2
\(\alpha\) = 0.025
Find z inside the Z-table because it has a p-value of 1 - \(\alpha\).
So, z = 1.96 with a p-value = 1 - 0.025 = 0.975.
Now, consider that margin of error M.
M = z × (σ ÷ √n)
The standard deviation is determined as the square root of variance.
σ = √522 = 22.84
With a 0.95 probability, the sample size that requires to be accepted if the expected margin of error is 4 or less is,
The sample size of at least n, in which n is encountered when M = 4. So,
M = z × (σ ÷ √n)
4 = 1.96 × (22.84 ÷ √n)
4√n = 1.96 × 22.84
√n = (1.96 × 22.84) ÷ 4
√n = 11.1916
n = (11.1916)²
n = 125.25 ≈ 126
A sample size of at minimum 126 people is required.
Learn more about the probability at
https://brainly.com/question/15121653?referrer=searchResults
#SPJ4
Cara has 3 1/4 pounds of ham. If Timothy has 4/5 as much ham as Cara, how many pounds of ham does Timothy have?
Answers
Answer: 2.6 or 2 2/3
Step-by-step explanation:
3 1/4 = 3.25
3.25/5 = 0.65
0.65 x 4 = 2.6 or 2 2/3
3.25/5 = 0.65
0.65 x4 = 2.6 or 2 2/3
1. How many centavos are there in P 125. 75?
Answers
As there are 100 centavos in one Philippine Peso, there are 12,575 centavos in P 125.75.
what is permutation ?An arrangement of things in a particular order is known as a permutation in mathematics. A permutation of a group of things is a particular technique to arrange them in a particular sequence, where each object is used exactly once. Consider the trio of letters A, B, and C as an example. This set can be permuted in six different ways: ABC, ACB,BAC, BCA, CAB, and CBA
These combinations each show a different way to arrange the letters in the set.
given
The Philippine Peso, represented by the letter "P," is the official unit of money in the Philippines.
One Philippine Peso is equal to 100 centavos.
We must multiply P 125.75 by 100 to convert it to centavos:
P = 12,575 centavos (125.75 x 100).
As there are 100 centavos in one Philippine Peso, there are 12,575 centavos in P 125.75.
To know more about permutation visit:
https://brainly.com/question/1216161
#SPJ1
pls, don't fight like my last one- What is the missing numerator? blank over seven-plus thirteen over fourteen equals one and three fourteenths 11, 5, or 2
Answers
Hi!
I'm 90% sure that the answer is: 5
Sorry its its wrong!
Hope this helps!
Let me know if you need anymore help!
Yours truly,
~~~PicklePoppers~~~Answer:
5
Step-by-step explanation:
We would like to estimate the proportion of uf students who owns a scooter to within 1% of the truth, with 95% confidence. We believe around 20% of students do. How many students should be sampled?.
Answers
We can estimate that (A) 3458 students should be sampled using proportions.
What are proportions?A proportion is an equation that sets two ratios at the same value. For instance, if there is 1 boy and 3 girls, you may express the ratio as 1: 3 (there are 3 girls for every boy), meaning that there are 1 in 4 boys and 3 in 4 girls.So, we have the following confidence interval of proportions for a sample of n people who were surveyed with a probability of success of π and a level of confidence of.
π ± z√[π(1-π)/n]Where the z-score with a p-value of x is designated as 1+a/2.
The margin of error:
M = z√[π(1-π)/n]So, with respect to M = 0.01, the sample size is n.
Now solve:
M = z√[π(1-π)/n]0.01 = 1.96√[0.01(0.09)/n]0.01√n = 1.96√0.01(0.09)√n = [1.96√0.1(0.9)]/0.01(√n)² = ([1.96√0.1(0.9)]/0.01)²n = 3457.4Rounding off: 3458
Therefore, we can estimate that (A) 3458 students should be sampled using proportions.
Know more about proportions here:
https://brainly.com/question/19994681
#SPJ4
The complete question is given below:
We would like to estimate the proportion of UF students who own a scooter to within 1% of the truth, with 95% confidence. We believe around 10% of students do. How many students should be sampled? Group of answer choices
A. 3458
B. 34575
C. 38416
D. 3842
The formula below gives the sum of the degrees, S, of the interior angles of a polygon. If n represents the number of sides, which equation solves for n?
S = (n-2) x 180
F. n = s/180 + 2
G. n = 180s + 2
H. n = s+2/180
J. n = (s+2) x 180
Answers
Answer: F. n = s/180 + 2
Step-by-step explanation:
To find the equation that solves for n, we will isolate the n variable.
Given:
S = (n - 2) x 180
Divide both sides of the equation by 180:
\(\frac{S}{180}\) = n - 2
Add 2 to both sides of the equation:
\(\frac{S}{180}\) + 2 = n
Reflexive property:
n = \(\frac{S}{180}\) + 2
F. n = s/180 + 2
NEED ASAP
What type of number is square root 2 (I put in square root because I don´t have a square root symbol, its kinda like this /```` almost but a slant at the end)
What I mean by type of number is like whole number, rational, irrational, integer, what is it?
Answers
Answer:
Step-by-step explanation:
Rational numbers come in 3 different forms; integers, fractions and terminating or recurring decimals such as
1
3
.
Irrational numbers are quite 'messy'. They can't be written as fractions, they are never-ending, non-repeating decimals. An example of this is the value of
π
.
A whole number can be called an integer and is either a positive or negative number, or zero. An example of this is
0
,
1
and
−
365
.
PLSSS HELP ME
(5x5)/[10x22]-4\52
Answers
Answer: 21/572
\(\frac{5.5}{10 . 22} - \frac{4}{52}\)
The dots stand for multiplication
Multiply
-3.7
A. -21
B. -11
C. 21
D. 11
Answers
Answer:
A
Step-by-step explanation:
negative times a postive is always negative unless squared
Answer:
A. -21
Step-by-step explanation:
Note that when you multiply a negative with a positive number, your answer will be negative. Multiply as usual:
-3 * 7 = -21
A. -21 is your answer.
~
What’s the answer Answer???

Answers
Answer:
B.
Step-by-step explanation:
I might have gotten this wrong but my mind was telling me it was B hope this helped.
Thanks for your time.
(>.<) <3 Kris
Solve the equation
-2(2x - 4) = 4x
Write it step by step. I really need help
Answers
Answer:
x = 1
Step-by-step explanation:
Step 1: Simplify both sides of the equation.
−2(2x−4)=4x
(−2)(2x)+(−2)(−4)=4x(Distribute)
−4x+8=4x
Step 2: Subtract 4x from both sides.
−4x+8−4x=4x−4x
−8x+8=0
Step 3: Subtract 8 from both sides.
−8x+8−8=0−8
−8x=−8
Step 4: Divide both sides by -8.

The Segment Addition Postulate involves the addition of two segments with a common endpoint. Which conditions regarding the endpoints must be met? select all that apply.
they are coplanar
one point is between the other two
they are non-coliner
the points are randomly place on the line
they are coliner
Answers
Answer:
they are collinear
one point is between the other two
Step-by-step explanation:
The conditions regarding the endpoints that must be met are;
Option B; One point is between the other two.
Option E; They are colinear.
The segment addition postulate states that if for example we have two points on a given line segment with endpoints, X and Z, then there will be a third point Y that resides on the line segment XZ if and only if the distances between the points meet the requirements of the equation XY + YZ = XZ.Let's look at the options;
Option A; This is not correct because coplanar means in the same plane but what we need is colinear points but coplanar points are not always colinear.Option B; This is correct because as seen above, point Y is between Point X and Z.Option C; This is not correct because they have to be colinear and not non-colinear.Option D; This is not correct because random placement may lead to non conformation with the equality of segments.Option E; This is correct because they have to be on the same line and are colinear.Read more at; https://brainly.com/question/17491162
what is the most convenient method to solve the quadratic equation ( x + 4 )² = 15
A. extracting square root
B. factoring
C. completing the square
D. quadratic formula
Answers
Answer:
Factoring
Step-by-step explanation:
(x+4)² = 15
x² + 8x + 16 = 15
x² + 8x + 1 = 0
\(x = \frac{ - 8 + \sqrt{64 - 4} }{2} = - 4 + \sqrt{15} \\ \\ x = \frac{ - 8 - \sqrt{64 - 4} }{2} = - 4 - \sqrt{15} \)
I hope this helps you :)
Answer:
B
Step-by-step explanation:
Is this a function of not??
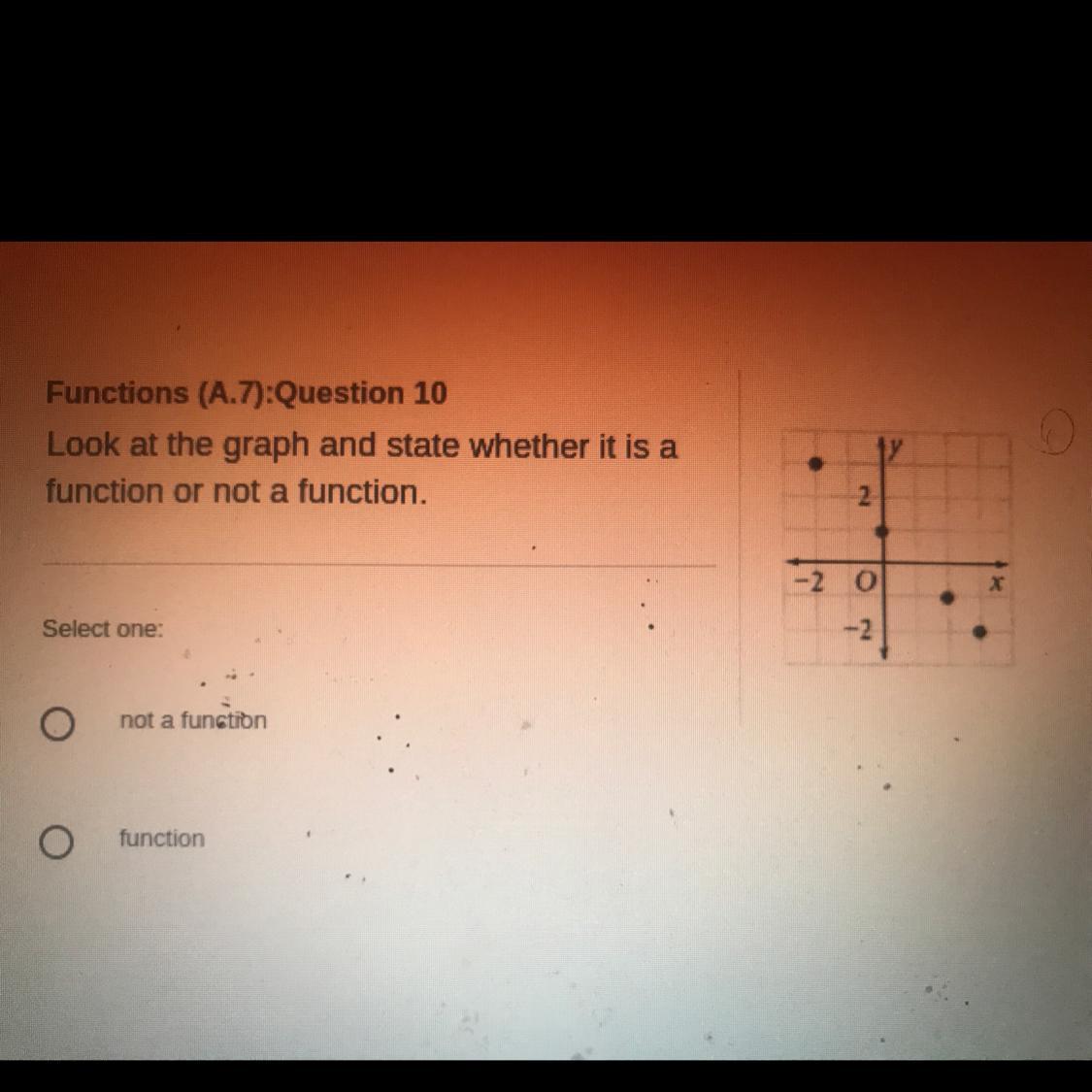
Answers
Answer:
Yes
Step-by-step explanation:
A vertical line would only touch 1 point at a time. Please vote brainliest
Answer:
no it is not fiction
Step-by-step explanation:
im not very sure so wait on another answer
In a local election, one candidate received 43% of the votes. Only 87 people voted in the election. Which proportion could be used to find how many votes the candidate received
Answers
we can use the proportion: (Number of votes received by the candidate) / (Total number of votes) = 43% / 100%. the candidate received approximately 37.41 votes.
In the given local election, the candidate received 43% of the votes. To determine the number of votes they obtained, we need to use a proportion. A proportion is an equation that states that two ratios are equal.
Let's represent the number of votes received by the candidate as "x." The total number of votes cast in the election is stated as 87.
We can set up the proportion:
x / 87 = 43% / 100.
To solve for "x," we can cross-multiply:
100 * x = 43% × 87.
Simplifying further, we have:
x = (43/100) × 87.
By multiplying the fraction (43/100) by 87, we can determine the number of votes received by the candidate. Evaluating this expression, the candidate received approximately 37.41 votes.
learn more about proportion here:
https://brainly.com/question/31548894
#SPJ11
Please Help
It took Alex 6 weekends to paint 15 fences. At that rate, how long will it take her to paint 20 fences?
Answers
help i can't do this and i rather not fail math

Answers
Explanation:
Answer:
(0,5)
Step-by-step explanation:
c'mon just watch a totorial it's easy.
(Q2) The set of line segments _____ meet the requirements to form a triangle.8 cm4 cm3 cm
Answers
To form a triangle, the set of line segments must satisfy the triangle inequality theorem, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. Therefore, we need to check if the given line segments 8 cm, 4 cm, and 3 cm meet this requirement.
We can start by checking if the sum of the two smaller sides (3 cm and 4 cm) is greater than the largest side (8 cm). 3 cm + 4 cm = 7 cm, which is less than 8 cm. Therefore, these three line segments cannot form a triangle.
In general, for a set of line segments to form a triangle, the largest side must be smaller than the sum of the other two sides. In this case, the line segment of 8 cm is too long compared to the other two sides, which makes it impossible to form a triangle.
In conclusion, there are no line segments that meet the requirements to form a triangle with lengths of 8 cm, 4 cm, and 3 cm.
learn more about the inequality theorem here: brainly.com/question/1163433
#SPJ11
Find the area of a 1/4 inch diameter circle. Express the answer to the nearest thousandth square inch. _______ square inch g
Answers
The area of the 1/4 inch diameter circle, expressed to the nearest thousandth square inch, is 0.049 square inches.
The formula to find the area of a circle is A = π\(r^2\).
Where,
r is the radius of the circle.
Since the diameter of the circle is given as 1/4 inch, the radius is 1/8 inch (radius = diameter/2).
Substituting the value of r in the formula, we get A = π(1/8) = π/64 square inches.
Now, to express the answer to the nearest thousandth square inch, we need to calculate the value of π/64 and round it to the nearest thousandth. Using a calculator, we get π/64 = 0.04908738521...
The area of a circle with a 1/4 inch diameter, we'll use the formula A = π\(r^2\), where A is the area, and r is the radius.
First, we need to find the radius.
Since the diameter is 1/4 inch, the radius (r) is half of that: 1/8 inch.
Now, we'll calculate the area: A = π\(\frac {1}{8} ^{2}\) = π(1/64) ≈ 0.0123 square inches. So, the area of the circle is approximately 0.0123 square inches to the nearest thousandth.
Rounding this value to the nearest thousandth gives us 0.049 square inches.
For similar question on area:
https://brainly.com/question/27683633
#SPJ11
Derive tan(A+B)+tan(A+B)- tan(A+B)+tan(A+B).
Answers
Step-by-step explanation:
the answer will be zero 0
a satellite flies 73680 73680 miles in 9.21 9.21 hours. how long would it take to fly 82000 82000 miles?
Answers
Therefore, it would take approximately 10.246 hours for the satellite to fly 82000 miles.
We can use the concept of unit rate to solve this problem.
Given that the satellite flies 73680 miles in 9.21 hours, we can calculate the unit rate of the satellite's speed:
Unit Rate = Distance / Time
= 73680 miles / 9.21 hours
≈ 8004.55 miles/hour
Now, to find out how long it would take for the satellite to fly 82000 miles, we can use the unit rate:
Time = Distance / Unit Rate
= 82000 miles / 8004.55 miles/hour
Calculating this value:
Time ≈ 10.246 hours
To know more about satellite,
https://brainly.com/question/28550904
#SPJ11