Mrs Buan has a wooden in the shape of a cube whose side is 1.5 meters she wants paint the outer surface how much area will be painted?
Answers
Answer:
If the side of the cube is 1.5 meters, then the total surface area to be painted is the sum of the areas of all six faces of the cube.
The formula for the surface area of a cube is given by:
Surface Area = 6s^2
where s is the length of one side of the cube.
Substituting s = 1.5m into the formula, we get:
Surface Area = 6(1.5)^2
= 6(2.25)
= 13.5 square meters
Therefore, Mrs. Buan will need to paint a total of 13.5 square meters of surface area on her wooden cube.
Related Questions
Data were taken from a sample of students. StatKey was used to conduct a randomization test for the difference in two means. Given a p-value of 0.072 the null hypothesis was not rejected. Later, data from the entire population were available.
Requried:
a. Using that population data, the researchers found that there was a difference in how many hours per week adult learners and traditional students planned to devote to STAT 200.
b. Was a Type I or Type II error committed here?
Answers
a. Based on the population data, the researchers found that there was a difference in how many hours per week adult learners and traditional students planned to devote to STAT 200. This suggests that the initial conclusion drawn from the randomization test (null hypothesis not rejected) may have been incorrect.
b. In this case, a Type II error was likely committed. A Type II error occurs when the null hypothesis is not rejected, even though it is false (i.e., there is a difference in the population means). Since the null hypothesis was not rejected initially based on the randomization test, but the population data revealed a difference, it indicates that the researchers failed to detect the true difference in means, leading to a Type II error.
Learn more about null hypothesis here:
https://brainly.com/question/30821298
#SPJ11
Find the measure of the arc or central angle indicated. Assume that lines which appear to be diameters are actual diameters

Answers
(11) The measure of angle IGJ is 46⁰.
(12) The measure of arc JH is determined as 138⁰.
What is the measure of the arc or central angle indicated?The measure of the arc or central angle indicated is calculated as follows;
(11) The measure of angle IGJ is calculated as follows;
m∠LGK = arc angle LK (interior angle of intersecting secants)
m∠LGK = 48⁰
m∠HGI = m∠LGK = 48⁰ (vertical opposite angles are equal)
m∠IGJ + m∠HGI + m∠JGK = 180⁰ (sum of angles in a straight line)
m∠IGJ + 48⁰ + 86⁰ = 180
m∠IGJ = 180 - 134
m∠IGJ = 46⁰
(12) The measure of arc JH is calculated as follows;
arc JH = arc JI + arc IH
arc JI = arc FG
arc FG = 180 - 137⁰ (sum of angle in a straight line)
arc FG = 43⁰
arc FG = arc JI (vertical opposite angles are equal)
arc JH = arc JI + arc IH
arc JH = 43⁰ + 95⁰
arc JH = 138⁰
Learn more about arc angles here: https://brainly.com/question/30543683
#SPJ1
Solve for x. 3(x + 4) + 2x = 5

Answers
Answer:
x=-7/5
Good Luck!!!
Answer:
x= -7/5 trust me
Step-by-step explanation:
i worked the equation out for u
ANYONE PLEASE HELP ME WITH MY MATH HOMEWORK I REALLY NEED THE ANSWER RIGHT NOW BECAUSE I HAVE TO PASS THIS LATER I HOPE Y'ALL CAN HELP ME:(
I'LL MARK YOU AS THE BRAINLIEST!

Answers
Answer:
1.
h⁸
2.
-14a⁵b²
A line has a y-intercept of 6 and a slope of 10. What is its equation in slope-intercept form?
Answers
Answer:
ewrwr33r2ewrwrerw3t
Step-by-step explanation:
1202
3ab + 2b; a
-5 and b = 4
Answers
Answer:
52, if I understand the question correctly.
Step-by-step explanation:
Is this:
3ab + 2b
and we want to know the value when a = -5 and b = 4?
If so:
3ab + 2b
3(-5)(4) + 2(4)
-60 + 8 = -52
-52, if I understand the question correctly.
3ab + 2b
and we want to know the value when a = -5 and b = 4
If so:
3ab + 2b
3(-5)(4) + 2(4)
-60 + 8 = -52
Values 3ab + 2b are fundamental and fundamental beliefs that guide and motivate attitudes and actions. They help us determine what is important to us. Value is the goods, services or monetary value of a thing or person. An example of value is the amount an appraiser declares after appraising a home. An example of value is the value of a consultant's contribution to a committee. noun.
Values reflect our sense of right and wrong. They help us grow and develop. They help shape the future we want. The choices we make every day reflect our values.
Learn more about value here
https://brainly.com/question/843074
#SPJ1
Nick just received his test back. He scored a 24 out of a possible 60 points. His teacher told him the mean score on the test was a 35, with a standard deviation of 6. What is nick's z-score?
Answers
Nick's z -score is -1.833.
According to the given question.
Total points scored by Nick, x = 24
Mean score for the test was, μ = 35
Standard deviation, σ = 6
As, we know that z-score can be caluated by using the formula
z = (x - μ)/σ
Where, x is a score
μ is mean score
σ is standard deviation
z is z-score
Therefore, Nick's z-score is given by
z = (24 - 35)/6
z = -1.833
ence, Nick's z -score is -1.833.
Find out more information about z-score here:
https://brainly.com/question/15016913
#SPJ4
Boden is making a prize wheel for the school fair. The ratio of winning spaces to losing spaces is shown in the diagram. The table shows the number of winning and losing spaces that could be on the wheel. Based on the ratio, complete the missing values in the table.

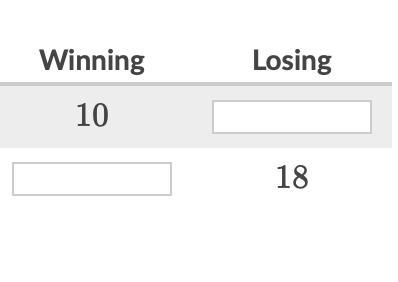
Answers
The correct answers are Losing 12; Winning 15
Explanation:
The ratio of winning to losing is 5: 6 or 5/6. This means for every 5 winning spaces in the wheel there are 6 losing spaces. This ration should be used to complete the values of the table.
1. The first row shows there are 10 winning and you need to calculate the number of losing spaces. The process is shown below.
\(\frac{5}{6} = \frac{10}{x}\) - Express the ratios using fractions; use x to show the missing value
\(5x = 60\) - Cross multiply to find the value of x
\(x = 60 / 5\) - Solve the equation to find x
\(x = 12\) - The number of losing is 12 if there are 10 winning spaces
2. The second row shows there are 18 losing spaces, and you need to calculate the number of winning spaces. Repeat the process.
\(\frac{5}{6} = \frac{x}{18}\)
\(6x = 90\)
\(x = 90 / 6\)
\(x =15\) - The number of winning spaces is 15 if there are 18 losing spaces
Answer:
22 and 15 i know because i got it right on khan
Step-by-step explanation:
Please mark mine the brainliest !!
The average age of 6 men is 35 years and the average age of four of them is 32 year.
Find the ages of the remaining two ment one is 3 years older than the other.
Answers
Let's denote the ages of the two remaining men as x and x + 3 (since one is 3 years older than the other).
We know that the average age of 6 men is 35 years. So, the sum of their ages is 6 * 35 = 210 years.
We also know that the average age of four of them is 32 years. So, the sum of their ages is 4 * 32 = 128 years.
To find the sum of the ages of the two remaining men, we subtract the sum of the ages of the four men from the sum of the ages of all six men:
210 - 128 = 82 years.
Now, we can set up an equation to solve for the ages of the remaining two men:
x + (x + 3) = 82.
Combining like terms, we get:
2x + 3 = 82.
Subtracting 3 from both sides:
2x = 79.
Dividing both sides by 2:
x = 39.5.
So, one of the remaining men is 39.5 years old, and the other is 39.5 + 3 = 42.5 years old.
Answer the following questions based on the lambda-calculus term (λx. λy. y x) (λ. y). (a) Calculate its free variables using the FV function we discussed in class. Show the steps. Note that "y x" stands for a function application calling y with argument x. y (b) Use lambda calculus reduction to reduce the term to its normal form. Begin by renaming bound variables and show every step. (c) Describe what would go wrong if you did not rename bound variables.
Answers
a) The free variables of the given lambda-calculus term are {x, y}.
b) The normal form of the given lambda-calculus term is (λ. t) s.
c) In lambda calculus, calculating the free variables, renaming bound variables, and avoiding variable capture are crucial steps to accurately reduce terms and obtain the normal form.
What is lambda calculus?In lambda calculus, calculating the free variables, renaming bound variables, and avoiding variable capture are crucial steps to accurately reduce terms and obtain the normal form.
(a) To calculate the free variables of the lambda-calculus term (λx. λy. y x) (λ. y), we can use the FV function. The FV function recursively checks the variables in a lambda term, excluding the ones bound by lambda abstractions. Here are the steps to calculate the free variables:
Start with the given term: (λx. λy. y x) (λ. y)
Apply the FV function to each subterm:
FV((λx. λy. y x)) = FV(λx) ∪ FV(λy. y x) = {x} ∪ (FV(λy) ∪ FV(y x)) = {x} ∪ ({y} ∪ (FV(y) ∪ FV(x))) = {x} ∪ {y} ∪ {y, x} = {x, y}
FV((λ. y)) = FV(λ) ∪ FV(y) = ∅ ∪ {y} = {y}
Take the union of the free variables from the previous steps:
FV((λx. λy. y x) (λ. y)) = {x, y} ∪ {y} = {x, y}
Therefore, the free variables of the given lambda-calculus term are {x, y}.
(b) Now let's reduce the term to its normal form by renaming the bound variables:
Start with the given term: (λx. λy. y x) (λ. y)
Rename the bound variables:
(λx. λy. y x) (λ. y) [Rename x to z] (λz. λy. y x) (λ. y) [Rename y to w] (λz. λw. w x) (λ. y) [Rename x to v] (λz. λw. w v) (λ. y) [Rename y to u] (λz. λw. w v) (λ. u) [Rename u to t] (λz. λw. w v) (λ. t) [Rename v to s] (λz. λw. w s) (λ. t)
Perform the reductions:
(λz. λw. w s) (λ. t) [Apply (λz. λw. w s) to (λ. t)] (λw. w s)[z := (λ. t)] [Substitute z with (λ. t)] (λw. w s) [Substitute w with (λ. t)] (λ. t) s [Substitute s with (λ. t)]
The term (λ. t) s is in normal form because there are no more reducible expressions.
Therefore, the normal form of the given lambda-calculus term is (λ. t) s.
(c) If we did not rename bound variables during reduction, we could encounter variable capture or unintentional variable collisions. Variable capture occurs when a variable bound in a lambda abstraction clashes with a free variable in the context it is being substituted into, leading to incorrect results. By renaming bound variables, we ensure that each variable remains distinct and does not interfere with other variables in the expression. This allows us to correctly perform reductions and reach the desired normal form without any unintended side effects.
Therefore, In lambda calculus, calculating the free variables, renaming bound variables, and avoiding variable capture are crucial steps to accurately reduce terms and obtain the normal form.
To know more about lambda check the below link:
https://brainly.com/question/31019087
#SPJ4
A tree that is 12 feet tall is growing at a rate of 2 1/2
feet each year. A tree that is 15 feet tall is growing at a rate of 1 foot each year.
Enter the number of years it will take the two trees to reach the same height.
HELP please
Answers
The number of years taken for the tree to reach the same height found using mathematical expression is 2 years.
What is an expression?
Mathematical statements are called expressions if they have at least two terms that are related by an operator and contain either numbers, variables, or both. Addition, subtraction, multiplication, and division are all possible mathematical operations.
Let x be the number of years taken to reach the same height.
Height of the first tree after x years,
12 + 2.5(x)
Height of the second tree after x years,
15 + 1(x)
Given that both heights are the same after x years.
So,
12 + 2.5x = 15 + x
1.5x = 3
x = 2
Therefore the number of years taken for the tree to reach the same height is 2 years.
To learn more about expressions, follow the link.
https://brainly.com/question/1859113
#SPJ1
4. What is the slope of
the line?

Answers
Find the area of a sector with a central angle of 140° and a diameter of 9.6 cm. Round to the nearest tenth.
Answers
Answer:
\(A=28.1\ cm^2\)
Step-by-step explanation:
The area of a circular sector with a central angle θ and radius r is given by:
\(\displaystyle A=\frac{1}{2}r^2 \theta\)
Note: The angle must be in radians.
We need to find both the radius and the angle in radians.
Diameter = 9.6 cm
Radius r=Diameter/2=4.8 cm
Angle in radians= Angle in degrees*π / 180
θ = 140*π / 180 = 2.4435 rad
Now we calculate the area:
\(\displaystyle A=\frac{1}{2}(4.8)^2 2.4435\)
\(\boxed{A=28.1\ cm^2}\)
Help me geometry 7th grade

Answers
We know 7 becomes 3.5, which means the second rectangle is half the size of the first one (because 7 divided by 2 is 3.5)
Therefore, to find the missing measure, or x cm, you have to divide 4 by 2, which is 2
Therefore, x=2
The locus of point equidistant from three vertices of a triangle is……………
Answers
Answer:
circumcenter
Step-by-step explanation:
You want to know the name of the point equidistant from the vertices of a triangle.
CircumcircleThe circle that passes through the vertices of a triangle is called a "circumcircle". It circumscribes the triangle. Its center is equidistant from all points on the circle, so is equidistant from the triangle's vertices.
The point equidistant from the vertices of a triangle is the circumcenter.
__
Additional comment
The circumcenter is at the intersection of the perpendicular bisectors of the sides of the triangle.
can someone help with this?

Answers
Explanation:
The proof can make use of the ASA congruence postulate.
__
Statement . . . . Reason
AB║CD, AD║BC . . . . definition of parallelogram
∠ACD ≅ ∠CAB, ∠ACB ≅ ∠CAD . . . . alternate interior angles theorem
ΔACD ≅ ΔCAB . . . . ASA congruence postulate
Please explain easily or make sure you have the answer right

Answers
Answer:
D. 5:9
Because it's 5 sixth grade students and 9 seventh grade students so it asked you what is the ratio of sixth grade students to seventh grade students on the team.
Tessa bought stock in a restaurant for $137.00. Her stock is now worth $201.39. What is the percentage increase of the value of Tessa's stock?
Answers
The percentage increase of the value of Tessa's stock is 47%.
According to the question,
We have the following information:
Tessa bought stock in a restaurant for $137.00. Her stock is now worth $201.39.
We know that the following formula is used to find the percentage increase if the final and initial amount for any question is given:
Percentage increase = 100*(final-initial)/initial
Percentage increase = 100*(201.39-137.00)/137.00
Percentage increase = 100*64.38/137.00
Percentage increase = 6438/137.00
Percentage increase = 46.99% or nearly 47%
(We have rounded off this percentage in the final result.)
Hence, the percentage increase of the value of Tessa's stock is 47%.
To know more about percentage increase here
https://brainly.com/question/26080842
#SPJ1
Pls help me:
1) 3/5 ÷ 3=
2 )2÷ 2/5=
Answers
1) 0.2
2) 5
Check the image below

chance of failure is independent of another's failure, what would the individual failure rate need to be so that our of 20 users only 20% failed
Answers
The individual failure rate needs to be approximately 3.33% for only 20% of 20 users to fail, assuming that the probability of failure is independent of another's failure.
If the chance of failure is independent of another's failure, it means that the probability of each individual failing is the same, and we can assume that the failures follow a binomial distribution.
Let p be the probability of an individual failing, and n be the number of trials (in this case, the number of users, n = 20).
The probability of exactly k failures out of n trials is given by the binomial probability formula:
\(P(k) = (n choose k) \times p^k \times (1-p)^{(n-k)\)
where (n choose k) is the binomial coefficient, equal to n! / (k! × (n-k)!).
To find the individual failure rate needed for 20% of 20 users to fail, we need to solve for p such that P(4) = 0.2, where k = 4 is the number of failures we want to allow.
P(4) = (20 choose 4) \(\times p^4 \times (1-p)^{(20-4) }= 0.2\)
Using a binomial calculator or software, we can solve for p and get:
p ≈ 0.0333 or 3.33%
for such more question on probability
https://brainly.com/question/13604758
#SPJ11
what is 950% as a fraction
Answers
Answer:
9 1/2
Step-by-step explanation:
\(\frac{950}{100}=9\frac{50}{100} = 9 \frac{1}{2}\)
Find an LU factorization of the matrix A (with L unit lower triangular). A=
⎣
⎡
4
−8
10
−8
8
−4
3
5
−7
7
6
−7
0
3
−3
⎦
⎤
Answers
The LU factorization of matrix A is A = LU, where L = [[1, 0, 0], [-2, 1, 0], [1.5, -3, 1]] and U = [[4, -8, 10], [0, 24, -27], [0, 0, -12.5]].
Let's go step by step to find the LU factorization of matrix A.
Matrix A:
A =
[4, -8, 10]
[-8, 8, -7]
[6, -7, 3]
Step 1:
Initialize the L matrix as an identity matrix of the same size as A.
L =
[1, 0, 0]
[0, 1, 0]
[0, 0, 1]
Step 2:
Perform Gaussian elimination to obtain U.
- Multiply the first row of A by (1/4) and replace the first row of A with the result.
A =
[1, -2, 2.5]
[-8, 8, -7]
[6, -7, 3]
- Subtract 8 times the first row of A from the second row of A and replace the second row of A with the result.
A =
[1, -2, 2.5]
[0, 24, -27]
[6, -7, 3]
- Subtract 6 times the first row of A from the third row of A and replace the third row of A with the result.
A =
[1, -2, 2.5]
[0, 24, -27]
[0, 5, -12.5]
Step 3:
Update the L matrix based on the operations performed during Gaussian elimination.
L =
[1, 0, 0]
[0, 1, 0]
[0, 0, 1]
Step 4:
The resulting matrix A is the upper triangular matrix U.
U =
[1, -2, 2.5]
[0, 24, -27]
[0, 5, -12.5]
Therefore, the LU factorization of matrix A is:
L =
[1, 0, 0]
[0, 1, 0]
[0, 0, 1]
U =
[1, -2, 2.5]
[0, 24, -27]
[0, 5, -12.5]
learn more about LU factorization of matrix from this link.
https://brainly.com/question/33069156
#SPJ11
Input Output Which ordered pair needs to be removed in order for the mapping to represent a function? -3 4 O (-3, 4) O (-2, -1) O (1, -3) 0 2 -2 O (3,7) -3 →_1 3 7
Answers
Answer:
Acording to my brain the answer is (-2,-1) In other Words The correct answer is Option B
Step-by-step explanation:
God Bless :)
The ordered pair that needs to be removed in order for the mapping to represent a function is either: (-2, -1) or (2, -2).
What is a function?The term "function" refers to a relationship between a set of inputs and outputs. Just one output is connected to each input in a function, which connects inputs in that manner. Every function has a domain, co-domain, and range.
To represent a function, each input (x-value) must be associated with only one output (y-value). Therefore, in order for the mapping to represent a function, we need to remove any ordered pairs that violate this rule.
Looking at the options given, we can see that only one option has a repeated x-value:
O (-2, -1) and 0 (2, -2)
Both of these ordered pairs have the same x-value (either -2 or 2), but different y-values. This means that they violate the rule that each input must be associated with only one output.
Therefore, the ordered pair that needs to be removed in order for the mapping to represent a function is either:
O (-2, -1)
or
0 (2, -2)
We need to remove one of these ordered pairs to make the mapping a function.
To learn more about the function;
brainly.com/question/28303908
#SPJ7
a) estimate the area under the graph of f(x) = 5 cos(x) from x = 0 to x = /2 using four approximating rectangles and right endpoints. (round your answers to four decimal places.)
Answers
The estimated area under the graph of f(x) = 5 cos(x) from x = 0 to x = π/2 using four approximating rectangles and right endpoints is approximately 0.8916.
To estimate the area under the graph of f(x) = 5 cos(x) from x = 0 to x = π/2 using four approximating rectangles and right endpoints, we can use the right Riemann sum method.
The width of each rectangle, Δx, is given by the interval width divided by the number of rectangles.
In this case, Δx = (π/2 - 0)/4 = π/8.
To calculate the right endpoint values, we evaluate f(x) at the right endpoint of each rectangle.
For the first rectangle, the right endpoint is x = π/8.
For the second rectangle, the right endpoint is x = π/4.
For the third rectangle, the right endpoint is x = 3π/8.
And for the fourth rectangle, the right endpoint is x = π/2.
Now, let's calculate the area for each rectangle by multiplying the width (Δx) by the corresponding height (f(x)):
Rectangle 1: Area = f(π/8) * Δx = 5cos(π/8) * π/8
Rectangle 2: Area = f(π/4) * Δx = 5cos(π/4) * π/8
Rectangle 3: Area = f(3π/8) * Δx = 5cos(3π/8) * π/8
Rectangle 4: Area = f(π/2) * Δx = 5cos(π/2) * π/8
Now, let's calculate the values:
Rectangle 1: Area = 5cos(π/8) * π/8 ≈ 0.2887
Rectangle 2: Area = 5cos(π/4) * π/8 ≈ 0.3142
Rectangle 3: Area = 5cos(3π/8) * π/8 ≈ 0.2887
Rectangle 4: Area = 5cos(π/2) * π/8 ≈ 0
Finally, to estimate the total area, we sum up the areas of all four rectangles:
Total Area ≈ 0.2887 + 0.3142 + 0.2887 + 0 ≈ 0.8916
Therefore, the estimated area under the graph of f(x) = 5 cos(x) from x = 0 to x = π/2 using four approximating rectangles and right endpoints is approximately 0.8916.
To know more about endpoints refer here:
https://brainly.com/question/31398203#
#SPJ11
a test contains 100 true/false questions. how many different ways can a student answer the questions on the test, if answers may be left blank?
Answers
There are 3^100 possible combinations of answers for a student taking a test with 100 true/false questions where answers may be left blank.
To answer this question, we need to consider the fact that each question on the test has two possible answers - true or false. Therefore, for each of the 100 questions, there are 2 possible ways that a student can answer the question.
If a student answers every single question on the test, there are a total of 2^100 possible combinations of answers. This is because for each question, there are 2 possibilities, and there are 100 questions in total.
However, the question states that answers may be left blank. This means that for each question, there are now 3 possibilities - true, false, or blank. Therefore, the total number of possible combinations of answers is now 3^100.
To put this into perspective, 3^100 is an extremely large number - it is approximately 5.15 x 10^47. This means that even if every single person on Earth were to take this test and answer every question in a unique way, it would still be highly unlikely that any two people would have the same combination of answers.
In conclusion, there are 3^100 possible combinations of answers for a student taking a test with 100 true/false questions where answers may be left blank.
for more questions on combinations
https://brainly.com/question/12725706
#SPJ11
I need help with this one

Answers
Answer:
S = 180C²
18,000 lbs
Step-by-step explanation:
Assuming the table attached with C in inches and S in pounds, we see that the first differences in S are ...
180, 540, 900
These are not constant, so the relation is not linear.
Second differences are ...
360, 360
These are constant, so the relation is quadratic. With a short trial, we find that a suitable equation is ...
S = 180·C²
__
The safe load for C=10 is then ...
S = 180·10² = 18,000 . . . pounds
better?
Find the value of x?!!!

Answers
Answer:
x = 118
Step-by-step explanation:
33 + 29 = 62
180 - 62 = 118
Answer:
x = 118°Step-by-step explanation:
We know that:
Triangle = 180° = 29 + 33 + xSolution:
180° = 29 + 33 + x=> 180° = 62 + x=> 180 - 62 = x=> 118° = xHence, the measure of ∠x is 118°.
Rooms in a hotel are numbered from 1 to 19.
Rooms are allocated at random as guests arrive.
(a) What is the probability that the first guest to arrive is given a room which is a prime number?
(1 is not a prime number.)
(b) The first guest to arrive is given a room which is a prime number.
What is the probability that the second guest to arrive is given a room which is a prime number?
Answers
Answer:
a) 8/19 chance
b) 7/19 chance (I'm not 100% sure)
Step-by-step explanation:
A) since there are 8 prime numbers from 1-19 we can say that the probability is 8 over 19 or 8/19
(3,4) is an example of a/an
Answers
Answer:
Ordered pair
Definition: An ordered pair is a pair of numbers in parentheses that declare the location of a point on a graph.
if a confusion matrix shows 46 tp, 6 fn, 500 tn, and 4 fp, what is the precision ratio? (round your answer to 2 decimal places.)
Answers
Answer:
Step-by-step explanation:
Precision is defined as the ratio of true positives (TP) to the total predicted positives (TP + FP). In this case, the confusion matrix shows 46 TP and 4 FP, so the precision can be calculated as:
precision = TP / (TP + FP) = 46 / (46 + 4) = 0.92
Rounding to 2 decimal places, the precision ratio is 0.92.