need answers to these two questions with work, must find the measurement indicated using the law of sines. round answer to the nearest tenth.

Answers
Applying the law of sines, the measurement indicated are:
7. AB = 24.1 cm
8. BC = 28.0 in.
What is the Law of Sines?Law of sines is: sin A/a = sin B/b = sin C/c.
7. AB (c) = ?
BC (a) = 22 cm
A = 180 - 138 - 22 = 20°
C = 22°
Apply the law of sines:
sin 22/AB = sin 20/22
AB = (sin 22 × 22)/sin 20
AB = 24.1 cm
8. BC (a) = ?
A = 58°
AC (b) = 33 in.
B = 180 - 58 - 33 = 89°
Apply the law of sines:
sin 58/BC = sin 89/33
BC = (sin 58 × 33)/sin 89
BC = 28.0 in.
Learn more about the law of sines on:
https://brainly.com/question/2807639
#SPJ1
Related Questions
What is the missing length?

Answers
The missing length is 6 yards
How to determine the missing lengthFrom the question, we have the following parameters that can be used in our computation:
The figure
Also, we have
Area = 45 square yards
Base = 7.5 yards
Using the above as a guide, we have the following:
Height = Area/Base
So, we have
Height = 45/7.5
Evaluate
Height = 6
Hence, the height is 6 yards
Read more about area at
https://brainly.com/question/25292087
#SPJ1
5 Rosi pays $7.56 for 6 bags of beads. How much does one bag of beads cost?
Answers
Answer:
\(7.56 \div 6 = 1.26\)
one bag of beads cost $1.26
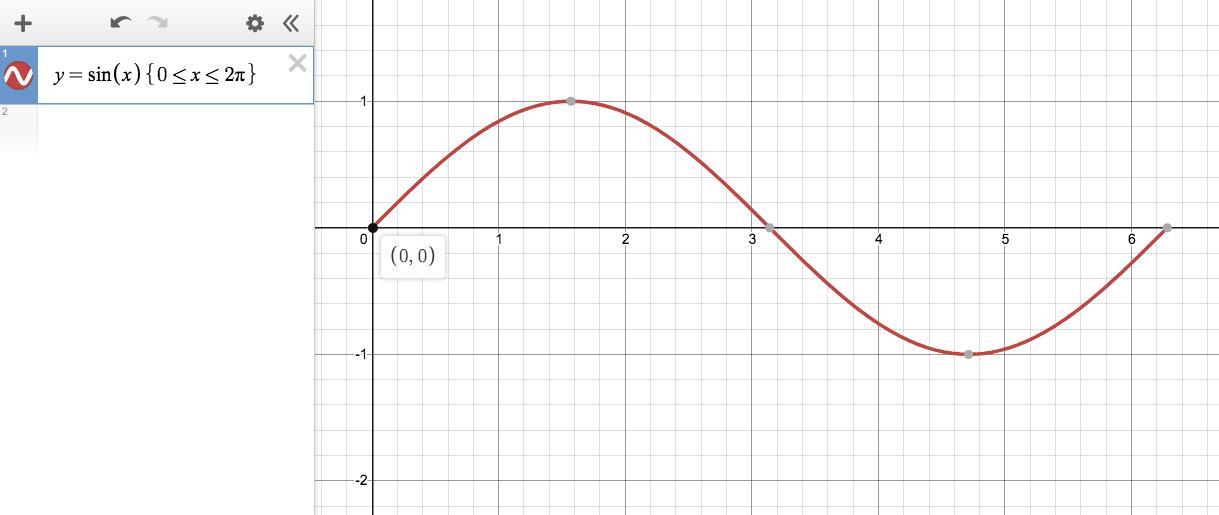
HELP!!!Where does the graph of y = sin x from x = 0 to x = 2π start? A. at its minimum B. at an x–intercept C. at its maximum D. between an x–intercept and its maximum
Answers
Answer:
B. at an x–intercept
Step-by-step explanation:
You can find a graph of the sine function in numerous places.
y = sin(0) = 0
y = 0 for x = 0
so, on the given interval, it starts at an x-intercept.
an unknown number multiplied by 8 gives 32
Answers
Answer:
4
Step-by-step explanation:
Cause 4×8 is 32.Am I right.Hpoe it helps.
Answer:
n=4
Step-by-step explanation:
n * 8 = 32
Do it backwards now
32 / 8 to make 4.\To check,
4*8 = 32
a rectangular window dimensions areX by X +2 and window has a 3 inch frame all the way around the total area of the window and frame is 195 in
Answers
Answer:
Length of window = 9 inch
Width of window = 7 inch
Step-by-step explanation:
Given;
Area of window and frame = 195 inch²
Frame width = 3 inch
Length of window = x + 2
Width of window = x
Find:
Value of x
Computation:
Length of window and frame = x + 2 + 3 + 3
Length of window and frame = x + 8
Width of window and frame = x + 3 + 3
Width of window and frame = x + 6
Area of window and frame = (l)(b)
(x + 8)(x + 6) = 195
x² + 6x + 8x + (8)(6) = 195
x² + 14x + 48 = 195
x² + 14x - 147 = 0
x² + 21x - 7x - 147 = 0
x(x + 21) - 7(x + 21) = 0
So,
x + 21 = 0 and x - 7 = 0
So,
X = 7
Value of x = 7
Length of window = 9 inch
Width of window = 7 inch
Factor the expression 4r^(2)-r-5=0
Answers
Answer:
(4r-5)(r+1)=0
Step-by-step explanation:
If you multiply this out, you will get the original equation.
Find two integers whose sum is 3 and product is -108.
Answers
Answer:
12 and -9
Step-by-step explanation:
12 x -9 = -108
12 + -9 = 3
Let f(x) = 5x – 4 and g(x) = x² – 1. Find each value.
(gºf)(-1)
(fºg)(2)
(gºf)(0)
f(g(16)
f(g(0))
g(f(4/5))

Answers
Answer:
35.) 80
36.) 11
37.) 15
38.) 21
39.) -9
40.) -1
Step-by-step explanation:
In simplified terms the question is asking
35.) g(f(-1)) =
g(-9) = 80
36.) f(g(2)) =
f(3) = 11
37.) g(f(0)) =
g(-4) = 15
38.) f(g(root6)) =
f(5) = 21
39.) f(g(0)) =
f(-1) = -9
40.) g(f(4/5)) =
g(0) = -1
Help me with this please 4 and 6 only though.

Answers
y=9x is proportional
y= 19x - 5 is non proportional.
Hope this helped
Answer:
well 4 is proportional.
6 is non proportinal
Step-by-step explanation:
A drug test for athletes has a 4 percent false positive rate and a 12 percent false negative rate. Of the athletes tested, 5 percent have actually been using the prohibited drug. If an athlete tests positive, what is the probability that the athlete has actually been using the prohibited drug
Answers
The probability that the athlete has actually been using the prohibited drug given that they tested positive is approximately 0.5789 or 57.89%.
How to find the probability and the application of Bayes' theorem to calculate the probability?To solve this problem, we can use Bayes' theorem, which relates the conditional probabilities of two events.
Let A be the event that the athlete has been using the prohibited drug, and let B be the event that the athlete tests positive.
We want to find the probability of A given B, which we can write as P(A | B).
Using Bayes' theorem, we have:
P(A | B) = P(B | A) * \(\frac{P(A) }{P(B)}\)
where P(B | A) is the probability of testing positive given that the athlete has been using the prohibited drug, P(A) is the prior probability of the athlete using the prohibited drug, and P(B) is the overall probability of testing positive, which can be calculated using the law of total probability:
P(B) = P(B | A) * P(A) + P(B | not A) * P(not A)
where P(B | not A) is the probability of testing positive given that the athlete has not been using the prohibited drug, and P(not A) is the complement of P(A), i.e., the probability that the athlete has not been using the prohibited drug.
Using the given information, we can plug in the values:
P(B | A) = 1 - 0.12 = 0.88 (probability of testing positive given the athlete is using the drug)
P(A) = 0.05 (prior probability of the athlete using the drug)
P(B | not A) = 0.04 (probability of testing positive given the athlete is not using the drug)
P(not A) = 1 - P(A) = 0.95 (probability that the athlete is not using the drug)
Then, we can calculate P(B) as:
P(B) = P(B | A) * P(A) + P(B | not A) * P(not A)
= 0.88 * 0.05 + 0.04 * 0.95
= 0.076
Finally, we can calculate P(A | B) as:
P(A | B) = P(B | A) * \(\frac{P(A) }{ P(B)}\)
= 0.88 * \(\frac{0.05 }{ 0.076}\)
= 0.5789
Therefore, the probability that the athlete has actually been using the prohibited drug given that they tested positive is approximately 0.5789 or 57.89%.
Learn more about Bayes' theorem and probability.
brainly.com/question/17010130
#SPJ11
A 150 pound crate is used to hold the boxes on the forklift. What is the maximum number of boxes that the forklift can carry in the crate.
Answers
Answer: To determine the maximum number of boxes that the forklift can carry in the crate, we need to know the weight of each box and the weight capacity of the forklift. Let's assume that each box weighs 20 pounds and the weight capacity of the forklift is 2000 pounds.
To find the maximum number of boxes, we need to subtract the weight of the crate from the weight capacity of the forklift and then divide by the weight of each box:
Maximum number of boxes = (Weight capacity of forklift - Weight of crate) / Weight of each box
Maximum number of boxes = (2000 - 150) / 20
Maximum number of boxes = 1850 / 20
Maximum number of boxes = 92.5
Since we cannot have a fraction of a box, we need to round down to the nearest whole number. Therefore, the forklift can carry a maximum of 92 boxes in the crate.
Step-by-step explanation:
What is an equation of the line that is perpendicular to y=−34x+6 and passes through the point (3, 9)?
Answers
Answer:
y = 4/3x + 5
Step-by-step explanation:
if the slope is -3/4 then the perpendicular slope is 4/3
y = mx + b
9 = 4/3(3) + b
9 = 4 + b
5 = b
y = 4/3x + 5
It takes 464646 minutes for 101010 people to paint 333 walls. How many minutes does it take 444 people to paint 666 wallsIt takes 464646 minutes for 101010 people to paint 333 walls. How many minutes does it take 444 people to paint 666 walls?
Answers
Answer:Answer: It takes 4 person 230 mins to paint 6 walls
Step-by-step explanation:
10 people : 46 mins : 3 walls
--------------------------------------------------------------------------------------
Find the number of people needed to paint 6 walls
--------------------------------------------------------------------------------------
(10 x 2) people : 46 mins : (3 x 2) walls
20 people : 46 mins : 6 walls
⇒ It takes 20 people 46 mins to paint 6 walls.
--------------------------------------------------------------------------------------
Find amount of time to paint all 6 wall by 1 person
--------------------------------------------------------------------------------------
(20 ÷ 20) people : (46 x 20) mins : 6 walls
1 person : 920 mins : 6 walls
⇒It takes 1 person 920 mins to paint 6 walls
--------------------------------------------------------------------------------------
Find the amount of time to paint 6 walls by 4 people
--------------------------------------------------------------------------------------
(1x 4) person : (920 ÷ 4) mins : 6 walls
4 person : 230 mins : 6 walls
⇒ It takes 4 person 230 mins to paint 6 walls
--------------------------------------------------------------------------------------
Answer: It takes 4 person 230 mins to paint 6 walls
--------------------------------------------------------------------------------------
HOPE I HELPED
PLS MARK BRAINLIEST
DESPERATELY TRYING TO LEVEL UP
✌ -ZYLYNN JADE ARDENNE
Write a linear equation in slope intercept form using the given point and slope.
(6,-6); slope = 2/3
Answers
Answer:
2/3x-10
Step-by-step explanation:
y=mx+b
y=2/3x+b
Now, what about b, the y-intercept?
To find b, think about what your (x,y) point means:
(6,-6). When x of the line is 6, y of the line must be -6.
Because you said the line passes through this point, right?
Now, look at our line's equation so far: . b is what we want, the 2/3 is already set and x and y are just two "free variables" sitting there. We can plug anything we want in for x and y here, but we want the equation for the line that specfically passes through the the point (6,-6).
So, why not plug in for x the number 6 and for y the number -6? This will allow us to solve for b for the particular line that passes through the point you gave!.
(6,-6). y=mx+b or -6=2/3 × 6+b, or solving for b: b=-6-(2/3)(6). b=-10.
so, y=2/3x-10
The measure of one interior angle of a parallelogram is 0. 25 times the measure of another angle.
The measure of the smaller interior angle is
and the measure of the larger interior angle is
Answers
The measure of the smaller interior angle is x = 36 degrees, and the measure of the larger interior angle is 4x = 144 degrees.
Let's assume that the measure of one interior angle of the parallelogram is x. Then, according to the problem statement, the measure of another angle would be 4x (since it is 0.25 times the measure of the first angle).
Now, we know that opposite angles in a parallelogram are congruent (they have the same measure), so the other two interior angles of the parallelogram would also have measures x and 4x.
The sum of the measures of the interior angles of a parallelogram is always equal to 360 degrees, so we can write:
x + 4x + x + 4x = 360
Simplifying this equation, we get:
10x = 360
Dividing both sides by 10, we obtain:
x = 36
Therefore, the measure of the smaller interior angle is x = 36 degrees, and the measure of the larger interior angle is 4x = 144 degrees.
Learn more about angle from
https://brainly.com/question/25716982
#SPJ11
Which equation can be used to find the volume of this solid?
4 cm
5 cm
TTT
3 cm)
O V-3x5x4
O V-3+5+4
O V=5x4
OV=3x5
Answers
Answer:
V = 3×5×4 cm^3
Step-by-step explanation:
The solid used in the question is assumed to be a rectangular box.
The volume of a rectangular box is;
Volume = length × Breadth × height
Given the dimensions of the box as;
4cm by 5cm by 3cm.
The volume V of the box can then be written as the product of the dimensions;
V = 3cm × 5cm × 4cm
V = 3×5×4 cm^3

Answer:a
Step-by-step explanation:
Which equation has exactly one solution in common with the equation y=6x-2?
A. 18x - 3y = 6
B. 1/2y = 3x - 2
C. 2y = 4x - 12
D. 18x - 12 = 3y
Answers
Answer:
A. 18x - 3y = 6
Step-by-step explanation:
By taking 3 common from the terms 18x and 3y we get,
3 (6x-3y) = 6
or, 6x - y = 6/3
or, 6x - y = 2
And,
The equation becomes,
y = 6x - 2
The equation has exactly one solution in common with the equation y=6x-2 will be 18x - 3y = 6. The correct option is A.
What is a system of equations?A finite set of equations for which common solutions are sought is referred to in mathematics as a set of simultaneous equations, often known as a system of equations or an equation system.
An equation is defined as the relation between two variables, if we plot the graph of the linear equation we will get a straight line.
By taking 3 commons from the terms 18x and 3y we get,
3 (6x-3y) = 6
6x - y = 6/3
6x - y = 2
The equation becomes,
y = 6x - 2
Therefore, the equation has exactly one solution in common with the equation y=6x-2 will be 18x - 3y = 6. The correct option is A.
To know more about equations follow
https://brainly.com/question/2972832
#SPJ2
I-Ready you now and no Du..a.s...s. answers or links

Answers
Answer:
13/18
Step-by-step explanation:
The two negatives cancel out.
1/6 * 4 1/3 is 13/18, first turn 4 1/3 to 13/3.
Hope this helps
trucks in a delivery fleet travel a mean of 90 miles per day with a standard deviation of 18 miles per day. the mileage per day is distributed normally. find the probability that a truck drives between 122 and 127 miles in a day. round your answer to four decimal places.
Answers
The probability that a truck drives between 122 and 127 miles in a day is 0.0165, rounded to four decimal places.
To find the probability that a truck drives between 122 and 127 miles in a day, we'll use the z-score formula and standard normal distribution table. Follow these steps:
Step 1: Calculate the z-scores for 122 and 127 miles.
z = (X - μ) / σ
For 122 miles:
z1 = (122 - 90) / 18
z1 = 32 / 18
z1 ≈ 1.78
For 127 miles:
z2 = (127 - 90) / 18
z2 = 37 / 18
z2 ≈ 2.06
Step 2: Use the standard normal distribution table to find the probabilities for z1 and z2.
P(z1) ≈ 0.9625
P(z2) ≈ 0.9803
Step 3: Calculate the probability of a truck driving between 122 and 127 miles.
P(122 ≤ X ≤ 127) = P(z2) - P(z1)
P(122 ≤ X ≤ 127) = 0.9803 - 0.9625
P(122 ≤ X ≤ 127) ≈ 0.0178
So, the probability that a truck drives between 122 and 127 miles in a day is approximately 0.0178 or 1.78%.
to learn more about probability click here:
https://brainly.com/question/13604758
#SPJ11
what is the probability that washing dishes tonight will take me between 15 and 16 minutes? give your answer accurate to two decimal places.
Answers
The probability of washing dishes by me tonight will take between 15 and 16 minutes is 14.29%.
The term "uniform distribution" refers to a type of probability distribution in which the likelihood of each potential result is equal.
Let's consider, the lower limit for this distribution as a and the upper limit for this distribution as b.
The following formula gives the likelihood that we will discover a value for X between c and d,
\(P(c\leq X\leq d)=\frac{d-c}{b-a}\)
Given the time it takes me to wash the dishes is 11 minutes and 18 minutes. From this, a = 11 and b = 18.
Then,
\(P(15\leq X\leq 16)=\frac{16-15}{18-11}=0.1429=14.29\%\)
The answer is 14.29%.
The complete question is -
The time it takes me to wash the dishes is uniformly distributed between 11 minutes and 18 minutes. What is the probability that washing dishes tonight will take me between 15 and 16 minutes?
To know more about uniform distribution:
https://brainly.com/question/17091893
#SPJ4
for the problem of approximating the probability of a 6 in rolling a die, a. identify an appropriate family of distributions;
Answers
The appropriate family of distributions to approximate the probability of rolling a 6 on a fair die is the discrete uniform distribution, which assumes equal probabilities for each outcome. In this case, the probability of rolling a 6 would be approximately 1/6 based on the assumption of fairness.
For the problem of approximating the probability of rolling a 6 on a fair die, an appropriate family of distributions to consider is the discrete uniform distribution.
The discrete uniform distribution is commonly used to model situations where each outcome has an equal probability of occurring. In the case of rolling a fair die, the die has six equally likely outcomes (numbers 1 to 6).
Each outcome has a probability of 1/6 of occurring, making the discrete uniform distribution a suitable choice.
By assuming a discrete uniform distribution, we can assign equal probabilities to each outcome (1/6 for rolling a 6) and approximate the probability of rolling a 6 based on the assumption of fairness.
To know more about discrete uniform distribution refer here:
https://brainly.com/question/31603031#
#SPJ11
How many possible 10-digit phone numbers are possible (7 digits plus the
area code)? Type your answer into the box.
Answers
Using the Fundamental Counting Theorem, it is found that 3,250,000,000 phone numbers are possible.
What is the Fundamental Counting Theorem?It is a theorem that states that if there are n things, each with \(n_1, n_2, \cdots, n_n\) ways to be done, each thing independent of the other, the number of ways they can be done is:
\(N = n_1 \times n_2 \times \cdots \times n_n\)
In this problem:
For the first 3 digits, which correspond to the area code, there are 325 options in total, that is, \(n_1n_2n_3 = 325\).For the final 7 digits, each of them has 10 options.Hence:
\(T = 325 \times 10^7 = 3,250,000,000\)
3,250,000,000 phone numbers are possible.
More can be learned about the Fundamental Counting Theorem at https://brainly.com/question/24314866
The triangles are similar. Find the value of x =

Answers
sam estimates that if he leaves his car parked outside his office all day on a weekday, the chance that he will get a parking ticket is 13%. if sam leaves his car parked outside his office all day next tuesday, what is the chance that he will not get a parking ticket?
Answers
The chance that Sam will not get a parking ticket is 87%.
No Parking Ticket ChanceTo find the chance of not getting a parking ticket, subtract the chance of getting a ticket from 100%.
Step 1: Take the chance of getting a ticket, which is 13%
Step 2: Subtract it from 100%
100% - 13% = 87%
So, the chance that he will not get a parking ticket is 87% if Sam want to leaves his car parked outside his office all day next tuesday.
This question is about the probability of Sam getting a parking ticket if he leaves his car parked outside his office on a weekday. Sam estimates that the chance of getting a ticket is 13%. To find the chance of not getting a ticket, the probability of getting a ticket (13%) was subtracted from 100%. The result, 87%, represents the chance that Sam will not get a parking ticket.
Learn more about No Parking Ticket Chance here:
https://brainly.com/question/25705613
#SPJ4
\ Evaluate the integral: 6x² 25 - x² dx + C\
Answers
The integral of 6x² - x² with respect to x is equal to 5x². Adding the constant of integration, the final result is 5x² + C.
To evaluate the integral, we first simplify the expression inside the integral: 6x² - x² = 5x². Now we can integrate 5x² with respect to x.
The integral of x^n with respect to x is given by the power rule of integration: (1/(n+1)) * x^(n+1). Applying this rule, we have:
∫ 5x² dx = (5/3) * x^(2+1) + C
= 5/3 * x³ + C
Adding the constant of integration (denoted by C), we obtain the final result:
5/3 * x³ + C
This is the indefinite integral of 6x² - x² with respect to x. The constant C represents the family of all possible solutions since the derivative of a constant is zero. Therefore, when evaluating integrals, we always include the constant of integration to account for all possible solutions.
Learn more about equal here: brainly.com/question/9070018
#SPJ11
Under certain conditions in certain devices, the behavior of the current in a conductor can be represented as a function of time by the equation I(t)=I
0e −t/τ
. Here I 0 is the current at t=0 and τ is known as the time constant. In this case, τ=7.00 ms and the initial current I 0=4.10 mA. Determine the amount of charge passing through a fixed point in the conductor in the time interval t 1=0 to t 2=τ
Answers
Therefore, the amount of charge passing through the fixed point in the conductor in the time interval t1 = 0 to t2 = τ is approximately -18.86 μC.
To determine the amount of charge passing through a fixed point in the conductor in the time interval t1 = 0 to t2 = τ, we need to calculate the integral of the current function I(t) over that time interval.
The current function is given by:
I(t) = I0 * e\(^(-t/τ)\)
Integrating this function over the time interval [t1, t2], we have:
Q = ∫[t1, t2] I(t) dt
Substituting the expression for I(t), we get:
Q = ∫\([t1, t2] I0 * e^(-t/τ) dt\)
Since I0 and τ are constant values, we can take them out of the integral:
Q = I0 * ∫[\(t1, t2] e^(-t/τ) dt\)
To evaluate this integral, we can use the following property of the exponential function:
∫\(e^(ax) dx = (1/a) * e^(ax) + C\)
Applying this property to our integral, we have:
Q = I0 * (-τ) * \(e^(-t/τ) |_t1 ^t2\)
Substituting the values t1 = 0 and t2 = τ, we get:
Q\(= I0 * (-τ) * (e^(-t2/τ) - e^(-t1/τ))\)
Substituting the given values I0 = 4.10 mA and τ = 7.00 ms, we have:
\(Q = 4.10 mA * (-7.00 ms) * (e^(-7.00 ms/7.00 ms) - e^(0/7.00 ms))\)
Simplifying the expression:
\(Q = 4.10 mA * (-7.00 ms) * (e^(-1) - 1)\)
Calculating the value:
Q ≈ -18.86 μC
To know more about interval,
https://brainly.com/question/17070177
#SPJ11
Which of the following represents an exponential relationship?
A
(1,5),(2,10),(3,15)(1,5),(2,10),(3,15)
B
(2,9),(3,27),(4,81)(2,9),(3,27),(4,81)
C
(2,4),(3,9),(4,16)(2,4),(3,9),(4,16)
D
(5,15),(6,18),(7,21)

Answers
Answer:
(C)
Step-by-step explanation:
2 power 2 = 4
3 power 2 = 9
4 power 2 = 16
66666666 help me plz plz plz

Answers
Answer:
XY would also be 7 centimeters which is answer D.
Step-by-step explanation:
This is a parallelogram, meaning that the adjacent sides are congruent. As well, the triangles making up the figure are congruent, so it makes sense that XY would also equal 7 centimeters.
Recently, many companies have been using an integrity test as part of their personnel selection devices. Suppose that scores of a standardized integrity test are normally distributed, with a mean of 600 and a standard deviation of 112.
If a random sample if n = 19 is drawn from this population distribution, within what limits would the central 95% of all possible sample means fall (in raw score units)? Report here the lower limit
Answers
The lower limit for the central 95% of all possible sample means, drawn from a population distribution with a mean of 600 and a standard deviation of 112, is approximately 551.66.
To determine the lower limit of the central 95% of sample means, we use the formula for the confidence interval:
Lower limit = sample mean - margin of error
The margin of error is calculated by multiplying the critical value (obtained from the Z-table for a 95% confidence level) by the standard deviation of the population divided by the square root of the sample size:
Margin of error = Z * (σ/√n)
In this case, the mean is 600, the standard deviation (σ) is 112, and the sample size (n) is 19. From the Z-table, the critical value for a 95% confidence level is approximately 1.96.
Plugging in the values, we get:
Margin of error = 1.96 * (112/√19) ≈ 23.33
Therefore, the lower limit is:
Lower limit = 600 - 23.33 ≈ 551.66
learn more about Confidence interval here:
https://brainly.com/question/30112103
#SPJ4
Find the reference angle for -369°
a.
9°
c.
99°
b.
81°
d.
171°
Please select the best answer from the choices provided
A
B
C
D
Answers
Answer:
A. 9
Step-by-step explanation:
