Newborn babies: A study conducted by the Center for Population Economics at the University of Chicago studied the birth weights of 778 babies born in New York. The mean weight was 3172 grams with a standard deviation of 888 grams. Assume that birth weight data are approximately bell-shaped. Estimate the number of newborns who weighed between 2284 grams and 4060 grams. Round to the nearest whole number. The number of newborns who weighed between 2284 grams and 4060 grams is
Answers
Rounding to the nearest whole number, the estimated number of newborns who weighed between 2284 grams and 4060 grams is 294.Approximately 95% falls within 2 standard deviations, and approximately 99.7% falls within 3 standard deviations.
To estimate the number of newborns who weighed between 2284 grams and 4060 grams, we can use the properties of the normal distribution and the empirical rule.
Given information:
Mean weight (μ) = 3172 grams
Standard deviation (σ) = 888 grams
We can assume that the birth weight data are approximately bell-shaped, which allows us to use the empirical rule.
According to the empirical rule, approximately 68% of the data falls within 1 standard deviation of the mean in a normal distribution.
Approximately 95% falls within 2 standard deviations, and approximately 99.7% falls within 3 standard deviations.
To estimate the number of newborns within the given weight range, we can calculate the z-scores for the lower and upper limits of the range using the mean (μ) and standard deviation (σ):
For 2284 grams:
z1 = (2284 - 3172) / 888
For 4060 grams:
z2 = (4060 - 3172) / 888
Using the z-table, we can find the area under the curve between these two z-scores. Since the data is approximately bell-shaped, we can estimate the percentage of newborns within the weight range.
Let's calculate the z-scores:
z1 = (2284 - 3172) / 888 = -0.0991
z2 = (4060 - 3172) / 888 = 0.9982
Using the z-table, we can find the areas associated with these z-scores. For z1, the area is approximately 0.4602, and for z2, the area is approximately 0.8382.
To estimate the percentage of newborns within the weight range, we subtract the area corresponding to the lower z-score from the area corresponding to the upper z-score:
Percentage = 0.8382 - 0.4602 = 0.3780
This means that approximately 37.8% of the newborns weighed between 2284 grams and 4060 grams.
To estimate the number of newborns within this weight range, we can multiply the percentage by the total number of newborns:
Number of newborns = 0.3780 * 778
Rounding to the nearest whole number, the estimated number of newborns who weighed between 2284 grams and 4060 grams is 294.
To learn more about standard deviation click here:
brainly.com/question/32635788
#SPJ11
Related Questions
If f(x)=x+12 and g(x)=x−5, find f(x)⋅g(x).
x2+17x−60
x2+7x−60
x2+7x−7
x2−7x+60
Answers
Answer:
x² + 7x - 60
Step-by-step explanation:
Multiply (x + 12) and (x - 5)
Use FOIL method:
FIRST terms (x)(x) = x²
OUTER terms (x)(-5) = -5x
INNER terms (12)(x) = 12x
LAST terms (12)(-5) = -60
Then add the outer and inner terms: (-5x) + (12x) = 7x
Bring down or copy the product of the first terms, the sum of outer and inner terms and the product of last terms.
x² + 7x - 60
Which graph represents y=1/2x+2
please help!!

Answers
Answer:
B
Step-by-step explanation:
Let’s first look at the y-intercept. Since the equation is in y = mx + b form, we know that m is the slope and b is the y-intercept, so the slope is 1/2 and the y-intercept is 2, or (0,2). We can narrow down our search by noticing that C and D don’t intersect (0,2). That leaves A and B.
The slope is calculated by (y_1 - y_2)/(x_1 - x_2) given points (x_1, y_1) and (x_2, y_2). The slope for A is (4-0)/(2-(-2)) = 4/4 = 1 and the slope for B is (2-0)/(0-(-4)) = 2/4 = 1/2. The slope of B matches the slope that we are looking for. So, the answer is B.
Plz help me!
If you help you get a brainliest!

Answers
Answer:
C) x=11
Step-by-step explanation:

1 What is the value of x? 45° m (2x - 5)° n

Answers
45, and
2x - 5
make up a straight angle pair.
So, they add up to 180 degrees.
Thus, we can write:
45 + 2x - 5 = 180
Now, we simply solve for x. Shown below:
\(\begin{gathered} 45+2x-5=180 \\ 2x+40=180 \\ 2x=180-40 \\ 2x=140 \\ x=\frac{140}{2} \\ x=70 \end{gathered}\)There is a population of 10 bacteria in a colony. If the number of bacteria doubles every 70
minutes, what will the population be 140 minutes from now?
Answers
Answer:
40
Step-by-step explanation:
Show your work. 21. The side of a square rug is 6 feet. What area of the floor will the rug cover?

Answers
Answer:
36ft²
Step-by-step explanation:
A=a²=6²=36ft²
sorry if im wrong
What is the value of e?

Answers
e equals 129
The answer is 129
who wants extra points and brainlist............

Answers
determine the graph of the polar equation 9/1-3sin theta
Answers
Answer: can you show us the graph?
Step-by-step explanation:
The sum of two numbers is 54 and the difference is 14. What are the numbers?
Answers
Answer:
20,34
Step-by-step explanation:
Let the two numbers bw a,b
Their sum is 54
a+b=54⋯(1)
Their difference is 14
a−b=14⋯(2)
(1)+(2)
2a=68
a=34⟹34+b=54
b=54−34=20
Answer
34 and 20.
Step-by-step explanation:
34+20 is equal to 54.
34-20 is equal to 14.
I hope this helps! :)
Im not sure if my answer is correct, i need clarification.
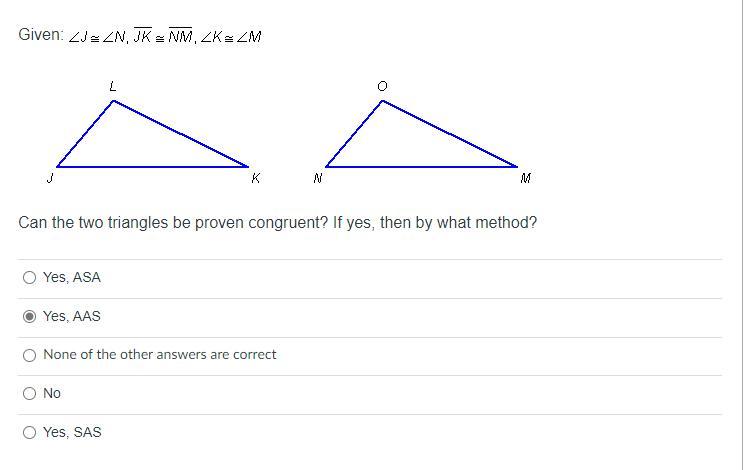
Answers
Answer:
Yes, ASA.
Step-by-step explanation:
The ASA, or Angle-Side-Angle Postulate, states that "If two angles and the included side of one triangle are congruent to two angles and the included side of a second triangle, then the triangles are congruent."
In this case, it is given that two pairs of angles are congruent:
1) ∠J ≅ ∠N (Given)
2) ∠K ≅ ∠M (Given)
One pair of side is also congruent:
1) JK ≅ NM
∴ Through ASA Postulate, ΔJLK ≅ ΔNOM.
Learn more about the ASA Postulate, here:
https://brainly.com/question/11872456
what is the slope of (1,3) and (2,-4)
Answers
Answer:
1/-7x
Step-by-step explanation:
All you have to do is find the distance between both points.
I have few questions i need help on 1. A student scored 65 marks out of a total marks off 100 in a test, what is his percentage score .
2. if i add 2 to a number and then triple the sum , the result is two times what i get when i add 4 to the number what is the number.
3a. simplify
\(1 - (2 \frac{1}{3} \times 1 \frac{1}{4} ) + \frac{3}{5} \)
b Evaluate
\( \frac{1}{3} \div ( \frac{5}{7} ( \frac{9}{10} - 1 + \frac{3}{4} ) \)
in this questions i used BODMAS still its saying I'm wrong pls help .
4. Find the sum of three consecutive even numbers if there mean is 24.
5. The sum of four consecutive odd numbers is 72,find the numbers.
6. if when 4 is added to half a number ,the result is 8 , what is the number?
7. change the expression
\( \frac{14}{13 - 3} \)
into words .
8. A customer paid 500 naira after a discount of 10% had been allowed. what was the marked price of the article ?
9. a salesman is paid 5% on his first 50 000 naira sales,10% of the next 30 000 naira and 20% of all sales in excess of 80 000 naira . calculate his commision on sales totalling 100 000 naira .
10. Find the multiplicative inverse of the following of each numbers
a 3
b -2
c 5x
11. find the additive inverse of the following
a. + 0.27
b. + 13
c. -6
That's all .
Answers
Answer:
1. His percentage score is 65%
WILL GIVE BRAINLIEST Questions 1-2 Linear One sentence explanation

Answers
Answer:
YesNoStep-by-step explanation:
The first table represents a linear function because:
For each increase in x by 5 points we have increase in y by 10 pointsThe second table is not linear as:
For the first two steps of x by 4 points we have increase of y by 8 points but for the rest of the steps it is 6 for each 4, therefore not linear1.Find the period of the following functions. a) f(t) = (7 cos t)² b) f(t) = cos (2φt²/m)
Answers
Period of the functions: The period of the function f(t) = (7 cos t)² is given by 2π/b where b is the period of cos t.The period of the function f(t) = cos (2φt²/m) is given by T = √(4πm/φ). The period of the function f(t) = (7 cos t)² is given by 2π/b where b is the period of cos t.
We know that cos (t) is periodic and has a period of 2π.∴ b = 2π∴ The period of the function f(t) =
(7 cos t)² = 2π/b = 2π/2π = 1.
The period of the function f(t) = cos (2φt²/m) is given by T = √(4πm/φ) Hence, the period of the function f(t) =
cos (2φt²/m) is √(4πm/φ).
The function f(t) = (7 cos t)² is a trigonometric function and it is periodic. The period of the function is given by 2π/b where b is the period of cos t. As cos (t) is periodic and has a period of 2π, the period of the function f(t) = (7 cos t)² is 2π/2π = 1. Hence, the period of the function f(t) = (7 cos t)² is 1.The function f(t) = cos (2φt²/m) is also a trigonometric function and is periodic. The period of this function is given by T = √(4πm/φ). Therefore, the period of the function f(t) = cos (2φt²/m) is √(4πm/φ).
The period of the function f(t) = (7 cos t)² is 1, and the period of the function f(t) = cos (2φt²/m) is √(4πm/φ).
To learn more about trigonometric function visit:
brainly.com/question/25618616
#SPJ11
dilation of 6,10 with a scale factor of 1/2
Answers
The new coordinate of the points after the dilation will be (3, 5).
What is dilation?Dilation is the process of increasing the size of an item without affecting its form. Depending on the scale factor, the object's size can be raised or lowered. There is no effect of dilation on the angle.
The point is (6, 10). And the scale factor is 1/2. Then the new coordinate of the points after the dilation is given as,
⇒ (1/2)(6, 10)
⇒ (1/2 x 6, 1/2 x 10)
⇒ (3, 5)
The new coordinate of the points after the dilation will be (3, 5).
More about the dilation link is given below.
https://brainly.com/question/2856466
#SPJ1
find the formuma f/g(x) and simplify your answer
Answers
To find the formula f/g(x), you need to know the specific functions f(x) and g(x). Once you have those functions, you can create the formula by dividing f(x) by g(x). For example, if f(x) = x^2 + 1 and g(x) = x - 1, the formula f/g(x) would be:
f/g(x) = (x^2 + 1) / (x - 1)
Know more about functions here:
https://brainly.com/question/21145944
#SPJ11
Solve for y:
3x - 5y = 20
Answers
Steps to solve:
3x - 5y = 20
~Subtract 3x to both sides
-5y = 20 - 3x
~Divide everything by -5
y = -4 + 3/5x
Best of Luck!
The equivalent value of the expression is y = ( 3x - 20 ) / 5
Given data ,
Let the equation be represented as A
Now , the value of A is
3x - 5y = 20
On simplifying the equation , we get
3x - 5y = 20
So , the left hand side of the equation is equated to the right hand side by the value of 20
Adding 5y on both sides , we get
3x = 20 + 5y
Subtracting 20 on both sides , we get
5y = 3x - 20
Divide by 5 on both sides , we get
y = ( 3x - 20 ) / 5
Therefore , the value of y = ( 3x - 20 ) / 5
Hence , the expression is y = ( 3x - 20 ) / 5
To learn more about equations click :
https://brainly.com/question/19297665
#SPJ6
everything you need is in the image
pls help TT

Answers
Answer:
6 x 7 is 42, so A m a y b e?
Step-by-step explanation:
I NEED HELP ASAP ABOUT TO FAIL

Answers
Hope that helped
If tan(t)=4/9 what is tan(t−π)
Answers
The value of tan(t−π) is 4/9.
According to the statement
we have given that tan(t)=4/9 and we have to find the value of tan(t−π).
So,
tan(t−π) -(1)
take negative sign common from equation (1) it then
tan(t−π) = -tan(-t+π)
and we know that the according to the mathematics formula it become
tan(-t+π) is -tan t
then
tan(t−π) = -(-tan t)
it becomes
tan(t−π) = tan t
then its value becomes
tan(t−π) = tan(t)=4/9.
because we have given that the value of tan t is tan(t)=4/9.
So, The value of tan(t−π) is 4/9.
Learn more about the TRIGONOMETRY here https://brainly.com/question/24349828
#SPJ4
An electron moving at 4.10 *10^3 m/s in a 1.28 T magnetic field experiences a mangetic force of 1.40* 10^-16 N.what angle dose the velocity of the electron make with the magnetic filed? there are two answer between 0° and 180° . Smaller value = ° larger value = °
Answers
The angle which the velocity of electron make with the magnetic field is : Smaller value = 88.3°, Larger value = 91.7°.
The angle that the velocity of the electron makes with the magnetic field is given by:
θ = arctan(F/mv²B)
where F is the magnetic force on the electron,
m is the mass of the electron,
v is the velocity of the electron, and
B is the magnetic field.
Substituting the given values, we have:
θ = arctan((1.40 × 10⁻¹⁶ N)/(9.11 × 10⁻³¹ kg × (4.10 × 10³ m/s)² × 1.28 T))≈ arctan(2.35 × 10⁷)
The angle θ lies between 0° and 90° because the tangent function is positive in the first quadrant.
Using a calculator, we find that:θ ≈ 88.3°
Therefore, the smaller value is 88.3° and the larger value is 180° - 88.3° = 91.7°.
To know more about tangent visit :
brainly.com/question/10053881
#SPJ11
Please help me with this

Answers
In each of Problems 38 through 42, a differential equation and one solution yı are given. Use the method of reduction of or- der as in Problem 37 to find a second linearly independent solution y2. . x2y" + xy' – 9y = 0 (x > 0); yı(x) = x3
Answers
A second linearly independent solution of y₂ is \(-\frac{1}{6x^3}\)
The general Equation is y" + P(x)y' + q(x)y = 0 ...............(i)
where P(x), Q(x) are continues in the internal I ≤ R.
If y₁(x) is a solution of equation 1 in I then y₁(x) ≠ 0.
Then y₂(x) = y₁(x)\(\int{\frac{e^{-\intP(x)dx}}{(y_{1}x)^2}}dx\) is another solution.
The differential equation is x²y" + xy' – 9y = 0 where x > 0.
As y₁(x) = x³ is one solution of differential equation.
Divide throughout by (x²) to given differential equation.
1/x² (x²y" + xy' – 9y = 0)
y" + (y'/x) – (9/x²)y = 0 ................(ii)
By comparing equation (i) & (ii) we get:
p(x)=1/x , q(x)= –are continuous for x>0
So, another solution,
y₂(x) = y₁(x)\(\int{\frac{e^{-\intP(x)dx}}{(y_{1}x)^2}}dx\)
Now putting the values of P(x) And Q(x)
y₂(x) = \(x^3\int\limits {\frac{e^{\int(1/x)dx} }{(x^3)^2}} \, dx\)
y₂(x) = \(x^3\int\limits {\frac{\frac{1}{x} }{x^6} }} \, dx\)
y₂(x) = \(x^3\int\limits {\frac{1}{x^7} }} \, dx\)
y₂(x) = \(x^3\int\limits {x^-7} } \, dx\)
y₂(x) = \(x^3\left[\frac{x^{-7+1}}{-7+1}\right]\)
y₂(x) = \(-\frac{1}{6}(x^3\times x^{-6})\)
y₂(x) = \(-\frac{1}{6x^3}\)
So, the answer of this question is \(-\frac{1}{6x^3}\).
To learn more about Differential equation link is here:
brainly.com/question/14620493
#SPJ4
very fast
Show, by induction, that \( T(n)=10 n^{2}-3 n \quad \) if \( n=1 \)
Answers
Given that \(\(T(n)\) = \(10n^2-3n\)\) if (\(\(n=1\)\)), you have to prove it by induction. So, we have proved it by induction that \($$\(T(n)=10n^2-3n\)$$\) if ( n= 1). The given statement is true for all positive integers n
Let's do it below: The base case (n=1) is given as follows: \(T(1)\) =\(10\cdot 1^2-3\cdot 1\\&\)=\(7\end{aligned}$$\). This implies that \(\(T(1)\)\) holds true for the base case.
Now, let's assume that \(\(T(k)=10k^2-3k\)\) holds true for some arbitrary \(\(k\geq 1\).\)
Thus, for n=k+1, T(k+1) = \(10(k+1)^2-3(k+1)\\&\) = \(10(k^2+2k+1)-3k-3\\&\)=\(10k^2+20k+7k+7\\&\) = \(10k^2-3k+20k+7k+7\\&\) = \(T(k)+23k+7\\&\) = \((10k^2-3k)+23k+7\\&\) = \(10(k+1)^2-3(k+1)\).
Therefore, we have proved that the statement holds true for n=k+1 as well. Hence, we have proved it by induction that \($$\(T(n)=10n^2-3n\)$$\) if (n=1). Therefore, the given statement is true for all positive integers n.
For more questions on: integers
https://brainly.com/question/17695139
#SPJ8
a) The nth term of a sequence is given by 3n + 5. Write down the first four terms of the sequence.
Answers
If you plug in n = 1, then you get 3*n+5 = 3*1+5 = 3+5 = 8. So 8 is the first term.
Repeat for n = 2 and you should get 3n+5 = 3*2+5 = 6+5 = 11. Or simply add 3 to the last term 8 to get 3+8 = 11.
Repeat for n = 3 and n = 4 to get the third and fourth terms to be 14 and 17 respectively.
Answer: The first four terms are 8, 11, 14, 17What is the value of x? (giving brainliest and thanks to all!)

Answers
Answer:
x = 12
Step-by-step explanation:
Given a line parallel to a side of the triangle and it intersects the other 2 sides then it divides those sides proportionally, that is
\(\frac{x}{24}\) = \(\frac{5}{10}\) ( cross- multiply )
10x = 120 ( divide both sides by 10 )
x = 12
1. Let x ∈ Z. Prove that if 3 | 2x, then 3 | x.2. Let n ∈ Z. Prove that 3 | (2n 2 + 1) if and only if 3 - n.
Answers
Answer:
1.Suppose 3 | 2x. Then we can write 2x = 3k for some integer k. Rearranging, we have x = (3/2)k. Since k is an integer, (3/2)k is also an integer, which means that x is divisible by 3. Hence, 3 | x.
First, suppose 3 | (2n^2 + 1). Then we can write 2n^2 + 1 = 3k for some integer k. Rearranging, we have 2n^2 = 3k - 1. Since 3k - 1 is odd, we can write it as 2m + 1 for some integer m. Substituting, we have 2n^2 = 2m + 1, which implies that n^2 = m + (1/2). But since m is an integer, (1/2)m is not an integer, which means that n^2 is not an integer. This is a contradiction, so our assumption that 3 | (2n^2 + 1) must be false.
Now suppose 3 - n. Then we can write n = 3k - 1 for some integer k. Substituting, we have 2n^2 + 1 = 18k^2 - 12k + 3. Factoring out 3, we have 2n^2 + 1 = 3(6k^2 - 4k + 1). But 6k^2 - 4k + 1 is always an integer, so if 3 - n, then 3 | (2n^2 + 1).
give me thanks for more! your welcome!
Step-by-step explanation:
A metal pole to hang banners and advertisements is attached to a brick
building to form a right angle. A diagonal brace is placed 5 feet below the
pole to give support. What is the length in feet of the pole?
13 ft
5 ft
Answers
The length in feet of the pole is 13 ft.
What is Pythagoras theorem?The right-angled triangle's relationship between its three sides is explained by the Pythagoras theorem, commonly known as the Pythagorean theorem. The square of a triangle's hypotenuse is equal to the sum of its other two sides' squares, according to the Pythagoras theorem. Let's learn more about the Pythagoras theorem, its proofs, and its equations before moving on to cases that have been solved using the triangle and square Pythagoras theorem. The hypotenuse's square is equal to the sum of the squares of the other two sides if a triangle has a straight angle (90 degrees), according to the Pythagoras theorem. Keep in mind that BC² = AB² + AC² in the triangle ABC signifies this.
Use the Pythagorean relationship:
c²= a² + b²
= 5² + 12²
= 25 + 144
= 169
c = 13
To know more about Pythagoras theorem ,visit:
brainly.com/question/343682
#SPJ13
What does x equal? −3(2x−3)=−6x+9
Answers
Answer: The answer for this question is x=1
Step-by-step explanation: -3(2x-3)=-6x+9 first you multiply -3x with 2x and -3 to get -6x+9=-6x+9 then subtract nine from both sides to leaves -6x=-6x then you divide -6 on both sides and you get x=1.
Any value of x makes the equation true. All real numbers
Interval Notation: (−∞,∞)
Because if you solve it
-3(2x-3)=-6x+9
distribute
-6x + 9 = -6x + 9
subtract 9 from both sides
-6x = -6x
simplify
0 = 0