On a coordinate plane, kite W X Y Z is shown. Point W is at (negative 3, 3), point X is at (2, 3), point Y is at (4, negative 4), and point Z is at (negative 3, negative 2).
What is the perimeter of kite WXYZ?
Answers
The perimeter of the kite is (10 + 2√53) units if the kite and the coordinates of the kite are point W is at (-3, 3), point X is at (2, 3), point Y is at (4, -4), and point Z is at (-3, -2).
What is quadrilateral?It is defined as the four-sided polygon in geometry having four edges and four corners. Kite is quadrilateral, in which. Two pairs of congruent sides, and it has one pair of opposite congruent angles.
The figure is missing.
On a coordinate plane, kite WXYZ is shown (please refer to the picture)
We have a kite and the coordinates of the kite are:
Point W is at (-3, 3), point X is at (2, 3), point Y is at (4, -4), and point Z is at (-3, -2).
Using the distance formula we can find the distance between coordinates:
\(\rm d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
Distance between W and X:
\(\rm WX=\sqrt{(2+3)^2+(3-3)^2}\)
WX = 5 units
Similarly, distance between X and Y:
XY = √53 units
YZ = √53 units
ZW = 5 units
Perimeter = sum of the all sides
Perimeter = 5 + √53 + √53 + 5
Perimeter = (10 + 2√53) units
Thus, the perimeter of the kite is (10 + 2√53) units if the kite and the coordinates of the kite are point W is at (-3, 3), point X is at (2, 3), point Y is at (4, -4), and point Z is at (-3, -2).
Learn more about the quadrilateral here:
brainly.com/question/6321910
#SPJ1

Related Questions
Let X and Y be discrete random variables and let a and b be constants Which of the following is. FALSE? (a) mean (X + Y) = mean (X) + mean (Y).
Answers
Mean (X + Y) = Mean (X) + Mean (Y).
The above statement is false.
Discrete Random Variable:
A discrete random variable can be defined as a type of variable whose value depends on the numerical outcome of some random phenomenon. Also called a random variable. Discrete random variables are always easily countable integers. A probability mass function is used to describe the probability distribution of a discrete random variable.
Probability Distributions of Discrete Random Variables:
Probability distributions of discrete random variables list the probabilities associated with each possible outcome. Also called probability function or probability mass function.
The probability of a discrete random variable is between 0 and 1. Also, the sum of the probabilities of a discrete random variable is equal to 1. The probability distribution of discrete random variables resembles the normal distribution.
Example:
Suppose two dice are rolled and a random variable X is used to represent the sum of the numbers. The minimum value of X goes from result 1 + 1 = 2 to 2 and the maximum value goes from result 6 + 6 = 12 to 12. Therefore, X can have any value between 2 and 12 (inclusive). If probabilities are assigned to each outcome, we can determine the probability distribution of X.
Discrete random variables should not be confused with algebraic variables. Algebraic variables represent the values of unknown quantities in computable algebraic equations. However, a discrete random variable can have a range of possible values that result from experimentation.
Learn more about Discrete Random Variable:
https://brainly.com/question/12970235
#SPJ4
There are 9.5 ounces of juice in a container. An additional 1.75 ounces of juice are poured into the container each second. How many ounces of juice are in the container after 6 seconds? Enter your answer in the box
Answers
By concept of capacity and the assumption of constant flow rate, the amount of ounces of juice in the container after 6 seconds is 20 ounces.
How to determine the final capacity of a container
Given that the additional juice is added to the container at constant rate. Hence, the final capacity (C'), in ounces is equal to the sum of the initial capacity (C), in ounces, and the product of the flow rate (q), in ounces per second, and time (t), in seconds.
C' = C + q · t (1)
If we know that C = 9.5 oz, q = 1.75 oz/s and t = 6 s, then the final capacity of the container is:
C' = 9.5 oz + (1.75 oz/s) · (6 s)
C' = 20 oz
By concept of capacity and the assumption of constant flow rate, the amount of ounces of juice in the container after 6 seconds is 20 ounces.
To learn more on ounces: https://brainly.com/question/26950819
#SPJ1
PLEASE HELP!!!!!!! IM STRUGGLING

Answers
please help with this

Answers

does a debit card use a pin or a credit card, or do both?
Answers
HELP PLEASE !!!!!!!!!!!!!!!!!!!!!!!!!!
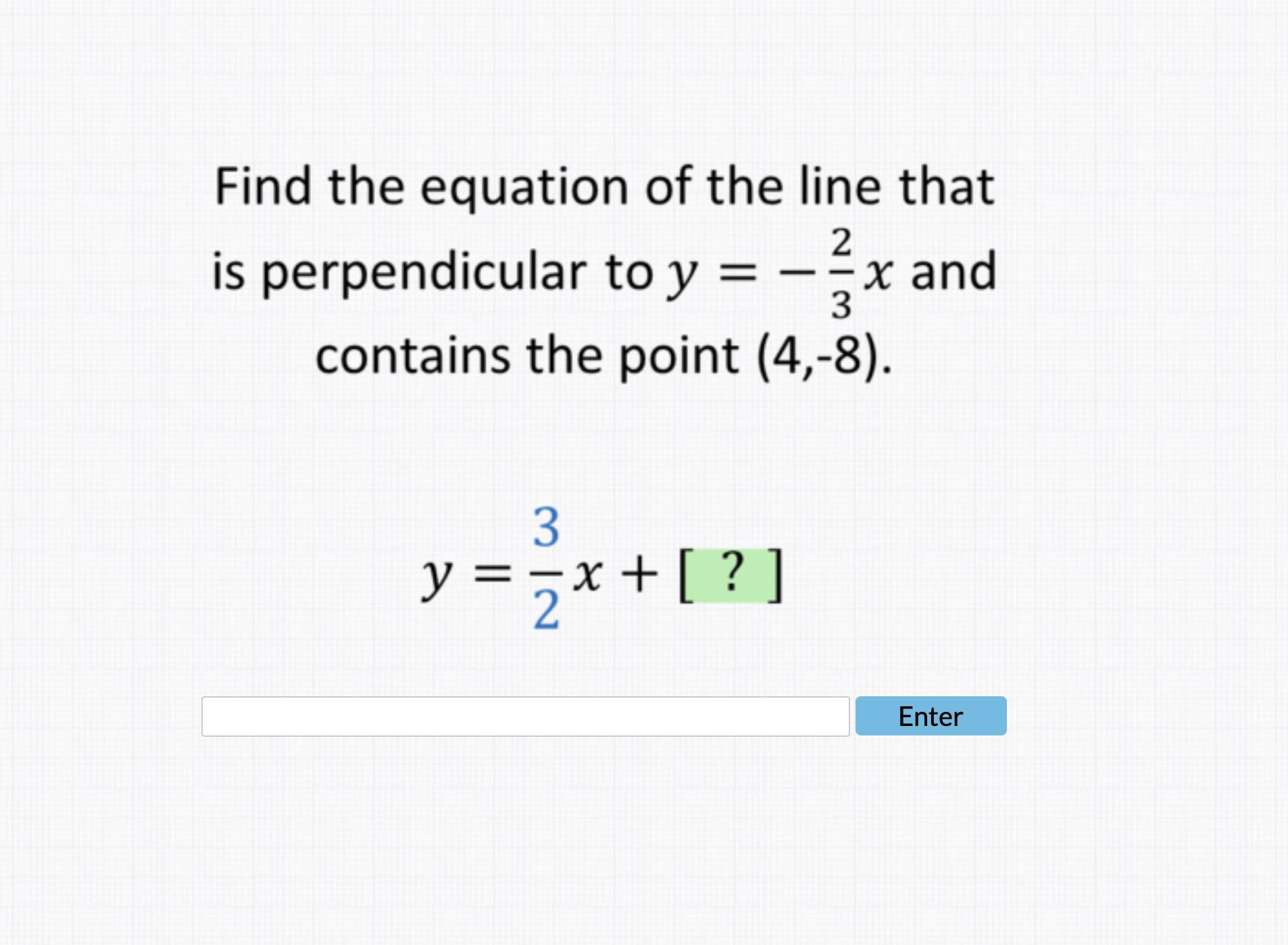
Answers
The equation of the line perpendicular to y = - (2 / 3) · x and the point (4, - 8) is y = (3 / 2) · x - 14.
How to determine the equation of a perpendicular line
Mathematically speaking, lines are represented by equations of the form y = m · x + b, where m and b are the slope and the intercept of the line. Additionally, two lines are perpendicular to each if the product of their slopes is equal to - 1.
First, we determine the slope of the perpendicular line:
m' = - 1 / m
m' = - 1 / (- 2 / 3)
m' = 3 / 2
Second, the intercept of the perpendicular line is:
b = y - m · x
b = - 8 - (3 / 2) · 4
b = - 8 - 6
b = - 14
Then, the equation of the perpendicular line is y = (3 / 2) · x - 14.
To learn more on perpendicular lines: https://brainly.com/question/17565270
#SPJ1
If start fraction 1 over 3 end fraction is equivalent to 33start fraction 1 over 3 end fraction%, what percent is equivalent to two-thirds? A. 33two-thirds% B. 150% C. 66two-thirds% D. 65%
Answers
Answer:
The answer is C 66%
Step-by-step explanation:
2
7
What is the area of the triangle?
units?

Answers
Step-by-step explanation:
the area of a triangle is always
baseline × height / 2
where baseline and height are perpendicular (at a 90° angle) to each other.
so, in our case the area is
7 × 2 / 2 = 7 × 1 = 7 units²
A conglomerate corporation develops, manufactures, and markets a wide range of products, including medical diagnostic imaging devices, jet engines, lighting products, and chemicals. In 2013, the stock price rose %, and in 2014, the stock price declined %. If one purchased $1,000 of some social media stock at the start of 2013, its value would be $ at the end of 2014.
Required:
Compute the geometric mean rate of return per year of the two-year period 2013-2014.
Answers
Complete question :
A conglomerate corporation develops, manufactures, and markets a wide range of products, including medical diagnostic imaging devices, jet engines, lighting products, and chemicals. In 2013, the stock price rose 34.83 %, and in 2014, the stock price declined 8.2%. If one purchased $1,000 of some social media stock at the start of 2013, its value would be $ at the end of 2014.
Answer: 0.2078330
Step-by-step explanation:
The geometric mean is calculated using :
[((1 + r1)(1+r2)(1+r3)...(1+rn))^(1/n) ]- 1
From the question:
2014 stock decline = 8.2% = rate 1(r1) = 0.082
2013 stock increase = 34.83% rate 2(r2) = 0.3483
Number of observations (n) = 2
Hence, geometric mean:
[((1 + r1)(1 + r2))^(1/n)] - 1
[((1 + 0.082) (1 + 0.3483))^(1/2)] - 1
Sqrt[(1.082)(1.3483)] - 1
Sqrt(1.4588606) - 1
= 1.2078330 - 1
= 0.2078330
Complete the following question:
Medical diagnostic imaging devices, jet engines, lighting products, and chemicals are just some of the products that a conglomerate corporation develops, manufactures, and markets. In 2013, the stock price increased by 34.83 percent, but it fell by 8.2 percent in 2014. If you bought $1,000 worth of social media stock in the beginning of 2013, it would be worth $ by the end of 2014.
0.02078330 is the answer.
Step-by-step instructions:
The geometric mean is found by multiplying the following numbers together:
[((1 + r1)(1+r2)(1+r3)...(1+rn))(1/n) ] (((1 + r1)(1+r2)(1+r3)...(1+rn) ] ((1 + r1)(1+r2)(1
+ 1
As a result of the question:
Rate 1(r1) = 0.082 for 2014 stock decline = 8.2 percent
Increase in stock in 2013 = 34.83 percent rate 2(r2) = 0.3483
The number of observations (n) is equal to two.
As a result, the geometric mean is calculated as follows:
[((1 + r1)(1 + r2))(1/n)] [(1 + r1)(1 + r2)] [(1 + r1)(1 + r2)] [ + 1
[((1 + 0.082) (1 + 0.3483)) [((1 + 0.082) (1 + 0.3483)) [((1 + 0.082) (1 + 0.3483)) [ + 1
Sqrt[(1.082)(1.3483)] is the square root of the number Sqrt[(1.082)(1.3483)]. + 1
sqrt(1.4588606) - 1 sqrt(1.4588606) sqrt(1.4588606) sqrt
1.2078330 - 1 = 1.2078330 + 1 = 1.2078330 + 1 = 1.
equals 0.2078330
Solve 2^x-2=8^4 but not solving for x
Answers
Without explicitly solving for x, we can conclude that the solution to the equation 2^x - 2 = 8^4 is x = 12.
To solve the equation 2^x - 2 = 8^4 without explicitly solving for x, we can simplify the equation using exponent rules and observe the relationship between the numbers.
First, let's simplify the equation:
2^x - 2 = 8^4
We know that 8 can be expressed as 2^3, so we can rewrite the equation as:
2^x - 2 = (2^3)^4
Applying the exponent rule (a^m)^n = a^(mn), we can simplify further:
2^x - 2 = 2^(34)
Simplifying the right side of the equation:
2^x - 2 = 2^12
Now, we can observe that both sides of the equation have the same base, which is 2. In order for the equation to hold true, the exponents must be equal:
x = 12
Therefore, we may deduce that the answer to the equation 2x - 2 = 84 is x = 12 without having to explicitly solve for x.
for such more question on equation
https://brainly.com/question/17482667
#SPJ8
A tank is full of water. Find the work (in ft-lb) required to pump the water out of the spout. Use the fact that water weighs 62.5 lb/ft3. (Round your answer to the nearest whole number.) 3 ft6 ft12 ft A frustum of a cone with a spout is given. The smaller radius is 3 ft, the larger radius is 6 ft, and the height is 12 ft.
Answers
The work required to pump the water out of the spout is approximately 64,307,077 ft-lb
To find the work required to pump the water out of the spout, we need to calculate the weight of the water in the tank and then convert it to work using the formula: work = force × distance.
First, let's calculate the volume of water in the tank. The frustum of a cone can be represented by the formula: V = (1/3)πh(r1² + r2² + r1r2), where r1 and r2 are the radii of the two bases and h is the height.
Given r1 = 3 ft, r2 = 6 ft, and h = 12 ft, we can calculate the volume:
V = (1/3)π(12)(9 + 36 + 18) = 270π ft³
Now, we can calculate the weight of the water using the density of water:
Weight = density × volume = 62.5 lb/ft³ × 270π ft³ ≈ 53125π lb
Next, we convert the weight to force by multiplying it by the acceleration due to gravity (32.2 ft/s²):
Force = Weight × acceleration due to gravity = 53125π lb × 32.2 ft/s² ≈ 1709125π lb·ft/s²
Finally, we can calculate the work by multiplying the force by the distance. Since the water is being pumped out of the spout, the distance is equal to the height of the frustum, which is 12 ft:
Work = Force × distance = 1709125π lb·ft/s² × 12 ft ≈ 20509500π lb·ft ≈ 64307077 lb·ft
for more search question water
https://brainly.com/question/17120212
#SPJ8
andrew bought a camera on sale at a 20% discount. it was marked down from its regular price of 120$ if there is an 8% sales tax on the sales price, how much did andrew pay for the camera?
Answers
Answer:
$103.68
Step-by-step explanation:
20% 0f 120 is 24
120-24=96
8% of 96 is 7.68
96 + 7.68 = 103.68
90 men and 60 women were asked if they had watched the latest ‘Expendables’ movie. Altogether 3/5 of the people said Yes.
3/10 of the women said Yes.
What percentage of the men said No? (Show your working)
(THE ANSWER SHOULD BE 20% BUT HOW TO PROVE THAT????)
HELP ME PLEASE
Answers
150 total people
3/5 * 150 = 90 total people said yes
3/10 * 60 = 18 women said yes
(42 women said no)
(72 men said yes)
Subtract the people who said yes from the total people to find the people who said no.
150 - 90 = 60 people said no
60 - 42 = 18 men said no
18/90 = 0.20 or 20%
The spring shown below is sitting on a flat surface and it is pushed together with a weight.
What type of energy does the spring currently have?
A.
elastic potential energy
B.
kinetic energy
C.
gravitational potential energy
D.
chemical potential energy
Help plz
Answers
Answer:
its elastic i got it correct trust me also put brainliet plz
Step-by-step explanation:
order the following expressions from least to greatest.
2 √3 √pie
Answers
The order of expressions from the least to greatest is \(\sqrt{3}\), \(\sqrt{pie}\) and 2
In order to find the value of these given expressions, we will have to convert them into a standard form, therefore we will find the value of these expressions by removing the root and finding its actual value
Value of 2 will remain the same as it is already in standard form
Value of \(\sqrt{3}\) in standard form = 1.732
Value of \(\sqrt{pie}\) in standard form = \(\sqrt{3.1459}\) = 1.77
No ordering these expressions we find that 2 is the greatest, followed by 1.77 and 1.732
Hence, the expressions from least to greatest is \(\sqrt{3}\), \(\sqrt{pie}\) and 2
Learn more about expressions:
https://brainly.com/question/14083225
#SPJ9
Chris saved x dollars and spent half of his savings buying video games. After earning an additional $10 cutting grass, he had $32. If the equation one half times x plus 10 equals 32 represents the scenario, how much did he start with in his savings? $11 $21 $44 $84
Answers
The amount Chris started with in his savings is $44. option C
EquationAmount Chris saved = $xAmount spent on video games = 1/2xAdditional amount earned = $10Total = $321/2x + 10 = 32
subtract 10 from both sides1/2x = 32 - 10
1/2x = 22
divide both sides by 1/2x = 22 ÷ 1/2
x = 22 × 2/1
x = $44
Therefore, the amount Chris started with in his savings is $44. option C
Learn more about equation:
https://brainly.com/question/2972832
#SPJ1
Answer:
Step-by-step explanation:
Which value of n makes this equation true?
3n + 3
5
5n– 1
9
OA.
n=-16
OB.
n=-2
O C.
n= 2
OD.
n = 16
Answers
Answer:
the answer is A. -16
Step-by-step explanation:
3(-16)+3= -45
-45/5=
-9
5(-16)-1=-81
-81/9=
-9
-9=-9
what is 0.26 rounded to the nearest tenth.
Answers
Answer:.3
Step-by-step explanation: if it is 5 or above it rounds up
Find the area of the shaded portion in the equilateral triangle with sides 6. Show all work for full credit.
(Hint: Assume that the central point of each arc is its corresponding vertex.)
Answers
The area of the shaded portion in the equilateral triangle with sides 6 is 9√3 - 36π.
To find the area of the shaded portion in the equilateral triangle, we need to determine the area of the three arcs and subtract it from the area of the equilateral triangle.
First, let's find the area of one arc. Each arc has a radius equal to the length of the side of the equilateral triangle, which is 6. The formula for the area of a sector is A = (θ/360)πr², where θ is the central angle in degrees.
In an equilateral triangle, each interior angle measures 60 degrees, so the central angle of the arc is 120 degrees (360 degrees divided by 3). Plugging these values into the formula, we get A_arc = (120/360)π(6)² = (1/3)π(6)² = 12π.
Since there are three identical arcs, the total area of the arcs is 3 times the area of one arc, which is 3(12π) = 36π.
Now, let's find the area of the equilateral triangle. The formula for the area of an equilateral triangle is A_triangle = (√3/4)s², where s is the length of a side.
Plugging in the value of the side length, we have A_triangle = (√3/4)(6)² = (√3/4)(36) = 9√3.
Finally, we subtract the area of the arcs from the area of the equilateral triangle to find the shaded portion's area: A_shaded = A_triangle - A_arc = 9√3 - 36π.
For more such questions on triangle
https://brainly.com/question/1058720
#SPJ8
A university administrator was interested in determining if there was a difference in the distance students travel to get from class from their current residence(in miles). Men and women at UF were randomly selected. The Minitab output is below. What is the best interpretation for the output?
Difference = mu (F) - mu (M)
T-Test of difference = 0 (vs not =): T-Value = -1.05 P-Value = 0.305 DF = 21
A. With a p-value of 0.305, we do have statistically significant evidence that the population mean distance traveled to class is different for men and women.
B. With a p-value of 0.305, we do have statistically significant evidence that the population mean distance traveled to class is the same for men and women.
C. With a p-value of 0.305, we do not have statistically significant evidence that the population mean distance traveled to class is the same for men and women.
D. With a p-value of 0.305, we do not have statistically significant evidence that the population mean distance traveled to class is different for men and women.
Answers
Answer:
D. With a p-value of 0.305, we do not have statistically significant evidence that the population mean distance traveled to class is different for men and women.
Step-by-step explanation:
What we are testing?
If the distance students travel to get from class is statistically different for men and women.
How we take the decision?
The significance level is the standard of 0.05.
If the p-value is greater than 0.05, there is no evidence that the distances are statistically different for the population.
If the p-value is less than 0.05, there is evidence that the distances are statistically different different for the population..
In this question:
p-value: 0.305 > 0.05, so there is no statistically significant evidence that the means are difference, and the correct answer is given by option d.
Given ZA and ZB are vertical angles with mZA= 50x + 10 and mZB= 70% – 10. What is x? Enter your answer in the box. X=
Answers
Vertical angles are angles that are opposite each other when the lines cross and they are equal to each other in that they have the same measure.
Keeping that in mind, we get the equation
\(50x+10=70x-10\)Let us subtract 50 x from both sides. This gets us
\(10=20x-10\)and then let us add 10 to both sides; this gives
\(20x=20\)Finally, dividing both sides by 20 gives
\(x=1\)which is our answer!
The height of a rectangular prism is 15 cm the area of its base is 270 square cm. What is the volume of the rectangular prism
Answers
Answer:
4050 cm cubed
Step-by-step explanation:
15 * 270 = 4050 cm cubed
The line 2x + 5y = 1 meets the curve x² + 5xy - 4y² + 10 = 0 at the points A and B
Answers
Answer:
A(3,-1) and B( -2,1)
Step-by-step explanation:
2x+5y=1. , or. y=(1–2x)/5………….(1)
x^2+5xy-4y^2+10=0…………………..(2)
Putting y=(1–2x)/5 from eqn. (1)
x^2+5x.(1–2x)/5–4/25.(1–2x)^2 +10=0
or. 25x^2+25x.(1–2x)-4.(1+4x^2–4x)+250 = 0
or. 25x^2+25x-50x^2–4–16x^2+16x+250=0
or. 41x^2–41x-246=0
or x^2– x - 6=0
or. (x-3).(x+2)=0
=> x= 3 or -2
But y=(1–2x)/5
=> y= (1–6)/5 or. (1+4)/5
hence, y= -1. or. 1
For each graphically defined function below, state the domain, the range, and the intervals over which the function is increasing, decreasing, or constant.

Answers
The domain of the function above is [-2, 3].
The range of the function above is [-2.5, 2].
The intervals over which the function is increasing is [-2, 1.5] U [-2.5, 2].
The intervals over which the function is decreasing is [1.5, -2.5].
What is a domain?In Mathematics and Geometry, a domain refers to the set of all real numbers for which a particular function is defined.
Furthermore, the vertical extent of any graph of a function represents all range values and they are always read and written from smaller to larger numerical values, and from the bottom of the graph to the top.
By critically observing the graph of this rational expression (function) shown in the image attached below, we can reasonably and logically deduce the following domain and range:
Domain = [-2, 3] or -2 ≤ x ≤ 3.
Range = [-2.5, 2] or -2.5 ≤ y ≤ 2.
Additionally, the intervals over which the function is increases over the interval [-2, 1.5] and [-2.5, 2], while it decreases over the interval [1.5, -2.5].
In conclusion, the given function is not constant over any interval.
Read more on domain here: brainly.com/question/17003159
#SPJ1
To get from home to his friend Akira's house, Jaden would have to walk 2.8 kilometers due
north. To get from home to his friend Cooper's house, Jaden would have to walk 6.3
kilometers due east. What is the straight-line distance between Akira's house and Cooper's
house? If necessary, round to the nearest tenth.
Answers
The straight-line distance between Akira's house and Cooper's house is 6.13 kilometers (rounded to the nearest tenth)
Given that,To get from home to his friend Akira's house, Jaden would have to walk 2.8 kilometers due east.The straight-line distance between Akira's house and Cooper's house is given by the distance between two points in a coordinate plane. Let the home be the origin (0, 0) of the coordinate plane and Akira's house be represented by the point (2.8, 4.7). Similarly, let Cooper's house be represented by the point (8.3, 7.4).The distance formula between the two points (2.8, 4.7) and (8.3, 7.4) is given by:distance = √[(8.3 - 2.8)² + (7.4 - 4.7)²]= √[5.5² + 2.7²]= √(30.25 + 7.29)= √37.54= 6.13 km (rounded to the nearest tenth)
for more search question distance
https://brainly.com/question/30395212
#SPJ8
Let x be a random variable that represents the batting average of a professional baseball player. Let y be a random variable that represents the percentage of strikeouts of a professional baseball player. A random sample of n = 6 professional baseball players gave the following information.
x 0.350 0.270 0.340 0.248 0.367 0.269
y 2.8 7.0 4.0 8.6 3.1 11.1
Verify that Σx = 1.844, Σy = 36.6, Σx2 = 0.579554, Σy2 = 279.62, Σxy = 10.4864, and r ≈ -0.896.
Σx 1
Σy 2
Σx2 3
Σy2 4
Σxy 5
r 6
Answers
the answer is Let x be a random variable that represents the batting average of a professional baseball player. Let y be a random variable that represents the percentage of strikeouts of a professional baseball player. A random sample of n = 6 professional baseball players gave the following information.
x 0.350 0.270 0.340 0.248 0.367 0.269
y 2.8 7.0 4.0 8.6 3.1 11.1
Verify that Σx = 1.844, Σy = 36.6, Σx2 = 0.579554, Σy2 = 279.62, Σxy = 10.4864, and r ≈ -0.896.
Σx 1
Σy 2
Σx2 3
Σy2 4
Σxy 5
r 6
7. Six regular hexagons surround a regular hexagon of side length 1 as shown. What is the area of A ABC? B 8. In rectangle ABCD, DC = 2CB and points E and Flie on AB so that ED and FD trisect ZADC as shown. What is the ratio of the area of A DEF to the area of rectangle ABCD? A E F B D С 9. Eight semicircles lie the inside of a square with side length 2 as shown. What is the area of the circle in the middle that touches all the semicircles? Chapter 10 Test - Take Home 1. If the area of an equilateral triangle is tripled, what happened to its perimeter? Show all your work A=²b-h P=3b 34= 1 / 2 1 2. Let R be the region enclosed by the x-axis, the y-axis and the line y = - -x +4. If a point is 2 chosen randomly from R, what is the probability that the distance between this point and the origin is larger than 2? 3. Let points A(0,0), B(1, 2), C(3, 3), and D(4, 0)). Quadrilateral ABCD is cut into equal area pieces by a line passing through A. Find the point at which this line intersects CD. 4. A regular hexagon has side length 6. Congruent arcs with radius 3 are drawn with the center at each of the vertices, creating circular sectors as shown. The region inside the hexagon but outside the sectors is shaded as shown. What is the area of the shaded region? 720 720 A=Tr? 9 =IM - 120° 3 12 3 60 5. Equilateral A ABC has side length 1, and squares A BCD, BCHI, CAF G lie outside the triangle. What is the area of hexagon DEFGHI? D 6. A square in the coordinate plane has vertices whose y-coordinates are 0, 1, 4 and 5. What is the area of the square?
Answers
If six regular hexagons surround a regular hexagon of side length 1 then \(3\sqrt{3}\) is the area of triangle ABC.
To find the area of triangle ABC, we need to first find the height of the equilateral triangle ABE. Since AB is the base of the equilateral triangle, we can use the formula for the height of an equilateral triangle, which is h = \((\sqrt{3} /2) * s\), where s is the side length.
Substituting s = 1, we get h = \((\sqrt{3} /2)\).
Now, we can use the formula for the area of a triangle, which is A = (1/2) x b x h, where b is the base of the triangle. Since the base of triangle ABC is AB, which is also the side length of the small hexagon, we have b = 1. Substituting the value of h, we get A = (1/2) x 1 x \((\sqrt{3} /2)\)= \((\sqrt{3} /4)\).
Since there are six identical triangles surrounding the small hexagon, the total area of the six triangles is 6 x\((\sqrt{3} /4)\)= (3/2) x \(\sqrt{3}\). Therefore, the area of triangle ABC is equal to the total area of the six triangles minus the area of the small hexagon. The area of the small hexagon can be found using the formula for the area of a regular hexagon, which is A = (3\((\sqrt{3} /2) * s^2\) , where s is the side length. Substituting s = 1, we get A = \((\sqrt{3} /2)\).
Therefore, the area of triangle ABC is (3/2) x \(\sqrt{3}\) - (3\(\sqrt{3} /2\)) = \((\sqrt{3} /2)\) square units.
So, the answer is (a) 3sqrt (3).
To learn more about hexagons visit;
https://brainly.com/question/3295271
#SPJ4
Correct question:
Six regular hexagons surround a regular hexagon of side length 1 as shown. What is the area of triangle ABC?
a) 3sqrt(3)
b) 4sqrt(3)
c) 6sqrt(3)
d) 9sqrt(3)
I need help to set up an equation the find the value of x. Then, to explain my reasoning.

Answers
the two horizontal lines are parallel so that mean that:
\(70=3x-14\)and we can solve for x
\(\begin{gathered} 70-14=3x \\ 56=3x \\ x=\frac{56}{3} \\ x=18.66 \end{gathered}\)Jeff's roof is three times larger than Monica's roof. What is the measurement of the side where there is a question mark in the picture below? A. 7.64 ft B. 7.73 ft C. 7.93 ft D. 11.6 ft
Answers
Answer:
Step-by-step explanation:
eff's roof is three times larger than Monica's roof. What is the measurement of the side where there is a question mark in the picture below? A. 7.64 ft B. 7.73 ft C. 7.93 ft D. 11.6 ft
Answer:
The answer is B: 7.73 ft
Step-by-step explanation:
I was able to solve it by dividing 23.19 ft (Jeff's roof side length) by 3. BONUS: I got it right on the test! :D
Hope you like my answer!
Determine the area of the given region.

Answers
\(\displaystyle \int_{0}^{\frac{\pi }{3}}~\sin(x)dx\implies \left.\cfrac{}{} -\cos(x)\right]_{0}^{\frac{\pi }{3}}\implies\left( -\cfrac{1}{2}\right) ~~ - ~~(-1)\implies \cfrac{1}{2}\)