Order of Operations With Integers. Please help me solve and how you got the answer!! help, please!!

Answer:

its 35

Step-by-step explanation:

Answer:o.75 you need to divide

Step-by-step explanation:

Pythagorean theorem tells us how the side lengths of right triangles are related.

The Pythagorean theorem, also known as the Pythagorean identity, states that the sum of the squares of the lengths of the two sides forming the right angle is equal to the square of the length of the hypotenuse in any right triangle (the side opposite the right angle). In other words, the Pythagorean theorem can be used to calculate the length of the hypotenuse given the lengths of the two sides of a right triangle.

This relationship can be written as the equation:

\(a^2 + b^2 = c^2\)

where a and b are the lengths of the legs, and c is the length of the hypotenuse. The Pythagorean Theorem is named after the ancient Greek mathematician Pythagoras, who is credited with its discovery.

For more question on Pythagorean theorem click on

https://brainly.com/question/343682

#SPJ4

This student performed better than 68% of the other test-takers in the verbal part and better than 27% in the quantitative part.

Given,

Test-taker scored at the 68th percentile for their verbal grade and at the 27th percentile for their quantitative grade .

Now,

68% percentile : 68% scores equal or less .

27% percentile : 27^ scored equal or less .

Thus option D

This student performed better than 68% of other test taker in verbal and better than 27% in quantitative part .

Know more about percentile,

https://brainly.com/question/2416601

#SPJ12

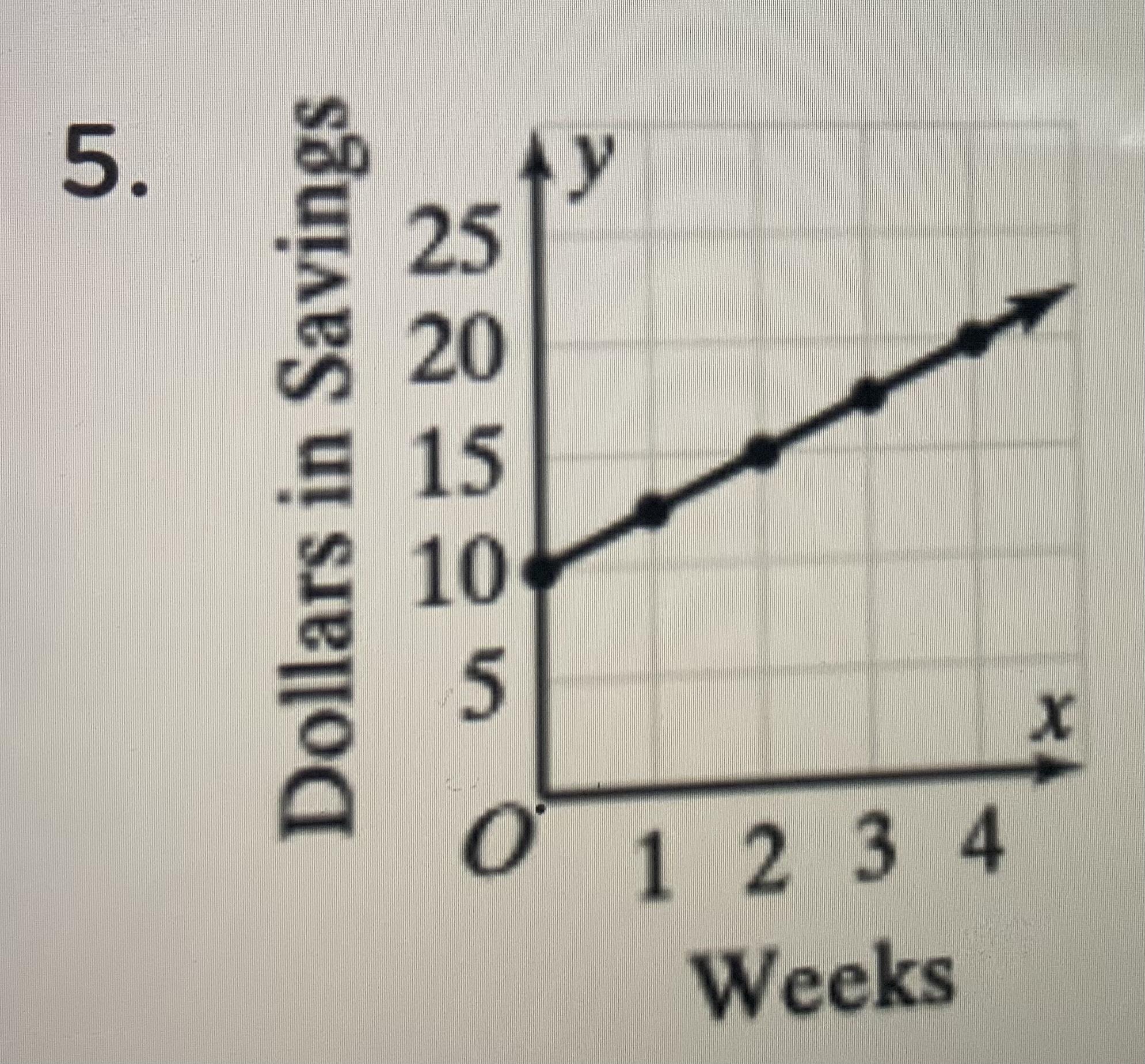
Answer:

Step-by-step explanation:

x+10=0

x=-10

so vertex is (-10,-105)

The mathematical word describing  both \(27x\) and \(6x\) in the expression \(27x+6x\) is "addition"

You can represent the unknown amounts by the use of variables. Follow whatever the description is and convert it one by one mathematically. For example if it is asked to increase some item by 4 , then you can add 4 in that item to increase it by 4. If something is for example, doubled, then you can multiply that thing by 2 and so on methods can be used to convert description to mathematical expressions.

For the given case, the terms were \(27x\) and \(6x\) and the expression formed from them is \(27x+6x\)

This means both the terms were added together, as denoted by '+' (called 'plus') sign.

When two terms are written with 'plus' sign in between, then that means they're added to each other and the result will be addition of both of their's values.

Thus, the mathematical word describing  both \(27x\) and \(6x\) in the expression \(27x+6x\) is addition"

Learn more about addition here:

https://brainly.com/question/14148883

Answer:

y = -x-6

Step-by-step explanation:

The slope intercept form is

y = mx+b

where m is the slope and b is the y intercept

y = -1x -6

y = -x-6

Answer:

f = 3

g = -1

h = 6

r = 18

Step-by-step explanation:

Box 1

f(x) = \(2x^{4}\)-\(12x^{3}\)+\(16x^{2}\)+4x+15 with x = 3

f(3) = \(2(3^{4)}\) - \(12(3^{3} )\) + \(16(3^{2})\)+ 4(3) + 15

Reorder

Evaluate

Multiply

3f = 2 x 81 - 12 + 27 +16 x 9 + 12 +15

3f = 162 - 324 + 144 + 12 + 15

3f =  9

3  ÷  3

f = 3

Box 1

g(x) = \(3x^{3}\) - \(16x^{2}\) - 7x - 36 with x = 6

g(6) = \(3(6^{3} )\) - \(16(6^{2})\) - 7(6) - 36

g6 = 3 x 216 - 576 - 42 - 36

g6 = -6

6   ÷ 6

g = -1

Box 2

h(x) = \(5x^{3}\) - \(2x^{2}\) - 3 with x = -1

h(-1) = \(5(-1^{3} )\) - \(2(-1^{2})\) -3

h-1 = -5 + 2 -3

h-1 = -6

-1    ÷  - 1

h = -6

Box 2

r(x) = \(4x^{4}\) - \(9x^{2}\) + 5x - 2 with x = 2

r(2) = \(4(2^{4} )\) - \(9(2^{2} )\) + 5(2) - 2

r2 = 64 - 36 + 10 - 2

r2 = 36

2   ÷  2

r = 18

Answer:

Step-by-step explanation:

The formula for finding the midpoint of two coordinates is expressed as;

M(X,Y) = \((\frac{x_1+x_2}{2} , \frac{y_1+y_2}{2})\)

Given the coordinates c(-4,5) and d(-1,-4), x1 = -4, y1 = 5, x2 = -1 and y2 = -4.

For the X coordinate of the midpoint

X = x1+x2/2

X = -4+(-1)/2

X = -4-1/2

X = -5/2

X = -2.5

Similarly for Y:

Y = y1+y2/2

Y = 5+(-4)/2

Y = 5-4/2

Y = 1/2

Y = 0.5

Hence the midpoint coordinate of C(-4,5) and D (-1,-4) is (-2.5, 0.5)

Total number of ways to paint rooms is 19,740.

We can solve this problem using the combinatorics. First, we choose the color for the living rooms, which can be done in 3*2 = 6 ways (yellow, red, or purple). Then, we choose the colors for the remaining 10 rooms.

Case 1 : living room is yellow and red

Then remaining colors are 3 yellow, 4 red, 3 purple

number of ways to paint living room = 2

number of ways to pain bedroom = 10!/(3! 4! 3!)

So number of ways = 2 * 10!/(3! 4! 3!) = 8400

Case 2 : living room is yellow and purple

Then remaining colors are 3 yellow, 5 red, 2 purple

So number of ways = 2 * 10!/(3! 5! 2!) = 5040

Case 3 : living room is red and purple

Then remaining colors are 4 yellow, 4 red, 2 purple

So number of ways = 2 * 10!/(4! 4! 2!) = 6300

Total number of ways = 8400 + 5040 + 6300 = 19,740

To know more on combinatorics

https://brainly.com/question/15181927

#SPJ4

Answer:

(- 1, 9 )

Step-by-step explanation:

6x - y = - 15 → (1)

x + 2y = 17 → (2)

multiplying (1) by 2 and adding to (2) will eliminate y

12x - 2y = - 30 → (3)

add (2) and (3) term by term to eliminate y

13x + 0 = - 13

13x = - 13 ( divide both sides by 13 )

x = - 1

substitute x = - 1 into either of the 2 equations and solve for y

substituting into (2)

- 1 + 2y = 17 ( add 1 to both sides )

2y = 18 ( divide both sides by 2 )

y = 9

solution is (- 1, 9 )

Answer:

(800 x 9) + (10 x 9)

Step-by-step explanation:

Find out what 810 x 9 is:

810 x 9 = 7290

Then see which expression has the same answer. Personally I'd check all of them, but luckily the first expression is the correct one so you don't need to go through the rest (since it only asks for one correct expression):

(800 x 9) = 7200

(10 x 9) = 90

7200 + 90 = 7290

The probability that X is between 57 and 105 is 0.8914.

Given:

* X is normally distributed with mean=90 and standard deviation=12

* P(57 < X < 105)

Solution:

* Convert the given values to z-scores:

   * z = (X - μ) / σ

   * z = (57 - 90) / 12 = -2.50

   * z = (105 - 90) / 12 = 1.25

* Use the z-table to find the probability:

   * P(Z < -2.50) = 0.0062

   * P(Z < 1.25) = 0.8944

* Add the probabilities to find the total probability:

   * P(57 < X < 105) = 0.0062 + 0.8944 = 0.8914

Therefore, the probability that X is between 57 and 105 is 0.8914.

Read more about probability here:

https://brainly.com/question/24756209

#SPJ1

Answer:

114.8

Step-by-step explanation:

The answer is 114.8 because 18% of 140 is 25.2, then if you subtract that by 140 then you will end up with 114.8.

∠1 and ∠2 are right angles because the measure of both angles is 90° and supplementary angles because the sum of both angles is 180°.

A triangle is a polygon that has three sides and three angles and the sum of the angle of the triangle is 180 degrees.

The figure is attached in the picture, please refer to the attached picture.

Given that:

So,

\(\angle2 = 90^\circ\) (vertically opposite angles are equal)

\(\angle1 + \angle2 = 180^\circ\) (Linear Pair)

\(\angle1 = 180 - 90\)

\(\angle1 = 90^\circ\)

\(\angle1 = \angle2 = 90^\circ\)

So, ∠1 and ∠2 are right angles because measure of both angles is 90°.

Adjacent angles because both a common arm and a common vertex.

Thus, ∠1 and ∠2 are right angles because the measure of both angles is 90° and supplementary angles because the sum of both angles is 180°.

To know more about triangles, visit:

https://brainly.com/question/28832368

The Linear function for the line represented by the point-slope equation y - 5 = 3(x - 2) is y = 3x - 1.

The point-slope equation for a line is of the form y - y1 = m(x - x1), where (x1, y1) is a point on the line and m is the slope of the line. Given the point-slope equation y - 5 = 3(x - 2),

we can see that the slope of the line is 3 and it passes through the point (2, 5).

To find the linear function for the line, we need to write the equation in slope-intercept form, which is y = mx + b, where m is the slope of the line and b is the y-intercept (the point at which the line intersects the y-axis).

To get the equation in slope-intercept form, we need to isolate y on one side of the equation.

We can do this by distributing the 3 to the x term:y - 5 = 3(x - 2)  y - 5 = 3x - 6  y = 3x - 6 + 5  y = 3x - 1

Therefore, the linear function for the line represented by the point-slope equation y - 5 = 3(x - 2) is y = 3x - 1.

For more questions on Linear .

https://brainly.com/question/2030026

#SPJ8

Answer:

70.3068

explanation:

divide the mass value by 2.205

Answer:

69.75 kg

Step-by-step explanation:

1:0.45

155:x

x=155×0.45

x=69.75 kg

Answer:

(.5x+10y)²

or  (1/2x+10y)²

Step-by-step explanation:

You should pay approximately $10,117.09 initially to secure the annuity and receive annual payments of $1500 over the 9-year period.

To find the cost of the annuity, we need to calculate the present value of the future payments. The present value represents the current worth of future cash flows, taking into account the interest earned or charged over time. In this case, we'll calculate the present value of the $1500 payments using compound interest.

The formula to calculate the present value of an annuity is:

PV = PMT × [1 - (1 + r)⁻ⁿ] / r

Where:

PV is the present value of the annuity (the amount you should pay initially)

PMT is the payment amount received annually ($1500 in this case)

r is the interest rate per period (6.28% or 0.0628)

n is the total number of periods (9 years)

Let's substitute the values into the formula:

PV = $1500 × [1 - (1 + 0.0628)⁻⁹] / 0.0628

Calculating this expression:

PV = $1500 × [1 - 1.0628⁻⁹] / 0.0628

PV = $1500 × [1 - 0.575255] / 0.0628

PV = $1500 × 0.424745 / 0.0628

PV ≈ $10117.09

To know more about compound interest here

https://brainly.com/question/29335425

#SPJ4

Answer:

4 minutes per lap

Step-by-step explanation:

20/5 is 4

Answer:

1/6

Step-by-step explanation:

There are 6 sides to a die, and 6 occupies one side, so the probability of rolling a 6 is 1/6.

Answer:

1/6 or 16.7%

Step-by-step explanation:

When you roll a standard die, the probability of getting 6 is 1/6 or 16.7%

Answer:

1571

Step-by-step explanation:

its 1570.8 but you have to round

Answer:

2 tons of dirt per hour

Step-by-step explanation:

helpful to know: 1/6 hours = 10 minutes

you can create a proportion to find unit rate by using (tons of dirt ÷ time)

we'll use 'd' to represent amount of dirt and use minutes

1/3÷10 = d/60

1/3÷10 = 1/3 × 1/10 = 1/30

we now have:

1/30 = d/60

we can cross-multiply to get:

30d = 60

d = 2

Answer:

2

Step-by-step explanation:

Given:

A children's pool contains 17,500 millilitres.

To find:

How many litres are in the pool?

Conversion: 1 liter = 1,000 milliliters

Formula:

The formula to convert millilitres to litres is:

1 mL ÷ 1000 = 0.001 L

Calculation:

Now, let's use the above formula:

17,500 millilitres ÷ 1000 = 17.5 liters

Thus, the children's pool contains 17.5 litres.

Answer: 17.5 liters.

Let's break it down step by step.

1 millilitre (ml) is equal to 1/1000th of a litre (L). So, to convert millilitres to litres, you divide the number of millilitres by 1000.

In this case, you have 17,500 millilitres, and you want to find out how many litres that is. So, you divide 17,500 by 1000:

17,500 ml / 1000 = 17.5 L

The result is 17.5 liters. This means that the children's pool contains 17.5 litres of water.

To know more about millilitres,visit:

https://brainly.com/question/696127

#SPJ11

The requried, after 9 days, Plant 2 is 10.09 inches taller than Plant 1.

We can use the equations given to find the heights of Plant 1 and Plant 2 after 9 days and then compare them.

For Plant 1, using the equation h = 2 + 1.06d, we have:

h₁ = 2 + 1.06(9) = 11.54 inches

So after 9 days, Plant 1 is 11.54 inches tall.

For Plant 2, we know that it increased by 2.07 inches every day after reaching a height of 3 inches. So after 9 days, it would have increased by:

2.07 x 9 = 18.63 inches

Starting from a height of 3 inches, the height of Plant 2 after 9 days would be:

h₂ = 3 + 18.63 = 21.63 inches

So after 9 days, Plant 2 is 21.63 inches tall.

Therefore, the difference in height between Plant 1 and Plant 2 after 9 days is:

h₂ - h₁ = 21.63 - 11.54 = 10.09 inches

So after 9 days, Plant 2 is 10.09 inches taller than Plant 1.

Learn more about the equation model here:

https://brainly.com/question/16107051

#SPJ1

By incorporating this information into the similarity measure, we can produce more accurate and informative results in clustering and classification tasks.

To modify the definition of clustering similarity to take into account the position of shared neighbors in the two nearest neighbor lists, we can introduce a weighting factor that considers the rank of the shared neighbor in each list. This can be achieved by multiplying the regular SNN similarity value by a weight factor that is calculated as the reciprocal of the sum of the ranks of the shared neighbors in the two lists. For example, if two points have a shared neighbor that is ranked 2nd in one list and 3rd in the other list, the weight factor would be 1/(2+3) = 0.2. This weight factor would then be multiplied by the regular SNN similarity value to produce a modified SNN similarity score that gives higher similarity to points that share the same nearest neighbors ranked higher in the nearest neighbor lists. This modification is justified because it takes into account the fact that having shared neighbors that are ranked higher in the nearest neighbor lists is a stronger indicator of similarity than having shared neighbors that are ranked lower.

Learn more about clustering here

https://brainly.com/question/29888905

#SPJ11

Answer:

{x | x < 2 and  x < -4 }

Step-by-step explanation:

Given the inequality 5(x - 2)(x + 4) > 0?

This also means x - 2 < 0 and x + 4<0

For x - 2 < 0

Add 2 to both sides

x - 2 + 2 < 0 + 2

x < 2

Similarlry for x + 4 <0

Subtract 4 from both sides

x+4-4 < 0 -4

x < 0 -4

x < -4

x < 2 and  x < -4

Hence the required solution is {x | x < 2 and  x < -4 }