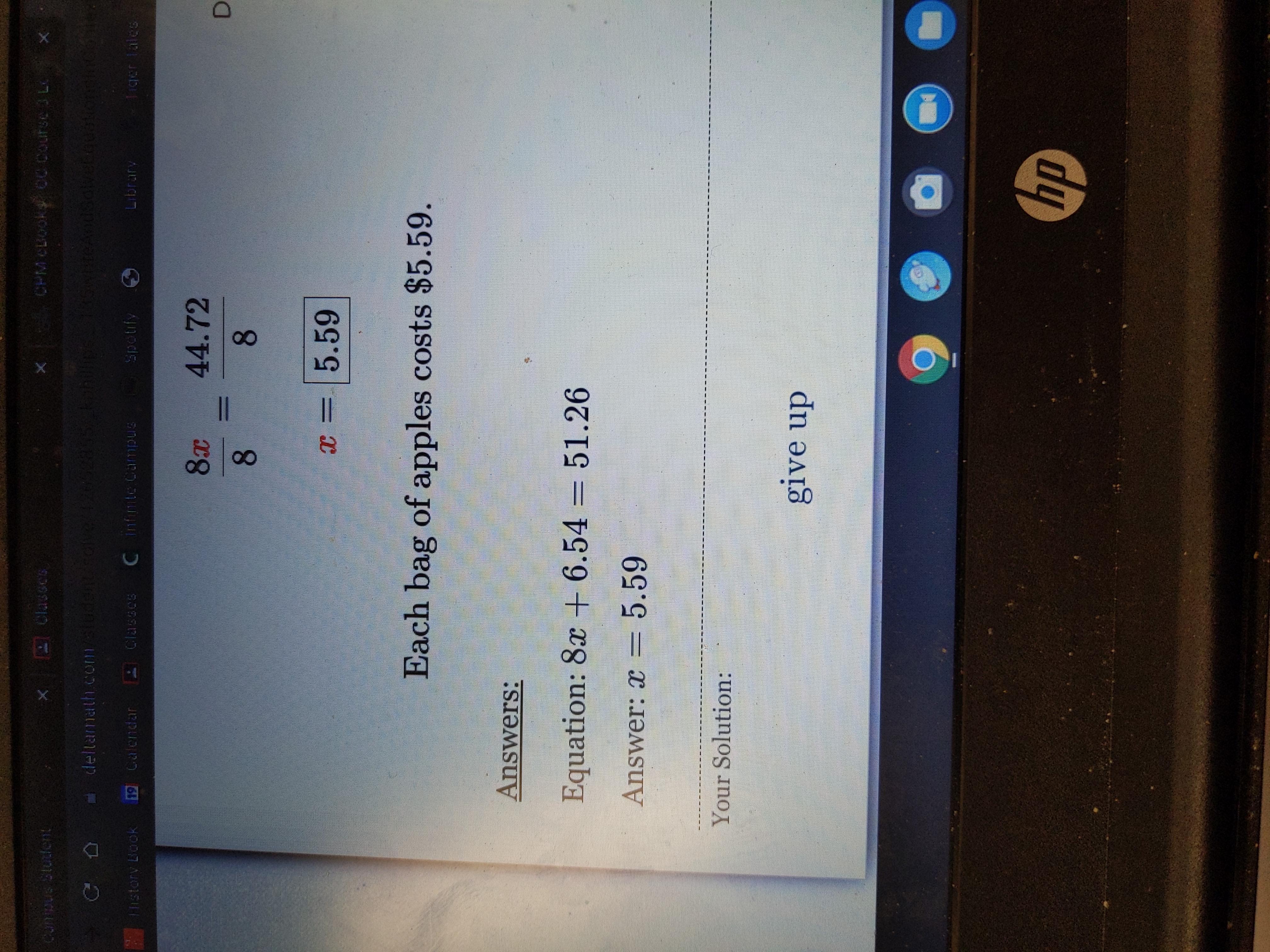
Peyton buys cookies and apples at the store.

She pays a total of $51.26.

She pays a total of $6.54 for the cookies.

She buys 8 bags of apples that each cost the same amount.


Write and solve an equation which can be used to determine

x

x, how much each bag of apples costs.

I need an answer please

Answer:

3 it 3 you got to haryy up it 3

Answer: number  2

Step-by-step explanation:

Answer:

Step-by-step explanation:

25ft x 25ft

Maximum Area with Fixed Perimeter

In order to have a perimeter of 100 feet, that means that each side needs to be 25 feet long. The area would then be 25ft x 25ft, or 625ft2.

Problem 2, Part (a)

A = event elevator's 1st stop at the 2nd floor

P(A) = 3/4

Since the elevator needs to go up one floor

B = event elevator has its 2nd stop at the 3rd floor

P(B given A) = probability event B happens given event A happened

P(B given A) = 3/4

-----------

P(A and B) = P(A)*P(B given A)

P(A and B) = (3/4)*(3/4)

P(A and B) = 9/16

-----------

===============================================================

Problem 2, Part (b)

A = event elevator's 1st stop at the 2nd floor

P(A) = 3/4

B = event elevator has its 2nd stop at the 3rd floor

P(B given A) = 3/4

C = event elevator has its 3rd stop at 4th floor

P(C given (A and B)) = 3/4

-----------

P(A & B & C) = P(A)*P(B given A)*P(C given (A and B))

P(A & B & C) = (3/4)*(3/4)*(3/4)

P(A & B & C) = 27/64

-----------

===============================================================

Problem 2, Part (c)

A = event elevator's 1st stop at the 2nd floor

x = P(A) = 3/4

B = event elevator has its 2nd stop at the 3rd floor

y = P(B given A) = 3/4

C = event elevator has its 3rd stop at 2nd floor

z = P(C given (A and B)) = 1/4

D = event elevator has its 4th stop at 1st floor

w = P(D given (A & B & C)) = 1/4

-----------

P(A & B & C & D) = x*y*z*w

P(A & B & C & D) = (3/4)*(3/4)*(1/4)*(1/4)

P(A & B & C & D) = 9/256

-----------

Answer:

B

Step-by-step explanation:

I'm sorry if I'm wrong

Answer:

5

Step-by-step explanation:

meant b haha

The statement that is true is Airplane B has a lesser median. Option C

When calculating the median, we find the middle value for the data set of both planes

Airplane A  data set are 200, 205, 210, 215, 220, 225, 230, 234

Median = (215 + 220)/2 = 217.5

Airplane B's data set includes  180, 185, 190, 195, 200, 205, 210, 215, 220, 225, 230, 235

Median = (205 + 210)/2 = 207.5

Therefore airplane B has a lesser median.

Find more exercises on median;

https://brainly.com/question/300591

#SPJ1

The slope-intercept form of the equation of a line is: y = mx + b

Where: y is the dependent variable (usually represented on the vertical axis of a graph). x is the independent variable (usually represented on the horizontal axis of a graph). m is the slope of the line (which represents the rate of change of y with respect to x). b is the y-intercept (which represents the value of y when x is equal to 0). To use this equation, you need to know the slope (m) and the y-intercept (b) of the line. You can find the slope of a line by taking any two points on the line and using the formula:

m = (y2 - y1) / (x2 - x1)

where (x1, y1) and (x2, y2) are any two points on the line. Once you know the slope, you can substitute it and the y-intercept into the slope-intercept form of the equation of the line to get the equation in its final form.

To learn more about slope-intercept click here

brainly.com/question/30216543

#SPJ4

Answer:

1) 100 degrees F

2) 50 degrees F

Step-by-step explanation:

its just simple subtraction

Answer:

The answer is x=1 and y=1

Step-by-step explanation:

Multiply the second equation by -1, then add the equations together.

(2x+3y=5)

−1(2x+7y=9)

Becomes:

2x+3y=5

−2x−7y=−9

Add these equations to eliminate x:

−4y=−4

Then solve−4y=−4for y:

−4y=−4 Divide -4

\(\frac{-4y}{-4}= \frac{-4}{-4}\)

y=1

Now that we've found y let's plug it back in to solve for x.

Here's was the original equation:

2x+3y=5

Substitute 1 for y in 2x+3y=5:

2x+(3)(1)=5

2x+3=5

2x+3+−3=5+−3 ( Add -3 on both sides)

2x=2 Divide by 2

\(\frac{2x}{2} =\frac{2}{2}\)

x=1

Given:

The given endpoints are ( -2,10) and (-10, -12).

Required:

We need to find the midpoint of te given endpoints.

Explanation:

Consider the formua to find the midpoint.

Consider the points ( -2,10) and (-10, -12).

Final answer:

Answer:

The original price was $864

Step-by-step explanation:

if the ring is now $840 $40 u do $40 - %40 = %24

$24 + $840 = $864

Answer:

k = - 20

Step-by-step explanation:

(x - h) is a factor of f(x), then by the remainder theorem f(a) = 0 , then

substituting x = 2 into f(x) and equating to zero, gives

3(2)³ - 2(2) + k = 0

3(8) - 4 + k = 0

24 - 4 + k = 0

20 + k = 0 ( subtract 20 from both sides )

k = - 20

The strategy for generating a hypothesis that does not fit among the options provided is option C) Thinking of things unilaterally.

Introspection, finding exceptions to the rule, and thinking about variables in terms of amount or degrees are all valid strategies for generating hypotheses.

Introspection involves reflecting on personal experiences, thoughts, and observations to generate hypotheses about a particular phenomenon or question.

Finding exceptions to the rule involves identifying instances that do not conform to the expected pattern or generalization, which can lead to the formulation of alternative hypotheses.

Thinking about variables in terms of amount or degrees involves considering how varying levels or quantities of a particular variable may impact the outcome or relationship being studied, which can help generate hypotheses about the nature and direction of the relationship.

On the other hand, "thinking of things unilaterally" is not a recognized strategy for generating hypotheses. The term "unilaterally" typically refers to actions or decisions made by one side or party without considering others.

Hypothesis generation involves considering multiple perspectives, factors, and possibilities, rather than approaching it unilaterally.The correct answer is option c.

Know more about  Introspection   here:

https://brainly.com/question/30784412

#SPJ11

2.4 hours

Ten percent is ten per every hundred, that is ten percent is Thus ten percent of 24 hours is 0.10 \times 24 = 2.4 hours.

Answer:

2.4 hours

Step-by-step explanation:

24÷10=2.4

To test whether the vector field F(x, y) = (3x² + 3y)i + (3x + 2y)j is conservative, we can apply the scalar curl test.

The scalar curl of a vector field F(x, y) = P(x, y)i + Q(x, y)j is defined as the partial derivative of Q with respect to x minus the partial derivative of P with respect to y:

curl(F) = ∂Q/∂x - ∂P/∂y

For the given vector field F(x, y) = (3x² + 3y)i + (3x + 2y)j, we have:

P(x, y) = 3x² + 3y

Q(x, y) = 3x + 2y

Now, let's calculate the partial derivatives:

∂Q/∂x = 3

∂P/∂y = 3

Therefore, the scalar curl of F is:

curl(F) = ∂Q/∂x - ∂P/∂y = 3 - 3 = 0

Since the scalar curl is zero, we conclude that the vector field F is conservative.

To compute the line integral ∮C F · dr, where C is the curve given by y = sin(x) starting at (0, 0) and ending at (2πt, 0), we can use the Fundamental Theorem of Calculus for line integrals.

The Fundamental Theorem of Calculus states that if F(x, y) = ∇f(x, y), where f(x, y) is a potential function, then the line integral ∮C F · dr is equal to the difference in the values of f evaluated at the endpoints of the curve C.

Since we have established that F is a conservative vector field, we can find a potential function f(x, y) such that ∇f(x, y) = F(x, y). In this case, we can integrate each component of F to find the potential function:

f(x, y) = ∫(3x² + 3y) dx = x³ + 3xy + g(y)

Taking the partial derivative of f(x, y) with respect to y, we obtain:

∂f/∂y = 3x + g'(y)

Comparing this with the y-component of F, which is 3x + 2y, we can see that g'(y) = 2y. Integrating g'(y), we find g(y) = y².

Therefore, the potential function is:

f(x, y) = x³ + 3xy + y²

Now, we can compute the line integral using the Fundamental Theorem of Calculus:

∮C F · dr = f(2πt, 0) - f(0, 0)

Plugging in the values, we have:

∮C F · dr = (2πt)³ + 3(2πt)(0) + (0)² - (0)³ - 3(0)(0) - (0)²

= (2πt)³

Thus, the line integral ∮C F · dr is equal to (2πt)³.

Learn more about curl of vector here:

https://brainly.com/question/31981036

#SPJ11

Answer:

no

Step-by-step explanation:

hey

The objective function formula that is nonlinear is - 2xy/2xy + z

From the question, we have the following parameters that can be used in our computation:

- 2xy/2xy + z

- x + y + z

- 3x - 2y + z

A linear function is a function that has a degree of 1

Other functions with other degrees are nonlinear

Using the above as a guide, we have the following functions with a degree of 1

- x + y + z

- 3x - 2y + z

Hence, the objective function formulas that is nonlinear is - 2xy/2xy + z

Read more about linear equations at

https://brainly.com/question/2030026

#SPJ1

Answer:

V≈18.63m³

Step-by-step explanation:

V=ABh

3=6.5·8.6

3≈18.63333m³

To compute the inverse Laplace transform of L^-1 {3s + 2/s^2 - s - 12 e^-4s}, we first need to break it up into simpler terms using partial fraction decomposition. We have:

L^-1 {3s + 2/s^2 - s - 12 e^-4s}

= L^-1 {3s} + L^-1 {2/s^2 - s} + L^-1 {12 e^-4s}

= 3 δ(t) + (2 u(t) - 1) - (1 - u(t - 4)) \* 3/2 e^(4(t-4))

where δ(t) is the Dirac delta function and u(t) is the Heaviside step function.

The first term, 3 δ(t), comes from the L^-1 {3s} term, which corresponds to a constant function.

The second term, (2 u(t) - 1), comes from the L^-1 {2/s^2 - s} term, which we can decompose as:

2/s^2 - s

= 2/s^2 - 2s/s^2 + s/s^2

= 2 (1/s - 1/s^2) - s/s^2

Taking the inverse Laplace transform of each term separately gives:

L^-1 {2 (1/s - 1/s^2)} = 2 (u(t) - 1) L^-1 {-s/s^2} = -(t u(t))

Putting these together, we get: L^-1 {2/s^2 - s} = 2 (u(t) - 1) - (t u(t))

The third term, (1 - u(t - 4)) \* 3/2 e^(4(t-4)), comes from the L^-1 {12 e^-4s} term, which corresponds to an exponentially decaying function.

We use the time-shifting property of the Laplace transform to shift the function by 4 units to the right, giving: L^-1 {12 e^-4s} = 3/2 e^(4(t-4)) u(t-4)

But we want the function to be 0 for t < 4, so we subtract off the Heaviside step function u(t - 4), giving: L^-1 {12 e^-4s} = (1 - u(t - 4)) \* 3/2 e^(4(t-4))

Putting everything together, we get: L^-1 {3s + 2/s^2 - s - 12 e^-4s} = 3 δ(t) + 2 (u(t) - 1) - (t u(t)) - (1 - u(t - 4)) \* 3/2 e^(4(t-4))

Know more about inverse Laplace transform,

https://brainly.com/question/30358120

#SPJ11

The solution to the inequality is y ≤ -8. This means that any value of y that is less than or equal to -8 will satisfy the original inequality.

To solve the inequality y + 7 ≤ -1, we need to isolate the variable y on one side of the inequality sign.

Starting with the given inequality:

y + 7 ≤ -1

We can begin by subtracting 7 from both sides of the inequality:

y + 7 - 7 ≤ -1 - 7

y ≤ -8

The solution to the inequality is y ≤ -8. This means that any value of y that is less than or equal to -8 will satisfy the original inequality.

In the context of a number line, all values to the left of -8, including -8 itself, will make the inequality true. For example, -10, -9, -8, -8.5, and any other value less than -8 will satisfy the inequality. However, any value greater than -8 will not satisfy the inequality.

For such more question on solution:

https://brainly.com/question/24644930

#SPJ8

The following question may be like this:

What is a solution of the inequality shown below? y+7≤-1

Answer:

The function may be rewritten in slope-intercept form y=7x-4, where slope is 7 and y-intercept is -4.  Starting at the y-intercept, (0,-4), y increases by 7 as x increases by 1, so the next point you plot will be (1, 3).  If you want to plot a point to the left of the y-axis, just decrease y by 7 as you decrease x by 1, so the next point would be (-1, -11).  Draw a line through the 3 plotted points.  The line will slope upward sharply from left to right.

Alternately, you could just pick several values for x and plug them into the given equation to solve for y and then plot those points.

Step-by-step explanation:

2170 toys Jamal assemble altogether during his first 20 days.

What is the arithmetic sequence?

A series of numbers called an arithmetic progression or arithmetic sequence has a constant difference between the terms.

Here, we have

Given: Jamal got a job working on an assembly line in a toy factory. On the 20th day of work, he assembled 137 toys. He noticed that since he started, every day he assembled 3 more toys than the day before.

We have to find how many toys did Jamal assemble altogether during his first 20 days.

We apply the arithmetic sequence formula and we get

tₙ = t₁ + (n-1)d

137 = t₁ + (20-1)(3)

t₁ = 80

To calculate the total number of toys that Jamal assemble in his first 20 days,

S₂₀ = (20/2)(2×80 + (20-1)(3))

S₂₀ = 2170

Hence, 2170 toys Jamal assemble altogether during his first 20 days.

To learn more about the arithmetic sequence from the given link

https://brainly.com/question/6561461

#SPJ1

Answer:

Step-by-step explanation:

5 : 25

In simplest form

1 : 25

In fraction form

1/25

In decimal form

0.04

150 : 300

In simplest form

1 : 2

In fraction form

1/2

In decimal form

0.5

Answer:

8

Step by step :

0.6x = 0.8x-1.1-0.5

0.6x-0.8x = -1.6

-0.2x = -1.6

x = -1.6/-0.2

x = 8

Answer:

0.5 3/5 5/8 65%

Step-by-step explanation:

Answer:

Your answer is 0.5, 3/5, 5/8, 65%.

Step-by-step explanation:

Convert all numbers to decimals:

0.5 = 0.5

5/8 = 0.625

65% = 0.65

3/5 = 0.6

Arrange the numbers accordingly: 0.5, 0.6, 0.625, 0.65. Hence, your answer is 0.5, 3/5, 5/8, 65%.

Answer:

98.

Step-by-step explanation:

98 degrees because 3 and 6 are interior alternate angles.

Answer:

∠ 3 = 98°

Step-by-step explanation:

∠ 6 and ∠ 3 are alternate angles and are congruent, thus

∠ 3 = ∠ 6 = 98°

For the whole numbers:

24

26

23

25

21

46

63

57

75

91

1. 24 and 23.8

2. 26 and 25.7

3. 23 and 22.8

4. 25 and 24.8

5. 21 and 20.9

6. 46 and 46.3

7. 64 and 63.7

8. 56 and 56.5

9. 75 and 74.6

10. 91 and 90.7

When rounding, whether it's a whole number or a decimal, you would round up if the number next to it is 5 and up. You wouldn't change the number next to it if it's below 5.

If you have any further question, please reach out to me :)

Answer:

Your answer is 0.300 rounded to the thousands place.

Step-by-step explanation:

Hope this helps!

Answer:

Assuming that Jerry is at ground level and Tom is sitting on a table 41 feet away from Jerry, we can use trigonometry to find the angle of elevation that the board would make with the floor.

Let's call the height that the board reaches on the table "h". From the information given, we know that the distance from Jerry to the point where the board touches the table is 57 feet (the length of the board), and the distance from that point to Tom is 41 feet. We can set up a right triangle with these distances as the legs and "h" as the hypotenuse, as shown in the diagram below:

         |\

         | \

        h|  \ 41 ft

         |   \

         |____\

          57 ft

Using the Pythagorean theorem, we can find the value of "h":

h^2 = 57^2 + 41^2

h^2 = 3241 + 1681

h^2 = 4922

h = sqrt(4922)

h ≈ 70.1 ft

Now we can use trigonometry to find the angle of elevation of the board. We want to find the angle whose tangent is equal to the opposite side (h) divided by the adjacent side (57 ft):

tan(theta) = h / 57

theta = arctan(h / 57)

theta ≈ arctan(70.1 / 57)

theta ≈ 51.1 degrees

Therefore, the angle of elevation that the board would make with the floor is approximately 51.1 degrees.

Answer:

(−2)×5<(−20)

Step-by-step explanation:

Evaluating the options given :

(−2)×5<(−20)

Open the bracket

- 10 < - 20 (false) ` This expression isn't true

(−2)×(−5)>(−25)

Open the bracket

10 > - 25 (true)

2×5>(−25)

Open the bracket

10 > - 25 (true)

2×(−5)<20

Open the bracket

- 10 < 20

Answer:

I believe it's 280.

Sorry if that was wrong.