p(Febuary 31) Calculate the probability of the event
Answers
Answer:
You can't find the probibilty of the event because it doesn't exist
Step-by-step explanation:
Related Questions
What is the slope of the line described by the equartion below?
y = -2x + 4
I need help.
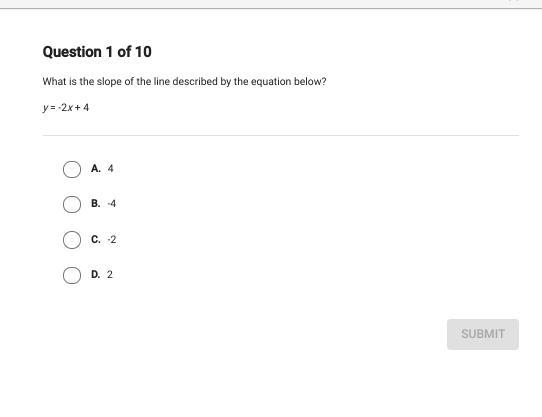
Answers
Answer:
C. –2
Step-by-step explanation:
The slope is equal to the number multiplying the x
Answer:
C
Step-by-step explanation:
Compare the equation to the line intercept form of a line, y=mx+c. m here represents the slope of the line. So m=-2
What I s the sum of the measures of the interior angles of a 13-sided polygon?
Answers
Answer:
What is the sum of the measures of the interior anles of a convex 13-gon? There is a formula... the sum of the measures of the interior angles of a convex n-gon is (n-2)180 degrees. For a triangle, n=3, so it's (1)180=180 degrees.
Step-by-step explanation:
180(n-2)
n=number of sides
from question, number of sides=13
substitute 13 into the equation given above
180(13-2)
180(11)
1980 is the answer
if we write $\sqrt{5} \frac{1}{\sqrt{5}} \sqrt{7} \frac{1}{\sqrt{7}}$ in the form $\dfrac{a\sqrt{5} b\sqrt{7}}{c}$ such that $a$, $b$, and $c$ are positive integers and $c$ is as small as possible, then what is $a b c$?
Answers
We can rewrite the given expression as follows:
$\sqrt{5} \frac{1}{\sqrt{5}} \sqrt{7} \frac{1}{\sqrt{7}} = \frac{\sqrt{5}}{\sqrt{5}} \cdot \frac{\sqrt{7}}{\sqrt{7}} = \frac{5}{\sqrt{5}\sqrt{5}} \cdot \frac{7}{\sqrt{7}\sqrt{7}}$
Now, we simplify further:
$= \frac{5}{5} \cdot \frac{7}{7} = \frac{1 \cdot 1}{1}$
So, in the form $\dfrac{a\sqrt{5} b\sqrt{7}}{c}$, we have $a = 0$, $b = 0$, and $c = 1$. Therefore, the product $abc = 0 \cdot 0 \cdot 1 = 0$.
First, let's simplify the expression:
$\sqrt{5} \frac{1}{\sqrt{5}} \sqrt{7} \frac{1}{\sqrt{7}} = \frac{\sqrt{5}}{\sqrt{5}} \cdot \frac{\sqrt{7}}{\sqrt{7}} = 1$
So now we want to write $1$ in the form $\dfrac{a\sqrt{5} b\sqrt{7}}{c}$ with positive integers $a$, $b$, and $c$, and $c$ as small as possible.
We can do this by setting $a=b=c=1$ since $1\cdot 1\cdot 1 = 1$ and $1$ is the smallest possible value for $c$. So we have:
$1 = \dfrac{1\sqrt{5}\cdot 1\sqrt{7}}{1}$
Therefore, $a=1$, $b=1$, and $c=1$, so $abc=1$.
Learn more about Expression:
brainly.com/question/14083225
#SPJ11
Which one(s) of the following statements is (are) correct for a uniformly distributed random variable? A. The expected value of the random variable is equal to 1. B. The probability distribution function is constant over the definition space. C. The probability density function is constant over the definition space. D. The probability density functions take on only negative numbers E. None of the above.
Answers
For a uniformly distributed random variable, the correct statement is B: The probability distribution function is constant over the definition space.
A uniformly distributed random variable has a constant probability distribution function (PDF) over its definition space. This means that the probability of obtaining any value within the range of the variable is equal.
Statement A is incorrect because the expected value of a uniformly distributed random variable is not necessarily equal to 1. The expected value is calculated by taking the average of all possible values, and for a uniform distribution, it is equal to the average of the minimum and maximum values in the distribution.
Statement C is also incorrect because a uniformly distributed random variable does not have a probability density function (PDF) since it is a discrete distribution. Instead, it has a probability mass function (PMF), which assigns equal probabilities to each value in its definition space.
Statement D is incorrect because the probability density function (PDF) or probability mass function (PMF) of a uniformly distributed random variable takes on non-negative values since probabilities must be non-negative.
Therefore, the correct statement is B: The probability distribution function is constant over the definition space.
Learn more about probability distribution function here:
https://brainly.com/question/32099581
#SPJ11
Jordan is using a number line to model the division expression Negative 24 divided by 12. What should be a step in his work?
Answers
Answer:
-24/12= -2
Step-by-step explanation:
Answer:
Its B
Step-by-step explanation:
If I'm wrong please tell me
What is the solution set to the following system?
x+y=3
(x² + y² = 9
Answers
Answer:
The solutions are 0 and 3
Step-by-step explanation:
x = 3-y
((3-y)^2+y^2)=9
=(y^2-6y+9)+y^2) = 9
= 2y^2 - 6y +9 -9 =0
=2y^2 -6y=0
= y*(2y -6) = 0
y1=0 y2=3
The solution of the system x + y = 3 and x² + y² = 9 is y = 0 and y = 3.
What is equation?A formula known as an equation uses the equals sign to denote the equality of two expressions.
Given:
x + y = 3
x² + y² = 9
Solve the equation as shown below,
x = 3 - y
Put this value of x in the second equation,
(3 - y)² + y² = 9
y² + 9 - 6y + y² = 9
2y² - 6y = 0
Solve the quadratic equation,
2y (y - 3)
y = 0 and y = 3
To know more about equation:
https://brainly.com/question/12788590
#SPJ2
In 2009, the average compensation for CEOs in the U.S. was approximately $10,800,000, and by 2016, this had risen to about $12,800,000. By comparison, the average compensation for workers was $54,700 in 2009 and $55,800 in 2016. Assume that both values are growing exponentially. Find the growth rate r for both salaries. State your answers as percentages rounded to the nearest tenth of a percent.For CEOs: r= %For workers: r = %
Answers
The exponential growth formula is given by
\(C=a(1+r)^t\)where C is the average compensation, a is the initial amount and t is the time (in years).
For the CEO's case, we have that
\(\begin{gathered} \text{for t=2009, C=10800000} \\ \text{for t=2016, C=12800000} \end{gathered}\)Then, we can find a and r with these values. By substituting the first values, we have
\(10800000=a(1+r)^{2009}\)By substituting the second values, we get
\(128000000=a(1+r)^{2016}\)By isolating a in both case, we have that
\(\begin{gathered} a=\frac{10800000}{(1+r)^{2009}} \\ \text{and} \\ a=\frac{12800000}{(1+r)^{2016}} \end{gathered}\)Then, we can state the following equation:
\(\frac{10800000}{(1+r)^{2009}}=\frac{12800000}{(1+r)^{2016}}\)or equivalently,
\(\begin{gathered} \frac{(1+r)^{2016}}{(1+r)^{2009}}=\frac{128000000}{108000000} \\ \frac{(1+r)^{2016}}{(1+r)^{2009}}=1.185185 \end{gathered}\)By applying natural logarithm in bot sides, we have
\(\begin{gathered} \ln (\frac{(1+r)^{2016}}{(1+r)^{2009}})=\ln 1.185185 \\ \ln (\frac{(1+r)^{2016}}{(1+r)^{2009}})=0.169899 \end{gathered}\)By the properties of logarithms:
\(\ln (\frac{A}{B})=\ln A-\ln B\)we obtain
\(\ln (1+r)^{2016}-\ln (1+r)^{2009}=0.169899\ldots.(a)\)By the property of logaritm:
\(\ln A^x=x\ln A\)The last result is equivalent to
\(\begin{gathered} 2016\ln (1+r)-2009\ln (1+r)=0.169899 \\ which\text{ gives} \\ 7\ln (1+r)=0.169899 \end{gathered}\)then, by moving the number 7 to the right hand side, this yields,
\(\begin{gathered} \ln (1+r)=\frac{0.169899}{7}\ldots(b) \\ \ln (1+r)=0.024271 \end{gathered}\)Now, by applying the exponential function in both sides, we have
\(\begin{gathered} 1+r=e^{0.024271} \\ 1+r=1.024568 \end{gathered}\)then, r is given by
\(\begin{gathered} r=1.024568-1 \\ r=0.0245 \end{gathered}\)Now, by converting this result into percent form. The answer for the CEO's case is
\(r=2.45\text{ \%}\)Now, for the workers case, we can do the same procedure and get an equation similar to equation (a). That is,
\(\begin{gathered} \ln (1+r)^{2016}-\ln (1+r)^{2009}=\ln (\frac{55800}{54700}) \\ \ln (1+r)^{2016}-\ln (1+r)^{2009}=\ln (1.020109) \\ \ln (1+r)^{2016}-\ln (1+r)^{2009}=0.0199 \end{gathered}\)and find somthing similar to equation (b)
\(\begin{gathered} \ln (1+r)=\frac{0.0199}{7}\ldots \\ \ln (1+r)=0.0028 \end{gathered}\)and finally,
\(\begin{gathered} 1+r=e^{0.0028} \\ 1+r=1.0028 \end{gathered}\)then, r will be
\(\begin{gathered} r=1.0028-1 \\ r=0.0028 \\ In\text{ percent form is} \\ r=\text{ 0.28 \%} \end{gathered}\)In summary,by rounding the answers to the nearest tenth percent, the answers are
\(\begin{gathered} \text{For CEO's:} \\ r=2.5\text{ \%} \\ \text{For workers:} \\ r=0.3\text{ \%} \end{gathered}\)3. Milo is displaying 20 framed photos on shelves. He wants the same number of photos on each shelf. He has up to 4 shelves to use. How can he arrange the photos?
Answers
Answer:
milo can put 5 framed photos on each shelf
Step-by-step explanation:
4÷20=5
Can anyone help with this?? (algerbra)

Answers
(x-2)(x+21)
Which would be the third option on your screen. Hope this helped!
A five-year $7,200 promissory note bearing interst at 6% compounded monthly (j12) was sold after two years and three months. Calculate the sale price using a discount rate of 10% compounded quarterly (j4). Round your answer to 2 decimal places.
Answers
The sale price of the promissory note is approximately $5,354.29.
To calculate the sale price, we need to determine the present value of the remaining payments on the promissory note using the given discount rate of 10% compounded quarterly. The remaining term of the promissory note is 5 years - 2 years 3 months = 2 years 9 months = 2.75 years.
Using the formula for present value, we can calculate the sale price as follows:
Sale Price = Remaining Payments / (1 + Discount Rate/Number of Compounding Periods)^(Number of Compounding Periods * Remaining Time)
Remaining Payments = $7,200 (the face value of the promissory note)
Discount Rate = 10% / 4 = 0.025 (quarterly rate)
Number of Compounding Periods = 4 (quarterly compounding)
Remaining Time = 2.75 years
Plugging in the values, we have:
Sale Price = $7,200 / (1 + 0.025)^(4 * 2.75)
= $7,200 / (1.025)^11
≈ $5,354.29
Learn more about present value here:
https://brainly.com/question/28304447
#SPJ11
Solve the equation. 6x−7=2x+13
Answers
Answer:
6x - 7 = 2x + 13
6x - 2x = 13 + 7
4x = 20
x = 20/4
x = 5
Hope it helps!
An herbal medicine brand contains amounts of the active ingredient (in milligrams, mg) that vary normally from capsule to capsule. A quality control engineer assesses whether capsules of this brand contain different amounts, on average, than the marketed amount of 10 mg. A random sample of 30 capsules finds a mean content of 9.25 mg per capsule. Assume that active ingredient for this medicine brand is approximately Normal with a standard deviation of 0.65mg significane level.
Required:
Which are the appropriate null and alternative hypotheses?
Answers
Null hypothesis assumes there is no significant difference between mean amount of active ingredient in capsules and marketed amount of 10 mg.
Alternative hypothesis there is a significant difference, either higher or lower, between the mean amount and the marketed amount.
The appropriate null and alternative hypotheses for assessing whether the capsules of the herbal medicine brand contain different amounts,
on average, than the marketed amount of 10 mg are as follows,
Null Hypothesis H₀,
The mean content of the capsules is equal to the marketed amount of 10 mg.
Alternative Hypothesis Hₐ,
The mean content of the capsules is different from the marketed amount of 10 mg.
Symbolically, the hypotheses can be written as
H₀: μ = 10
Hₐ: μ ≠ 10
Therefore, the null hypothesis states that the mean content of the capsules is equal to 10 mg,
while the alternative hypothesis states that the mean content of the capsules is not equal to 10 mg.
learn more about null hypothesis here
brainly.com/question/31691895
#SPJ4
To get the total number of iterations in a nested loop, add the number of iterations in the inner loop to the number of iterations in the outer loop.
True
False
Answers
It is False that by adding the number of inner loops , we get the total number of iterations.
A loop is defined as a segment of code that executes multiple times. Iteration refers to the process in which the code segment is executed once. One iteration refers to 1-time execution of a loop. A loop can undergo many iterations.
There are 3 types of iteration: tail-recursion, while loops, and for loops. We will use the task of reversing a list as an example to illustrate how different forms of iteration are related to each other and to recursion. A recursive implementation of reverse is given below.
To learn more about iteration of loops
brainly.com/question/14266710
#SPJ4
whats 3/5 in simplest form
Answers
Answer: 3/5 is the simplest form that can be made of that fraction. If you happened to need it as a decimal it is 0.6
Answer:
its 3/5 because it can't be any smaller
32) Convert this fraction to a decimal number: 4/10
Answers
Answer:
40%
Step-by-step explanation:
Hope this helps
Have a great day/night
100000+1X2 222+222+22222222222x111111111111111111111+222222222222222222
Answers
So, 100000+1X2 + 222+222+22222222222x111111111111111111111+222222222222222222 = 24,691,458,024,691,358,024,691,826
What does an expression mean?
It is preferable to use moving integer variables, which can be rising, decreasing, or blocking, rather than estimates that are generated at random. They could only assist one another by exchanging resources, knowledge, or answers to problems. A declaration of truth equation may include the justifications, components, and mathematical comments for strategies like extra disapproval, production, and mixture.
Let's solve this equation step by step:
100000+1X2
This is a simple expression which involves addition and multiplication.
100000+1X2 = 100000 + 2 (since 1X2 equals 2)
Therefore, 100000+1X2 = 100002
222+222+22222222222x111111111111111111111
This expression involves addition and multiplication as well.
We need to multiply 22222222222 by 111111111111111111111 first, and then add 222+222 to the result.
22222222222 x 111111111111111111111 = 24,691,358,024,691,358,024,691,358
222+222 = 444
So, 222+222+22222222222x111111111111111111111 = 24,691,358,024,691,358,024,691,802
222222222222222222
This is a simple number.
So, 100000+1X2 + 222+222+22222222222x111111111111111111111+222222222222222222 = 24,691,458,024,691,358,024,691,826
To know more about expressions visit :-
brainly.com/question/14083225
#SPJ1
give me the answer pleaseeeeeeeeeeeeeeerreeeeeeee

Answers
PARTY PLANNING The cost of decorations for a party is $32. This is split among a
group of friends. The amount each person pays y is given by y =32 over x, where x is the
number of people. Graph the function.
Help me please
Answers
Answer:
the function would be y = 32/x
Step-by-step explanation:
In a poll of 200 randomly selected U.S. adults, 104 said they favored a new proposition. Based on this poll, compute a 90% confidence interval for the proportion of all U.S. adults in favor of the proposition (at the time of the poll). Then complete the table below. Carry your intermediate computations to at least three decimal places. Round your answers to two decimal places. lower limit of 90%? upper limit of 90%
Answers
The lower limit of the 90% confidence interval is 0.429 when in a survey of 200 randomly chosen American adults, 104 responded in favor of the proposed proposal.
Given that
In a survey of 200 randomly chosen American adults, 104 responded in favor of the proposed proposal. Calculate a 90% confidence interval for the percentage of all U.S. adults who support the proposal based on the results of this poll (at the time of the poll). Complete the table below after that. Carry your calculations to a minimum of three decimal places.
We have to find the lower limit of 90%.
We know that
In a poll of 200 randomly selected U.S. Among adults, 104 expressed support for the novel idea.
p-hat = 104/200 = 0.52
ME = z×√[pq/n] = 2.5758×√[0.52*0.48/200] = 0.091
Now, the lower limit of the 90% confidence interval well be
p-hat-ME = 0.52-0.091 = 0.429
Therefore, The lower limit of the 90% confidence interval is 0.429 when in a survey of 200 randomly chosen American adults, 104 responded in favor of the proposed proposal.
To learn more about limit visit: https://brainly.com/question/29394831
#SPJ4
If A (2, 3) maps to A' (4, 6) under a certain enlargement, find the center and scale
factor of enlargement.full step pls
Answers
Answer:
The image A'(4, 6) is the result of dilation of the original point A(2, 3) by a scale factor 2 with a center at the origin.
Therefore, we conclude that If A (2, 3) maps to A' (4, 6) under a certain enlargement:
The center = (0, 0)Scale factor = 2Step-by-step explanation:
We know that when any object is dilated by a certain scale factor, it gets reduced or enlarged. The reduction or enlargement depends upon the scale factor.
If the scale factor > 1, the image is an enlargement.
If the scale factor < 1, the image is reduced.
For example, if a point P(x, y) is dilated by a scale factor of 2 with a center at the origin, the image P' will have the coordinates (2x, 2y).
As scale factor 2 > 1, so the image P' is enlarged.
Given the point
A(2, 3)
Rule of dilation by a scale factor 2 with a center at the origin
P(x, y) → P'(2x, 2y)
A(2, 3) → A'(2(2), 2(3)) = A'(4, 6)
It is clear that image A' has the coordinates (4, 6). i.e. A'(4, 6).
In other words, the image A'(4, 6) is the result of dilation of the original point A(2, 3) by a scale factor 2 with a center at the origin.
Therefore, we conclude that If A (2, 3) maps to A' (4, 6) under a certain enlargement:
The center = (0, 0)Scale factor = 2How to round a mixed number to the nearest half
Answers
Given the following set of ordered pairs, determine if it is direct or inverse variation and find the value of k. (2, -10) (4, -20) (7, -35) (8, -40)
Answers
Answer:
direct variation
Step-by-step explanation:
The constant of variation k for direct variation is
k = \(\frac{y}{x}\)
Calculate this ratio for each set of points
\(\frac{-10}{2}\) = \(\frac{-20}{4}\) = \(\frac{-35}{7}\) = \(\frac{-40}{8}\) = - 5
Then ordered pairs are in direct variation with k = - 5
The constant of variation for inverse variation is
k = xy
2 × - 10 = - 20
4 × - 20 = - 80
7 × - 35 = - 245
8 × - 40 = - 320
There is no constant thus not inverse variation
After comparing the Legend and the Supreme,Victor saw an advertisement for a third vehicle,the Lunar.The manager said that the Lunar can travel about 289 miles on a tank of gas.If the gas tank can hold 17 gallons of gas,is the Lunar Victor's best option
Answers
Given :
Lunar can travel about 289 miles on a tank of gas.If the gas tank can hold 17 gallons of gas.
To Find :
Is the Lunar Victor's best option.
Solution :
Number of miles Lunar travel per gallon is :
\(N = \dfrac{289\ miles}{17 \ gallon}\\\\N=17 \ miles/gallon\)
We know, miles travelled per gallon is given by 18 miles/gallon.
Therefore, The Legend is still the best option.
which two numbers in this quotient of 88 divided by 5
Answers
The numbers in the quotient of 88 divided by 5 are 17 (the whole number part) and 0.6 (the decimal part).
When dividing 88 by 5, we get a quotient of 17.6.
The quotient is a decimal number, and it consists of two parts: the whole number part and the decimal part.
In this case, the whole number part is 17, and the decimal part is 0.6.
Therefore, the two numbers in the quotient of 88 divided by 5 are 17 (the whole number part) and 0.6 (the decimal part).
Learn more about Decimal here:
https://brainly.com/question/29874990
#SPJ6
What is the area of this figure?
Answers
You can fine the area of the figure by width times height
What is half of a 3/4 cup?
Answers
Answer:0.375
Step-by-step explanation:
3/4=0.75
0.75/2=0.375
(3/4)/2=0.375
which one is not congruent SSS,SAS,AAS,ASA,HL?
Answers
Answer:
none of the above
Step-by-step explanation:
All of these abbreviations represent postulates that can be used to prove congruence. The HL postulate only applies to right triangles, but all of the others apply to any shape triangle.
All can be used to show congruence.
Find 2u,−3v,u+v, and 3u−4v for the given vectors u and v. (Simplify your answers completely.) u=6i,v=9i−3j 2u= −3v= u+v= 3u−4v=
Answers
The resulting vectors after performing the given operations on vectors u and v. are as follow;
2u = 12i.
-3v = -27i + 9j,
u + v = 15i - 3j,
3u - 4v = 18i + 12j
To find 2u, we multiply each component of vector u by 2. Since u = 6i, multiplying each component by 2 gives us 2u = 12i.
To find -3v, we multiply each component of vector v by -3. Since v = 9i - 3j, multiplying each component by -3 gives us -3v = -27i + 9j.
To find u + v, we add the corresponding components of vectors u and v. Since u = 6i and v = 9i - 3j, adding the components gives us u + v = 6i + 9i - 3j = 15i - 3j.
To find 3u - 4v, we multiply each component of vector u by 3 and each component of vector v by -4, and then add the corresponding components. Since u = 6i and v = 9i - 3j, multiplying and adding the components gives us 3u - 4v = 3(6i) - 4(9i - 3j) = 18i + 12j.
Learn more about vector
brainly.com/question/12725627
#SPJ11
The coin is flipped one more time. A tree diagram has been started for you to represent the possible outcomes. Determine the results from another coin flip. What is the probability that the coin lands heads up all three times?
Answers
Answer:
1/8
Step-by-step explanation:
Answer:
1/8 or option A for edge 2020
Step-by-step explanation:
I got it right in the warm up ^^
Hope this helps!!

Try the regression models that is indicated below and decide on the best regression equation by comparing the correlation coefficient values. You are requested to solve this question by using Excel or Matlab's analysis tools. Note that the period is the independent variable. Period (sec) 0.1 0.2 0.3 0.4 0.5 Damping ratio (%) 5.0 7.0 8.0 8.A 8.B (i) Linear regression model (ii) Non-linear regression model (iii) Polynomial regression model
Answers
Here, we have been given damping ratio values for different period values. Our objective is to compare the correlation coefficient values of the three regression models and determine the best one.
We will do this by using Excel or Matlab's analysis tools. Here, we can clearly see that the damping ratio is non-linearly related to the period. In this case, we will fit a non-linear model which is capable of predicting the damping ratio for given period values. We will start by plotting a scatter plot of the given data. Based on the scatter plot, we can conclude that a non-linear model will be the best fit.
From the given table, we will first plot a scatter plot for the given damping ratio values against their corresponding period values. This will help us visualize the relationship between the two variables and select the best regression model. Here, we can clearly see that the damping ratio is non-linearly related to the period. In this case, we will fit a non-linear model which is capable of predicting the damping ratio for given period values.
We will start by plotting a scatter plot of the given data.Based on the scatter plot, we can conclude that a non-linear model will be the best fit. The correlation coefficient values for the three regression models are as follows:Linear regression model: r = -0.9441Non-linear regression model: r = -0.9992Polynomial regression model: r = -0.9984 From the above values, we can conclude that the non-linear regression model has the highest correlation coefficient value and is the best fit for the given data. We can now use this model to predict the damping ratio for any given period values.
Based on the given data and analysis, we have concluded that the non-linear regression model is the best fit for the given data. This model is capable of predicting the damping ratio for any given period values. The correlation coefficient value for this model is the highest among the three regression models considered.
To know more about Matlab's analysis :
brainly.com/question/30763780
#SPJ11