Answers
Answer:
103 to 107 is (2). 108 to 112 is (4). 113 to 117 is (2)
Step-by-step explanation:
Related Questions
Which expression is equivalent to 9-3?
3(y-1)
3(3y-1)
9(y-3)
9(3y-3)
if its correct I will give brainliest!♡
Answers
Answer:
B because 3*3=9 And 3*1=3 so it would be 3(3y-1) foil the answer
Step-by-step explanation:
The answer is B, I took the test.
3. The expression 2x+6 is equal to 9 for some value of x. Without finding the value of x, determine the
values for each of the following expressions. Show how you arrived at each answer.
(b) 2x+9
(a) 4x+12
2 (2x+6)
2(a)
18
(d) -6x-18
-3(2x+6)
-3(a)
-27
(e) 2x+1
22460
(c) x+3
26200
(f) 10x+32
in of
Answers
Answer:
E, B, 2 = (2x + 6)
Step-by-step explanation:
I think those are correct.
Let C be a cylindrical can (including top and bottom lids) with height h and radius r.
(a) Write a multivariable function S(h, r) for the surface area of the can.
(b) Calculate S(3,2), S (3,2), and S,(3,2).
(c) Give a linear approximation for S(2.75, 2.1).
Answers
The linear approximation for S(2.75, 2.1) is approximately 7.9 times the area of a circle with radius 2.1.
(a) The surface area of the can can be divided into three parts: the top lid, the bottom lid, and the lateral surface.
The area of each lid is a circle with radius r, so the combined area of the two lids is 2πr^2. The lateral surface area is a rectangle with width 2πr (the circumference of the circle) and height h, so its area is 2πrh. Therefore, the total surface area is:
S(h, r) = 2πr^2 + 2πrh
(b) To calculate S(3,2), we plug in h=3 and r=2:
S(3,2) = 2π(2)^2 + 2π(2)(3) = 4π + 6π = 10π
To calculate Sr(3,2), we take the partial derivative of S with respect to r and evaluate at h=3 and r=2:
Sr(h,r) = 4πr + 2πh
Sr(3,2) = 4π(2) + 2π(3) = 8π + 6π = 14π
To calculate Sh(3,2), we take the partial derivative of S with respect to h and evaluate at h=3 and r=2:
Sh(h,r) = 2πr
Sh(3,2) = 2π(2) = 4π
(c) The linear approximation for S(2.75, 2.1) is:
S(2.75, 2.1) ≈ S(3,2) + Sr(3,2)(2.75-3) + Sh(3,2)(2.1-2)
We already calculated S(3,2), Sr(3,2), and Sh(3,2) in part (b), so we plug in the values:
S(2.75, 2.1) ≈ 10π + 14π(-0.25) + 4π(0.1) = 10π - 3.5π + 0.4π = 7.9π
Therefore, the linear approximation for S(2.75, 2.1) is approximately 7.9 times the area of a circle with radius 2.1.
To learn more about radius, refer below:
https://brainly.com/question/13449316
#SPJ11
fully simplify 7h² + 2h + 5 - h² + h
Answers
Answer:
6h² + 3h + 5
Step-by-step explanation:
7h² + 2h + 5 - h² + h
= 7h² - h² + 2h + h + 5
= 6h² + 3h + 5
Find the point at which the line intersects the given plane.
x = t − 1, y = 1 + 2t, z = 3 − t; 6x − y + 5z = 9
Answers
The point at which the line intersects the plane x = t − 1, y = 1 + 2t, z = 3 − t and 6x − y + 5z = 9 is (-2, -1, 4).
Substituting the parametric equations of the line into the equation of the plane and solving for the parameter t.
Now substituting the parametric equations of the line into the equation of the plane, we get:
6x - y + 5z = 9
6(t-1) - (1+2t) + 5(3-t) = 9
Simplifying this expression, we get:
-3t + 8 = 9
Solving for t, we get:
t = -1
Now that we have found the value of t, we can substitute it back into the parametric equations of the line to find the point of intersection:
x = t - 1 = -2
y = 1 + 2t = -1
z = 3 - t = 4
Therefore, the point at which the line intersects the plane is (-2, -1, 4).
Learn more about parametric equations here:
https://brainly.com/question/30286426
#SPJ11
Pls solve these polynomial
(2x-4)(x+5)
(X-2^2)
(3x+1)^2
(3x-1)(2x^2+5x-4)
Answers
1- (2x-4)(x+5)
Multiplying using the distributive property, we get:
(2x-4)(x+5) = 2x(x) + 2x(5) - 4(x) - 4(5)
= 2x^2 + 10x - 4x - 20
= 2x^2 + 6x - 20
Therefore, (2x-4)(x+5) simplifies to 2x^2 + 6x - 20.
2-(x-2)^2
Expanding using the formula for the square of a binomial, we get:
(x-2)^2 = x^2 - 4x + 4
Therefore, (x-2)^2 simplifies to x^2 - 4x + 4.
3- (3x+1)^2
Expanding using the formula for the square of a binomial, we get:
(3x+1)^2 = (3x)^2 + 2(3x)(1) + (1)^2
= 9x^2 + 6x + 1
Therefore, (3x+1)^2 simplifies to 9x^2 + 6x + 1.
4- (3x-1)(2x^2+5x-4)
Using the distributive property, we can multiply each term in the first polynomial by each term in the second polynomial:
(3x-1)(2x^2+5x-4) = 3x(2x^2) + 3x(5x) - 3x(4) - 1(2x^2) - 1(5x) + 1(4)
= 6x^3 + 15x^2 - 12x - 2x^2 - 5x + 4
= 6x^3 + 13x^2 - 17x + 4
Therefore, (3x-1)(2x^2+5x-4) simplifies to 6x^3 + 13x^2 - 17x + 4.
Work out the value of (3^2)^2x(10^3)^2
Answers
Answer:
81,000,000
Step-by-step explanation:
\(( {3}^{2} )^{2} \times ({10}^{3} )^{2} \\ \\ = ( 9)^{2} \times (1000 )^{2} \\ \\ = 81 \times 1000000 \\ \\ = 81,000,000\)
hlp me hlp me hlp me hlp me
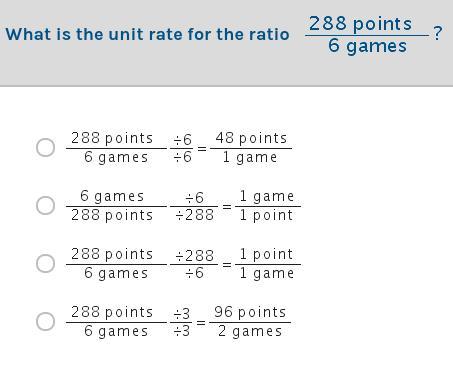
Answers
The unit rate would be points per game.
To find the points per game, divide both numbers by 6:
288/6 = 48
the unit rate would be 48 points / 1 game.
The answer is the first choice.
Answer:
\(\frac{288 points}{6 games}\) ÷ \(\frac{6}{6} =\frac{48 points}{1 game}\) or the 1st option
Step-by-step explanation:
\(\frac{288 points}{6 games}\) ÷ \(\frac{6}{6} =\frac{48 points}{1 game}\)
We get this answer because to find the unit rate, we need to get however many points over one game.
at what rate, how long will it take to go 4.5 miles
Answers
Step-by-step explanation:
they need a car to drive duhhhhh
Answer:
0.36 hours
Step-by-step explanation:
Divide 4.5 with 12.5 mph.
y> 3x-2
Graph the solution
Answers
Answer:
4 es esa ????k nose
Can somebody who knows both plz answer correctly!!!!
(Will mark as brainliest)
:D

Answers
19. 3 1/3, 2 1/5, 9/5, 9/10, 2/15
How do you write 140% as a fraction, mixed number, or whole number?
Answers
The requried, 140% is equivalent to 7/5 as a fraction, 1 2/5 as a mixed number, and 2 as a whole number.
To write 140% as a fraction, we first recognize that "percent" means "per hundred," so 140% can be written as the fraction 140/100. We can simplify this fraction by dividing both the numerator and denominator by their greatest common factor (GCF), which is 20:
140/100 = 7/5
To write 7/5 as a mixed number, we divide the numerator by the denominator and express the result as a whole number plus a fraction. In this case:
7 ÷ 5 = 1 with a remainder of 2
So 7/5 can be written as the mixed number 1 2/5.
To write 7/5 as a whole number, we can round it to the nearest whole number. Since 7/5 is greater than 1.5 and less than 2.5, it rounds to 2.
Learn more about fractions here:
https://brainly.com/question/10708469
#SPJ1
In Exercises 3-6, write a compound inequality that is
represented by the graph.

Answers
Answer:
3). x > -3 and x <=2
4) x >7 and x < 14
5) x <= -7 or x > = - 4
6) x <= 4 or x >6
7. p < 6 and p >2
6 > p > 2
8. n <= -7 or n > 12
- 7 <= n > 12
Step-by-step explanation:
What multiplication equattion can be used to explain the solution to 15 / 1/3
Answers
Step-by-step explanation:
15 / (1/3) is equal to 15 x 3/1 = 15 x 3 = 45
Reciprocal of 1/3 = 3/1
So, the multiplication equation that explains the solution is:
15 * (3/1) = 45
Therefore, 15 divided by 1/3 is equal to 45.
write an inequality to express the relationship between -2 and -5?
Answers
\(\qquad\qquad\huge\underline{{\sf Answer}}\)
The inequality that express the relationship between -2 and -5 is : " greater than " or " > "
\(\qquad \tt \dashrightarrow \: - 2 > - 5\)
Graph and Label the Points on the coordinate plane below

Answers
The X-Axis contains the first number in your ordered pair, and goes left, right (horizontally) for example the first ordered pair you have.
(2,5 1/2). To do this, move 2 spaces to the right on your X-axis. now go 5 and a half spaces up, and plot the point.
(-2 1/2, 0.5) For this one, you move 2 and a half spaces to the left on the X-axis, and half a space up. put a point there.
(3.5, -4 1/2) move 3 and a half spaces to the right on your X-axis, and go 4 and a half spaces down. put a point there.
let me know if you have questions! :)
Nevaeh has read 168 pages of a 210- page book. What percent of the book has Nevaeh not read?
Answers
Answer:
168 ÷ 210 X 100
80 % is your answer
Step-by-step explanation:
folloe me plzzz
find two positive real numbers such that the sum of the first number and the second number is 48 and their product is a maximum
Answers
Answer:
x = 24 and y = 24
Step-by-step explanation:
Let's use algebra to solve this optimization problem.
Let x be the first number, and y be the second number. Then we have the following two equations based on the problem statement:
x + y = 48 (sum of the two numbers is 48)
xy = ? (product of the two numbers, which we want to maximize)
To solve for x and y in terms of each other, we can use the fact that:
(x + y)^2 = x^2 + 2xy + y^2
Expanding the left side of the equation gives:
x^2 + 2xy + y^2 = 2304
And substituting xy for its value in terms of x and y gives:
x^2 + 2xy + y^2 = x^2 + 2(48 - x)y + y^2 = 2304
Simplifying this equation gives:
2y^2 - 96y + x^2 - 2304 = 0
To maximize the product xy, we need to maximize the value of xy = x(48 - x) = 48x - x^2. This function is a quadratic that opens downwards, and therefore, its maximum value occurs at the vertex of the parabola, which is located at x = -b/2a = -48/(2*-1) = 24.
Thus, the two positive real numbers that sum up to 48 and their product is a maximum are x = 24 and y = 24.
What point on the line 3x – y = 4 is closest to the point A(–2, 3)?
Answers
The closest point on the line 3x - y = 4 to the point A(-2, 3) is (4, 3).
The point on the line closest to point A can be found by finding the line that is perpendicular to the line 3x - y = 4 and passes through A, then finding the point of intersection between the two lines.
To find the perpendicular line, we can use the slope-point form of a line, which is y - y1 = m(x - x1), where m is the slope of the line and (x1, y1) is a point on the line. The slope of a line perpendicular to the line 3x - y = 4 is the negative reciprocal of the slope of the line, which is undefined since the line 3x - y = 4 is a vertical line. Hence, the perpendicular line is a horizontal line with slope 0, and it passes through the point A(-2, 3). So, the equation of the line is y = 3.
The point of intersection of the two lines is the point closest to A. Solving the equations 3x - y = 4 and y = 3, we get the intersection point (x, y) = (4, 3).
To learn more about line here:
https://brainly.com/question/30087020
#SPJ4
x²-5x divided by 2
= 0
Answers
Answer:
x = 10, x = 0
I dont know if you wanted an answer but yeah-
x^2-5x=0x2
x^2-5x=0
x(x-5)=0
Either, x=0 or x-5=0
x=0 or x=5
Find the perimeter of a rectangle with a diagonal of 20 inches that make angle measure 30 and 60 using trigonometric function (sine, cosine, tangent)
Answers
The perimeter of the rectangle is 20 + 20√3 inches.
How to find the perimeter of a rectangle?The perimeter of a rectangle is sum of the whole side of the rectangle. The side bounded by the rectangle is the perimeter of the rectangle.
Therefore, the diagonal of the rectangle is given as 20 inches.
Using trigonometric ratios, let's find the sides of the rectangle.
sin 30 = opposite / hypotenuse
sin 30 = x / 20
x = 20 sin 30
x = 20 × 0.5
x = 10 inches
Therefore, using Pythagoras theorem,
20² - 10² = b²
b² = 400 - 100
b = √300
b = √100 × 3
b = 10√3
Hence,
perimeter of the rectangle = 2(l + w)
perimeter of the rectangle = 2(10 + 10√3)
perimeter of the rectangle = 20 + 20√3
learn more on perimeter here: https://brainly.com/question/10807998
#SPJ1
A company's vice president's salary n years after becoming vice president is defined by the formula S(n) = 70000(1.2)". Which of the following statements is true? She will be receiving a 2% raise per year. Her salary will increase $14,000 every year. The rcent increase of her salary is 120% every year. Her salary is always 0.2 times the previous year's salary. The percent increase of her salary is 20% every year.
Answers
The correct statement is the percent increase of her salary is 20% every year. Hence, the answer is option E. Given that a company's vice president's salary n years after becoming vice president is defined by the formula S(n) = 70000(1.2)". We have to determine which of the following statements is true:
Given that a company's vice president's salary n years after becoming vice president is defined by the formula S(n) = 70000(1.2)". We have to determine which of the following statements is true:
She will be receiving a 2% raise per year. Her salary will increase $14,000 every year. The percent increase of her salary is 120% every year. Her salary is always 0.2 times the previous year's salary. The percent increase of her salary is 20% every year.
To calculate the salary of the vice president after n years of becoming a vice president, we use the given formula:
S(n) = 70000(1.2)
S(n) = 84000
The salary of the vice president after one year of becoming a vice president: S(1) = 70000(1.2)
S(1) = 84000
The percent increase of her salary is: S(n) = 70000(1.2)n
S(n) - S(n-1) / S(n-1) × 100%
S(n) - S(n-1) / S(n-1) × 100% = (70000(1.2)n) - (70000(1.2)n-1) / (70000(1.2)n-1) × 100%
S(n) - S(n-1) / S(n-1) × 100% = 20%
Therefore, the correct statement is the percent increase of her salary is 20% every year. Hence, the answer is option E.
To know more about salary visit:
https://brainly.com/question/14371457
#SPJ11
Which type of tide occurred on June 8?
A spring tide
B neap tide
C) full tide
D) half tide
PLEASE HELP
Answers
Answer:
A) spring tide
Step-by-step explanation:
Two hundred and four kids are going on a field trip. there needs to be one cheoparne for every four students. if a bus can hold 50 people how many buses will they need
Answers
A field trip will be taken by 204 children. One cheoparne must be assigned to every four students. 4 buses will be required if a bus can seat 50 people.
Two hundred and four kids are going on a field trip.
if a bus can hold 50 people
1 bus= 50 people
4 bus = 50* 4 = 200 people
A field trip or excursion is a travel taken by a group of people to a location that is different from their typical habitat. When done for kids, as it is in many school systems, it is referred to as a school trip in the United Kingdom, Australia, New Zealand, and Bangladesh, and a school tour in Ireland.
To learn more about field trip visit:https://brainly.com/question/15744154
#SPJ9
State whether the equation 2 2 = 3 2 defines (enter number of statement): 1. A hyperboloid of two sheets 2. A hyperboloid of one sheet 3. An ellipsoid 4. None of these 2 (1 point) State whether the equation y 2 2= + defines: A hyperbolic paraboloid
Answers
The equation\(2^2 = 3^2\) does not define any of the given shapes, as it is simply a false statement. The equation \(y^{2/2 }= x^{2/2\) does define a hyperbolic paraboloid.
On the other hand, the equation \(y^{2/2 }= x^{2/2\) defines a hyperbolic paraboloid. A hyperbolic paraboloid is a three-dimensional surface that has a saddle-like shape, with two opposing parabolic curves that cross each other. It is also known as a "saddle surface" due to its shape.
The equation \(y^{2/2 }= x^{2/2\) can be rewritten as \(y^{2/2 }= x^{2/2\), which is in the form of a hyperbolic paraboloid equation. This surface can be obtained by taking a parabolic curve and sweeping it along a straight line in a perpendicular direction. This creates a surface with a hyperbolic cross-section in one direction and a parabolic cross-section in the other direction.
Hyperbolic paraboloids have a wide range of applications in architecture, engineering, and design. They are often used in the construction of roofs, shells, and other structures that require strong and lightweight materials. They can also be used to create interesting and unique shapes in art and sculpture.
for such more question on hyperbolic paraboloid.
https://brainly.com/question/7242646
#SPJ11
The equation 2x^2 = 3y^2 does not define any of the given three-dimensional shapes.
This is because it does not contain a z variable, which is necessary to define these shapes in three dimensions. Therefore, the equation cannot represent any of the given shapes.
On the other hand, the equation y^2 = 2x defines a hyperbolic paraboloid. This is a three-dimensional shape that resembles a saddle. It is formed by taking a hyperbola and rotating it around its axis. In this case, the hyperbola is oriented along the x-axis, and the parabolic cross-sections occur in the y-direction.
The equation can be rewritten as y^2 = 2(x - 0)^2, which is the standard form of a hyperbolic paraboloid. This equation can be graphed in a three-dimensional coordinate system, with the x-axis and y-axis forming the base and the z-axis representing the height of the surface above the base.
The shape is characterized by its saddle-like appearance, with two opposing hyperbolic curves along the x-axis and two opposing parabolic curves along the y-axis.
To learn more about hyperbolic paraboloid, click here: https://brainly.com/question/7242646
#SPJ11
Jon deposits $2000 into his account and pays at a rate of 4% per year. how much will he be paid in 3 years
Answers
$2000*.04=80
80*3=240
2000*3=6000
6000-240=5760.
Hope this helps :)
the answer is 240
because 4% of 2000 is 80 so you just times 80 by 3 then you get 240
Gabby received 6 job offers from 15 interview he did last month.Which ratio best describes the relationship between the number of jobs he was not offered and the number of jobs for which he was interviewed
Answers
Answer:
the answer is 3:5
Step-by-step explanation:
Total number of jobs for the interview = 15
number of job offers received by Gabby = 6
number of jobs not offered = 15 - 6 = 9
therefore, the relationship will be 9:15
3:5
gauri, the youngest one at home, was playing with a pack of cards. she lost a card. later on, two cards were drawn at random from the incomplete pack. both came out to be spade. can you tell us the probability that lost card is a heart.
Answers
Without additional information about the number of hearts in the incomplete pack, we cannot determine the probability that the lost card is a heart.
To find the probability that the lost card is a heart, we can use conditional probability.
Let's denote the event of drawing two spades as A and the event of the lost card being a heart as B.
The probability of drawing two spades from an incomplete pack can be calculated as follows:
P(A) = (number of spades in the incomplete pack / total number of cards in the incomplete pack) * ((number of spades in the incomplete pack - 1) / (total number of cards in the incomplete pack - 1))
Now, since both cards drawn were spades, we know that event A has occurred.
To find the probability of the lost card being a heart given event A, we can use conditional probability:
P(B|A) = P(A ∩ B) / P(A)
The intersection of events A and B is the probability that both events A and B occur simultaneously.
However, since we don't have any information about the number of hearts in the incomplete pack, we cannot calculate the exact value of P(A ∩ B).
Therefore, without additional information about the number of hearts in the incomplete pack, we cannot determine the probability that the lost card is a heart.
To know more about probability visit :
https://brainly.com/question/31828911
#SPJ11
A quadratic equation of the form 0=ax2+bx+c has a discriminant value of -16. How many real number solutions does the equation have? -1012.
Answers
The given quadratic equation will have zero real-number solutions.
Since the given equation is: ax²+bx+c =0, and the discriminant value is -16, since the discriminant is a portion of the quadratic equation, the formula for calculating is b²-4ac, where a, b and c are the coefficients now if the discriminant is more than 0, then the respective equation has two real solutions, whereas if it is less than 0, it has zero real solutions and last, if the discriminant is equal to zero then the equation has only one real solution.We know the :-b±√b²-4ac /2a, here the square root of -16 can't give us a real number instead it will give us an imaginary number
To know more about quadratic equations refer to the link https://brainly.com/question/17177510?referrer=searchResults.
#SPJ4
a function is a relation in which each possible input value leads to exactly one output value. the input values make up the range and the output values make up the domain
Answers
A relation between sets is referred to as a function when there is exactly one output for each input.
Which statement is true?A function is a relation where each output value is assigned to exactly one input value.The domain of a function is the set of all output values, or y-values, for which the function is defined.The range of a function is the set of all input values, or x-values, for which the function is defined.To write the equation y = ax + b in function notation, substitute f(x) for y.?To write the equation y = ax + b in function notation, substitute f(x) for y
The only true statement is the last one. A function assigns exactly one output value to each input value. The domain is the set of all input, or x-values. The range is the set of all output, or y-values.
y=ax+b can be written in function notation by replacing y with f(x).
To know more about Function , visit :
ttps://brainly.com/question/17810174
#SPJ4
