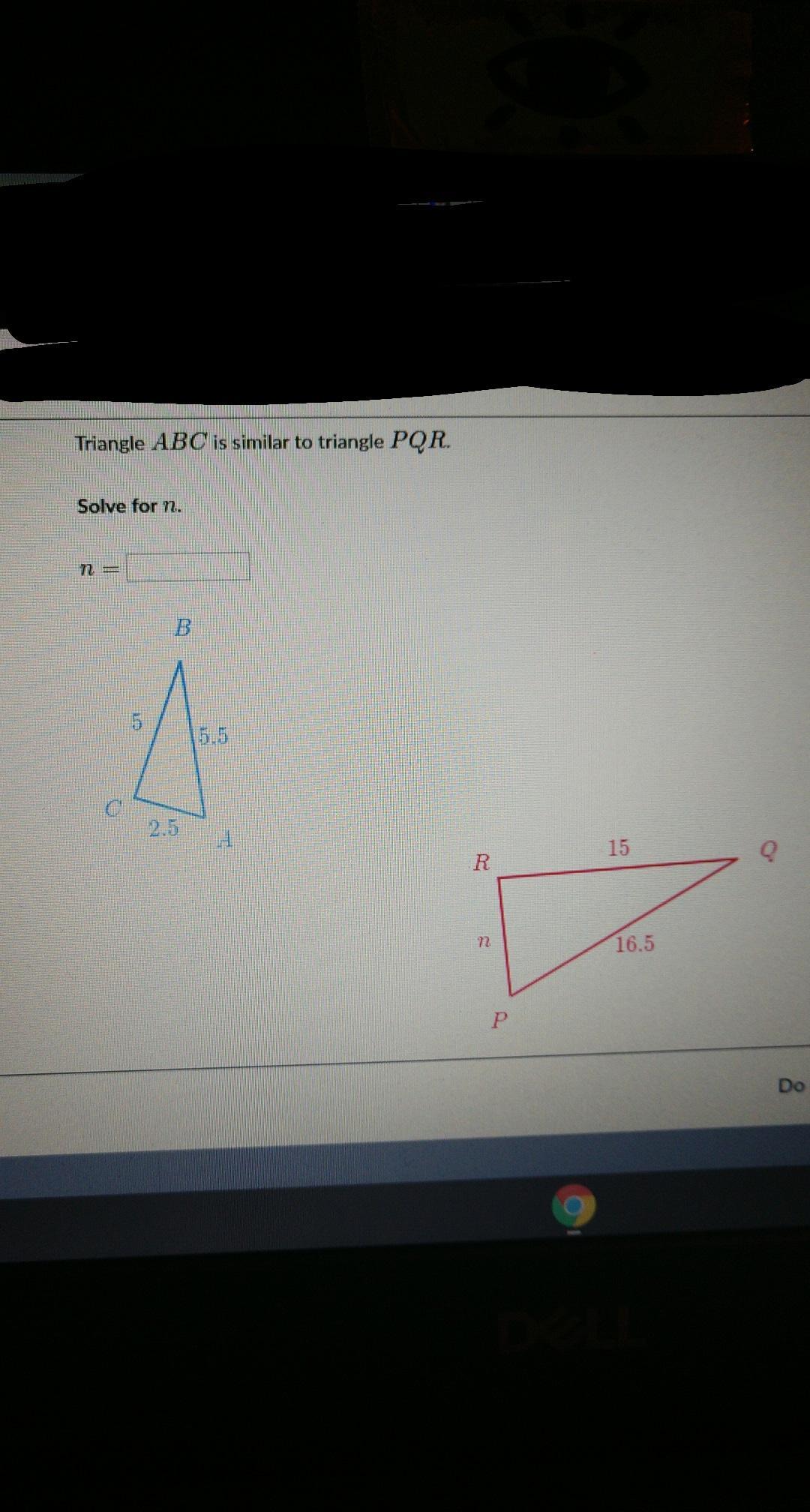
Please help me solve for x =
I message my teacher she said the side are not equal to each other, they are proportional ( please help me awnser)
Answers
Answer:
x = 13
Step-by-step explanation:
Given that Δ NML and Δ PST are similar right triangles, we can set up the following proportional statement to establish their relationship:
\(\frac{ML}{NM} = \frac{ST}{PS}\)
\(\frac{8}{10} = \frac{x - 1}{x + 2}\)
Cross multiply:
8(x + 2) = 10 (x - 1)
8x + 16 = 10x - 10
Subtract 8x from both sides:
8x - 8x + 16 = 10x - 8x - 10
16 = 2x - 10
Add 10 to both sides:
16 + 10 = 2x - 10 + 10
26 = 2x
Divide both sides by 2:
\(\frac{26}{2} = \frac{2x}{2}\)
13 = x
Verify whether x = 13 is the correct value:
\(\frac{ML}{NM} = \frac{ST}{PS}\)
\(\frac{8}{10} = \frac{x - 1}{x + 2}\)
\(\frac{8/2}{10/2} = \frac{4}{5}\)
\(\frac{x -1}{x+2} = \frac{13 - 1}{13 + 2} = \frac{12}{15} = \frac{12 / 3}{15 /3} = \frac{4}{5}\)
This shows the proportional relationship between \(\frac{ML}{NM} = \frac{ST}{PS}\), and that ΔNML and ΔPST are indeed similar right triangles.
Therefore, the correct answer is x = 13.
Related Questions
what goes in the blank box? hurry pls!
Answers
Answer:
your
Step-by-step explanation:
Find the area of the region R bounded by the graph of f and the x-axis on the given interval. Graph f and show the region R. f(x) = x^2 (x-6): [-1, 7] The area is. (Round to the nearest hundredth as needed.)
Answers
The area of the region R bounded by the graph of f(x) = x^2(x-6) and the x-axis on the interval [-1, 7] is approximately 371.00 square units.
Area of region with function f(x) = x^2(x-6) on interval [-1, 7]?To find the area of the region R bounded by the graph of f(x) = x^2(x-6) and the x-axis on the interval [-1, 7], we need to integrate the function f(x) over that interval. The area can be calculated using the definite integral:
Area = ∫[a,b] f(x) dx
In this case, a = -1 and b = 7. Let's calculate the area using integration:
Area = ∫[-1,7] x^2(x-6) dx
To solve this integral, we expand the polynomial and simplify:
Area = ∫[-1,7] (x^3 - 6x^2) dx
Next, we integrate term by term:
Area = (1/4)x^4 - (2/3)x^3 | [-1,7]
Now, we substitute the upper and lower limits of integration:
Area = [(1/4)(7^4) - (2/3)(7^3)] - [(1/4)(-1^4) - (2/3)(-1^3)]
Simplifying further:
Area = (1/4)(2401) - (2/3)(343) - (1/4)(1) + (2/3)(-1)
Area = 600.25 - 228.33 - 0.25 - 0.67
Area ≈ 371.00 (rounded to the nearest hundredth)
Therefore, the area of the region R bounded by the graph of f(x) = x^2(x-6) and the x-axis on the interval [-1, 7] is approximately 371.00 square units.
Learn more about find the area
brainly.com/question/29045725
#SPJ11
The difference of two positive integers is 5 and the sum of their squares is 433. Find their integers.
Answers
Answer:
12, 17
Step-by-step explanation:
Let the two positive integers be x and (5 + x).
According to the given description:
\( {x}^{2} + {(x + 5)}^{2} = 433 \\ {x}^{2} + {x}^{2} + 10x + 25 = 433 \\ \\ 2 {x}^{2} + 10x + 25 - 433 = 0 \\ \\ 2 {x}^{2} + 10x - 408 = 0 \\ \\ 2( {x}^{2} + 5x - 204) = 0 \\ \\ {x}^{2} + 5x - 204 = 0 \\ \\ {x}^{2} + 17x - 12x - 204 = 0 \\ \\ x(x + 17) - 12(x + 17) = 0 \\ \\ (x + 17)(x - 12) = 0 \\ \\ x + 17 = 0 \: or \: x - 12 = 0 \\ \\ x = - 17 \: or \: x = 12 \\ \\ \because \: x \: is \: a \: + ve \: integer \\ \\ \implies x \neq - 17 \\ \\ \therefore \: x = 12 \\ \\ 5 + x = 5 + 12 = 17 \\ \)
Thus, the two positive integers are 12 and 17.
Answer:
12 and 17
Step-by-step explanation:
17 - 12 = 5, and 17^2 (289) + 12^2 (144) = 433
Hope this helps :D
45 is 0.9% of what number
Answers
Answer:
0.405
Step-by-step explanation
45 x 0.9%= 0.405
Answer:
x = 5000
Step-by-step explanation:
Let us say 45 is 0.9% of x
=> 0.9% × x = 45
=> 0.9/100 × x = 45
=> x = 45 × 100/0.9
=> x = 4500/0.9
=> x = 45000/9
=> x = 5000
Drag the expressions to the correct functions. Not all expressions will be used.
Consider the functions fand g.
= 4x² + 1
g(x) =
Perform the function compositions:
x² - 3
Answers
The function composition exists an operation " ∘ " that brings two functions f and g, and has a function h = g ∘ f such that h(x) = g(f(x)).
Let the functions be f(x) = 4x² + 1 and g(x) = x² - 3
The correct answer is (f o g)(x) = 4x⁴ - 96x + 37 and
(g o f)(x) = 16x⁴ + 8x² - 2.
What is composition function?The function composition exists an operation " ∘ " that brings two functions f and g, and has a function h = g ∘ f such that h(x) = g(f(x)). In this operation, the function g exists used for the outcome of applying the function f to x.
Given:
f(x) = 4x² + 1 and g(x) = x² - 3
a) (f o g)(x) = f[g(x)]
f[g(x)] = 4(x² - 3)² + 1
substitute the value of g(x) in the above equation, and we get
= 4(x⁴ - 24x + 9) + 1
simplifying the above equation
= 4x⁴ - 96x + 36 + 1
= 4x⁴ - 96x + 37
(f o g)(x) = 4x⁴ - 96x + 37
b) (g o f)(x) = g[f(x)]
substitute the value of g(x) in the above equation, and we get
g[f(x)] = (4x² + 1)²- 3
= 16x⁴ + 8x² + 1 - 3
simplifying the above equation
= 16x⁴ + 8x² - 2
(g o f)(x) = 16x⁴ + 8x² - 2.
Therefore, the correct answer is (f o g)(x) = 4x⁴ - 96x + 37 and
(g o f)(x) = 16x⁴ + 8x² - 2.
To learn more about the function refer to:
https://brainly.com/question/26709985
#SPJ9
Help me get this:
Y=3x+C
Answers
Hi there!
~
\(Y = 3x + c\)
Simplifying
\(Y = 3x + c\)
Reorder the terms:
\(Y = c + 3x\)
Solving
\(Y = c + 3x\)
Solving for variable 'Y'.
Move all terms containing Y to the left, all other terms to the right.
Simplifying
\(Y = c + 3x\)
Hope this helped you!
A school band went on a trip to a music festival. The band director was admitted for free, but it cost $100 to rent a bus for the trip and $4 for each student to get into the festival. The total cost of the trip was $244. Which equation can be used to find s, the number of students that went on the trip?
Answers
Answer:
100 + 4s = 244
Step-by-step explanation:
Cost of the ticket for s students = 4s
Bus rent + cost of ticket for 's' students = $ 244
100 + 4s = 244
Answer:
i cant answer it either its so hard
Step-by-step explanation:
If 4.3m=0.086, then find the value of m
Answers
M=0.02
Answer:
\(m=0.02\)
Step-by-step explanation:
\(4.3m=0.086\\m=\frac{0.086}{4.3} \\m=0.02\)
Solve the system of equations: {2(3x-2y)+1=7x, 12(x+y)-15=7x+12y
Answers
Step-by-step explanation:
hope this help have a great day!
Angie has 18 oatmeal cookies.She splits them evenly among b bags.Choose the expression that shows how many cookies are in each bag. 1.B-18 2.18/b 3.18 4.B
Answers
Answer:
2, 18/b
Step-by-step explanation:
if you divide by the number of bags you will get the number of cookies in each bag.
Answer:2,18/b
Step-by-step explanation:If you multiply the cookies in a bag by b, you will get 18
A rectangle has a length that is five more than its width. If the total perimeter of the rectangle is 42, what is the
width of the rectangle?
Answers
Answer:
Width is 8
Step-by-step explanation:
2(x) + 2(x+5)=42
2x+ 2x + 10 = 42
4x=32
x= 8
This is probably simple but can someone help me with this??
Answers
Answer:
7.5 units
Step-by-step explanation:
First, create a proportion by picking 2 sides:
2.5/n = 5/15
Next, cross multiply:
5n = 37.5
Finally, divide both sides to isolate the variable:
7.5
Therefore your answer is 7.5 units.
three equal charges q form an equilateral triangle of side a . part a find the potential, relative to infinity, at the center of the triangle. express your answer in terms of the variables a , q , and the coulomb constant k . activate to select the appropriates template from the following choices. operate up and down arrow for selection and press enter to choose the input value typeactivate to select the appropriates symbol from the following choices. operate up and down arrow for selection and press enter to choose the input value type activate to select the appropriates template from the following choices. operate up and down arrow for selection and press enter to choose the input value type v
Answers
the potential at the center of the equilateral triangle is 3kq√3 / a.To find , we can use the formula for the electric potential due to a point charge:
V = kq / r
where k is the Coulomb constant, q is the charge, and r is the distance between the point charge and the center of the triangle.
Assuming the charges are all positive, the potential at the center due to each charge will be the same, so we can find the total potential by multiplying the potential due to one charge by three.
The distance from the center of the equilateral triangle to each charge is a/√3, since the height of the equilateral triangle is √3/2 times the side length.
Therefore, the potential at the center is:
V = 3(kq / (a/√3))
Simplifying:
V = 3kq√3 / a
Hence, the potential at the center of the equilateral triangle is 3kq√3 / a.
To learn more about triangle click here:brainly.com/question/2773823
#SPJ11
Kayla drives 15 miles in 20 minutes. If she drove one hour in total at the same rate,
how far did she go?
Answers
Answer:
45 miles
Step-by-step explanation:
first divide miles/minutes: 15 / 20 = 0.75
then multiply: 0.75 x 60 = 45
Help!!! Will give brainiest!!
Answers
Answer:
a: $3.75
b: $13.50
c: $7.50
d: $6.00
Step-by-step explanation:
hope this helps!
Answer:
1. c
2.a
3.d
4.b
Step-by-step explanation:
1.£7.50/3
2.£3.75/5
3.£6.00/4
4.£13:50/6
Let me know if this helps xx
an elementary school teacher measures the heights of the students in her classroom. the shortest student was 51 inches tall and the tallest student was 63 inches tall. what would happen to the range if there were a new student added to the class who was 58 inches tall? question 13 options:
Answers
The range of the class between shortest student and longest student is 12 inches.
Range is the difference between highest and lowest possible values.
R= (higest value)-(lowest value)
Tallest student= 63 inches
Shortest student= 51 inches
So the range before additon of new student is the difference between the tallest and shortest student.
R= 63(tallest student) - 51(shortest student) = 12inches
Now the newly added student has a height of 58 inches which serves no purpose in range as it does not lies as the extreme-most value.
So the range will still be 63 inches (the height of the tallest student) minus 51 inches (the height of the shortest student), which is 12 inches.
Learn more about finding range : https://brainly.com/question/26242034
#SPJ11
Len can travel 2,485 miles in 35 hours . How many miles can he travel in 12 hours
Answers
Answer:
Assuming Len doesn't need to eat, drink, or sleep at all, they can travel about 852 miles in 12 hours.
If an isosceles triangle ABC has a vertex B, and AB=6×+3, BC
BC=8x-1, and AC=10x-10, find x
Answers
Using the given information, the value of x is 2
Calculating the length of the leg of an isosceles triangleFrom the question, we are to determine the value of x
From the given information,
The isosceles triangle ABC has its vertex at B
If the vertex is at B, then we can conclude that
AB = BC (Legs of an isosceles triangle are equal a)
Also, from the given information,
AB = 6×+3
BC = 8x-1
Thus,
6x + 3 = 8x - 1
Collect like terms
3 + 1 = 8x - 6x
4 = 2x
2x = 4
∴ x = 4/2
x = 2
Hence, the value of x is 2
Learn more on Isosceles triangle here: https://brainly.com/question/18640272
#SPJ1
For f(x) = sin x + cos x on [0,27], determine all intervals where f is increasing or decreasing.
Answers
To determine where the function f(x) = sin x + cos x is increasing or decreasing on the interval [0, 27], we need to find the intervals where the derivative is positive (increasing) or negative (decreasing).
First, let's find the derivative of f(x):
f'(x) = d/dx(sin x + cos x) = cos x - sin x
Now, let's find where f'(x) = 0:
cos x - sin x = 0
Rearranging the equation, we have:
cos x = sin x
Dividing both sides by cos x (assuming cos x is not zero), we get:
1 = tan x
Now, let's analyze the intervals where f'(x) is positive or negative by considering the signs of cos x - sin x within these intervals.
1) Interval [0, π/2]:
In this interval, both cos x and sin x are positive, so cos x - sin x is also positive. Therefore, f'(x) > 0, and f(x) is increasing on [0, π/2].
2) Interval (π/2, π]:
In this interval, cos x is negative, and sin x is positive. Thus, cos x - sin x is negative. Therefore, f'(x) < 0, and f(x) is decreasing on (π/2, π].
3) Interval (π, 3π/2]:
In this interval, both cos x and sin x are negative, so cos x - sin x is positive. Hence, f'(x) > 0, and f(x) is increasing on (π, 3π/2].
4) Interval (3π/2, 2π]:
In this interval, cos x is positive, and sin x is negative. Thus, cos x - sin x is positive. Therefore, f'(x) > 0, and f(x) is increasing on (3π/2, 2π].
Based on the analysis above, we can conclude that f(x) = sin x + cos x is increasing on the intervals [0, π/2], (π, 3π/2], and (3π/2, 2π], and decreasing on the interval (π/2, π].
Learn more about derivative here:
brainly.com/question/25324584
#SPJ11
What is the answer for this question (625\256)3/4 I need it asap
Answers
Answer:
1 11/64
Step-by-step explanation:
we will have to square root the numerator and the denominator that is 625/256 which will give us 25/16. No need to change it to mixed number then multiply 25/16 by 3/4, you can't divide through so you multiply and it will give you 75/64. Then change it to a mixed number, thats how we got 1 11/64.
You would like to build another rectangular garden but this time you would like it to be split in half (so there will be a fence down the middle). If you have 1,200 feet of fencing, what are the dimensions of the largest garden you can create
Answers
Answer:
Dimensions of each rectangular garden is 75ft by 150ft.
The total area of both rectangular gardens combined is
(75 x 150) x 2 = 22500 square feet.
If you want to build a rectangular garden that will be split in half with a fence down the middle, then it means you will have two rectangular gardens.
The amount of fencing you have is 1200 feet and you want to know the largest dimensions you can create.
The perimeter of a rectangle is calculated using the formula:
P = 2(L + W)
where P is the perimeter,
L is the length and
W is the width.
To maximize the area, the two rectangular gardens should be of equal size.
This means the two gardens will each have the perimeter
P = 600 ft.
Let L be the length of each garden and W be the width of each garden.
L + 2W = 600 (the length of one garden plus the length of the two fences on either side)
2L + W = 600 (the length of both gardens plus the length of the fence in between)
Also, the total amount of fencing is 1200ft which can be expressed as:
P = 2(L + W) + 600 (because there is a fence down the middle of the two gardens).
Therefore:
1200= 2(L + W) + 600
Simplify the above equation:
2(L + W) = 600
The value of 2(L + W) from the first equation can be substituted into the second equation:
1200 = 2(L + W) + 600
1200 = 1200L + W = 300
Therefore, L = 150 - W (by rearranging the equation)
Now substitute L into the area formula:
Area = L x W
Area = (150 - W)W
Area = 150W - W²
To find the maximum area, we need to differentiate and set the derivative equal to zero.
Area = 150W - W²A = 0 =
dA/dW = 150 - 2W
W = 75
Therefore:
L = 150 - 75 =
dimensions of each rectangular garden is 75ft by 150ft.
The total area of both rectangular gardens combined is (75 x 150) x 2 = 22500 square feet.
Learn more about Perimeter and area of rectangle ,visit here
https://brainly.com/question/31414959
#SPJ11
Polygon i a caled copy of Polygon. 2 4-ided polygon, P and Q. 1 ide in polygon P i 4 and it correponding ide in Q i 3. Another ide in polygon P i x, and it correponding ide in Q i y. The value of i 6, what i the value of ?
What i the cale factor?
Answers
When a side in polygon P is 6 the corresponding side in polygon Q is 4.5
the scale factor is 4/3
How to find the corresponding side of a scaled polygonFrom the question it can be deduced that
The polygon is four sided
1 side in polygon P is 4 and it corresponding side in Q is 3
let the scale factor be k
4 * k = 3
k = 3/4
When a side in polygon P is 6 the corresponding side in polygon Q is solved using the scale factor
the side in polygon Q = 6 * k
the side in polygon Q = 6 * 3/4
the side in polygon Q = 4.5
complete question
Polygon P is a scaled copy of Polygon Q. the 2 are 4-sided polygon, P and Q. 1 side in polygon P is 4 and it's correponding side in Q is 3. Another side in polygon P is x, and it correponding side in Q is y. The value of x is 6, what is the value of y?
What is the scale factor?
Learn more about scale factor:
https://brainly.com/question/26855848
#SPJ1
The blueprint of a fishing pier uses a scale of 1/8 inch = 5 feet. If the pier is 3 19/4 inches long on the blueprint, find its actual length.
pls!
Answers
Answer:
190
Step-by-step explanation:
1/8= 5 ft
19/4 = 38/8
38/8 = 38*5 = 190 feet
What percent of newborn African lions weigh less than 3 pounds? b. What percent of newborn African lions weigh more than 3.8 pounds?
Answers
The weight distribution of newborn African lions may not necessarily follow a normal distribution, and the actual percentages can vary based on the specific data available.
To determine the percentage of newborn African lions that weigh less than 3 pounds and the percentage that weigh more than 3.8 pounds, we would need statistical data on the weight distribution of newborn African lions. Since we don't have that information, we cannot provide the exact percentages.
However, if we assume that the weights of newborn African lions follow a normal distribution, we can use the properties of the standard normal distribution to make an estimation.
For the percentage of newborn African lions weighing less than 3 pounds:
We would need to know the mean and standard deviation of the weight distribution to calculate the Z-score for a weight of 3 pounds and then find the corresponding percentage using a standard normal distribution table. Without this information, we cannot provide an accurate estimation.
For the percentage of newborn African lions weighing more than 3.8 pounds:
Similarly, we would need the mean and standard deviation of the weight distribution to calculate the Z-score for a weight of 3.8 pounds and find the corresponding percentage using a standard normal distribution table. Without the required information, we cannot provide a specific estimation.
Please note that the weight distribution of newborn African lions may not necessarily follow a normal distribution, and the actual percentages can vary based on the specific data available.
To know more about percentages visit
https://brainly.com/question/32197511
#SPJ11
a rocket is fired from the gorund at a n angle of 1.02 radians. suppose the rocket has rtraveled 435 yards since it was launched. Draw a diagram and label the values that you know. a) How many yard has rocket travelled horizontally from where it was launched?
b) What is the rocket's height above the ground
Answers
A rocket is launched from the ground at an angle of 1.02 radians and has traveled 435 yards. The task is to determine the horizontal distance traveled by the rocket and its height above the ground.
The diagram of the situation can be drawn using the given information. The angle of launch can be labeled as 1.02 radians, and the horizontal distance traveled can be labeled as 435 yards. We need to determine the horizontal and vertical components of the rocket's displacement.
The horizontal displacement is the distance traveled by the rocket parallel to the ground, and it can be determined using the formula:
Horizontal displacement = Distance traveled x cos(angle of launch)
Substituting the given values, we get:
Horizontal displacement = 435 x\(cos(1.02)\) = 435 x 0.454 = 197.49 yards (rounded to two decimal places)
The vertical displacement is the change in the height of the rocket from the ground. It can be determined using the formula:
Vertical displacement = Distance traveled x sin(angle of launch)
Substituting the given values, we get:
Vertical displacement = 435 x sin(1.02) = 435 x 0.891 = 388.19 yards (rounded to two decimal places)
Therefore, the rocket has traveled 197.49 yards horizontally from where it was launched, and its height above the ground is 388.19 yards.
Learn more about height here:
https://brainly.com/question/21836055
#SPJ11
Find the median. 12,5,9,18,22,25,5
Answers
Answer:
The answer is 12.
Answer:
12
Step-by-step explanation:
To Find Median;
Arrange data values from lowest to highest valueThe median is the data value in the middle of the setIf there are 2 data values in the middle the median is the mean of those 2 values.Solve;
Least to Greatest:
5, 5, 9, 12, 18, 22, 25
5, 5, 9, 12, 18, 22, 25
5, 5, 9, 12, 18, 22, 25
5, 5, 9, 12, 18, 22, 25
Hence Median = 12.
[RevyBreeze]
What term is defined by the formula?
Answers
Ana has 8 stamps. Together, Ricky and ana have 23 stamps. The equation 23 = r + 8 represents this situation,where r is the number of stamps Ricky has. Solve the equation to find the number of stamps ricky has
Answers
Ricky has the number of stamps in the number of 15 stamps.
According to the question, the given parameters can be used to form the expression by using simplification method and the parameters are as follows:
Stamps for Ana: 8
Stamps for Ricky and Ana: 23
Given equation: 23 = r + 8
The equation formed by the simplification method is: r + a = 23
Solving above two equations, we get a = 8 stamps and r = 15 stamps.
Hence, Ricky has the number of stamps in the number of 15 stamps.
What is simplification?
The word simplification is the procedure in which complicated expression can be written in a simpler form to make the calculation easy. This method is used to calculated the answers for the unknown quantity.
To learn more about the simplification in fraction from the given link:
brainly.com/question/432678
#SPJ1
2. The following set of count readings was made in a gradient-free γ-ray field, using a suitable detector for repetitive time periods of one minute: 18,500;18,410; 18,250;18,760;18,600;18,220;18,540;18,270;18,670;18,540. (a) What is the mean value of the number of counts? (b) What is its standard deviation (S.D.)? (c) What is the theoretical minimum S.D. of the mean? (d) What is the actual S.D. of a single reading? (e) What is the theoretical minimum S.D. of a single reading?
Answers
The inflection point of f(t) is approximately t = 3.73.
(a) To determine if the function f(t) = -0.425t^3 + 4.758t^2 + 6.741t + 43.7 is increasing or decreasing, we need to find its derivative and examine its sign.
Taking the derivative of f(t), we have:
f'(t) = -1.275t^2 + 9.516t + 6.741
To determine the sign of f'(t), we need to find the critical points. Setting f'(t) = 0 and solving for t, we have:
-1.275t^2 + 9.516t + 6.741 = 0
Using the quadratic formula, we find two possible values for t:
t ≈ 0.94 and t ≈ 6.02
Next, we can test the intervals between these critical points to determine the sign of f'(t) and thus the increasing or decreasing behavior of f(t).
For t < 0.94, choose t = 0:
f'(0) = 6.741 > 0
For 0.94 < t < 6.02, choose t = 1:
f'(1) ≈ 14.982 > 0
For t > 6.02, choose t = 7:
f'(7) ≈ -5.325 < 0
From this analysis, we see that f(t) is increasing on the intervals (0, 0.94) and (6.02, ∞), and decreasing on the interval (0.94, 6.02).
(b) To find the inflection point of f(t), we need to find the points where the concavity changes. This occurs when the second derivative, f''(t), changes sign.
Taking the second derivative of f(t), we have:
f''(t) = -2.55t + 9.516
Setting f''(t) = 0 and solving for t, we find:
-2.55t + 9.516 = 0
t ≈ 3.73
Therefore, The inflection point of f(t) is approximately t = 3.73.
Learn more about inflection point here:
https://brainly.com/question/29249123
#SPJ11
A bolt manufacturer is very concerned about the consistency with which his machines produce bolts. The bolts should be 0.2 centimeters in diameter. The variance of the bolts should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level? Step 1 of 5: State the hypotheses in terms of the standard deviation. Round the standard deviation to four decimal places when necessary. A bolt manufacturer is very concerned about the consistency with which his machines produce bolts. The bolts should be 0.2 centimeters in diameter. The variance of the bolts should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level? Step 2 of 5: Determine the critical value(s) of the test statistic. If the test is twotailed, separate the values with a comma. Round your answer to three decimal places. A bolt manufacturer is very concerned about the consistency with which his machines produce boits. The bolts should be 0.2 centimeters in diameter. The variance of the boits should be 0.025. A random sample of 15 bolts has an average diameter of 0.21 cm with a standard deviation of 0.1587. Can the manufacturer conclude that the bolts vary by more than the required variance at α=0.01 level?
Answers
To determine if the bolts vary by more than the required variance, we can conduct a hypothesis test. The null hypothesis (H₀) states that the variance of the bolts is equal to or less than the required variance (σ² ≤ 0.025), while the alternative hypothesis (H₁) states that the variance is greater than the required variance (σ² > 0.025).
Next, we need to determine the critical value(s) of the test statistic. Since we are testing for variance, we will use the chi-square distribution. For a one-tailed test with α = 0.01 and 14 degrees of freedom (n-1), the critical value is 27.488.
Now, we can compare the test statistic to the critical value. The test statistic is calculated as (n-1) * s² / σ², where n is the sample size (15), s² is the sample variance (0.1587²), and σ² is the required variance (0.025).
If the test statistic is greater than the critical value, we reject the null hypothesis and conclude that the bolts vary by more than the required variance. Otherwise, we fail to reject the null hypothesis.
To learn more about Hypothesis test - brainly.com/question/29996729
#SPJ11
To determine if the bolts vary by more than the required variance, we can conduct a hypothesis test. The null hypothesis (H₀) states that the variance of the bolts is equal to or less than the required variance (σ² ≤ 0.025), while the alternative hypothesis (H₁) states that the variance is greater than the required variance (σ² > 0.025).
Next, we need to determine the critical value(s) of the test statistic. Since we are testing for variance, we will use the chi-square distribution. For a one-tailed test with α = 0.01 and 14 degrees of freedom (n-1), the critical value is 27.488.
Now, we can compare the test statistic to the critical value. The test statistic is calculated as (n-1) * s² / σ², where n is the sample size (15), s² is the sample variance (0.1587²), and σ² is the required variance (0.025).
If the test statistic is greater than the critical value, we reject the null hypothesis and conclude that the bolts vary by more than the required variance. Otherwise, we fail to reject the null hypothesis.
To learn more about Hypothesis test - brainly.com/question/29996729
#SPJ11