PLEASE HELP!! Thank you in advance.
The formula for the lateral surface area of a cylinder is S=2πrh , where r is the radius of the bases and h is the height.
Solve for h.
Answers
Answer:
Solving for h gives us:
\(h = \frac{S}{2\pi r}\)
Step-by-step explanation:
Given that
Lateral surface area of cylinder = S = \(2\pi rh\)
Solving the equation or formula for a particular variable involves isolating the variable on one side of the equation without any factor or denominator.
In the given formula:
Dividing both sides by 2πr
\(\frac{S}{2\pi r} = \frac{2\pi rh}{2\pi r}\\h = \frac{S}{2\pi r}\)
Hence,
Solving for h gives us:
\(h = \frac{S}{2\pi r}\)
Related Questions
14th term of geometric sequence 10,20,40
Answers
Explanation: The general formula for nth term in a geometric sequence is an=a1*r^(n-1), where a1 is the first term, r is the common ratio, and n is the position in the term (in this case 14).
To find the common ratio, we can simply divide the next term after the first (20/2=10).
So, an=10*2^(14-1).
After doing the parentheses and multiplication, we have 20^13, which is 81920.
PLZ HELP ASAP ITS KHAN WILL GIVE 5 STARS AND HEART AND 10 POINTS AND BRAINLIST

Answers
Answer:
14.4
Step-by-step explanation:
Cross multiply.
5x = 8 * 9
5x = 72
x = 14.4
Answer:
x= 14.4
Step-by-step explanation:
1. cross multiply
5*x and 8*9
5x=72
2. divide 72 by 5
x= 14.4
what is the difference between the amount of money jill has and the amount of money Mark has?
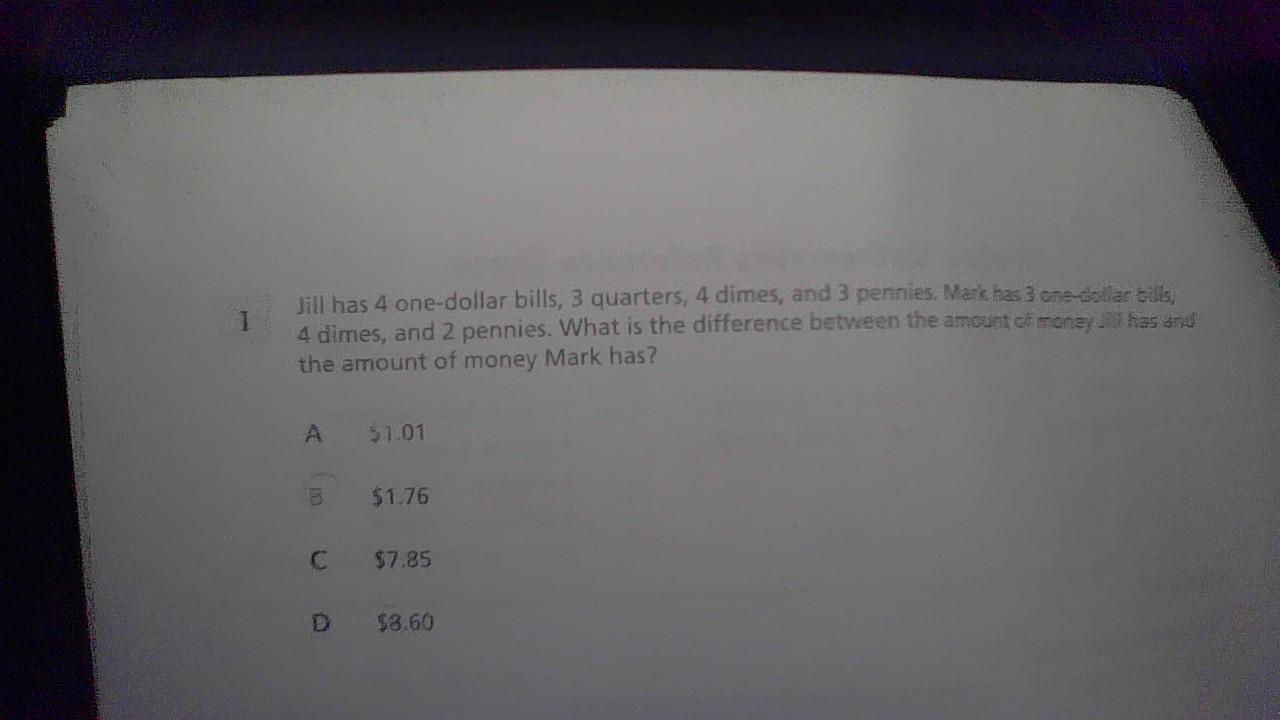
Answers
Answer:
Step-by-step explanation:
jill has 4 one dollar bills, and Mark has 3 one dollar bills so 3 of them would cancel out, so jill would have one more dollar. And then we also know that Jill has 4 dimes, and Mark also has 4 dimes. So they cancel out. And Jill has 3 pennies, and Mark has 2, so Mark has one more penny than Mark. And lastly we know that Jill has 3 quarters. So know we add it all up Jill has 1 dollar, 3 quarters and 1 penny. Which is $1.76.
Answer:
Step-by-step explanation:
Jill's money:
4 one dollar bills- 4 x 1 = 4
3 quarters: 3 x 0.25 = 0.75
4 dimes: 4 x 0.10 = 0.4
3 pennies: 3 x 0.01 = 0.03
4 + 0.75 + 0.4 + 0.03 = $5.18
Jill has $5.18
Mark's Money:
3 one dollar bills: 3 x 1 = 3
4 dimes: 4 x 0.10 = 0.4
2 pennies: 2 x 0.01 = 0.02
3 + 0.4 + 0.02 = $3.42
Mark has $3.42
To find the difference between these values, we subtract.
5.18 - 3.42 = $1.72
The difference between Jill's money and Mark's money is $1.72
$1.72 is your answer.
What is the slope of the line graphed below?

Answers
Answer:
A
Step-by-step explanation:
Use rise over run. The graph rises by 9 units between the 2 points and runs 3 units on the x axis.
Rise/ run = \(\frac{9}{3}\), which can be simplified to 3.
The slope is 3.
Answer:
3(A)
Step-by-step explanation:
To find the slope, you have to find the difference of the two y values divided by the difference of the two x values. The equation for that would be y₁ - y₂ divided by x₁ - x₂. Let's say for this problem (x₁, y₁) is (3,3) and (x₂, y₂) is (0, -6). (It has a subscript just to show that they are different x and y values)
We can then substitute these values into the equation.
y₁ - y₂ divided by x₁ - x₂ would be 3 - (-6) divided by 3-0
This would then be simplified into
3+6 (2 negatives make a positive) divided by 3
This equals 9/3 which equals 3 as the slope.
6.00+1.50(m)=26.25 find what m is
Answers
Answer:
13.5
Step-by-step explanation:
first let's subtract 6.00 from 6.00 and 26.25
26.25-6=20.25
1.50(m)=20.25
so we need to get rid of the 1.50
so 1.50m divided by 1.50=m
20.25÷1.50=13.5
m=13.5 hope this helps
NEED DONE ASAP! A fence must be built to enclose a rectangular area of 20,000 ft^2. Fencing material costs $4 per foot for the two sides facing north and south and $8 for the other two sides. Find the cost of the least expensive fence.

Answers
Answer:
Step-by-step explanation:
To find the cost of the least expensive fence, we need to determine the dimensions of the rectangular area that minimize the cost. Let's assume the length of the rectangle is L and the width is W.
The area of a rectangle is given by the formula: A = length × width.
In this case, we have A = 20,000 ft².
So, L × W = 20,000.
To minimize the cost, we need to minimize the perimeter of the rectangle since that's where the cost of the fence lies.
The perimeter of a rectangle is given by the formula: P = 2(length + width).
In this case, we have P = 2(L + W).
To find the least expensive fence, we need to minimize the cost, which is the sum of the costs of the four sides of the fence.
Cost = (2 sides × $4/ft) + (2 sides × $8/ft)
Cost = 8(L + W) + 16(L + W)
Cost = 24(L + W)
Now we can substitute L × W = 20,000 into the cost equation:
Cost = 24(L + W)
Cost = 24(20000/W + W)
To minimize the cost, we need to find the value of W that minimizes the equation. We can do this by finding the derivative of the cost equation with respect to W and setting it equal to zero. However, this calculation is beyond the scope of this text-based interface.
So, we can solve this problem by trial and error or using mathematical software to find the values of L and W that minimize the cost.
Once we have the values of L and W, we can calculate the cost using the equation:
Cost = 24(L + W).
Without knowing the specific values of L and W, we cannot calculate the exact cost of the least expensive fence.
Use the Law of Sines to find the missing angle of the triangle.
Find m∠B to the nearest tenth.
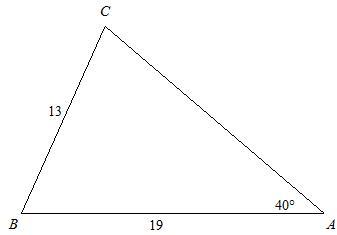
Answers
Answer:
m∠B = 70.0° (nearest tenth)
Step-by-step explanation:
Sine Rule for Angles
\(\sf \dfrac{\sin A}{a}=\dfrac{\sin B}{b}=\dfrac{\sin C}{c}\)
(where A, B and C are the angles and a, b and c are the sides opposite the angles)
Given:
a = 13c = 19A = 40°Substituting the given values into the formula to find m∠C:
\(\implies \sf \dfrac{\sin 40^{\circ}}{13}=\dfrac{\sin C}{19}\)
\(\implies \sf \sin C=\dfrac{19\sin 40^{\circ}}{13}\)
\(\implies \sf C=\sin^{-1}\left(\dfrac{19\sin 40^{\circ}}{13}\right)\)
\(\implies \sf m \angle C=69.96086904^{\circ}\)
Interior angles of a triangle sum to 180°
\(\implies \sf m \angle A+ m \angle B+m \angle C=180^{\circ}\)
\(\implies \sf 40^{\circ} + m \angle B+69.960...^{\circ}=180^{\circ}\)
\(\implies \sf m \angle B=70.03913...^{\circ}\)
Therefore, m∠B = 70.0° (nearest tenth)
Let X denote the number of paint defects found in a .square yard section of a car body painted by a robot. These data are obtained: 8 5 0 10 0 3 1 12 2 7 9 6 Assume that X has a Poisson distribution with parameter lambda s. Find an unbiased estimate for lambda s. Find an unbiased estimate for the average number of flaws per square yard. Find an unbiased estimate for the average number of flaws per square foot.
Answers
To find an unbiased estimate for lambda s, we can use the sample mean as an estimate for the parameter. The sample mean is calculated by adding up all the observed values of X and dividing by the number of observations.
In this case, we have:
Sample mean = (8+5+0+10+0+3+1+12+2+7+9+6)/12 = 5.5
Therefore, an unbiased estimate for lambda s is 5.5.
To find an unbiased estimate for the average number of flaws per square yard, we simply use the same estimate as above since lambda s represents the average number of flaws per square yard.
Thus, an unbiased estimate for the average number of flaws per square yard is also 5.5.
Learn more about :
unbiased estimate : brainly.com/question/30982574
#SPJ11
Find the volume of a
cone with a radius of 3
cm and a height of
5 cm
Answers
Answer:
V≈47.12cm³
Step-by-step explanation:
i really hope this helps
What's 0.32*10^-12 in scientific notation
Answers
Answer:
3.2 × 10^-13
Step-by-step explanation:
Henry is going to invest in an account paying an interest rate of 2.5% compounded continuously. How much would henry need to invest for the value of the account to reach $690 in 6 years?
Answers
Answer:
$595.34
Step-by-step explanation:
Step one
given data
interest rate of 2.5%= 0.025
final amount= $690
time = 6 years
Required
Principal P
Step two
The compound interest formula is
\(A= P(1+r)^t\)
substitute
\(690=P(1+0.025)^6\\\\690=P(1.025)^6\\\\690=P1.159\\\\\)
divide both sides by 1.159
P=690/1.159
P= $595.34
Answer:
590
Step-by-step explanation:
Answer from delta math

Write each of the following in the simplest exponential form please help

Answers
Answer:
Step-by-step explanation:
\(a^{m}*a^{n} =a^{m+n}\\\\\\2^{3}*2^{5} = 2^{3+5} =2^{8}\\\\\\3^{2}*3^{4}=3^{2+4} = 3^{6}\\\\\\a^{2}*a^{5}=a^{2+5}=a^{7}\)
Which expression is equivalent to n2 + 26n + 88 for all values of n?
(n + 8)(n + 11)
(n + 4)(n + 22)
(n + 4)(n + 24)
(n + 8)(n + 18)
Answers
Answer:
(n + 4)(n+ 22)
Step-by-step explanation:
One way to determine which factored expression is accurate is by asking the question: "Which two numbers multiply to 88, but add to 26?" If you are having difficulty using this method, you can always try the guess-and-check method with a multiple choice question. This way involves multiplying the terms inside one set of parentheses with the terms inside the other set of parentheses.
Step #1: Multiply the first terms
(n + 4)(n + 22) ----> n x n = n²
Step #2: Multiply the inside terms
(n + 4)(n + 22) -----> 4 x n = 4n
Step #3: Multiply the outside terms
(n + 4)(n + 22) -----> n x 22 = 22n
Step #4: Multiply the last terms
(n + 4)(n + 22) -----> 4 x 22 = 88
Step #5: Write the new expanded equation and simplify
(n + 4)(n + 22) -----> n² + 4n + 22n + 88 -----> n² + 26n + 88
A fair coin is tossed 10 times. If is the number of times that heads is tossed, what is P(3<≤6)?
Answers
The probability of P(3 < X ≤ 6) is approximately 0.6575.
What is probability?The study of probabilities, which are determined by the ratio of favourable occurrences to probable cases, is known as probability.
To find P(3 < X ≤ 6), where X represents the number of times heads is tossed when a fair coin is tossed 10 times, we need to calculate the probability of obtaining more than 3 but less than or equal to 6 heads.
Since the coin is fair, the probability of getting heads on any single toss is 0.5, and the probability of getting tails is also 0.5.
We can use the binomial probability formula to calculate the probability for a specific number of heads in a given number of coin tosses:
P(X = k) = (n choose k) * \(p^k\) *\((1-p)^{(n-k)\),
where n is the number of trials, k is the number of successful outcomes, p is the probability of success on each trial, and (n choose k) is the binomial coefficient.
In this case, n = 10 (10 coin tosses), p = 0.5 (probability of heads), and we want to calculate the probability for 4, 5, and 6 heads.
P(3 < X ≤ 6) = P(X = 4) + P(X = 5) + P(X = 6)
Using the binomial probability formula, we can calculate these probabilities:
P(X = 4) = (10 choose 4) * \((0.5^4) * (0.5^6)\) = 210 * 0.0625 * 0.015625 = 0.2063
P(X = 5) = (10 choose 5) * \((0.5^5) * (0.5^5)\) = 252 * 0.03125 * 0.03125 = 0.2461
P(X = 6) = (10 choose 6) * \((0.5^6) * (0.5^4)\) = 210 * 0.015625 * 0.0625 = 0.2051
Finally, we can calculate the desired probability:
P(3 < X ≤ 6) = P(X = 4) + P(X = 5) + P(X = 6) = 0.2063 + 0.2461 + 0.2051 = 0.6575
Therefore, P(3 < X ≤ 6) is approximately 0.6575.
Learn more about probability on:
https://brainly.com/question/13604758
#SPJ4
the number of toy cars that ray has is a multiple of . when he loses two of them, the number of cars that he has left is a multiple of . if is a positive even integer less than , then how many possible values are there for ?
Answers
we need to find the positive even integers less than k that satisfy the condition nx divided by x leaves a remainder of 2.
To solve this problem, we need to use the information given and work step by step. Let's break it down:
1. The number of toy cars that Ray has is a multiple of x. This means the number of cars can be represented as nx, where n is a positive integer.
2. When Ray loses two cars, the number of cars he has left is a multiple of x. This means (nx - 2) is also a multiple of x.
3. If x is a positive even integer less than k, we need to find the possible values for x.
Now, let's analyze the conditions:
Condition 1: nx - 2 is a multiple of x.
To satisfy this condition, nx - 2 should be divisible by x without a remainder. This means nx divided by x should leave a remainder of 2.
Condition 2: x is a positive even integer less than k.
Since x is even, it can be represented as 2m, where m is a positive integer. We can rewrite the condition as 2m < k.
To find the possible values for x, we need to find the positive even integers less than k that satisfy the condition nx divided by x leaves a remainder of 2. The number of possible values for x depends on the value of k. However, without knowing the value of k, we cannot determine the exact number of possible values for x.
To know more about remainder visit:
brainly.com/question/29019179
#SPJ11
solve the system of equations by graphing. y=4x+2 y=7/2x+1 plz help
Answers
Answer:
LOL i belive its 200 because i did this exact same thing yesterday for homework and got it right so yeah i guess it is?
Step-by-step explanation:
i used to describe a food item having a spicy or salty quality without being sweet?
Answers
Answer:
UMAMI I think may be the answer to your question
please help with algebra 2 question! arithmetic sequence
erica is playing a card game with her friend marquis. her points are shown in the following table:
round 1: 30 pts
round 2: 42 pts
round 3: 58.8 pts
marquis’s points in each round are represented by the following sequence:
50, 75, 112.5,…
in the table below, determine whether each row applies to eeica’s points or marquis’s points. select erica, marquis, or neither option.
(answers attached in pic) pls help!

Answers
Answer:
neitherMarquisEricaMarquisStep-by-step explanation:
The matching of exponential functions to table values involves matching both initial values and rate of growth. The value of a geometric series can be calculated from its formula, or can be shown by a calculator or spreadsheet.
Function determinationThe starting values of the two sequences are different, as are all of the starting values of the answer choices. Matching starting values is sufficient to identify which sum goes with which player.
The series ...
\(\displaystyle S=\sum_{k=1}^n{AB^{k-1}}\)
gives the sum of a geometric sequence with first term A and common ratio B.
The first term of Erica's series is A=30. The ratio is B=42/30 = 1.4.
The first term of Marquis's series is 50. The ratio is B=75/50 = 1.5.
Using these values in the series formula, the total score after n rounds will be ...
\(\displaystyle \text{Erica:}\quad \sum_{k=1}^n{30(1.4)^{k-1}}\qquad\text{matches Row 3}\\\\\text{Marquis:}\quad \sum_{k=1}^n{50(1.5)^{k-1}}\qquad\text{matches Row 2}\)
Total scoreThe explicit expression for these sums is ...
\(S=A\dfrac{B^n-1}{B-1}\)
Then the total scores after 6 rounds are ...
Erica: 30(1.4^6 -1)/0.4 = 489.7152
Marquis: 50(1.5^6 -1)/0.5 = 1039.0625 . . . . . matches row 4
TableThen the required table gets filled in ...
neitherMarquisEricaMarquis__
Additional comment
The values in the attached table are total scores after each round. One must subtract the previous round's total to find the points scored in a given round.
The purpose of showing totals is to permit matching the value seen in Row 4 of the table.

if each day you work is 8 hrs & u work for 121 days how much hrs would that be
Answers
HELP!
Find the measure for
A)
127°
B)
131°
C)
135°
D)
139°

Answers
Answer:
B) 131°
Step-by-step explanation:
-4a + 139 = 4a + 123 because they are vertical angles
-4a + 139 = 4a + 123
reduce:
8a = 16
a = 2
4(2) + 123 = 131°
Teddy has two sisters. One of them is 3 years younger than Teddy, the other one is 7 years younger than her only brother. How old was the older sisters when the younger sister was born?
Answers
Given:
Teddy has two sisters. One of them is 3 years younger than Teddy, the other one is 7 years younger than her only brother.
To find:
The age of older sister at the time when the younger sister was born.
Solution:
Let the age of Teddy be x.
One sister is 3 years younger than Teddy.
Age of one sister = x-3 years
The other one is 7 years younger than her only brother. It means other sister is 7 years younger than Teddy.
Age of other sister = x-7 years
Clearly (x-3) > (x-7). So, difference between the ages of sisters is
\(Difference=(x-3)-(x-7)\)
\(=x-3-x+7\)
\(=4\)
Therefore, the age of older sister is 4 years when the younger sister was born.
Answer:
d
Step-by-step explanation:
Which of the following is not a rate?
I WILL GIVE BRAINLIEST IF YOUR ANSWER IS RIGHT

Answers
Answer:
540 feet 5 feet
Step-by-step explanation:
they're both feet, it cant be a rate.
A regular octagon is formed by cutting an isosceles right triangle from each of the corners of a square with sides of length 2000. What is the length of each side of the octagon
Answers
A regular octagon is formed by cutting an isosceles right triangle from each of the corners of a square. To find the length of the side of the octagon, we can use the Pythagorean theorem to calculate the length of the hypotenuse of the isosceles right triangle.
In an isosceles right triangle, the two legs have the same length and the hypotenuse is the side opposite the right angle. Since the square has sides of length 2000, half of the side of the square is the leg of the isosceles right triangle.
So the leg of the isosceles right triangle is 2000/2 = 1000.
Applying the Pythagorean theorem to find the hypotenuse of the isosceles right triangle:
c^2 = a^2 + b^2
c = sqrt(a^2 + b^2)
c = sqrt(1000^2 + 1000^2)
c = sqrt(1000000)
c = 1000*sqrt(2)
So the length of each side of the octagon is 1000sqrt(2)
in which quadrant is point (-4,5) located?
Answers
First quadrant (positive, positive)
Second quadrant (negative, positive)
Third quadrant (negative, negative)
Fourth quadrant (positive, negative)
Solve the equation.
-9x + 1=-X+17
Ox=-8
Ox=-2
Ox=2
Ox=8
Answers
Answer:
x = -2
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightAlgebra I
Equality PropertiesStep-by-step explanation:
Step 1: Define
-9x + 1 = -x + 17
Step 2: Solve for x
Add 9x on both sides: 1 = 8x + 17Subtract 17 on both sides: -16 = 8xDivide 8 on both sides: -2 = xRewrite: x = -2A music group expects to sell a new compact disc (CD) at the rate R(t)=20,000e−0.12t CDs per week, where t denotes the number of weeks since the CD was first released. To the nearest thousand, how many CDs are expected to be sold during the first 12 weeks after the release?
Answers
Answer:
4739 CDs
Step-by-step explanation:
Given the function that models the rate at which the disc is sold expressed as;
R(t)=20,000e^−0.12t
t is the number of weeks since the CD was first released
We are to look for the number of CDs that are expected to be sold during the first 12 weeks after the release. To do this, you will simply substitute t = 12 into the modeled function and get R(12) as shown;
R(t)=20,000e^−0.12t
R(12)=20,000e^−0.12(12)
R(12)=20,000e^−1.44
R(12)=20,000(0.2369)
R(12) = 4738.56
Hence the total number of CDs expected to be sold to nearest thousand is 4739 CDs
Answer:
127,000
Step-by-step explanation:
you take the integral from 0 to 12 of R(t)
1 4x2 + 4x + 2 dx = P arctan(ax + b) + c, where p and q have only 1 as common divisor with P 9 p=
Answers
The given integral ∫(4x^2 + 4x + 2) dx can be evaluated to obtain an expression in the form P arctan(ax + b) + c, where P, a, b, and c are constants. The common divisor of P and q is 1, and the value of P is 9.
In the given expression, the integral of 4x^2 is (4/3)x^3, the integral of 4x is 2x^2, and the integral of 2 is 2x. Summing up these integrals, we get (4/3)x^3 + 2x^2 + 2x + C, where C is the constant of integration.
To express this in the form P arctan(ax + b) + c, we need to manipulate the expression further. We can rewrite (4/3)x^3 + 2x^2 + 2x as (4/3)x^3 + (6/3)x^2 + (6/3)x, which simplifies to (4/3)x^3 + (6/3)(x^2 + x).
Comparing this with the form arctan(ax + b), we can see that a = √(6/3) and b = 1. Therefore, the expression becomes 9 arctans (√(6/3)x + 1) + C, where C is the constant of integration.
Learn more about common divisor here: brainly.com/question/13257989
#SPJ11
If a/2 =b/3, What is the value of 6a-4b ?
a. 0
b. 1
c. 2
d. 3
Answers
Answer:
A
Step-by-step explanation:
6 (2) - 4 (3)
12 - 12
0
hope this helps you
Frank measured 56 cm. The actual length was 61 cm. Which expression shows his percent error?
Answers
Answer: 8.54701% difference
Step-by-step explanation: (61-56)/[(61+56)/2] x 100= 8.55%
answerrrrrr plssss ill giveee brainliesttttt

Answers
\(m\angle E=\sin \dfrac{\sqrt{10}}{2\sqrt5}=\sin \dfrac{\sqrt2}{2}=45^{\circ}\)