PLS HELP 15 POINTS!! Which two points on the number line represent numbers that can be combined to make zero?
B and D
A and B
C and D
A and C
Answers
Related Questions
Tower A and B are 151 m apart.
From the top of tower A at 68 m above ground, the angle of elevation to the top of tower B is 23°.
How tall is tower B to the nearest metre?

Answers
Answer:
132m
Step-by-step explanation:
Height of tower B = Height of tower A + difference in height between tower B and A
The difference in height can be determined using tan
tan 23 = opposite / adjacent
tan 23 = opposite / 151
0.4245 x 151 = opposite
opposite = 64m
Height of tower B = 64m + 68m = 132m
Write down two factors of 24 that are primenumber
Answers
the prime factors of 24 are 2 and 3, which combine to give the unique prime factorization of 24 as 2^3 × 3.
There are no factors of 24 that are prime numbers. A factor of a number is a whole number that divides that number without leaving a remainder. Prime numbers, on the other hand, are numbers that are divisible only by 1 and themselves, and cannot be expressed as the product of any other numbers.
The prime factors of 24 are 2, 2, and 3. We can factorize 24 as 2 × 2 × 2 × 3 or 2^3 × 3. Here, 2 and 3 are both prime numbers, but they are not factors of 24 in isolation. They are only prime factors of 24 when combined in the manner shown.
This fact highlights an important concept in number theory: the uniqueness of prime factorization. Every composite number can be expressed as a unique product of prime numbers. This fundamental theorem of arithmetic is crucial in many areas of mathematics, including cryptography, where it is used to secure communications and protect sensitive information.
In summary, there are no factors of 24 that are prime numbers. However, the prime factors of 24 are 2 and 3, which combine to give the unique prime factorization of 24 as 2^3 × 3.
To know more about prime factorization click here:
brainly.com/question/29763746
#SPJ4
the hypotenuse of a right angle is 26 ft one leg is 10 fr find the length of the other leg in feet
Answers
If the hypotenuse of a right angle is 26 ft one leg is 10 ft, and the length of the other leg is 24 feet.
What is a right-angle triangle?It is a triangle in which one of the angles is 90 degrees and the other two are sharp angles. The sides of a right-angled triangle are known as the hypotenuse, perpendicular, and base.
It is given that, the hypotenuse of a right angle is 26 ft one leg is 10 ft.
We have to find the length of the other leg in feet,
To the Pythagoras theorem, the square of the hypotenuse in a right-angled triangle is equal to the sum of the squares of the other two sides.
h²=p²+b²
26²=10²+b²
b²=576
b=24
Thus, if the hypotenuse of a right angle is 26 ft one leg is 10 ft, and the length of the other leg is 24 feet.
Learn more about the right-angle triangle here:
brainly.com/question/3770177
#SPJ1
the screen on briannas new phone is 2.85 centimetres long what mixed number represents the length of the phone screen
Answers
2.85 as a mixed number would be \(2\frac{85}{100}\)
next, simpifly 85/100, by dividing by 5
85/5 = 17
10/5 = 20
The answer is \(2 \frac{17}{20}\)
I hope this helps, have a great day! <33
A boy who is flying a kite lets out 300 feet of string which makes an angle of 60o with the ground. Assuming that the string is stretched taut, find, to the nearest foot, how high the kite is above ground.
Answers
See the attachment for better understanding.
Given : Length of string (AC) = 300ft
To Find : Height of the kite from ground (AB)
\(:\implies\sf\:sin60^{\circ}=\dfrac{AB}{AC}\)
\(:\implies\sf\:\dfrac{\sqrt{3}}{2}=\dfrac{H}{300}\)
\(:\implies\sf\:H=\dfrac{300\sqrt{3}}{2}\)
\(:\implies\sf\:H=150\sqrt{3}\)
\(:\implies\:\boxed{\bf{\red{H\approx 260\:feet}}}\)
Hope it helps !

The kite is 260 ft. above ground.
What is right triangle?"It is a triangle whose one of the angle is 90"
What is sine of the angle?For right triangle the sine of the angle 'x' is,
sin(x) = opposite side of angle x / hypotenuse
For given example,
Consider the diagram given below.
KL represents the string length.
So, KL = 300 ft.
A string makes an angle of 60° with the ground.
This means, ∠KLM = 60°
Let, 'h' represents the height of of the kite above the ground.
For a right triangle KLM,
⇒ sin(KLM) = KM/KL
⇒ sin(60°) = h/300
⇒ h = sin(60°) × 300
⇒ h = 259.8 ft.
⇒ h ≈ 260 ft.
Therefore, the kite is 260 ft. above ground.
https://brainly.com/question/16853308
Learn more about sine here:
#SPJ2

1 distributive property for 9(413)2 distributive property for 6(593)
Answers
Answer:
9(413)2= 7434 and 6(593)=3558
Step-by-step explanation:
Hope this helps
What is the slope of the line?
3(y - 1) = 2x + 2
Answers
hope it helped
3(y-1)=2x+2
3(y-1)/3=2x+2/3
y-1=2x/3+2/3
y=2x/3+2/3+1
y=2x/3+5/3
m=2/3
marcio is playing a video game. the number of minutes, x, marcio plays is related to the number of points, y, he earns. How many points does he earn per minute
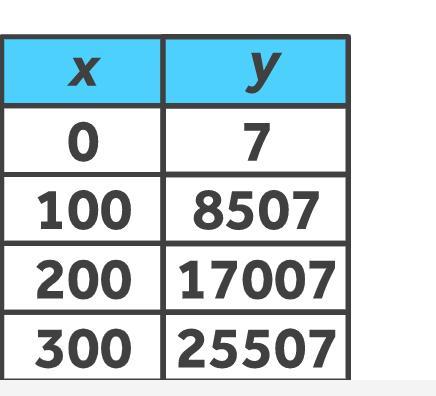
Answers
Answer:
85 points per minute
Step-by-step explanation:
the points earned per minute is calculated as
\(\frac{y_{2}-y_{1} }{x_{2}-x_{1} }\)
with (x₂, y₂) = (200, 17007) and (x₁, y₁ ) = (100,8507) ← 2 ordered pairs from table
points earned per minute = \(\frac{17007-8507}{200-100}\) = \(\frac{8500}{100}\) = 85
25% of what number equals 10?
Answers
Answer:
40%
Step-by-step explanation:
mark branliest
Calculate derivatives f'(x) (unsimplified) for (a) f(x) = (x^2 + e^x - ln x)^4 (b) f(x) = x(x^2 + 1)/x^3 - x
Answers
The derivatives of the functions are :
(a) 4(x² + eˣ - ln x)³ (2x + eˣ - 1/x)
(b) -2/x³ - 1
(a) To calculate the derivative of the function f(x) = (x^2 + e^x - ln x)^4, we can use the chain rule and the power rule.
First, we can take the derivative of the function inside the parentheses using the sum and difference rule:
f'(x) = 4(x^2 + e^x - ln x)^3 (2x + e^x - 1/x)
Next, we can simplify this expression by multiplying the two factors together and raising the entire expression to the fourth power:
f'(x) = 4(x² + eˣ - ln x)^3 (2x + eˣ - 1/x)
Therefore, the derivative of the function
f(x) = (x² + eˣ - ln x)⁴ is
f'(x) = 4(x² + eˣ - ln x)³ (2x + eˣ - 1/x)
(b) To calculate the derivative of the function f(x) = (x(x² + 1)/x³) - x, we can use the quotient rule and the product rule.
First, we can simplify the expression inside the parentheses by multiplying x through:
f(x) = (x³ + x)/x³ - x
Next, we can take the derivative of this expression using the product rule and the power rule:
f'(x) = [x³(3x² + 1) - (x³ + x)3x²]/ x⁶ - 1
Simplifying this expression, we get:
f'(x) = [3x⁵ + x³ - 3x⁵ - 3x³]/ x⁶ - 1
= -2/x³ - 1
Therefore, the derivative of the function
f(x) = x(x² + 1)/x³ - x is
f'(x) = -2/x³ - 1
To know more on derivatives
https://brainly.com/question/29255160
#SPJ4
Wilson Green sells home security systems. He earns an 13% commission on every system he sells. Wilson's sales for this month totaled $6425. What is Wilson's commission? *
Answers
Answer
$835,25
Explanation
Total sales = $6425
Commission = 13%
100% - $6425
13% - x
13 * 6425 = 83525
83525 \ 100 = 835,25
6 + 3 • 4 = please hurry
Answers
Answer:
18
Step-by-step explanation:
PEMDAS
3x4=12
12+6=18
Hope this helps.
30. In the figure below, rectangle ABCD shares CD with ACDE,
diagonal BD of the rectangle extends in a straight line
beyond D to E to create DE, and the measure of CDE is
155°.
What is the measure of ZCBD?
F. 25
G.55
H.65
J.90
K.155

Answers
The measure of angle ZCBD is 90°. Therefore, the correct option is J. 90.
To find the measure of angle ZCBD, we need to examine the given information.
From the figure, we know that angle CDE is 155°.
Since the opposite angles of a rectangle are congruent, angle BCD is also 155°.
In a rectangle, the sum of the interior angles at a vertex is always 90°.
Therefore, angle CBD is 90°.
Hence, the measure of angle ZCBD is 90°.
Therefore, the correct option is J. 90.
for such more question on measure of angle
https://brainly.com/question/25716982
#SPJ8
Lines and are parallel lines cut by a transversal. Use the lines and the angles formed to select all that are true statements. Select 3 correct answer(s)
a. Angle 3 and Angle 6 are vertical angles.
b. Angle 1 and Angle 5 are corresponding angles.
c. Angle 6 and Angle7 are vertical angles.
d. Angle 7 and angle 8 are supplementary angles.
e. Angle 2 and angle 4 are alternate interior angles.
f. Angle 1and angle 4 are same side interior angles.
Answers
The corrrect statements about angle are:
Angle 1 and angle 5 are corresponding anglesAngle 6 and angle 7 are vertical anglesAngle 7 and angle 8 are supplementary anglesPlease refer to the attached picture below for visualization of the angles.
Based on the attached picture, we can consider the relationship of each angle, such as:
Alternate interior angles and congruent:
Angle 4 and angle 5
Angle 3 and angle 6
Alternate exterior angles and congruent:
Angle 1 and angle 8
Angle 2 and angle 7
Consecutive interior angles and supplementary:
Angle 3 and angle 5
Angle 4 and angle 6
Corresponding angles and congruent:
Angle 1 and angle 5
Angle 2 and angle 6
Angle 3 and angle 7
Angle 4 and angle 8
Vertical angles and congruent:
Angle 1 and angle 4
Angle 2 and angle 3
Angle 5 and angle 8
Angle 6 and angle 7
Supplementary angles:
Angle 1 and angle 2
Angle 3 and angle 4
Angle 5 and angle 6
Angle 7 and angle 8
Learn more about Angle here: brainly.com/question/28451077
#SPJ4

how many ways are there to seat six people around a circular table where two seating's are considered the same when everyone has the same two neighbors without regard to whether they are right or left neighbors? note: 6!
Answers
Therefore, the number of combination to seat 6 people around a circular table where two seating are considered the same when everyone has the same two neighbors is 20.
When seating around a circular table, there are (n-1)! ways to seat n people. However, in this case, we need to divide by 2 since we're counting identical arrangements twice. Additionally, each seating can be rotated 6 times, so we need to divide by 6 to get rid of the redundancies.
Therefore, the number of ways to seat 6 people around a circular table where two seating are considered the same when everyone has the same two neighbors is:
(6-1)! / (2 x 6) = 5! / 12
= 60 / 12
= 5 * 4
= 20
To know more about combination,
https://brainly.com/question/20211959
#SPJ11
The equation 3xy = 9 is a linear equation.
Group of answer choices:
True or False
Answers
Linear equations are a subset of non-linear equations, and the equation 3xy = 9 is a non-linear equation.
The equation 3xy = 9 is not a linear equation. It is a non-linear equation. Linear equations are first-degree equations, meaning that the exponent of all variables is 1. A linear equation is represented in the form y = mx + b, where m and b are constants.
The variables in linear equations are not raised to powers higher than 1, making it easier to graph them. In contrast, non-linear equations are any equations that cannot be written in the form y = mx + b. Non-linear equations have at least one variable with an exponent that is greater than or equal to 2. Non-linear equations are harder to graph than linear equations.
The answer is false, the equation 3xy = 9 is a non-linear equation, not a linear equation. Non-linear equations are any equations that cannot be written in the form y = mx + b. They have at least one variable with an exponent that is greater than or equal to 2.
Linear equations are a subset of non-linear equations, and the equation 3xy = 9 is a non-linear equation.
To know more about Linear visit:
brainly.com/question/31510530
#SPJ11
solving right triangle find the missing side. round to the nearest tenth number 14

Answers
To find the missing value we can us the sine function:
\(\sin \theta=\frac{\text{opp}}{\text{hyp}}\)in this case the missing value x is the hypotenuse, the opposite leg is 35 and the angle is 45°, then we have:
\(\begin{gathered} \sin 45=\frac{35}{x} \\ x=\frac{35}{\sin 45} \\ x=42.4 \end{gathered}\)Then we have that x=42.4
if \(cosA=\frac{2}{5}\) and \(tanA\ \textless \ 0\) then \(sin(2A)=?\)
Please help
Absurd answers will not be tolerated
Answers
Answer:
\(\displaystyle \sin(2A)=\frac{-4\sqrt{21}}{25}\)
Step-by-step explanation:
We are given that:
\(\displaystyle \cos(A)=\frac{2}{5}\text{ and } \tan(A)<0\)
And we want to determine the value of:
\(\sin(2A)\)
First, since cos(A) is positive and tan(A) is negative, this means that ∠A must be in QIV.
In QIV, cosine is positive, sine is negative, and tangent is also negative.
The given ratio tells us that the adjacent side to ∠A is 2, and the hypotenuse is 5.
Then by the Pythagorean Theorem, the opposite side will be given by:
\((2)^2+o^2=(5)^2\)
Solve for the opposide side:
\(o=\sqrt{5^2-2^2}=\sqrt{21}\)
So, with respect to ∠A, the adjacent side is 2, the opposite side is √(21), and the hypotenuse is 5.
Using the double-angle identity, we can rewrite our original expression as:
\(\sin(2A)=2\sin(A)\cos(A)\)
Using the above information, substitute in values. Remember that cosine is positive and that sine is negative:
\(\displaystyle \sin(2A)=2\Big(-\frac{\sqrt{21}}{5}\Big)\Big(\frac{2}{5}\Big)\)
Simplify. Therefore:
\(\displaystyle \sin(2A)=\frac{-4\sqrt{21}}{25}\)
Answer:
sin 2A=2sin Acos A
=2×2/5×√21/5=±4√21/25
Step-by-step explanation:
cos A=2/5
B/H=2/5
corresponding value we get
B=2
H=5
by using Pythagoras law
H²=P²+B²
5²=P²+2²
P²=25-4
p=√21
now
sin A=P/H=√21/5
now
sin 2A=2sin Acos A=2×2/5×√21/5=±4√21/25
*Geometry* Need help, will give brainliest

Answers
Answer:
\(sinC = \frac{7}{25}\\~\\cosC = \frac{24}{25}\\~\\tanC = \frac{7}{24}\)
Step-by-step explanation:
To get the sine of a non-right angle C in a right triangle, just divide the side opposing C by the long side. To get the cosine, divide the sides at C (shorter by longer). To get the tangent, divide the sine by the cosine.
Let's go:
\(sinC = \frac{7}{25}\\~\\cosC = \frac{24}{25}\\~\\tanC = \frac{7}{25} / \frac{24}{25} = \frac{7}{24}\)
Answer:
sin(7/25), cos(24/25), tan(7/24)
Step-by-step explanation:
The sin, cos, and tan are found through the sides of the triangle.
The acronym SOH CAH TOA can be used to find which goes where.
S = sin
C = cos
T = tan
O = opposite
A = adjacent
H = hypotenuse
since it asks to use angle C you start from there, for sin its O/H hint by the SOH the rest are the same.
A model that describes the population of a fishery in which harvesting takes place at a constant rate is given by dP dt = kP − h, where k and h are positive constants. (a) Solve the DE subject to P(0) = P0. P(t) = (b) Describe the behavior of the population P(t) for increasing time in the three cases P0 > h/k, P0 = h/k, and 0 < P0 < h/k. P0 > h/k P(t) decreases as t increases P(t) increases as t increases P(t) = 0 for every t P(t) = P0 for every t P0 = h/k P(t) decreases as t increases P(t) increases as t increases P(t) = 0 for every t P(t) = P0 for every t 0 < P0 < h/k P(t) decreases as t increases P(t) increases as t increases P(t) = 0 for every t P(t) = P0 for every t (c) Use the results from part (b) to determine whether the fish population will ever go extinct in finite time, that is, whether there exists a time T > 0 such that P(T) = 0. If the population goes extinct, then find T. (If the population does not go extinct, enter DNE. )
Answers
The population will go extinct in finite time if P0 < h/k, and the time T for the population to go extinct can be found by solving the equation P(T) = 0.
The solution to the DE is \(P(t) = P0e^(kt-h)\). From this we can see that for P0 > h/k, the population decreases as t increases. This is because the exponential term \(e^(kt-h)\) is less than 1, so P(t) = P0e^(kt-h) decreases as t increases. For \(P0 = h/k, P(t) = P0e^(kt-h)\) is constant with respect to t, meaning the population remains the same. Finally, for 0 < P0 < h/k, P(t) = P0e^(kt-h) increases with t. This is because the exponential term e^(kt-h) is greater than 1, so \(P(t) = P0e^(kt-h)\) increases as t increases. Therefore, the population will go extinct in finite time if P0 < h/k, since the population will continue to increase until it reaches 0. The time T for the population to go extinct can be found by solving the equation P(T) = 0, which gives T = (h - ln(P0))/k.
Learn more about equation here:
https://brainly.com/question/10413253
#SPJ4
suppose you take a multiple choice test of 100 questions. each question has 5 possible answers (only one of which is correct) and you select one answer for each question randomly. what is the standard deviation of the number of questions you get correct?
Answers
Answer:
20
Step-by-step explanation:
So there is a total of 1/5 chance of getting an answer right, because there are 5 possibilities and only 1 is correct.
Now because there are 100 Questions, we will multiply our probability with the total value (in this case its a 100).
So 1/5 = .2
.2 × 100 = 20
The standard deviation of the number of questions answered correctly is 4. This means that on average, the number of questions answered correctly will be within 4 of the expected value.
The standard deviation of the number of questions answered correctly in a multiple-choice test of 100 questions with 5 possible answers for each question, assuming random selection of one answer per question, can be calculated using the formula for the binomial distribution, which is the square root of n times p times q, where n is the total number of trials, p is the probability of success on each trial, and q is the probability of failure on each trial.
Using the formula for the binomial distribution, the standard deviation of the number of questions answered correctly can be calculated as follows:
n = 100 (total number of trials)
p = 1/5 (probability of success on each trial, i.e., selecting the correct answer)
q = 4/5 (probability of failure on each trial, i.e., selecting one of the incorrect answers)
Therefore, the standard deviation is:
√(100 x 1/5 x 4/5) = √16 = 4
Therefore, the standard deviation of the number of questions answered correctly is 4. This means that on average, the number of questions answered correctly will be within 4 of the expected value.
To learn more about standard deviation here:
brainly.com/question/23907081#
#SPJ11
There is a slide in a park. One of its side walls has been painted in some colour with a message "KEEP THE PARK GREEN AND CLEAN” (see Fig. 12.10 ). If the sides of the wall are 15 m, 11 m and 6 m, find the area painted in colour.
Answers
Answer:
I don't know yujvhkjghjghhhgg
2.2f+0.3f−13−4=
Pls hurry!
Answers
Answer:
-14.5
Step-by-step explanation:
To get the calculator and type 2.2+0.3-13-4
One firefly flashes every 8 seconds. Another firefly flashes every 10 seconds. Both fireflies just flashed. After how many second will both fireflies flash at the same time again? (I know it's somehow related to LCM, GCF, Prime factorization)
Answers
Answer:
Step-by-step explanation:
You're correct that this problem involves finding the least common multiple (LCM) of 8 and 10, which will give us the number of seconds after which both fireflies will flash at the same time again.
To find the LCM of 8 and 10, we can use their prime factorizations:
8 = 2^3
10 = 2 × 5
The LCM of 8 and 10 is the product of the highest powers of all the prime factors involved, which in this case are 2^3 and 5. Therefore:
LCM(8, 10) = 2^3 × 5 = 40
So after 40 seconds, both fireflies will flash at the same time again. We can check this by dividing 40 by each of the individual flash intervals to see if we get a whole number:
40 ÷ 8 = 5
40 ÷ 10 = 4
Both of these divisions result in whole numbers, which confirms that 40 is the least common multiple of 8 and 10. Therefore, both fireflies will flash at the same time again after 40 seconds.
Answer:
40 sec
Step-by-step explanation:
So here we want to find the LCM of 8 and 10
➝ 8 = 2×2×2 = 2³
➝ 10 = 2×5
LCM(8,10) = 2³×5 = 40
So they will flash again after 40 sec.
DUE IN A FEW MINS PLEEEASE HELP QUICKLY TY
A bank offers two different types of savings account which pay interest as shown below. Hannah wants to invest £3200 in one of these accounts for 13 years. a) Which account will pay Hannah more interest after 13 years? b) How much more interest will that account pay? Give your answer in pounds (£) to the nearest 1p. Account 1 Simple interest at a rate of 5% per year Account 2 Compound interest at a rate of 4% per year
Answers
Part A: account 2 gives the more interest when Compounding.
Part A: account 2 give more interest of amount £1170.
Explain about the Simple interest?Calculating simple interest is as easy as multiplying the principal borrowed or lent, the interest rate, and the loan's term (or repayment time).
Given data:
Account 1 - Simple interest at a rate of 5% per year.
Account 2 - Compound interest at a rate of 4% per year.
Principal P - £3200
Time t - 13 years.
Part A:
SI = PRT/100
SI = 3200*4*13 / 100
SI = £1664
CI = A - P
CI = P\((1 + r)^{t}\) - P
CI = 3200\((1 + 0.05)^{13}\) - 3200
CI = 6034 - 3200
CI = £2834
Thus, account 2 gives the more interest when Compounding.
Part B:
Difference = CI - SI
Difference = 2834 - 1664
Difference = 1170
Thus, account 2 give more interest of amount £1170.
Know more about the Simple interest
https://brainly.com/question/25793394
#SPJ1
Simplify the variable expression: g - 16/4 + 2
Answers
Answer:
free points, thanks ✌
Step-by-step explanation:
convert the following measurements into
the indicated units
1.) 100 m^2
– as ft^2
2.) 2 km _____ ft
3.)2,057 grams - as kg
4.) 1.25 × 10-7 meters - as µm
5.) 6.58 × 104 meters - as km
6.) 2.78 × 10-1 grams - as mg
Answers
Answer:
1) 1076.4ft^2
2). 6561.68ft
3). 2.057kg
4). 1.25×10^-1
5). 6.58×10^1km
6). 278mg
z=1+4i
Find the angle 0(theta) in degrees that z makes in the complex plane.
Round your answer, if necessary, to the nearest tenth. Express 0(theta) between -180 and 180 degrees.
Answers
Answer:
76.0 degrees
Step-by-step explanation:
Just got it right on the Khan Academy quiz.
Find the measure of the indicated arc

Answers
Answer:
m(ABC) = 218°
Step-by-step explanation:
The measure of any arc with end points of an inscribed angle = 2 × inscribed angle
Inscribed angle = m<AMC = 109°
Intercepted arc = m(ABC)
Therefore,
m(ABC) = 2 × 109°
m(ABC) = 218°
Find the surface area
Please explain

Answers
Step-by-step explanation:
There are two trapezium(s), area of 2:
= 2 [½ x (sum of || sides) x height]
= 2[½ x (4 + 8) x 4.6 ] [not clearly visible if it is 4.5 or 4.6]
= 55.2 yd²
Area of vertically tilted rectangles
1): area = 5 x 4 = 20 yd²
2): area = 5 x 4 = 20 yd²
Total area = 20 + 20 = 40 yd²
Area of horizontal rectangles:
1): area = 4 x 8 = 32 yd² [upper]
2): area = 4 x 4 = 16 yd² [lower]
Area of given surface = 55.2 + 40 + 32 + 16 = 143.2 yd²