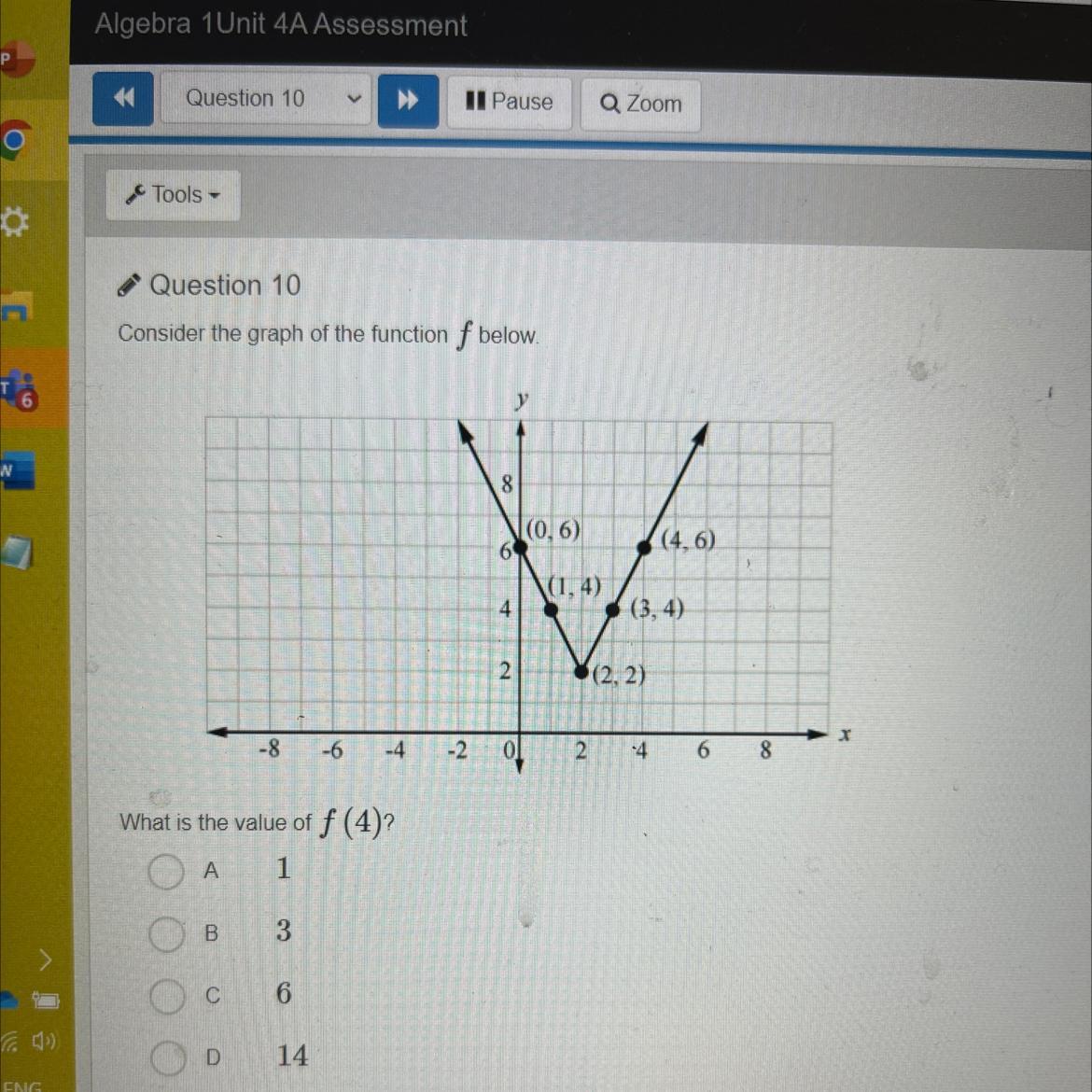
Answers
Using the graph of the function, the value of f(4) is 6.
How to find the value of f(4) of the graph?A function is an expression that shows the relationship between the independent variable and the dependent variable. A function is usually denoted by letters such as f, g, etc.
The set of inputs is called the domain of the function, and the set of outputs is called the codomain. A function maps each input to exactly one output, so it is also sometimes referred to as a mapping.
Using the graph, the value of f(4) can be found by checking for the value y when x = 4. Check the attached image.
Therefore, the value of f(4) is 6
Learn more about function on:
brainly.com/question/1415456
#SPJ1
Related Questions
A car dealership had a 45% increase in sales from June to August. If sales increased 25% from June to July, what was the
Increase in sales from July to August?
(A) 10%
(3) 12%
(C) 14%
(D) 16%
(E) 20%
Answers
Answer:
1.25x = 1.45, so x = 1.16
The correct answer is (D) 16%
Answer: D - 16%
Step-by-step explanation:
Pretend their first sale was 100 dollars. The 25% INCREASE makes that into 125 dollars. If you multiply that by 16% and add to 125, you get 145 dollars.
Multiply the original 100 dollars with the 45% increase, and you also get 145 dollars.
Therfore, D is correct.
On a map, 2 cm represents 30 mi. The actual distance between two towns is 75 mi. What is the distance between, the towns on the map? Show your work.
Answers
Answer:
The distance between the two towns would be 5 cm on the map.
Step-by-step explanation:
First, think of the 2cm and 30 mi as a ratio meaning that wherever you measure 2cm on the map with a ruler, it will represent 30 mi in the real life scale.
Then, with a bit of reasoning, we can logically state that if 2cm = 30 mi, then 4cm = 60mi (if you double the 2cm, the 30mi will also be doubled) and 1cm = 15mi (if you divide the 2cm by 2, 30mi will also be divided by 2). Therefore, 75mi ( which is 30mi + 30mi + 15mi) is as much 5cm (which is 4cm + 4cm + 1cm) on the map.
Records show that Oliver is typically 10–30 minutes late for his shift at work. The distribution for the minutes he is late forms a consistent pattern, which can be graphed as the given uniform density curve.
A uniform density curve is at y = startFraction 1 Over 20 EndFraction from 10 to 30.
What percentage of the time will Oliver arrive between 15 and 20 minutes late to work?
5%
16.7%
25%
50%
Answers
Answer:
I believe its C- 25%
Step-by-step explanation:
If you count how far it is from 15 to 20 (5) and divide that by 1/20 you should end up with 0.25. Turn that into a percent and you get 25%.
Let me know if Im wrong!
The percentage of the time will Oliver arrive between 15 and 20 minutes late to work is 25%. The correct option is 3.
What is density curve?A probability density function, also known as the density of a continuous random variable in probability theory, is a function whose value at any given sample in the sample space can be interpreted as providing a relative likelihood that the random variable's value will be equal to that sample.
The total area under the uniform density curve between 10 and 30 is equal to 1, which represents 100% of the time.
To find the percentage of the time that Oliver arrives between 15 and 20 minutes late, we need to find the area of the uniform density curve between 15 and 20 and then convert it to a percentage.
The width of the uniform density curve between 15 and 20 is 5 units (20 - 15 = 5), and the height is 1/20. Therefore, the area of this region is:
Area = width x height = 5 x (1/20) = 1/4
To convert this to a percentage, we multiply by 100:
Percentage = (Area/Total area) x 100% = (1/4) x 100% = 25%
Therefore, the answer is 25%.
For more details regarding density curve, visit:
https://brainly.com/question/18345488
#SPJ7
d is the median of set m. n is a positive integer. if set m contains only the numbers 37, 45, 7, 12, 21, 22, and n, then what is the value of d?
Answers
To find the median d of set M, we first need to arrange the numbers in ascending order and then determine the middle value. Set M contains the numbers 37, 45, 7, 12, 21, 22, and n. We know n is a positive integer.
First, arrange the known numbers: 7, 12, 21, 22, 37, 45. Next, consider the position of n in the sorted sequence:
1. If n ≤ 7, the sorted sequence becomes: n, 7, 12, 21, 22, 37, 45.
2. If 7 < n ≤ 12, the sorted sequence becomes: 7, n, 12, 21, 22, 37, 45.
3. If 12 < n ≤ 21, the sorted sequence becomes: 7, 12, n, 21, 22, 37, 45.
4. If 21 < n ≤ 22, the sorted sequence becomes: 7, 12, 21, n, 22, 37, 45.
5. If 22 < n ≤ 37, the sorted sequence becomes: 7, 12, 21, 22, n, 37, 45.
6. If 37 < n ≤ 45, the sorted sequence becomes: 7, 12, 21, 22, 37, n, 45.
7. If n > 45, the sorted sequence becomes: 7, 12, 21, 22, 37, 45, n.
Since there are 7 numbers in the set, the median d will be the 4th value. In all cases, the 4th value remains 21. Therefore, the value of d is 21.
To know more about ascending order refer here
https://brainly.com/question/31946606#
#SPJ11
Carson's favorite store is having a sale. All clothing is 40% off, and Carson has a coupon for another $10 off his purchase. He buys a sweater for $65.99. How much does Carson pay for the sweater after the discounts?
Answers
Answer:
First, we can calculate the discount from the sale. The sweater costs $65.99, and with a 40% discount, the price is reduced by:
$65.99 x 0.40 = $26.40
So the sale price of the sweater is:
$65.99 - $26.40 = $39.59
Next, we can apply Carson's $10 coupon to the sale price:
$39.59 - $10 = $29.59
Therefore, Carson pays $29.59 for the sweater after the discounts.
To calculate the total discount that Carson receives, we can first find the amount of the 40% discount on the sweater, which is:
40% of $65.99 = 0.4 x $65.99 = $26.40
Then, we can add the additional $10 coupon discount to get the total discount:
$26.40 + $10 = $36.40
Therefore, the total discount that Carson receives on the sweater is $36.40.
To find the final price that Carson pays after the discounts, we can subtract the total discount from the original price:
$65.99 - $36.40 = $29.59
Therefore, Carson pays $29.59 for the sweater after the discounts.
janet can make 4/5 of a necklace in 20 minutes. at this rate, how many necklaces, to the nearest tenth of a necklace, Can Janet make in 1 hour ?
Answers
Answer:
Step-by-step explanation:
Janet can make:
4/5 of a necklace in 20min
2(4/5)=8/5 ............in 40 min
3(4/5)=12/5 ............in 60 min( one hour)
12/5=2 2/5 necklaces in one hour
or 2.4n in one hour
Suppose that x and y vary inversely. Write a function that models the inverse variation.
x=-12 when y=4
Answers
y = -48 (1/x) is the variation equation.
How to write the function that models the relationship?A relationship between a set of values for one variable and a set of values for other variables is known as a variation.
One of the most fundamental concepts in mathematics is the idea of a function, in part because functions are a useful tool for describing and illuminating patterns.
given that
x and y vary inversely that means x = k 1/y or y = k 1/x.
so, x= k(1/y)
xy = k
from the question x=-12 when y=4
-12(4) = k
k = -48
now,
y = K (1/x)
y = -48 (1/x)
This is the variation equation.
Learn more about variation equations from here:
https://brainly.com/question/13998680
#SPJ4
Answer this “PROPERLY”
Answers
can you write an algorithm which utilize the recursive concept to calculate recursive_question.gif the function should look like algorithm( a, n ) { ....... }
Answers
An algorithm that uses recursion to calculate the function in the given image:
The Algorithmfunction algorithm(a, n):
if n == 0:
return a
else:
return algorithm(a, n-1) + 2 * n - 1
This algorithm defines a function algorithm that takes two arguments a and n.
In the event that n holds a value of zero, the function will yield the result a.
Subsequently, a recursive invocation ensues whereby the function calls itself using the parameters a and n-1. Additionally, the sum of 2 multiplied by n-1 is added to the resulting value. This process persists until the variable n attains a value of zero, which represents the juncture at which the ultimate outcome is yielded.
The algorithm can be implemented by invoking the function algorithm(a, n) using the desired values for "a" and "n" as input parameters. The resultant value of the function can then be obtained.
Read more about algorithm here:
https://brainly.com/question/29674035
#SPJ1
please try to answer the questions you know; with workings. i would recommend doing it on a hardcover book and uploading a picture. thank you so so much <3
Answers
The measures of the angles and the proofs are shown below
Calculating the measures of the anglesCircle 4
The angle at the centre is twice the angle at the circumference
So, we have
360 - ∠BOD = 2 * 110
Evaluate
∠BOD = 140
Circle 5
Angle in a semicircle is 90 degrees
So, we have
∠ABD + 19 = 90
∠ABD = 71
Angles in the same segment are equal
So, we have
∠ACB = 19
Circle 6
Angle in a semicircle is 90 degrees
So, we have
5y + y = 90
y = 15
So, we have
∠BAC = 5 * 15
∠BAC = 75
Circle 7
By corresponding angle theorem, we have
∠ABO = ∠CDO
By the sum of opposite internal angles in a triangle, we have
∠BOC = ∠BAO + ∠ABO
Substitute ∠ABO = ∠CDO
∠BOC = ∠BAO + ∠CDO --- proved
The angles at the edges are equal because they are corresponding angles of congruent isosceles triangles
Cyclic Quadrilateral
The opposite angles of cyclic quadrilaterals add up to 180 degrees
So, we have
180 - ∠x + 180 - ∠y = 180
Evaluate
∠x + ∠y = 180 --- proved
Read more about circle theorem at
https://brainly.com/question/31385923
#SPJ1
can someone please help me graph this ? not rlly sure
Answers
The graph of the given equation is attached below.
Plotting a line graphThe equation of a line in slope-intercept form is expressed as y = mx + b.
Given an xy plane, we are to plot the graph of the given equation
y = -2/3 x + 1
The given equation has a slope of -2/3 and a y-intercept of 1. This shows that the constructed line must cut the y-axis at the point (0, 1).
The graph of the equation is attached below.
Learn more on line graph here: https://brainly.com/question/26233943
#SPJ1
graph the equation. y=-4(x+2)^2-1
Answers
Hope this helps you with your question.
Answer:
Step-by-step explanation:
I did it on a graphing app
Help me out sharty's
Answers
A number, .f, rounded to 1 d.p. is 49.2
Another number, g,
rounded to 1 d.p. is 7.8
What are the lower and upper bounds of
f - g?
Answers
Answer:
3
Step-by-step explanation:
The lower bound of f-g is 41.31 .
The upper bound of f-g is 41.49 .
Given,
A number 'f' rounded to 1 decimal point is Number = 49.2
A number 'g' rounded to 1 decimal point is Number = 7.8
Now,
The range of f rounded to 1 decimal point is [49.15 , 49.24].
The range of g rounded to 1 decimal point is [7.75 , 7.84].
So,
Lower bound of f-g = 49.15 - 7.84
Lower bound of f-g = 41.31
Next,
Upper bound of f-g = 49.24 - 7.75
Upper bound of f-g = 41.49
Therefore the lower bound of f - g is 41.31 and upper bound of f - g is 41.49.
Learn more about lower and upper bound, here:
https://brainly.com/question/32676654
#SPJ4
Solve question 11 for me please
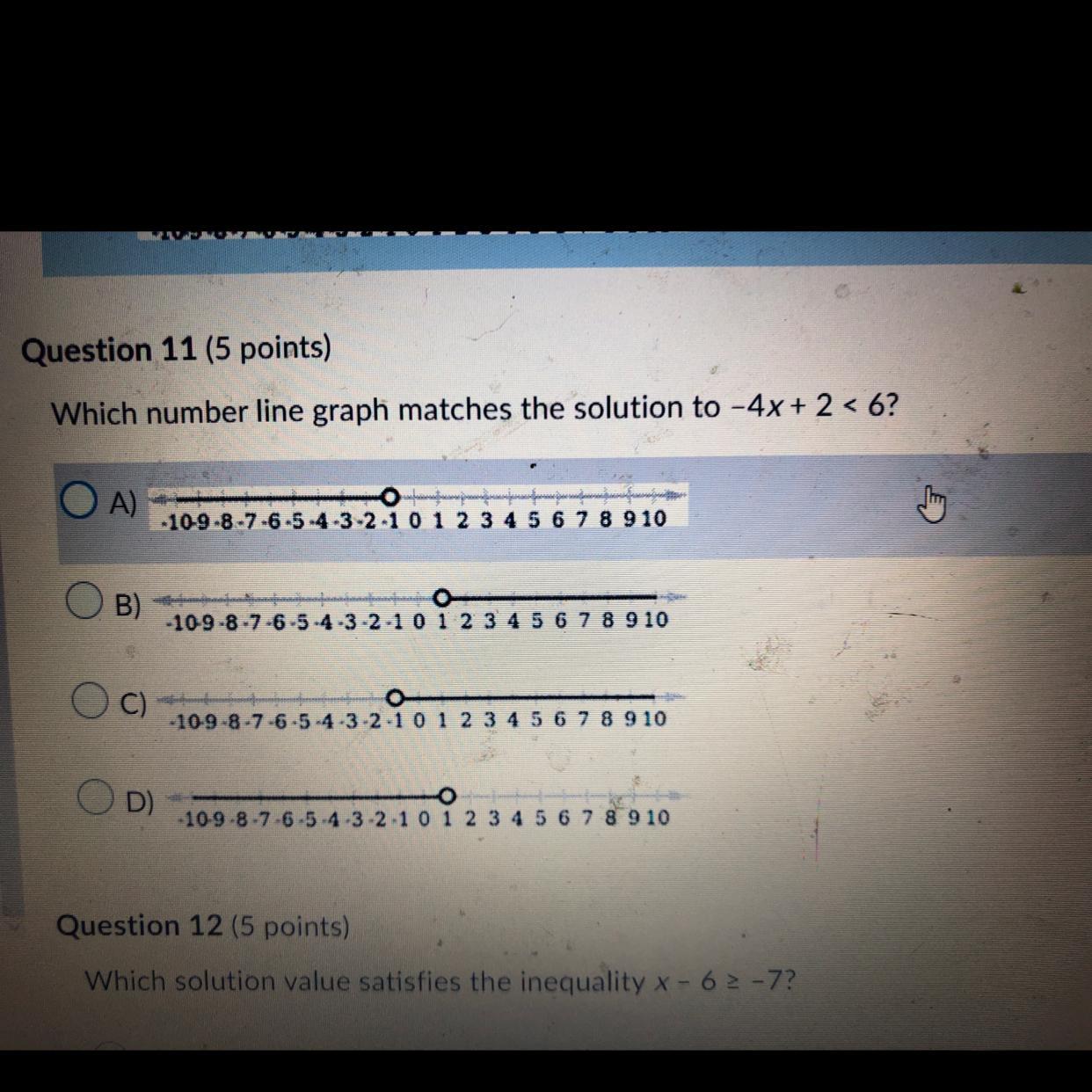
Answers
Answer:
C
Step-by-step explanation:
-4x+2<6
-4x<4
x<-1
because the sign doesnt have a line under it, it is an open hole
Let u(t) = (3,2t,3t^2) and (t) = (2t^2 – 3t,1). Compute the derivative of the following function. u(t) x v(t)
Answers
The derivative of u(t) x v(t) is \((-12t^2 - 6t, -6t^2 + 15, 6 - 6t^2 + 9t).\)
First, we need to find the cross product of u(t) and v(t):
\(u(t) x v(t) = (3, 2t, 3t^2) x (2t^2 – 3t, 1)\\= (6t^2 - 9t, 9t^2 - 6t, 3)\)
Then, we can take the derivative of this function using the product rule of differentiation:
d/dt (u(t) x v(t)) = d/dt (u(t)) x v(t) + u(t) x d/dt (v(t))
\(= (0, 2, 6t) x (2t^2 – 3t, 1) + (3, 2t, 3t^2) x (4t – 3, 0)\\= (-12t^2 + 9t, -6t^2 + 6, 6) + (-6t, 9, -6t^2 + 9t)\\= (-12t^2 - 6t, -6t^2 + 15, 6 - 6t^2 + 9t)\)
Therefore, the derivative of u(t) x v(t) is \((-12t^2 - 6t, -6t^2 + 15, 6 - 6t^2 + 9t).\)
To know more about cross product refer to-
https://brainly.com/question/29164170
#SPJ11
Sertista A (60) maiks): Answer ALL questions in this section: On. 81 . A pistoncylinder device initialiy contains 1.777 m^2
of superheated steam at 050MPa and Soo"c. The piston is then compressed to 0.3 m^4
such that the temperature remains constant. (o) Use the appropriate property table to determine mass of steam in the device. [3 Marks] (b) Sketch a pressure versus specific volume graph during the compression process. [2. Marics] (c) Drtermine the work done during the compression process. [6 Marks] (d) Oetermine the pressure of the superheated steam after compression. (e) Suggest three factors that will make the process irreversible.
Answers
The mass of steam in the device is 3.011 kg. The pressure of the superheated steam after compression is 0.5 MPa. This is an irreversible process.
(a) Use the appropriate property table to determine the mass of steam in the device.
Given, Piston cylinder device initially contains = 1.777 m³
Pressure = 0.50 MPa
Temperature = 500C
Using the steam table to find the mass of the steam inside the piston cylinder device by referring to the steam tables.
Using steam tables, the values are: Entropy = 6.8018 kJ/kgK
Enthalpy = 3194.7 kJ/kg
Mass of steam in device = volume / specific volume = 1.777 m³ / 0.5901 m³/kg = 3.011 kg
Therefore, the mass of steam in the device is 3.011 kg.
(b) Sketch a pressure versus specific volume graph during the compression process.
(c) Determine the work done during the compression process.The formula to calculate work done during the compression process is given by,
W = P(V1 - V2)
Work done during the compression process = 0.5[1.777-0.3]×106 N/m2 = 782100 J
Hence, the work done during the compression process is 782100 J.(d) Determine the pressure of the superheated steam after compression.The pressure of the superheated steam after compression is 0.5 MPa.
(e) Suggest three factors that will make the process irreversible. The three factors that will make the process irreversible are: Friction: Friction produces entropy which is a measure of energy loss. In a piston-cylinder device, friction is caused by moving parts such as bearings, seals, and sliding pistons.Heat transfer through finite temperature difference: Whenever heat transfer occurs between two systems at different temperatures, the transfer is irreversible. This is because of entropy creation due to the temperature gradient. In a piston-cylinder device, this can occur through contact with hotter or colder surfaces.Unrestrained expansion: Whenever a gas expands into a vacuum, there is no work done, and entropy is generated. This is an irreversible process.
To know more about pressure, visit:
https://brainly.com/question/30673967
#SPJ11
A square tile mesures 20 cm by 20cm a rectangular tile is 3 cm longer and 2 cm narrower. What is the different in area between the two tiles?
Answers
Answer:
Rectangular tile has 14 cm² larger area-----------------
Find each area and then find their difference.
A(square) = 20² = 400 cm²A(rectangle) = (20 + 3)(20 - 2) = 23*18 = 414 cm²The difference is:
414 - 400 = 14 cm²Maggie baked a berry pie. The recipe called for 6 ounces of blackberries, 9 ounces of raspberries, and 5 ounces of strawberries. In the supermarket, she only found 12-ounce containers of berries. She bought 1 container of each kind of berry. After she baked the pie, how many ounces of berries did Maggie have left?
Answers
Answer:
16 berries
Step-by-step explanation:
Blackberries: 12 - 6 = 6Raspberries: 12 - 9 = 3Strawberries: 12 - 5 = 7 Now we know how many individual berries will be left: 6 blackberries, 3 raspberries, and 7 strawberries.6 + 3 + 7 = 16Name MA3 - Derivatives (Power Rule) Find the derivative of the following functions. 1. f(x) = 12x² + 4x5 Solution 2. f(x) = 3x + 2x 4 + 23x Solution 3. f(x) = 9x* – 5x + 14,000,605 Solution
Answers
1) the fuction is:
\(f(x)=12x^2+4x^5\)and the derivate will be:
\(f^{\prime}(x)=24x+20x^4\)2) The function is:
\(f(x)=3x^6+2x^{-4}+23x\)and the derivate:
\(f^{\prime}(x)=18x^5-8x^{-5}+23\)3) the function is:
\(f(x)=9x^{\frac{11}{4}}-5x+14000605\)and the derivate will be:
\(\begin{gathered} f^{\prime}(x)=\frac{11}{4}\cdot9x^{\frac{11}{4}-\frac{4}{4}}-5+0 \\ f^{\prime}(x)=\frac{99}{4}x^{\frac{7}{4}}-5 \end{gathered}\)4) the function is:
\(f(x)=\frac{4x^6-13x^3-21}{x^3}\)and the derivation will be:
\(f^{\prime}(x)=\frac{(24x^5-39x^2)x^3-(4x^6-13x^3-21)3x^2}{(x^3)^2}\)5) the equation is:
\(f(x)=\frac{11}{x^3}-2\sqrt[5]{x}\)first we rewrite the equation so:
\(f(x)=11x^{-3}-2x^{\frac{1}{5}}\)and now we derivate so:
\(f(x)=-33x^{-4}-\frac{2}{5}x^{-\frac{4}{5}}\)The cross section of a prism is an n sided polygon.
Circle the number of edges that the prism has.
2n
n+2
n+ 3
3n
Answers
The number of edges that the prism has is
n + 2What does a prism's cross section look like?When a plane intersects a prism, the shape formed is known as the cross section. The cross section of the prism will have the same form as the base if it is divided by a plane that runs horizontally and parallel to the base.
A prism is a 3-dimensional object with two congruent and parallel bases (which are polyggonal) connected by rectangular lateral faces. The number of edges of a prism is equal to the sum of the number of edges of the two bases and the number of lateral faces.
Each base of the prism has n edges, so the two bases together have 2n edges. The number of lateral faces of the prism is equal to the number of edges of one of its bases, so there are n lateral faces. Each lateral face has 4 edges, so the total number of edges of the lateral faces is 4n.
Therefore, the total number of edges of the prism is equal to the sum of the number of edges of the two bases (2n) and the number of lateral faces (4n), which is 2n + 4n = 6n.
In conclusion, the cross section of a prism is an n-sided polygon and the prism has n + 2 edges.
Learn more about polygons at:
https://brainly.com/question/26583264
#SPJ1
It costs $363 to make 90 bags of cotton candy and $440 to make 200 bags of cotton candy.
a) find the cost equation.
Answers
Answer:
363=90bags
then you look into it and say
440=200bags
The citizens of Herrington County have an existing dog
park for dogs to play, but have decided to build another
one so that one park will be for small dogs and the other
will be for large dogs. The plan is to build a rectangular
fenced in area adjacent to the existing dog park, as
shown in the sketch. The county has enough money in
the budget to buy 1000 feet of fencing.
What should the dimensions of the dog park be to maximize the area?What is the maximum area of the park?
Answers
To maximise the area it should be build as a square having side=250 feet each and having area=62500 feet.
what are squares?
A square is a two-dimensional plane figure with four equal sides and all four angles are equal to 90 degrees. The properties of the rectangle are somewhat similar to a square, but the difference between the two is, a rectangle has only its opposite sides equal. Therefore, a rectangle is called a square only if all its four sides are of equal length.
The most important properties of a square are listed below:
All four interior angles are equal to 90°.All four sides of the square are congruent or equal to each other.The opposite sides of the square are parallel to each other.The diagonals of the square bisect each other at 90°.The two diagonals of the square are equal to each other.The square has 4 vertices and 4 sides.The diagonal of the square divides into two similar isosceles triangles.The length of diagonals is greater than the sides of the square.Area of square=(side)²
Perimeter of square=4*side
Now,
Given
total fencing available=1000feet
perimeter=1000
4*s=1000
s=250 feet
Therefore,
Area=250^2
=62500 feet^2
To know more about squares visit the link
https://brainly.com/question/28776767?referrer=searchResults
#SPJ1
I LITTERALY BEG OF YOU TO HELP ME!!
LMN≅PQR
What is the value of x in degrees?
Answers
Answer: 56
Step-by-step explanation:
Find the length of QR
Answers
Answer:
QR = 15
Step-by-step explanation:
The product of the length of the secant segment and its external part equals the square of the length of the tangent segment.
(QR+5) *5 = 10^2
(QR+5) *5 = 100
Divide each side by 5.
(QR+5) *5/5 = 100/5
(QR+5) = 20
Subtract 5 from each side.
QR = 20-5
QR = 15
A21 and 23 For Problems A21-A23, construct a linear mapping L: VW that satisfies the given properties.
A21 V = R³, W = P2(R); L (1,0,0) = x², L(0, 1, 0) = 2x, L (0, 0, 1) = 1 + x + x² 2
A22 V = P2(R), W Range(L) = Span = 1 0 M2x2(R); Null(Z) 0 = {0} and
A23 V = M2x2(R), W = R4; nullity(Z) = 2, rank(L) = 2, and L (6 ) - 1 1 0
Answers
Constructed a linear mapping are:
A21: L(a, b, c) = (a², 2b, 1 + c + c²).
A22: L(ax² + bx + c) = (a, b, c) for all ax² + bx + c in V.
A23: L(a, b, c, d) = (a + b, c + d, 0, 0).
A21:
For V = R³ and W = P2(R), we can define the linear mapping L as follows:
L(a, b, c) = (a², 2b, 1 + c + c²), where a, b, c are real numbers.
A22:
For V = P2(R) and W = Span{{1, 0}, {0, 1}}, we can define the linear mapping L as follows:
L(ax² + bx + c) = (a, b, c) for all ax² + bx + c in V.
A23:
For V = M2x2(R) and W = R⁴, where nullity(Z) = 2 and rank(L) = 2, we can define the linear mapping L as follows:
L(a, b, c, d) = (a + b, c + d, 0, 0), where a, b, c, d are real numbers.
Note: In A23, the given condition L(6) = [1, 1, 0] seems to be incomplete or has a typographical error. Please provide the correct information for L(6) if available.
Learn more about linear mapping
https://brainly.com/question/31944828
#SPJ11
how do I solve this please help!
Answers
Answer:
9 x + 8 y = 10 (I)
y = -4x + 7 (II)
One way is to rewrite the second as
4x + y = 7
Now multiply this equation by 8
32 x + 8 y = 56
9 x + 8 y = 10 and now subtract the equations
23 x = 46 this gives x as 2
From equation (II) y = -4 (2) + 7 = -1
So our values are (2, -1)
Check:
(I) 9 x + 8 y = 10 9 * 2 - 8 = 10
(II) 4 x + y = 7 or 4 * 2 - 1 = 7
Or you can substitute equation (II) directly into equation (I)
Graph the following
y=3-4x
Answer correct and tell me the points to graph it on or show a picture of the graph, and ill give you the brainiest.
Answers
Answer:
Step-by-step explanation:
y = -4x +3
Y-intercept = 3
The slope is negative: - 4/1
4 down ( from y = 3) and one to the right or
4 up (from y=3) and 1 to the left.
(You only need 2 points to trace a line)
I totally don't understand this I will give you 20 points if you help me with it
Answers
When subtracting from a negative number, the answer will be even more negative. The first two choices automatically don’t make sense because they made 1.5 positive. The third choice won’t work because two negatives beside each other means addition but for this question, you are subtracting. The last choice is right because it is the same as -1.5 - 2.75.
Answer:
-4.25
Step-by-step explanation:
What's the solution ?
5^3x=25^(x+2)
Answers
there is no solution