Answers
Answer:
Step-by-step explanation:

Related Questions
f is inversely proportional to √ g . When f = 8 , g = 81 Work out g when f = 4
Answers
Answer:
Step-by-step explanation:
The question is basically saying that f is equal to \(\frac{k}{\sqrt{g} }\)
when f=8, g=81. Therefore 8=\(\frac{k}{\sqrt{81} } =\frac{k}{9}\).
Then the next step is to simplify the equation, so k = 72.
The next step is to plug k=72 back into the original equation.
f=\(\frac{72}{\sqrt{g} }\)
Then you do the last step which is to plug f=4 into the equation which will result in 4=72/sqrt(g) => 4*sqrt (g) =72. Therefore sqrt (g) = 72/4 = 18. Then you square both sides and you get g=324.
A forestry study found that the diameter of the trees in a forest is normally distributed with mean 34 cm with a standard deviation of 8 cm. A group of 4 trees will be used as timber if the average of the 4 trees diameter is not too thick or thin. Specifically it is desired for the mean diameter to be between 30 and 40 cm in diameter. Find the probability that a randomly chosen group of 4 trees can be used as timber
Answers
Answer:
The probability that a randomly selected group of four trees can be used as timber is 4.5 × 10⁻⁵
Step-by-step explanation:
The given parameters are;
Mean = 34 cm
The standard deviation = 8 cm
The mean
The Z score is \(Z=\dfrac{x-\mu }{\sigma }\), which gives;
For x = 30 we have;
\(Z=\dfrac{30-34 }{8 } = -0.5\)
P(x>30) = 1 - 0.30854 = 0.69146
For x = 40, we have
\(Z=\dfrac{40-34 }{8 } = 0.75\)
P(x < 40) = 0.77337
Therefore, the probability that the mean of four trees is between 30 and 40 is given as follows;
P(30 < x < 40) = 0.77337 - 0.69146 = 0.08191
The probability that a randomly selected group of four trees can be used as timber is given as follows;
Binomial distribution
\(P(X = 4) = \dbinom{4}{4} \left (0.08191\right )^{4}\left (1-0.08191 \right )^{0} = 4.5 \times 10^{-5}\)
Solve the equation for v.
0.5v + 0.06 < 3.46
v > 1.8
v < 1.8
v > 6.8
v < 6.8
Answers
Answer:
\(\boxed{\tt v < 6.8}\)
Step-by-step explanation:
\(\tt 0.5v+0.06 < 3.46\)
Multiply both sides by 100:-
\(\tt 0.5v\times\:100+0.06\times\:100 < 3.46\times\:100\)
\(\tt 50v+6 < 346\)
Subtract 6 from both sides:-
\(\tt 50v < 340\)
Divide both sides by 50:-
\(\tt \cfrac{50v}{50} < \cfrac{340}{50}\)
\(\tt v < \cfrac{34}{5}\)
Or
\(\tt v < 6.8\)
Therefore, your answer is v < 6.8!!! :)
______________________
Hope this helps!
Have a great day!
Taxable
Income Tax
$49,020 or less………………………………………………………………………..…15%
in excess of $49
Answers
For taxable income of $49,020 or less, the tax rate is 15%. Any taxable income exceeding $49,020 will be subject to a different tax rate, which is not specified in the given information.
The provided information outlines the tax rates based on different taxable income brackets. For taxable income equal to or less than $49,020, the tax rate is 15%.
This means that if an individual or entity has a taxable income within this range, they will be required to pay 15% of their income as taxes. However, the information does not provide the tax rate for taxable income exceeding $49,020. To accurately calculate the tax liability for income above this threshold, the specific tax rate for that income range is required. Without knowing the tax rate for taxable income above $49,020, it is not possible to determine the exact tax liability for income in that range. The given information only provides the tax rate for taxable income up to $49,020, which is 15%.
Learn more about tax rate here:- brainly.com/question/30629449
#SPJ11
The product of the sum of 3 and 6 and the sum of 2 and 7
Answers
She puts a value in the box and says that the equation represents a direct variation. Which explains whether the equation could represent a direct variation
Answers
Answer:
y = kx where k>0
Step-by-step explanation:
A direct variation is in the form
y = kx
where k is a numerical positive constant ( i.e. > 0 )
Answer:
0
Step-by-step explanation:
If she puts 0 in the box she would have a direct variation.
Step-by-step explanation:
Given : She writes the equation y = 5x - __ with a missing value.
She puts a value in the box and says that the equation represents a direct variation.
We have to choose the correct option from the given options that explain that the equation could represent a direct variation.
When two variables are such that one is a constant multiple of other, we said they are in Direct variation.
That is in form of y = ax , here y and x are in direct variation.
Consider the given equation y = 5x - __
For the equation to be in direct variation the value of missing term has to be 0.
then equation becomes,
y = 5x
Thus, If she puts 0 in the box she would have a direct variation.
Let us suppose a population size of 67 million, and innovation parameter of 0.005 and imitation parameter of 0.84 for Color TV. Estimate how many new users would be added during time period 7.
Answers
To estimate the number of new users that would be added during time period 7, we can use the Bass diffusion model, which is commonly used to model the adoption of new products or technologies.
The Bass diffusion model is given by the formula:
\(\[N(t) = \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot t)}}}\]\)
where:
- N(t) represents the cumulative number of adopters at time \(t\).
- p is the innovation parameter, representing the coefficient of innovation.
- q is the imitation parameter, representing the coefficient of imitation.
- e is the base of the natural logarithm.
Given a population size of 67 million, an innovation parameter of 0.005, and an imitation parameter of 0.84 for Color TV, we can substitute these values into the Bass diffusion model and calculate the number of new users added during time period 7.
\(\[N(7) - N(6) = \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot 7)}}} - \frac{{p \cdot q}}{{q + (p/q) \cdot e^{-((p+q) \cdot 6)}}}\]\)
Substituting the given values into the equation:
\(\[N(7) - N(6) = \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 7)}}} - \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 6)}}}\]\)
Evaluating the expression will give us the estimated number of new users added during time period 7.
In LaTeX, the solution can be represented as:
\(\[N(7) - N(6) = \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 7)}}} - \frac{{0.005 \cdot 0.84}}{{0.84 + (0.005/0.84) \cdot e^{-((0.005+0.84) \cdot 6)}}}\]\)
After evaluating this expression, you will obtain the estimated number of new users added during time period 7.
To know more about LaTex visit-
brainly.com/question/18882901
#SPJ11
Question 7 of 10
Which of the following graphs represents a function that has a negative
leading coefficient? Check all that apply.
Answers
The leading coefficient determines the shape of the graphs, such as how
the characteristic of a function are directed.
Correct response:
The graphs that represents functions that have a negative leading
coefficient are;
Graph A, Graph B, and Graph CMethods by which the correct options are selectedGraph A:
The function in graph A is x³
When the coefficient of x³ is negative, the value of the function rises as x decreases from 0 to -∞, and decreases as x increases from 0 to ∞
Therefore;
The leading coefficient of Graph A is negative.Graph B:
The value of the graph decreases as the magnitude of x increases
therefore, the graph is similar to a quadratic function, such that the leading
coefficient is negative, which inverts the function to give increasing output
with negative value as the value of x increases.
Therefore;
The leading coefficient of the quadratic function in Graph B is negative.Graph C:
The given function in graph C is a linear function having a negative slope,
therefore;
The leading coefficient of x in the function in Graph C is negative.Graph D:
The function of the graph in Graph D, that have y values that increases
exponentially as x increases is a quadratic function.
Given that y increases as the value of x increases, the leading coefficient
(coefficient of x²) is positive.
Therefore;
The graphs that represents functions that have negative leading coefficient are; Graph A, Graph B, and Graph C.Learn more about the factors that depend on leading coefficient of a function here:
https://brainly.com/question/12209936




Given that the square root of 225 is 15 and the square root of 256 is 16, which number is closest to the square root of 240?
Responses
15.1
15.1
15.5
15.5
15.8
15.8
15.9
15.9
Answers
We can approach this problem by first finding the two perfect squares that 240 lies between:
15^2 = 225 and 16^2 = 256.
Since the square root of 240 must lie between the square root of 225 and 256, we can choose the number that is closest between 15 and 16.
To do this, we can calculate the distance between the square root of 240 and each of the two numbers:
The distance between 15 and the square root of 240 is |15 - sqrt(240)| = 1.18
The distance between 16 and the square root of 240 is |16 - sqrt(240)| = 0.34
Therefore, 16 is closest to the square root of 240.
Find the time it takes for $9,200 to double when invested at an annual interest rate of 10%, compounded continuously.
___________years
Find the time it takes for $920,000 to double when invested at an annual interest rate of 10%, compounded continuously.
___________years
Answers
It takes 69.3147 years for $9,200 to double when invested at an annual interest rate of 10%, compounded continuously.
It takes 69.3147 years for $920,000 to double when invested at an annual interest rate of 10%, compounded continuously.
To find the time it takes for an investment to double at an annual interest rate of 10%, compounded continuously, we can use the formula:
t = ln(2) / (r ln(e))
Let's calculate the time for each scenario:
1. For $9,200 to double:
r = 0.10 (10% annual interest rate)
t = ln(2) / (0.10 x ln(e))
t = 6.93147 / 0.10
t = 69.3147 years
Therefore, it takes 69.3147 years for $9,200 to double when invested at an annual interest rate of 10%, compounded continuously.
2. For $920,000 to double:
r = 0.10 (10% annual interest rate)
t = ln(2) / (0.10 x ln(e))
t = 6.93147 / 0.10
t= 69.3147 years
Therefore, it takes 69.3147 years for $920,000 to double when invested at an annual interest rate of 10%, compounded continuously.
Learn more about Compound Interest here:
https://brainly.com/question/22621039
#SPJ4
Helene invested a total of $1000 in two simple-interest bank accounts. One account paid 5% annual interest; the other paid 7% annual interest. The total amount of interest she earned after 1 year was $60. Find the amount invested in each account.
Answers
x + y = 1000, (1)
0.05x + 0.06y = 58. (2)
From (1), express y = 1000-x and then substitute it into (2)
0.05x + 0.06*(1000-x) = 58
0.05x + 60 - 0.06x = 58
-0.01x = 58 - 60 = -2 ====> x = %28-2%29%2F%28-0.01%29 = 200%2F1 = 200.
Answer. $200 was invested at 5%. The rest $1000 - $200 = $800 was invested at 6%.
Check. 200*0.05 + 800*0.06 = 58. ! Correct !
Solved. // I used the Substitution method to solve the system of 2 equations in 2 unknowns.
Please Help ASAP! I NEED THIS NOW!.


Answers
The quadratic equation is \(x^2+x-12\)
What is quadratic equation?
Quadratics can be defined as a polynomial equation of a second degree, which implies that it comprises a minimum of one term that is squared. It is also called quadratic equations. The general form of the quadratic equation is:
ax² + bx + c = 0
where x is an unknown variable and a, b, c are numerical coefficients.
Given, the roots of quadratic equation as (3,-4).
The formula to find the quadratic equation, as
x2 – (Sum of the roots)x + Product of the roots = 0
Sum of roots = 3-4
=-1
Product of roots = 3(-4) = -12
The quadratic equation is
x²+x-12
To know more about quadratic equation, visit:
https://brainly.com/question/17482667
#SPJ1
Billie & Tony are siblings. It’s summer of 2015. Twice Billie’s age decreased by 3 is Tony’s age. Tony is fourteen years older than Billie. What are the ages of Billie & Tony?
Answers
Answer:
Tony = 31 Billie = 17
Step-by-step explanation:
17 x 2 = 34
34 - 3 = 31
17(billie's age) + 14(years that Tony is older) = 31
Answer:
what the other person said
Step-by-step explanation:
4.Beyunka is creating a diagram to prove that a certain triangle is a right triangle.
In her diagram, she uses three white quadrilaterals that are squares. Which
diagram could be the one that Beyunka is creating?

Answers
Option 'D' is the correct option.
The correct diagram for Beyunka creating is given in option D.
What is Right triangle?
When an angle of a triangle is measure 90 degree then the triangle is called a right triangle.
Given that;
Beyunka is creating a diagram to prove that certain triangle is a right triangle.
Since, The right triangle is satisfy the Pythagoras theorem.
Now, By option D;
Let sides of a triangle are,
Hypotenuse (H) = c
Perpendicular side (P) = a
Then, The base of the right triangle = √H² - P² = √c² - a²
And, All other diagrams are not satisfy the Pythagoras theorem.
Thus, The correct diagram for Beyunka creating is given in option D.
Learn more about the right triangle visit:
https://brainly.com/question/15249087
#SPJ2
Help with questions 7-12 on ratios (geometry)

Answers
The measures of the sides in a triangle with the ratio 21:8:14 are 105, 40 and 70.
What is the ratio?The ratio is defined as the comparison of two quantities of the same units that indicates how much of one quantity is present in the other quantity.
7) The ratio of the measures of two complementary angles is 7:8.
We know that, sum of complementary angles is 90°.
7x+8x=90°
15x=90
x=6
7x=42° and 8x=48°
8) The ratio of the measures of the three angles in a triangle is 2:9:4.
Sum of interior angles of a triangle is 180°.
2x+9x+4x=180°
15x=180°
x=12°
Here, 2x=24°, 9x=108° and 4x=48°
9) The ratio of the measures of the three angles in a triangle is 10:3:7.
Sum of interior angles of a triangle is 180°.
10x+3x+7x=180°
20x=180°
x=9°
Here, 10x=90°, 3x=27° and 7x=63°
10) The ratio of the measure of the vertex angle to the base angle of an isosceles triangle is 8:5.
Sum of interior angles of a triangle is 180°.
8x+5x+5x=180°
18x=180°
x=10°
Here, 8x=80°, 5x=50°
11) The ratio of the measures of the sides in a triangle is 21:8:14.
21x+8x+14x=215
43x=215
x=5
So, sides are 21x=105, 8x=40, 14x=70
12) The ratio of the measures of the sides in a triangle is 4:7:5.
4x+7x+5x=128
16x=128
x=8
So, sides are 4x=32, 7x=56 and 5x=40
Therefore, the measures of the sides in a triangle with the ratio 21:8:14 are 105, 40 and 70.
To learn more about the ratio visit:
brainly.com/question/13419413.
#SPJ1
What is the solution for the compound 5/2+x>1/3 or x+2 < -29/6
Answers
Answer:
x < -41/6 or x > -13/6.
Step-by-step explanation:
5/2+x>1/3
x > 1/3 - 5/2
x > 2/6 - 15/6
x > -13/6
x+2 < -29/6
x < -29/6 - 2
x < -41/6
The answer is x < -41/6 or x > -13/6.
Answer:
x > -13/6 or x < -41/6
Step-by-step explanation:
5/2+x>1/3 or x+2 < -29/6
x > 1/3 - 5/2 or x < -29/6 - 2
x > 2/6 - 15/6 or x < -29/6 - 12/6
x > -13/6 or x < -41/6
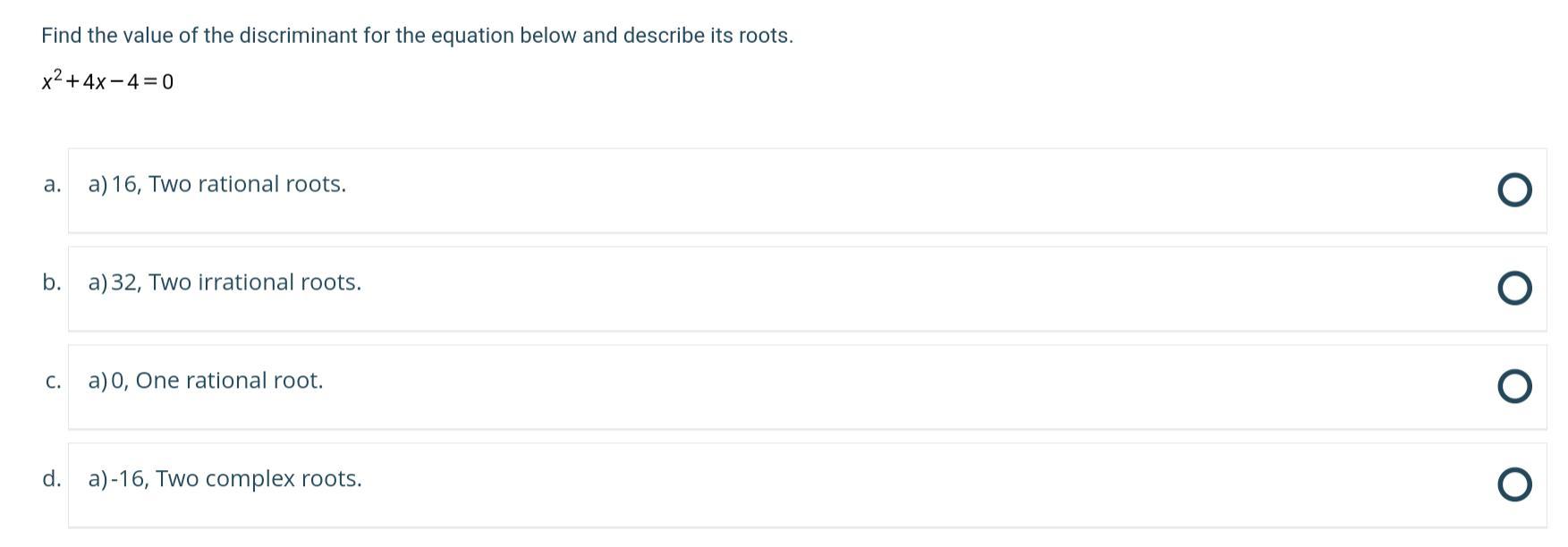
Find the length of AB.
AB= [ ? ]in
![Find the length of AB. AB= [ ? ]in](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/CkeI3kKwAVUsU34JEQO0ErdTZMED5dQe.png)
Answers
A hat contains 100 coins. With the probability of 0.5, a hat contains 100 fair coins. Otherwise, a hat contains 99 fair coins and one double-headed (always landing Heads). A coin is chosen uniformly at random. The chosen coin is flipped 7 times, and it lands Heads all 7 times. Given this information, what is the probability that one of the coins is double-headed? 0.00004 0.39 0.005 0.004
Answers
The probability that one of the coins is double-headed is approximately 0.00004.
The probability that one of the coins is double-headed can be determined using Bayes' theorem. Given that a coin is chosen uniformly at random and flipped 7 times, landing Heads all 7 times, we can calculate the probability that one of the coins is double-headed.
Let's denote the event of choosing a fair coin as F and the event of choosing the double-headed coin as D. We need to calculate the probability of D given that we observed 7 consecutive Heads, denoted as P(D | 7H).
Using Bayes' theorem, we have:
P(D | 7H) = (P(7H | D) * P(D)) / P(7H)
We know that P(7H | D) = 1 (since the double-headed coin always lands Heads), P(D) = 0.5 (given that the probability of choosing the double-headed coin is 0.5), and P(7H) can be calculated as:
P(7H) = P(7H | F) * P(F) + P(7H | D) * P(D)
= (0.5^7) * 0.5 + 1 * 0.5
= 0.5^8 + 0.5
Substituting these values into the equation for Bayes' theorem:
P(D | 7H) = (1 * 0.5) / (0.5^8 + 0.5)
= 0.5 / (0.5^8 + 0.5)
Calculating this expression, the probability that one of the coins is double-headed is approximately 0.00004.
To learn more about probability click here: brainly.com/question/32117953
#SPJ11
The area of the surface of the swimming pool is 210 square feet. what is the length of the deep end?
Answers
The length of the deep end is 12 feet of the swimming pool.
Given: Area of the swimming pool is 210 square feet
Width of the pool = 10 feet
The length of the shallow end is 9 feet and the length of the deep end is d.
To find the value of d.
Let's solve the problem.
The area of the swimming pool is 210
The width is 10
The deep end length is d
The shallow end length is 9
The total length of the swimming pool = The length of the deep end + The length of the shallow end
=> d + 9
Therefore, the total length of the swimming pool is d + 9
The surface of the swimming pool is rectangular, so
The area of rectangle = width × length
Therefore,
area of swimming pool = width of the pool × length of the swimming pool
=> 210 = 10 × (9 + d)
or 10 × (9 + d) = 210
Dividing both sides by 10:
10 × (9 + d) / 10 = 210 / 10
9 + d = 21
Subtracting 9 on both sides:
9 + d - 9 = 21 - 9
d = 12
Therefore the length of the deep end is 12 feet
Hence the length of the deep end is 12 feet of the swimming pool.
Know more about "areas" here: https://brainly.com/question/27683633
#SPJ4

The length of the deep end of the swimming pool is 12 feet.
We are given that:
The Area of the swimming pool = 210 square feet
width of the swimming pool = 10 feet
Length of shallow end = 9 feet
Let the length of the deep end be d.
Total length of the swimming pool = length of deep end + length of shallow end = d + 9
Area of swimming pool = width × length
Substituting the values, we get that:
210 = 10 × (9 + d)
9 + d = 21
d = 12
Therefore the length of the deep end of the swimming pool is 12 feet.
Learn more about area here:
brainly.com/question/27683633
#SPJ4
17. Who am I? ___ Collection of one or more different types of variables, including arrays and pointers, that have been grouped under a single name for each manipulation.
a) template
b) array
c) structure
d) local variables
Answers
You are c) a structure. A structure is a collection of one or more different types of variables, including arrays and pointers, that have been grouped under a single name for each manipulation.
A structure is a user-defined data type that allows you to group together related data. For example, you could create a structure to store the name, age, and address of a person. The structure would have three variables, each of a different type: a string variable for the name, an integer variable for the age, and a string variable for the address.
The advantage of using a structure is that it allows you to treat the related data as a single unit. This makes it easier to manipulate the data and to pass the data to functions.
The other answer choices are incorrect. A template is a blueprint for creating a generic class or function. An array is a collection of elements of the same type. Local variables are variables that are declared within a function and that are only accessible within the function.
To learn more about integer variable click here : brainly.com/question/11873770
#SPJ11
Using the Method of Undetermined Coefficients, determine the form of a particular solution for the differential equation. (Do not evaluate coefficients.) y" +25y = 7t² sin 5t Brea The root(s) of the auxiliary equation associated with the given differential equation is/are (Use a comma to separate answers as needed.) Write the form of a particular solution. Choose the correct answer below. OA. Yp()=(Ast+A₂t²+A₁t+Ao) cos 5t+ (B3t+B₂t² + B₁t+Bo) sin 5t OB. Yp(t)= (Ast+At+At+Apt) e' cos 5t+ (Byt +B₂t+B₁+Bo) e' sin 5t OC. Yp(t)=t(Ast+A₂t²+A₁t+Ao) e' cos 5t+1(8₂1 +8₂ +B₁t+Bo) 'sin 5t OD. Yp(t)=t(Ast+A₂t² +A₁t+A) cos 5t+t(Byt + B₂t² +B₁t+Bo) sin 5t
Answers
The given differential equation is y'' + 25y = 7t²sin5t.Using the method of undetermined coefficients, The correct option is (OC) i.e. Yp(t)=t(Ast+A₂t²+A₁t+Ao) e' cos 5t+1(8₂1 +8₂ +B₁t+Bo) 'sin 5t.
The given differential equation is y'' + 25y = 7t²sin5t.
Using the method of undetermined coefficients, let the particular solution be Yp(t) = A1t²sin5t + A2tsin5t + A3tcos5t + A4sin5t + A5cos5t.
where Ai's are the constants to be determined.A2t and A3t represent the coefficient of t and cost in particular solutions since the roots of the auxiliary equation are complex.
Let's compute Yp′(t) and Yp″(t).Yp(t) =
A1t²sin5t + A2tsin5t + A3tcos5t + A4sin5t + A5cos5tYp′(t) = 5A1t²cos5t + A2sin5t + 5A2tcos5t - 5A3tsin5t - 5A3sin5t + 5A4cos5t - 5A5sin5tYp″(t)
= - 25A1t²sin5t + 10A2cos5t - 50A2tsin5t - 10A3sin5t - 10A3cos5t - 25A4sin5t - 25A5cos5t.
Substituting Yp(t), Yp′(t), and Yp″(t) into the differential equation y'' + 25y = 7t²sin5t, we get: - 25A1t²sin5t + 10A2cos5t - 50A2tsin5t - 10A3sin5t - 10A3cos5t - 25A4sin5t - 25A5cos5t + 25(A1t²sin5t + A2tsin5t + A3tcos5t + A4sin5t + A5cos5t) = 7t²sin5t.
Simplifying and grouping the coefficients, we get: (- 25A1 + 25A1)t²sin5t + (10A2 - 25A5)cos5t + (- 50A2 - 10A3)sin5t + (5A2 + 25A4)cos5t + (5A3 - 25A5)sin5t = 7t²sin5tComparing the coefficients on the left side and the right side, we get the following equations:10A2 - 25A5 = 0 ------------ (1)5A2 + 25A4 = 0 ------------ (2)5A3 - 25A5 = 0 ------------ (3)- 50A2 - 10A3 = 0 ------------ (4)- 25A1 + 25A1 = 7 ----------- (5)Solving these equations, we get:A1 = - 7/2500A2 = 7/1250A3 = 0A4 = 0A5 = 7/1250.
Therefore, the particular solution isYp(t) = (- 7/2500)t²sin5t + (7/1250)tsin5t + 7/1250cos5t.
Hence, the form of the particular solution is: Yp(t)=t(Ast+A₂t²+A₁t+Ao) cos 5t+t(Byt + B₂t² +B₁t+Bo) sin 5t.
Thus, the correct option is (OC) i.e. Yp(t)=t(Ast+A₂t²+A₁t+Ao) e' cos 5t+1(8₂1 +8₂ +B₁t+Bo) 'sin 5t.
To know more about Differential visit :
https://brainly.com/question/31383100
#SPJ11
Factor the following expression.
10,5 + 513 – 14,2 - 7
Answers
((10,5 + 513) - 14,2) - 7 =502.3
Can someone help me solve this whole thing please it’s a bed project for geometry

Answers
The total cost of building the raised garden bed and adding mulch will be $12 + $39.90, which is $51.90.
What is probability?Probability is a measure of the likelihood of an event occurring. It is a number between 0 and 1, where 0 means the event is impossible and 1 means the event is certain to happen.
To determine if building a raised garden bed with mulch is within your budget of $40, you need to calculate the cost of the materials.
Assuming each panel is 12 inches by 12 inches, six panels will cover an area of 2 feet by 2 feet or 48 inches by 48 inches.
So the total area of the garden bed will be 48 inches by 48 inches, which is 2,304 square inches.
To build the garden bed, you will need 6 panels, which cost $2 each, for a total of $12.
To calculate the amount of mulch you need, divide the area of the garden bed (2,304 square inches) by the coverage of one bag of mulch (240 square inches), which gives you 9.6 bags of mulch.
Since you can't buy a fraction of a bag, you'll need to round up to 10 bags of mulch.
The total cost of the mulch will be 10 bags x $3.99 per bag, which is $39.90.
Therefore, the total cost of building the raised garden bed and adding mulch will be $12 + $39.90, which is $51.90.
To learn more about probability from the given link:
https://brainly.com/question/30034780
#SPJ1
(-13,20) (-12,-10) slope
Answers
Answer:
10
Step-by-step explanation:
\(\frac{-20-(-10)}{-13-(-12)}=\frac{-10}{-1}=10\)
Find the output, y, when the input, x, is 30
y=14 - 0.5x
Answers
Answer:
y = -1
Step-by-step explanation:
Given x = 30 , y = 14 -0.5x
Find y
let (x = 30) fill in y
y = 14 - 0.5(30)
y = 14 - 15
Thus , y = -1Find the maximum area of a rectangle whose perimeter is 32m
Answers
64m is the maximum area of the rectangle
8x8=64
8+8+8+8=32
The graph of y=3x is shown. What is the value of x when y=27?
A. 2
B. 3
C. 9
D. 24
It said c was wrong
Answers
Answer:
x = 3
Step-by-step explanation:
Is x an exponent?
\( y = 3^x \)
\( 27 = 3^x \)
\( 3^3 = 3^x \)
\( x = 3 \)
Question 4(Multiple Choice Worth 5 points)
(05.02)Find the measure of angle x in the figure below:
X
155
30
95°
o
55
O 30
O 85

Answers
Answer:
95°
Step-by-step explanation:
y° = 180° - (55° + 30°)
y° = 180° - 85°
y ° = 95°
y = 95
x = y ( vertical angles)
x = 95°
A 10 kilogram object suspended from the end of a vertically hanging spring stretches the spring 9.8 centimeters. At time t = 0, the resulting mass-spring system is disturbed from its rest state by the force F(t) = 100cos(8t). The force F(t) is expressed in Newtons and is positive in the downward direction, and time is measured in seconds.
Determine the spring constant k.
k = ? Newtons / meter
Formulate the initial value problem for y(t), where y(t) is the displacement of the object from its equilibrium rest state, measured positive in the downward direction. (Give your answer in terms of y,y′,y′′,t.)
Differential equation: ?
Initial conditions: y(0) = ? and y′(0) = ?
Solve the initial value problem for y(t).
y(t) = ?
Plot the solution and determine the maximum excursion from equilibrium made by the object on the time interval 0 ≤ t < [infinity]. If there is no such maximum, enter NONE.
maximum excursion = ? meters
Answers
Using Hooke's law the maximum value of |cos(8t)| is 1, so the maximum excursion is 1/6 meters.
To find the spring constant k, we use Hooke's law:
F = -ky
where F is the weight of the object, and y is the distance it is stretched from its rest position. At equilibrium, F = mg = 10 × 9.81 = 98.1 N. Thus,
98.1 = -k × 0.098
k = -1000 N/m
The equation of motion for the system is given by:
my'' + ky = F(t)
Substituting the given values, we get:
10y'' + (-1000)y = 100cos(8t)
y'' - 100y = 10cos(8t)
with initial conditions y(0) = 0 and y'(0) = 0.
The characteristic equation is r² - 100 = 0, with roots r = ±10i. The complementary solution is therefore y_c(t) = c1cos(10t) + c2sin(10t).
For the particular solution, we assume a form of yp(t) = Acos(8t) + Bsin(8t), and substitute it in the differential equation to get:
-64Acos(8t) - 64Bsin(8t) - 100(Acos(8t) + Bsin(8t)) = 10cos(8t)
Solving for A and B, we get A = -1/6 and B = 0. Thus, the particular solution is yp(t) = (-1/6) × cos(8t).
The general solution is therefore y(t) = c1cos(10t) + c2sin(10t) - (1/6)*cos(8t). Applying the initial conditions, we get c1 = 0 and c2 = 0, so the particular solution is simply y(t) = (-1/6) × cos(8t).
The maximum excursion from equilibrium can be found by taking the absolute value of y(t) and finding its maximum value. We have:
|y(t)| = (1/6) × |cos(8t)|
The maximum value of |cos(8t)| is 1, so the maximum excursion is 1/6 meters.
Learn more about Hooke's law at
https://brainly.com/question/29126957
#SPJ4
11-4 skills practice areas of regular polygons and composite figures answers
Answers
The 11-4 skills practice areas of regular polygons and composite figures involve finding the areas of different geometric shapes using specific formulas and methods.
To find the area of regular polygons, you can use the formula (1/2) x apothem x perimeter.
For composite figures, you can break the shape into smaller, more manageable shapes like rectangles, triangles, or circles, and then calculate the area of each component before adding them together.
Hence, The 11-4 skills practice areas of regular polygons and composite figures teach you to find areas using the appropriate formulas and methods, improving your geometry understanding and problem-solving abilities.
learn more about polygons click here:
https://brainly.com/question/26583264
#SPJ11
