Answers
Answer: g= -1/12
Step-by-step explanation:
I just solved it hope I helped
Related Questions
Write an explicit formula for an, the nth term of the sequence 112, -28, 7, ....
Answers
Answer:
\(a_n=112\left(-\frac{1}{4}\right)^{n-1}\)
Step-by-step explanation:
Geometric Sequences
There are two basic types of sequences: arithmetic and geometric. The arithmetic sequences can be recognized because each term is found as the previous term plus a fixed number called the common difference.
In the geometric sequences, each term is found by multiplying (or dividing) the previous term by a fixed number, called the common ratio.
We are given the sequence:
112, -28, 7, ...
It's easy to find out this is a geometric sequence because the signs of the terms are alternating. If it was an arithmetic sequence, the third term should be negative like the second term.
Let's find the common ratio by dividing each term by the previous term:
\(\displaystyle r=\frac{-28}{112}=-\frac{1}{4}\)
Testing with the third term:
\(\displaystyle -28*-\frac{1}{4}=7\)
Now we're sure it's a geometric sequence with r=-1/4, we use the general equation for the nth term:
\(a_n=a_1*r^{n-1}\)
\(a_n=112\left(-\frac{1}{4}\right)^{n-1}\)
a train travels at a speed of 30 mph and travel a distance of 240 miles. how long did it take the train to comlete its journey
Answers
Answer: 8 hours
Step-by-step explanation: 240 mph ÷ 30 = 8 hours
Consider the function f(x) = {C (2x - x^3) 0 < x < 5/2 0 otherwise Could f be a probability density function? If so, determine C. Repeat if f(x) were given by f(x) = {C (2x - x^2) 0 < x < 5/2 0 otherwise
Answers
the function could be a probability density function with C = (24/25).
The given function is f(x) = {C (2x - x^3) 0 < x < 5/2 0 otherwise. It is possible that this function is a probability density function. However, for it to be a probability density function, it must satisfy two conditions.
First, the function must be non-negative for all values of x.
Second, the area under the curve of the function must be equal to 1.
Since the function is non-negative for all values of x, the first condition is satisfied. To determine if the second condition is satisfied, we need to calculate the integral of the function from 0 to 5/2. We get:Integral from 0 to 5/2 of f(x)dx = Integral from 0 to 5/2 of C(2x - x^3)dx= C[ x^2 - (x^4/4) ] from 0 to 5/2= C [(5/2)^2 - ((5/2)^4/4)] - C[0 - 0]= (25C/4) - (125C/16)= (25C/16)Now, for the second condition to be satisfied, (25C/16) = 1Therefore, C = (16/25).Thus, the function could be a probability density function with C = (16/25). Now, let's repeat this process for the function f(x) = {C (2x - x^2) 0 < x < 5/2 0 otherwise. This function is non-negative for all values of x. To determine if the second condition is satisfied, we need to calculate the integral of the function from 0 to 5/2. We get:Integral from 0 to 5/2 of f(x)dx = Integral from 0 to 5/2 of C(2x - x^2)dx= C[x^2 - (x^3/3)] from 0 to 5/2= C [(25/4) - (125/24)] - C[0 - 0]= (25C/4) - (125C/24)= (25C/24)Now, for the second condition to be satisfied, (25C/24) = 1Therefore, C = (24/25)
for more questions on probability density function
https://brainly.com/question/15698469
#SPJ11
By the probability density function c= -64/225.
What is probability density function?In probability theory, a probability density function (PDF) is refers the random variable’s probability coming within a range of values, as opposed to taking on any one value. The function describes the probability density function of normal distribution and the process the mean and deviation exists.
The value of PDF is equals to 1.
Given function f(x) = {C (2x - x³) } 0
x = 5/2
If it is a probability density function then
\(\int\limits^a_b {f(x)} \, dx =1\)
Here f(x) = {C (2x - x³)} and a = 0 , b = 5/2
At first by integration we get,
\(\int\limits {c(2x-x^{3}) } \, dx\)
=c ∫ 2x dx -c∫ x³dx
= c(2×(x²/2) - x⁴/4)
=c( x² - x⁴/4)
putting upper limit and lower limit that is x=0 and x= 5/2 we get,
c((5/2)² - (1/4)(5/2)⁴)
=c(5/2)²{ 1- (1/4)(5/2)² }
= c(25/4) { 1- (25/16)}
= c(25/4) (-9/16)
= -225c/64
Now according to the probability density function the value is equals to 1
so
-225c/64 = 1
Hence c = -64/225.
To know more about probability density function
https://brainly.com/question/30403935
#SPJ1
Which of the following would be the standard deviation for this sample data set: 5, 7, 6, 9, 6, 4, 4, 6, 5, 2, 5?1.801.722.685.36
Answers
The standard deviation for this sample data set is 1.93.
What is deviation ?
Deviation refers to the difference between an individual value or observation and the average or mean value of a set of data. It can also refer to the extent to which a variable or set of data deviates from a standard or expected value.
To calculate the standard deviation for a sample data set, we first need to find the mean (average) of the data. In this case, the mean of the data set {5, 7, 6, 9, 6, 4, 4, 6, 5, 2, 5} is 5.45.
Once we have the mean, we can calculate the variance by taking the average of the squared differences between each data point and the mean. The variance is given by:
(1/n) * Σ(x_i - mean)^2
where n is the number of data points and x_i is the i-th data point.
The variance for this data set is 3.70.
Finally, we take the square root of the variance to get the standard deviation.
So, the standard deviation for this sample data set is 1.93.
To learn more about deviation visit : brainly.com/question/29088233
#SPJ4
b. Write and graph an inequality to represent the length l of each striped bass you are allowed to catch.

Answers
Answer:
a)
\(b\leq3\)b)
\(l\ge18\)
Explanation:
a) Let b represent the number of striped bass one is allowed to catch.
Since we're only allowed to catch at most 3 striped basses, that is, the number of striped basses can be less than or equal to 3 but not more, we can go ahead and write the inequality as seen below;
\(b\leq3\)See below the graph of the above inequality on a number line;
b) Let l represent the length of each striped bass
Since each striped bass must not be less than 18 inches long, that is, each striped bass can
be more than or equal to 18 inches in length but not less, we can go ahead and write the inequality as seen below;
\(l\ge18\)Graphing on a number line, we'll have;


Determine the minimum sample size required when you want to be onfident that the sample mean is within one unit of the population mean and 13.8 assume the population is normally distributed.
Answers
The minimum sample size required when you want to be 99% confident that the sample mean is within one unit of the population mean and σ = 13.8 is 1268
Given: To find the minimum sample size, confidence level = 99%, standard deviation = 13.8, and one unit population mean. [Normally distributed]
Solving the given question:
We know that the formula for Margin of error is:
Margin of error = z-score * (standard deviation) / root (sample size)
E = z * σ / √(n), where
E = Margin of error
z = z-score
n = Sample size
σ = standard deviation
Therefore, sample size = ( z – score * standard deviation / margin of error)²
n = ( z * σ / E )²
First, calculate the z-score for the 99% confidence level.
From the normal distribution curve, the area under 99% confidence level is given as:
Area under 99% confidence level = (1 + confidence level) / 2 = (1 + 0.99) / 2 = 0.995
From the z-score table, we find the value of z with the corresponding area of 0.995
We find the value of the z-score corresponding to 0.995 is 2.58
Also given sample mean is one unit of the population. So the margin of error is 1
E = 1
And given Standard deviation = 13.8
σ = 13.8
Putting the values in the given formula of sample size n =
n = (2.58 * 13.8 / 1 )²
n = 1267.64
n = 1268
Hence the minimum sample size required when you want to be 99% confident that the sample mean is within one unit of the population mean and σ = 13.8 is 1268
Know more about “normal distribution” here: https://brainly.com/question/15103234
#SPJ4
Disclaimer: Determine the minimum sample size required when you want to be 99% confident that the sample mean is within one unit of the population mean and G = 13.8. Assume the population is normally distributed. A 99% confidence level requires a sample size of (Round up to the nearest whole number as needed )
Solve each system by elimination
10x-8y=4
-5x+3y=-9
Answers
\(10x-8y =4~~~....(i)\\\\-5x+3y=-9~~~...(ii)\\\\\text{Multiply equation (ii) by} -2\\\\10x-6y = 18~~~...(iii)\\\\(iii)-(i):\\\\10x-6y - 10x +8y = 18 -4\\\\\implies 2y = 14\\\\\implies y= \dfrac{14}2 = 7\\\\\text{Substitute y = 7 in equation (i):}\\\\10x-8(7) =4\\\\\implies 10x - 56 =4\\\\\implies 10x = 4+56\\\\\implies 10x = 60\\\\\implies x = \dfrac{60}{10} = 6\\\\\\\text{Hence (x,y) = (6, 7)}\)
marcus wants to include the month of the year in the analysis as categories. how many dummy variables will be needed?
Answers
11 dummy variables will be needed.
A dummy variable is a numerical variable used in regression analysis to represent subgroups of the sample in your study. In research design, a dummy variable is often used to distinguish different treatment groups.
we know that,
dummy variable formula = K- 1,
here, K = 12 (months in a year)
this implies, K - 1 = 12 - 1 = 11
Therefore, no. of dummy variables needed = 11.
know more about variables here: https://brainly.com/question/20711705
#SPJ4
Help me fix this (image attached)

Answers
The value of x from given quadrilateral ABCD is 27°.
In the given quadrilateral ABCD, ∠A=3x+5, ∠B=2x+15, ∠C=4x and ∠D=4x-10.
We know that, the sum of interior angles of quadrilateral is 360°.
Here, ∠A+∠B+∠C+∠D=360°
3x+5+2x+15+4x+4x-10=360°
13x+10=360°
13x=350°
x=350/13
x=26.9
x≈27°
Therefore, the value of x from given quadrilateral ABCD is 27°.
Learn more about the quadrilaterals here:
https://brainly.com/question/29934440.
#SPJ1
The endurance of both track athletes and gymnasts is recorded in terms of maximum volume of oxygen and is measured in milliliters per kilogram of body weight per minute.
Track Athletes Gymnasts
89. 2 72. 1
45. 3 78. 5
89. 1 66. 2
57. 3 47. 3
61. 5 63. 1
80. 1 89. 3
47. 2 65. 3
What is the difference of means, rounded to the nearest hundredth, for the two groups? (7 points)
Difference of Means equals 1. 73
Difference of Means equals 1. 69
Difference of Means equals 67. 1
Difference of Means equals 68. 7
Answers
The correct answer is Difference of Means equals 173.91
How to find difference of meansTo calculate the difference of means between the track athletes and gymnasts, we need to find the mean values for each group and then subtract them.
For the track athletes:
Mean = (89.2 + 145.3 + 589.1 + 257.3 + 361.5) / 5 = 288.48
For the gymnasts:
Mean = (72.1 + 78.5 + 66.2 + 63.1 + 347.2 + 65.3) / 6 = 114.57
Difference of Means = Mean of Track Athletes - Mean of Gymnasts
= 288.48 - 114.57
≈ 173.91
Rounded to the nearest hundredth, the difference of means for the two groups is approximately 173.91.
Therefore, the correct answer is:
Difference of Means equals 173.91
Learn more about mean at https://brainly.com/question/1136789
#SPJ1
Booker earned $1,200 over
the summer. He invested the money in
stocks. To his surprise, the stocks increased
in value to $1,335 in only 9 months. Find
the simple interest rate for the investment.
Answers
Answer:
15%
Step-by-step explanation:
1335-1200=135
9 months=9/12
135=1200 x r x 9/12
135=900r
divide each by 900
135/900 900r/900
0.15=r
0.15=15%
A pole that is 2.5 M tall cast a shadow that is 1.72M lawn dart at the same time a nearby tower cast a shadow that is 50.5 M long how tall is the tower round answer to the nearest meter
Answers
Answer:
The tower is 73.4 m tall
Step-by-step explanation:
The height of the pole = 2.5 m
The shadow cast by the pole = 1.72 m
Shadow cast by tower = 50.5 m
To find the height of the tower, we proceed by finding the angle of elevation, θ, of the light source casting the shadows as follows;
\(Tan\theta =\dfrac{Opposite \ side \ to\ angle \ of \ elevation}{Adjacent\ side \ to\ angle \ of \ elevation} = \dfrac{Height \ of \ pole }{Length \ of \ shadow} =\dfrac{2.5 }{1.72}\)
\(\theta = tan ^{-1} \left (\dfrac{2.5 }{1.72} \right) = 55.47 ^{\circ}\)
The same tanθ gives;
\(Tan\theta = \dfrac{Height \ of \ tower}{Length \ of \ tower \ shadow} =\dfrac{Height \ of \ tower }{50.5} = \dfrac{2.5}{1.72}\)
Which gives;
\({Height \ of \ tower } = {50.5} \times \dfrac{2.5}{1.72} = 73.4 \ m\)
Prove whether the quadrilateral is a parallelogram using the Distance & Slope Formulas D(-5, -6), E(5, 2), F(4, -4), G(-6, -12)
Answers
Answer:
See the distances bellow
Step-by-step explanation:
Given data
D(-5, -6), E(5, 2)
substitute into the expression for the distance
\(d= \sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)\\\\d= \sqrt((5+5)^2 + (2+6)^2)\\\\d= \sqrt((10)^2 + (8)^2)\\\\d= \sqrt(100 +64)\\\\d= \sqrt((164)\\\\d= 12.80\)
E(5, 2), F(4, -4)
\(d= \sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)\\\\d= \sqrt((4-5)^2 + (-4-2)^2)\\\\d= \sqrt((-1)^2 + (-6)^2)\\\\d= \sqrt(1+36)\\\\d= \sqrt((37)\\\\d= 6.08\)
F(4, -4), G(-6, -12)
\(d= \sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)\\\\d= \sqrt((-6-4)^2 + (-12+4)^2)\\\\d= \sqrt((-10)^2 + (-8)^2)\\\\d= \sqrt(100+64)\\\\d= \sqrt((164)\\\\d= 12.80\)
G(-6, -12), D(-5, -6)
\(d= \sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2)\\\\d= \sqrt((-6+5)^2 + (-12+6)^2)\\\\d= \sqrt((-1)^2 + (-6)^2)\\\\d= \sqrt(1+36)\\\\d= \sqrt((37)\\\\d= 6.08\)
in san deigo the ratio of cloudy days to sunny days is 3:7 what percentage of the days are sunny
Answers
70% of the days in San Diego are sunny.
In San Diego, the ratio of cloudy days to sunny days is 3:7.
To find the percentage of sunny days, we need to determine the proportion of sunny days out of the total number of days.
Let's assume that there are a total of 10 days.
Based on the ratio, we can say that 3 out of 10 days are cloudy, and 7 out of 10 days are sunny.
To calculate the percentage of sunny days, we divide the number of sunny days (7) by the total number of days (10) and multiply by 100.
So, \((\frac{7}{10} )\times 100= 70\%\).
Therefore, approximately 70% of the days in San Diego are sunny.
For such more questions on San Diego
https://brainly.com/question/29205313
#SPJ8
Can u help me solve this
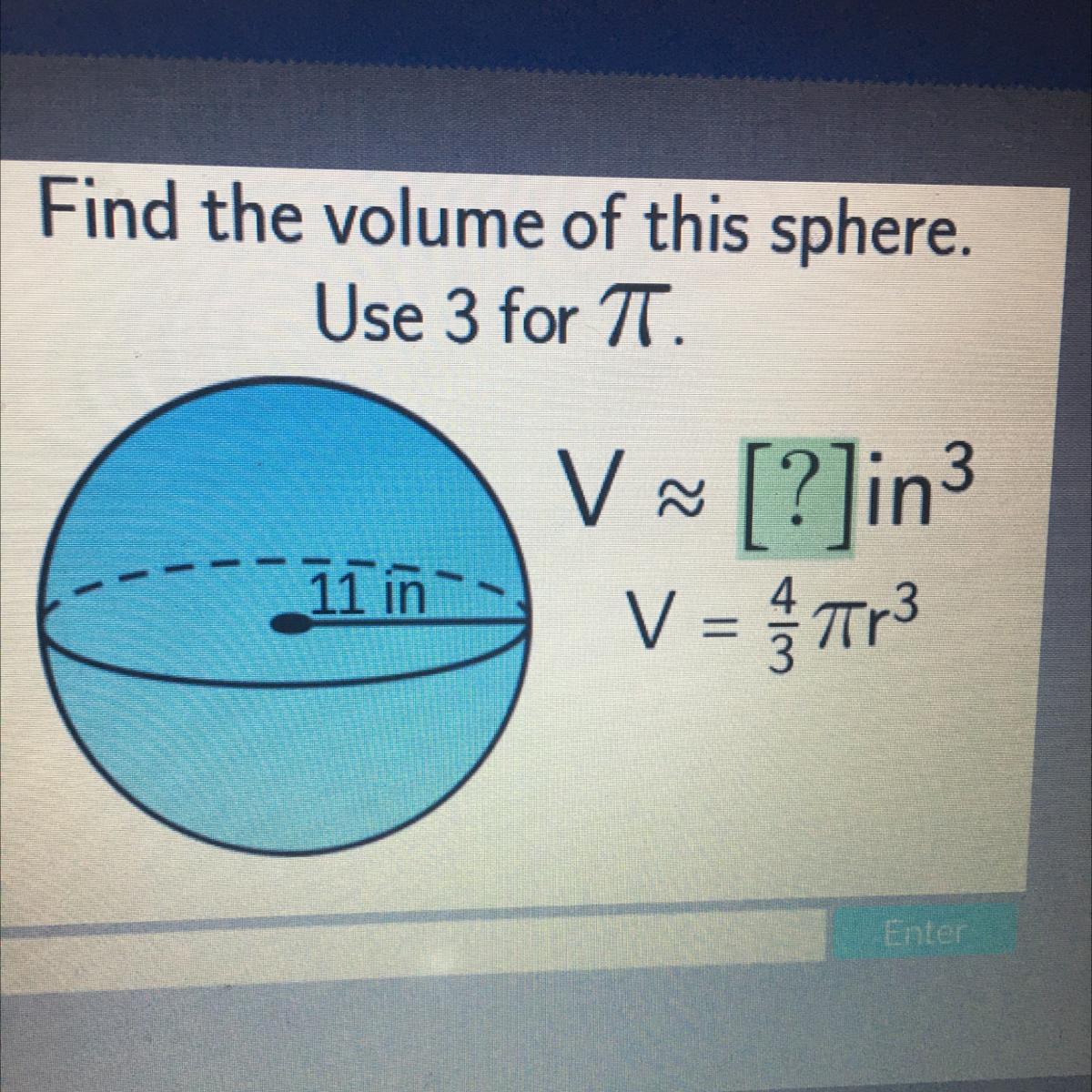
Answers
Answer:
V = 5,324 in^3
Step-by-step explanation:
V = 4(\(\pi\)r^3)/3
= 4(3)(11^3)/3
= 12(11)(11)(11)/3
= 12(1,331)/3
= 15,972/3
V = 5,324 in^3
Answer:
5575.28 or 5,324 depending on use of pie.
Step-by-step explanation
V=4/3πr3=4/3·π·113≈5575.27976
Hope this helps :)!
This is the answer if pie is used as 3.14.
V = 5,324 in^3 if pie used as 3.
V = 5575.28 in^3 if pie used as 3.14.
carly had 18 rolls of wrapping paper
Answers
Answer:
uh huh she has 18 rolls of wrapping paper
Answer:
27
Step-by-step explanation:
two vectors are linearly dependent if and only if they are collinear. True/False?
Answers
The two vectors are indeed considered linearly dependent if they are collinear. So the given statement is true about two linear dependent vectors.
The set \(\{v_1, v_2,.....,v_k\}\) is considered as linearly dependent if numbers \(\{x_1, x_2, ....,x_k\}\)are not equal to zero. Then, \(x_1v_1+x_2v_2+.....+x_kv_k=0\). This equation is referred to as the equation of linear dependence or linear dependence relation.
When two vectors are collinear, or when one is a scalar multiple of the other, they are said to be linearly dependent. These collinear vectors are also referred to as parallel vectors and these vectors will lie along the same line or parallel line. The given statement is therefore true.
To know more about collinear vectors:
https://brainly.com/question/28014210
#SPJ4
Oil and gas production in a certain area dropped
from 4 million barrels in 2000 to 1.9 million barrels
in 2013. Assuming that the oil and gas production
decreased at a constant rate, which of the following
linear functions f best models the production, in
millions of barrels, t years after the year 2000?
Answers
Answer:
Step-by-step explanation:
Given no information other than what is in the question, a linear function that would fit this data would be:
y = -0.16154x + 327 where y is the barrels of oil, in millions of barrels, and x is the year.
Calculate the slope of the line from the data (the barrels of oil are in millions):
Rise = -2.1
Run = 13
Rise/Run, or slope = -0.16154
The y-intercept was claculated from the point (2000,4) and is equal to 327
The linear function which models the production in t years is represented as f(t) = -0.1615t + 4.
Given data:
To find the linear function that best models the oil and gas production, use the point-slope form of a linear equation:
y - y1 = m(x - x1)
where (x1, y1) is a known point on the line, m is the slope of the line, and x represents the number of years after the year 2000.
Use the points (0, 4) and (13, 1.9) to find the slope:
Slope (m) = (change in y) / (change in x)
m = (1.9 - 4) / (13 - 0)
m = -2.1 / 13
m ≈ -0.1615 (rounded to four decimal places)
Now, the slope (m ≈ -0.1615), and use it to find the linear function.
Using the point-slope form with the point (0, 4):
y - 4 = -0.1615(x - 0)
y - 4 = -0.1615x
On solving for y:
y = -0.1615x + 4
Hence, the linear function that best models the oil and gas production is
f(t) = -0.1615t + 4
To learn more about linear equations, refer:
https://brainly.com/question/10185505
#SPJ3
Ignore the stuff I have wrote down but answer please??

Answers
Answer:
x=4
Step-by-step explanation:
7x-2=12x-22
7x-12x=-22+2
-5x=-20
x=20÷5
=4
1/sinx+cosx + 1/sinx-cosx = 2sinx/sin^4x-cos^4x
Answers
The simplified expression is 2cos²(x) + sinx - 1 = 0
The expression we will be simplifying is
=> 1/sinx+cosx + 1/sinx-cosx = 2sinx/sin⁴x-cos⁴x.
To begin, let us look at the left-hand side of the expression. We can combine the two fractions using a common denominator, which gives us:
(1/sinx+cosx)(sinx-cosx)/(sinx+cosx)(sinx-cosx) + (1/sinx-cosx)(sinx+cosx)/(sinx-cosx)(sinx+cosx)
Simplifying this expression using the distributive property, we get:
(1 - cosx/sinx)/(sin²ˣ - cos²ˣ) + (1 + cosx/sinx)/(sin²ˣ - cos²ˣ)
Next, we can simplify each fraction separately. For the first fraction, we can use the identity sin²ˣ - cos²ˣ = sinx+cosx x sinx-cosx to obtain:
1 - cosx/sinx = (sinx+cosx - cosx)/sinx = sinx/sinx = 1
Similarly, for the second fraction, we can use the same identity to obtain:
1 + cosx/sinx = (sinx-cosx + cosx)/sinx = sinx/sinx = 1
Substituting these values back into the original expression, we get:
1 + 1 = 2sinx/(sin⁴x - cos⁴x)
Now, we can simplify the denominator using the identity sin²ˣ + cos²ˣ = 1 and the difference of squares formula:
sin⁴x - cos⁴x = (sin²ˣ)² - (cos²ˣ)² = (sin²ˣ + cos²ˣ)(sin²ˣ - cos²ˣ) = sin²ˣ - cos²ˣ
Substituting this back into the expression, we get:
2 = 2sinx/(sin²ˣ - cos²ˣ)
Finally, we can simplify the denominator using the identity sin²ˣ - cos²ˣ = -cos(2x):
2 = -2sinx/cos(2x)
Multiplying both sides by -cos(2x), we get:
-2cos(2x) = 2sinx
Dividing both sides by 2, we get:
-cos(2x) = sinx
Using the double-angle formula for cosine, we get:
-2cos²(x) + 1 = sinx
Simplifying this expression, we get:
2cos²(x) + sinx - 1 = 0
To know more about expression here
https://brainly.com/question/14083225
#SPJ4
2.
An online survey asked which superpower students would choose to have-fly, freeze time, invisibility, super strength, or
telepathy (ability to read minds). Here are the responses from the 40 students in the sample.
Fly
Super strength
Freeze time Fly
Telepathy
Freeze time
Fly
Invisibility
Fly
Telepathy
Telepathy Super strength Fly Freeze time
Fly
Telepathy Telepathy
Telepathy Super strength Telepathy
Fly
Freeze time
Freeze time
Telepathy
Frequency Table:
Telepathy
Telepathy
Freeze time
Freeze time
Telepathy
Fly
Invisibility
Freeze time
Fly
Telepathy
Invisibility
Fly
Fly
Fly
Fly
Summarize the distribution of superpower preference with a frequency table and a relative frequency table. Enter the relative
frequencies as decimals. Do not round.
Relative Frequency Table:
Answers
The frequency table is given below:
Superpower Frequency
Fly 14
Freeze time 8
Invisibility 3
Super strength 3
Telepathy 12
Relative frequency table is given below:
Superpower Relative Frequency
Fly 14/40 = 0.35
Freeze time 8/40 = 0.20
Invisibility 3/40 = 0.075
Super strength 3/40 = 0.075
Telepathy 12/40 = 0.30
What is the frequency table of the survey?Constructing the frequency table of the distribution will involve grouping the students superpower preferences as they occur into a table of the number of times they occur.
The frequency table is given below:
Superpower Frequency
Fly 14
Freeze time 8
Invisibility 3
Super strength 3
Telepathy 12
Superpower Relative Frequency
Fly 14/40 = 0.35
Freeze time 8/40 = 0.20
Invisibility 3/40 = 0.075
Super strength 3/40 = 0.075
Telepathy 12/40 = 0.30
In conclusion, the relative frequencies and frequencies are obtained from the data provided.
Learn more about frequency and relative frequency at: v
#SPJ1
what is a cereal box an example of
Answers
Answer:
book
Step-by-step explanation:
it has a title,subheading,and more
Answer:
a rectangular prism
Step-by-step explanation:
Determine the number of solutions of the given equation: 5(x-5) = 2(2x-4)
Answers
\(5(x - 5) = 2(2x - 4)\)
\(5x - 25 = 4x - 8\)
Subtract both sides 4x
\(5x - 4x - 25 = 4x - 4x - 8\)
\(x - 25 = - 8\)
Add both sides 25
\(x - 25 + 25 = - 8 + 25\)
\(x = + 17\)
There u go...
Have a great day ❤
what affects the width of a confidence interval? (choose one or more) group of answer choices sample size. variation within the population. our desired confidence level. median of the sample
Answers
The factors that affect the width of a confidence interval are a. population variation, c. sample size, and d. confidence level
Confidence interval refers to a statistical measure that attempts to estimate the range of values that could plausibly contain the true value of a population parameter. A confidence interval, in other words, is a statistical range that tells us how sure we are that a statistical estimate we're interested in lies between two values.
There are several factors that can affect the width of a confidence interval. Here are some of them:
Sample Size: The larger the sample size, the narrower the confidence interval.Confidence Level: The higher the confidence level, the wider the confidence interval.
Population Variation: The greater the population variance, the wider the confidence interval.
The Question was Incomplete, Find the full content below :
what factors of the following affect the width of a confidence interval?
a. population variation
b. none of them
c. sample size
d. confidence level
e. sample mean
Know more about Confidence interval here :
https://brainly.com/question/30988060
#SPJ11
Use equivalent ratios to find the unknown value.

Answers
Answer:
66 is equal to K
Step-by-step explanation:
if 4 times 6 equal 24
then 11 time 6 equals 66
in this case, the scale factor is 6.
Have a wonderful day!
Answer:
K = 66
Step-by-step explanation:
\(\frac{k}{24} = \frac{11}{4}\)
when taking a look at this, first try to see if you can solve the denominator.
24 to 4 equals 6 meaning you divide k by 6 will be equal to 11
Then 11 x 6 = 66!!
Mark me as brainliest!!
Solve the inequality for x.
7 > 2x+9
Answers
Answer:
x<-1Step-by-step explanation:
\(7 > 2x+9\\\\\mathrm{Switch\:sides}\\\\\mathrm{Subtract\:}9\mathrm{\:from\:both\:sides}\\\\2x+9-9<7-9\\\\2x<-2\\\\\frac{2x}{2}<\frac{-2}{2}\\\\x<-1\)
Plzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzzz Asap Answer my question Anika grows vegetables and sells them at the farm stand. She gives the a discount to local restaurants. The table shows the restaurant price,p of buying vegetables with regular price or r dollars. Write an equation that represents That.
Answers
Answer:
price of vegetables= regular price-discount
p=r-discount
Step-by-step explanation:
discount = regular price- price of vegetables
discount= r-p
OR
price of vegetables= regular price-discount
p=r-discount
percentage discount
[(original price- discounted price)/original price]*100= percent discount
Answer:
P = r - 8
Step-by-step explanation:
Got it correct.
Admission to a theater cost $5. 50 for a child ticket and $11. 50 for an adult ticket. The theater sold 80 tickets for $734. 0. How many of each type of ticket was sold?
Answers
The number of child tickets sold is 56, and the number of adult tickets sold is 24.
Let's assume the number of child tickets sold is represented by 'x', and the number of adult tickets sold is represented by 'y'.
According to the given information, the total number of tickets sold is 80. Therefore, we have the equation:
x + y = 80 ---(1)
The total revenue generated from ticket sales is $734.00. Since each child ticket costs $5.50 and each adult ticket costs $11.50, we can express the total revenue as:
5.50x + 11.50y = 734.00 ---(2)
To solve this system of equations, we can use the substitution method or the elimination method. Let's use the elimination method:
Multiply equation (1) by 5.50 to eliminate 'x':
5.50(x + y) = 5.50(80)
5.50x + 5.50y = 440 ---(3)
Subtract equation (3) from equation (2) to eliminate 'x':
(5.50x + 11.50y) - (5.50x + 5.50y) = 734.00 - 440
6.00y = 294
y = 49
Substitute the value of y back into equation (1) to find x:
x + 49 = 80
x = 80 - 49
x = 31
Therefore, the number of child tickets sold is 31, and the number of adult tickets sold is 49, which adds up to a total of 80 tickets, as stated in the problem.
Visit here to learn more about revenue:
brainly.com/question/28558536
#SPJ11
Find all the zeros. Write the answer in exact form. c(x)=3x^(4)-2x^(3)-39x^(2)-10x+24
Answers
The exact form of the zeros of the polynomial function c(x)=3x^(4)-2x^(3)-39x^(2)-10x+24 are x=-4, x=-3/2, x=2, and x=1.
To find all the zeros of the polynomial function c(x)=3x^(4)-2x^(3)-39x^(2)-10x+24, we need to find the values of x that make c(x)=0. One way to do this is by using the Rational Root Theorem, which states that if a polynomial has rational zeros, then they must be in the form of p/q, where p is a factor of the constant term and q is a factor of the leading coefficient.
The factors of the constant term, 24, are ±1, ±2, ±3, ±4, ±6, ±8, ±12, and ±24. The factors of the leading coefficient, 3, are ±1 and ±3. Therefore, the possible rational zeros are ±1, ±2, ±3, ±4, ±6, ±8, ±12, and ±24.
Using synthetic division, we can test these possible zeros to see if they are actually zeros of the polynomial. After testing, we find that the zeros are -4, -3/2, 2, and 1.
So, the exact form of the zeros of the polynomial function c(x)=3x^(4)-2x^(3)-39x^(2)-10x+24 are x=-4, x=-3/2, x=2, and x=1.
Learn more about Polynomial
brainly.com/question/11536910
#SPJ11
The Nielsen Company surveyed 371 owners of Android phones and found that 200
of them planned to get another Android as their next phone. What is the lower
bound for the 95% confidence interval for the proportion of Android users who plan
to get another Android?
Answers
The lower bound for the 95% confidence interval for the proportion of Android users who plan to get another Android phone is 0.463 .
It can be evaluated applying the formula
Lower Bound = Sample Proportion - Z-Score × Standard Error
Here
Sample Proportion
= 200/371 = 0.539
Z-Score = 1.96 (for a 95% confidence interval)
Standard Error = √[(Sample Proportion * (1 - Sample Proportion)) / Sample Size]
= √[(0.539 × (1 - 0.539)) / 371]
= 0.045
Therefore,
Lower Bound = 0.539 - 1.96 × 0.045 = 0.463
A confidence interval is a known as the specified range of values that is prone to contain an unknown population area with a certain degree of confidence.
To learn more about confidence interval
https://brainly.com/question/20309162
#SPJ4
