Answers
Answer:
1/4/4/3 is the same as 1/4 x 3/4
Step-by-step explanation:
first fraction-keep
Division sign-flip to multiplacation
Second sign-flip
Related Questions
Find the product. (4.0 × 10−^2) × (5.2 × 10^8)
Answers
Answer:
20800000
Step-by-step explanation:
put it in a calculator
The diameter of a music CD is 12 centimeters. Find the circumference of a CD to the nearest tenth
Answers
C = 2πr
C = 2(3.14)6
C = 37.7 rounded
the radius is 6 because it is half of the diameter
The number of goldfish in a tank is 22, and the volume of the tank is 56 cubic feet. what is the density of the tank? 0.34 goldfish per cubic foot 0.39 goldfish per cubic foot 1.41 goldfish per cubic foot 2.55 goldfish per cubic foot
Answers
Option B is correct. 0.39 goldfish per cubic feet is the density of the tank given that there are 22 goldfishes and volume of the tank is 56 cubic feet. This can be obtained by using the formula of density.
Calculate the density of tank:Given that there are 22 goldfishes,
mass of tank = 22 goldfishes
volume of tank = 56 cubic feet
Density = mass/volume= 22/56
= 0.39 goldfish per cubic feet
Hence 0.39 goldfish per cubic feet is the density of the tank given that there are 22 goldfishes and volume of the tank is 56 cubic feet.
Learn more about density:
brainly.com/question/14762317
#SPJ1
Answer: 0.39 goldfish
Step-by-step explanation:
Please help! My test is timed and im having trouble
Solve the following problem. It may be helpful to use draw a chart on scrap paper to organize the information and write the equation. Be sure to show all steps (V.E.S.T.) and work in order to receive full credit.
A grocer wants to make a 10-pound mixture of cashews and peanuts that he can sell for $3.64 per pound. If cashews cost $5.80 per pound and peanuts cost $2.20 per pound, how many pounds of each must he mix?
Answers
He will have to mix 3 pounds of cashews and 7 pounds of peanuts .
What is equation ?
The definition of an equation in algebra is a mathematical statement that demonstrates the equality of two mathematical expressions. For instance, the equation 3x + 5 = 14 consists of the two equations 3x + 5 and 14, which are separated by the 'equal' sign.
A mathematical statement known as an equation is made up of two expressions joined together by the equal sign. A formula would be 3x - 5 = 16, for instance. When this equation is solved, we discover that the value of the variable x is 7.
Let c be denoted as cashew and p be denoted as peanuts
c + p = 10 ⇒ (1)
5.6c + 2.3p = 3.29*10 ⇒ (2)
Multiply eq(1) by 560
Multiply eq(2) by 100
560c + 560p = 5600
560c + 230p = 3290
Subtract and solve for "p":
330p = 2310
p = 7 (amt. of peanuts needed)
substitute the value of p in eq(1)
c + 7 = 10
c = 3 (amt. of cashews needed)
To learn more about equation from the given link
https://brainly.com/question/22688504
#SPJ1
Please help I put 50 points

Answers
Answer:
output = input - equation
y = x - 7
so 7 is your correct equation!!
Step-by-step explanation:
as u can see
When input x is subtracted from output y, we are getting answer 7
like here
imma gonna tell u how 7 is came
1-8=7
2-9=7
3-10=7
4-11=7
so 7 is our equation
its just a explanation okay
so we get our solution
•°• Y = X - 7
hope it may help you!!
mark as brainlist
Which of the following shapes can you use to highlight important parts of a chart? A. arrow. B. circle. C. callout. D. all of the above.
Answers
The C. Ghost rectangle or callout shape is used to represent comments in flowcharts"
This shape is used to provide additional information or comments about a particular process or decision in a flowchart.
It is typically a rectangular shape with a dashed or dotted border, and a tail pointing to the relevant step or decision.
The parallelogram shape is used to represent input or output in a flowchart, while the diamond shape is used to represent decision points. The rectangle shape is used to represent a process or action in the flowchart.
It is important to use the correct symbols and shapes in flowcharts to ensure clarity and accuracy in the representation of processes and decision-making.
learn more about callout shape here:
brainly.com/question/19704602
#SPJ1
complete question:
Which of the following is used to represent comments in flowcharts?
A. Parallelogram
B. Diamond
C. Ghost rectangle or callout shape
D. Rectangle
6.1 Colby bought a laptop worth Rx for his university studies. The value of the laptop decreased at r% per annum using the reducing balance method. After 4 years, the value of the laptop was worth 31 of its original price. Calculate r, the rate of depreciation.
6.2 On 1 February 2014 , Ncominkosi took a loan from a bank to buy a car. His first payment for the loan was due on 31 July 2014 . Once he started paying the loan, it took him 6 years to fully pay the loan at an interest rate of 9,5% p.a. compounded monthly. In total, he paid the bank R596 458,10.
6.2.1 How much was his monthly instalment?
6.2.2 How much money did he borrow from the bank? Write down your answer to the nearest rand.
Answers
6.1). the rate of depreciation, r, is approximately 10.77%.
6.2.1). Ncominkosi's monthly installment amount was approximately R 10,505.29.
6.2.2). Ncominkosi borrowed approximately R 377,510.83 from the bank.
6.1) Let's assume the original price of the laptop is P. According to the reducing balance method, the value of the laptop after 4 years can be calculated as P * (1 - r/100)^4. We are given that this value is 31% of the original price, so we can write the equation as P * (1 - r/100)^4 = 0.31P.
Simplifying the equation, we get (1 - r/100)^4 = 0.31. Taking the fourth root on both sides, we have 1 - r/100 = ∛0.31.
Solving for r, we find r/100 = 1 - ∛0.31. Multiplying both sides by 100, we get r = 100 - 100∛0.31.
Therefore, the rate of depreciation, r, is approximately 10.77%.
6.2.1) To determine the monthly installment amount, we can use the formula for calculating the monthly payment on a loan with compound interest. The formula is as follows:
\(P = \frac{r(PV)}{1-(1+r)^{-n}}\)
Where:
P = Monthly payment
PV = Loan principal amount
r = Monthly interest rate
n = Total number of monthly payments
Let's calculate the monthly installment amount for Ncominkosi's loan:
Loan amount = Total amount paid to the bank - Interest
Loan amount = R 596,458.10 - R 0 (No interest is deducted from the total paid amount since it is the total amount paid)
Monthly interest rate = Annual interest rate / 12
Monthly interest rate = 9.5% / 12 = 0.0079167 (rounded to 7 decimal places)
Number of monthly payments = 6 years * 12 months/year = 72 months
Using the formula mentioned above:
\(P = \frac{0.0079167(Loan Amount}{1-(1+0.0079167)^{-72}}\)
Substituting the values:
\(P = \frac{0.0079167(596458.10}{1-(1+0.0079167)^{-72}}\)
Calculating the value:
P≈R10,505.29
Therefore, Ncominkosi's monthly installment amount was approximately R 10,505.29.
6.2.2) To determine the amount of money Ncominkosi borrowed from the bank, we can subtract the interest from the total amount he paid to the bank.
Total amount paid to the bank: R 596,458.10
Since the total amount paid includes both the loan principal and the interest, and we need to find the loan principal amount, we can subtract the interest from the total amount.
Since the interest rate is compounded monthly, we can use the compound interest formula to calculate the interest:
\(A=P(1+r/n)(n*t)\)
Where:
A = Total amount paid
P = Loan principal amount
r = Annual interest rate
n = Number of compounding periods per year
t = Number of years
We can rearrange the formula to solve for the loan principal:
\(P=\frac{A}{(1+r/n)(n*t)}\)
Substituting the values:
Loan principal (P) = \(\frac{596458.10}{(1+0.095/12)(12*6)}\)
Calculating the value:
Loan principal (P) ≈ R 377,510.83
Therefore, Ncominkosi borrowed approximately R 377,510.83 from the bank.
Learn more about interest rate here:
https://brainly.com/question/14599912
#SPJ11
How many 1/3 pound servings are in 4 2/3 pounds of cheese?
Answers
Answer:
14 Serving
Step-by-step explanation:
If you multiply 1/3 14 times.
they're 13 1/3 pound servings in 4 2/3 pounds of cheese
Joan wants to have $250,000 when she retires in 29 years. How much should she invest annually in her sinking fund to do this if the interest is 4% compounded annually?
Answers
Joan should invest $4720 annually in her sinking fund to have $250,000 when she retires
Calculating the amount to investWe can use the future value formula for an annuity to solve this problem:
FV = PMT * [(1 + r)^n - 1] / r
Where:
FV = future valuePMT = annual paymentr = interest raten = number of periodsWe want to find PMT, so we can rearrange the formula:
PMT = FV * r / [(1 + r)^n - 1]
Plugging in the values we know:
FV = $250,000
r = 0.04
n = 29
PMT = $250,000 * 0.04 / [(1 + 0.04)^29 - 1]
PMT = $250,000 * 0.04 / 22.718
PMT = $4720
So Joan should invest approximately $4720 annually in her sinking fund to have $250,000 when she retires in 29 years, assuming an interest rate of 4% compounded annually.
Read more about compound interest at
https://brainly.com/question/24924853
#SPJ1
Help solve for the area

Answers
Answer:
B
Step-by-step explanation:
half × base × height
height × length
Answer: B
Step-by-step explanation:
Triangle)
25 - 7 = 18
\(A=\frac{1}{2}(b)(h)\\A=\frac{1}{2}(18)(17)\\A=153cm^2\)
Rectangle)
\(A=b(h)\\A=7(17) = 119cm^2\)
Total)
\(153+119=272 cm^2\)
I need help solving for x

Answers
That’s the answer
which is not a solution to the differential equation y" + 4y = 0? A y = 10 B y=4e-2X C. y=3 sin(2x) D. y-2 cos(2x) + 4
Answers
D. y-2 cos(2x) + 4 is not a solution to the differential equation y" + 4y = 0.
The homogeneous Linear DifferentialThe differential equation y" + 4y = 0 is a second-order, homogeneous linear differential equation with constant coefficients. The general solution to this type of differential equation is of the form y = e^(rt) where r is a root of the characteristic equation r² + 4 = 0. The roots of this equation are r = +/- √(4) = +/- 2i, which are both imaginary. Therefore, the general solution to the differential equation is y = c1cos(2x) + c2*sin(2x), where c1 and c2 are arbitrary constants.
Option D. y - 2 cos(2x) + 4 is not a solution to this differential equation because it doesn't have the same form as the general solution. It is a combination of a constant term, cosine and sine function but the general solution only contains sine and cosine function.
Learn more about homogeneous linear differential here:
https://brainly.com/question/22212112
#SPJ4
If 6 workers take 700 hours to finish a project, how long will it take 12 workers to complete the same project?
Answers
Answer:
350 hours
Step-by-step explanation:
700/2 = 350
There are double the amount of workers so it is half the time.
can someone please help me in finding the range

Answers
Answer:
\(\{-20, -2 ,13\}\)
Step-by-step explanation:
The range of a function is the set of its possible output values
\(f(x) = 3x-8\\\\f(-4) = 3(-4) -8 = -12 -8 = -20\\\\f(2) = 3(2) -8 =6-8 = -2\\ \\f(7) = 3(7) -8 = 21 -8 = 13\\\\\text{Range} = \{-20, -2 ,13\}\)
2. Find the missing side length of the right
triangle below?

Answers
Answer:
x=16
Step-by-step explanation:
use the Pythagorean theorem.
\(\sqrt{a^{2}+b^2 \\)= \(c^{2}\) Another way, you can do the problem is that you can see
\(\sqrt{12^2+b^2\)= \(20^{2}\) that 12 divided by 4 is 3 and 20 divided by 4 is 5, which is
144+b^2=400 the two numbers from the special 3,4,5 triangle, and since
-144 -144 you have figured that much out, you can multiply 4*4 and
------------------------ get 16.
b^2=256
\(\sqrt{256\\}\) = 16
How tall is this building?
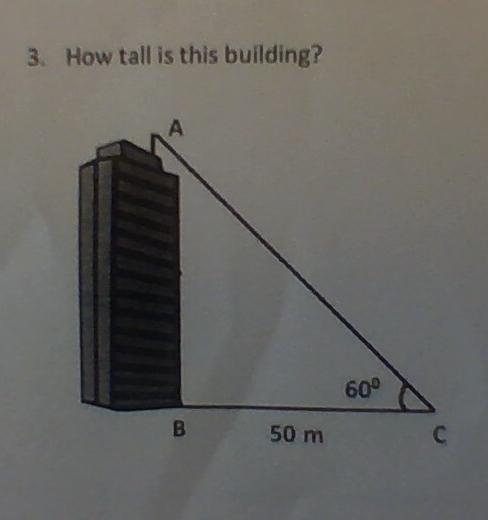
Answers
Answer:
The building is 86.6 m tall.
Step-by-step explanation:
Coh Cah Toa
Coh: sin(theta) = Opp/Hyp
Cah: cos(theta) = Adj/Hyp
Toa: tan(theta) = Opp/Adj
Using Toa.
tan(theta) = Opp/Adj
tan(60°) = x/50
x = 50 * tan(60°)
x = 50 * √(3)
x ≈ 86.60254038
x = 86.6 m
Mr. Yara has 200 acres of land available to plant for three types of fruit trees (lemon, peach and
cherry). Based on the market survey, lemon has a selling price of $2 per kg; peach has a selling
price of $1. 5 per kg and cherry has a selling price of $4 per kg. The average yield per acre for
lemon, peach and cherry is 4, 6 and 2 tonne, respectively. The fertilizer requires per acre for
lemon, peach and cherry is 100, 100 and 50 kg, respectively. An acre of lemon and cherry
requires 10 labour hours each whereas an acre of peach requires 12 labour hours. Maximum
availability of labour is 20,000 hours. The cost of fertilizer is $2 per kg and the cost of labour is
$40 per hour. What is the optimal allocation of acre to the three types of fruit trees?
[1 tonne = 1000kg]
(a) Formulate a linear programming model to maximize the profit.
(b) Find the optimal solution by using simplex algorithm.
Answers
(a) The linear programming model to maximize the profit is written as,
Maximize Z = 2(4x1)(100) + 1.5(6x2)(100) + 4(2x3)(1000) - 2(100x1 + 100x2 + 50x3) - 40(10x1 + 12x2)
(b) The optimal solution is x1 = 0, x2 = 8/3, x3 = 8, x4 = 0, and the maximum profit is $40.
(a) Linear programming model:
Let x1, x2, and x3 be the number of acres allocated to lemon, peach, and cherry trees, respectively. Then, the objective function we want to maximize is:
Maximize Z = 2(4x1)(100) + 1.5(6x2)(100) + 4(2x3)(1000) - 2(100x1 + 100x2 + 50x3) - 40(10x1 + 12x2)
The first term represents the revenue from selling lemons, the second term represents the revenue from selling peaches, the third term represents the revenue from selling cherries, the fourth term represents the cost of fertilizer, and the last term represents the cost of labor.
The constraints are:
x1 + x2 + x3 ≤ 200 (total available land)
10x1 + 12x2 + 10x3 ≤ 20000 (total available labor hours)
x1, x2, x3 ≥ 0 (non-negativity constraints)
(b) Simplex algorithm:
Convert the linear programming model into standard form by introducing slack variables and expressing all variables in terms of these variables.
Maximize Z = 2(4x1)(100) + 1.5(6x2)(100) + 4(2x3)(1000) - 2(100x1 + 100x2 + 50x3) - 40(10x1 + 12x2)
Subject to:
x1 + x2 + x3 + x4 = 200
10x1 + 12x2 + 10x3 + x5 = 20000
x1, x2, x3, x4, x5 ≥ 0
In this case, the entering variable is x1, as it has the highest coefficient in the objective function.
Step 5 (continued):
1.6 0.1 0 1 -48 32 <- Z
0.4 0.9 1 1/10 0 40 <- x1
8.4 3.3 0 -1/10 1 1840 <- x5
The entering variable is x3, as it has the highest coefficient in the objective function.
The leaving variable is x1, as it has the smallest ratio of RHS to its coefficient in the x3 column.
x2 x1/12 x3 x5/120 x4 RHS
2.5 1/5 0 -1/60 0 40 <- Z
0.5 -1/5 1 1/60 0 8 <- x3
0.5 2/15 0 1/60 1/10 8/3 <- x2
To know more about linear programming model here
https://brainly.com/question/28036767
#SPJ4
Can someone help with an explanation please

Answers
The percentage of the total hours did Andrea work is,
⇒ 27.1%
What is mean by Percentage?A number or ratio that can be expressed as a fraction of 100 or a relative value indicating hundredth part of any quantity is called percentage.
Given that;
Total number of hours to work is,
⇒ 5.8 + 8.1 + 7.3 + 8.7 hours
⇒ 29.9 hours
Here, Andre work 8.1 hours.
Hence, The percentage of the total hours did Andrea work is,
⇒ 8.1 / 29.9 × 100
⇒ 27.1%
Thus, The percentage of the total hours did Andrea work is,
⇒ 27.1%
Learn more about the percent visit:
https://brainly.com/question/24877689
#SPJ1
Using long division method, show that x+2 is a factor of x power 3 + 8
Answers
Using the long division method, it is proved that (x + 2) is a factor of (x³ + 8), because the result of the remainder is 0.
To show that (x + 2) is a factor of (x³ + 8) using long division, we can divide (x³ + 8) by (x + 2) and see if the remainder is 0. If the remainder is 0, then (x + 2) is a factor of (x³ + 8). Here's how the long division would look:
x² - 2x + 4
x+2 | x³ + 0x² + 0x + 8
- (x³ + 2x²)
--------------------
-2x² + 0x + 8
- (-2x² - 4x)
---------------
4x + 8
- (4x + 8)
--------
0
Since the remainder is 0, we can conclude that (x + 2) is a factor of (x³ + 8).
See more about long division at https://brainly.com/question/25289437
#SPJ11
4x(3+5)-10=4x3+5-10=??
Answers
Answer:
x = 17/32
Step-by-step explanation:
Firstly, you want to simplify by combining like terms:
4x(3+5)—10 = 4 · 3 + 5 – 10
32x—10 = 7
Add ten to both sides:
32x - 10 = 7
+10 +10
32x = 17
Divide both sides by 32:
32x = 17
/32 /32
32 divided by 32x makes x
so we get x = 17/32
(we dont divide 17/32 since we would get an irrational number (0.53125))
LE
Majid rents a bicycle to ride around the city.
There is AED 80 deposit plus AED 22 per hour charge.
If Majid pays AED 146, Majid can ride the bicycle for
hour
B
22
12
80
Answers
Answer:
3 hours
Step-by-step explanation:
The rent of the bicycle comes with a charge of:
Initial deposit = AED 80
cost per hour = AED 22
If Majid pays AED 146, then;
AED 146 - AED 80 = AED 66
Given that the charge per hour is AED 22, then to determine the number of hours that Majid can ride;
number of hours = \(\frac{AED 66}{AED 22}\)
= 3
Majid would be able to ride the bicycle for 3 hours when he pays AED 146.
HELP ME PLEASE I don’t want to fail also I’m give Brainlist and 10 points
Answers
Answer:
theres no question or answers
Step-by-step explanation:
Answer:
Where is the question? I don't see it did you forget to attach it?
Step-by-step explanation:
find the measure of the angles indicated

Answers
Answer: 60º
Step-by-step explanation:
With respect to the line ZB, it makes an angle of 180º if measured using DZ. If the angle outside the triangle measures 140º, subtract from 180º to find the angle inside the triangle.
180º-140º=40º
So, now we have angle c=80º and angle b=40º, we can make an equation to find angles D which we'll call x.
The sum of the angles of a triangle must be equal to 180º.
80+40+x=180
120+x=180
x=180-120
x=60
Answer:
Find the measure of the angles indicated
∠CDB = 60°
Step-by-step explanation:
∠CBD + ∠CBZ = 180°
∠CBD + 140° = 180°
∠CBD = 180° - 140°
∠CBD = 40°
∠CBD + ∠BCD + ∠CDB = 180°
40° + 80° + ∠CDB = 180°
120° + ∠CDB = 180°
∠CDB = 180° - 120°
∠CDB = 60°
need help!!!!
No guessing,no links

Answers
Answer:
d = mn/F
Step-by-step explanation:
F = mn/d
multiply both sides with d
d*F = mn/d*d
dF=mn
divide F from both sides
d/F = mn/F
So..
d = mn/F
Answer:
Given:
\( \boxed{ \mathsf{F = \frac{mn}{d} }}\)
Divide both sides by "mn":
\( \mathsf{ \frac{F}{mn} = \frac{mn}{d \times mn} }\)
The two mn's on the RHS gets canceled out. So we're left with:
\( \mathsf{ \frac{F}{mn} = \frac{1}{d} }\)
Invert the fractions present on either side of the "equals" sign
(In other words, flip it on its head so its numerator becomes its denominator and vice):
\( \mathsf{ \frac{mn}{F} = \frac{d}{1} }\)
Hence, we got the value of d:
\( \underline{\mathsf{ d= \frac{mn}{F} }}\)
help please
.............

Answers
Answer:
x ≈ 41.7 ° (a)
Step-by-step explanation:
b² = a² + c² - 2ac × cosβ
~~~~~~~~~~~
25² = 32² + 37² - 2(32)(37)cosx
625 = 2,393 - 2,368 cosx
cosx = \(\frac{-2393+625}{-2368}\) ≈ 0.74662162
x ≈ 41.7 ° (a)
Evaluate. 3x^2 + 5x+1, when x = -2
Answers
Answer:
3
Step-by-step explanation:
Since we know x = -2 we can plug it into the equation
3*-2^2+5*-2+1
Solve!
3*-2^2+5*-2+1 = 3
Hope this helps!
jennifer has three more dimes than nickels and three times as many quarters as nickels. if she has $7.50, how many quarters does she have? www.wyzant
Answers
Jennifer has 48 nickels, which means she has 144 quarters.
Let's call the number of nickels Jennifer has "n".
Since Jennifer has three more dimes than nickels, she has n + 3 dimes.
And since she has three times as many quarters as nickels, she has 3n quarters.
We can start by figuring out the total value of the nickels and dimes in terms of cents:
n * 5 cents + (n + 3) * 10 cents = 750 cents
Expanding the right side of the equation and solving for n:
5n + 10n + 30 cents = 750 cents
15n + 30 cents = 750 cents
15n = 720 cents
n = 48
So Jennifer has 48 nickels, which means she has 3 * 48 = 144 quarters.
You can learn more about finding missing quantity at
https://brainly.com/question/29417721
#SPJ4
1945 men and 2849 women regiter to audition for a inging competition. The number of participant who are not ucceful in their audition what’ five time the number of thoe who are ucceful. How many participant were ucceful
Answers
1945 men and 2849 women register to audition for a singing competition. The number of participants who are not successful in their auditions what’s five times the number of those who are successful. There are 799 participants were successful.
The successful participants can be calculate by solving a linear equation as follows
First, it's crucial to understand linear equations.
Equation connects the two algebraic expressions with an equal to sign to demonstrate the equality between the two algebraic expressions.
Linear equations are those with one degree.
In this case, a linear equation must be resolved.
1945 for the men's total
Women are present in 2849.
Participants in total: 1945 + 2849 = 4794
Let x be the proportion of participants that were successful.
Men who failed were 5 times as numerous.
Participants in total: 5x + x = 6x
Due to the issue,
The linear formula is
6x = 4794
x = 4794/6
x = 799
799 of the participants had success.
To learn more about linear equation, refer to the link-
brainly.com/question/2030026
#SPJ4
What is 164 divided by 5.40
Answers
Answer:
30.37
Step-by-step explanation:
164÷5.40164×100/54016400/540give 30.37Answer:
\(164 \div 5.40 ={\ 30.3704{}}\)
The answer is 30.3704
in a geometrical progression,the product of the 2nd and 4th term is double the the 5th terms. and the sum of the first four terms is 80 find the G.p
Answers
The 15th term of the geometric progression is 9,565,94.
What is the 15th term of the geometric progression?A geometric progression can be expressed as an = a1rn-1 for some a1 and r. You are told that a2 * a4 = 2a5 and a1 + a2 + a3 + a4 = 80.
From the first equation, a2 = a1 r, a4 = a1 r3, and a5 = a1 r4. This tells us that
a1 r a1 r3 = 2 a1 r4
The r's and one a1 cancel leaving a1 = 2.
Now, plugging similarly into a1 + a2 + a3 + a4 = 80,
2 + 2r + 2r2 + 2r3 = 80
r3 + r2 + r + 1 = 40
In general, the easiest way to solve a cubic is by graphing, but here you know the answer will be a small integer, so just try a few. If we try r=3, we get 27 + 9 + 3 + 1 = 40 so r = 3.
So the geometric sequence here is an = 2 3n-1. The 15th term is then:
a15 = 2*3^14
a15 = 9,565,938.
Full question:
A G.P, the product of the 2nd and the 4th term is double the 5th term, and the sum of the first 4th terms is 80. find the 15th term.
Read more about geometric progression
brainly.com/question/24643676
#SPJ1
