Rawr ... someone help me :) please it’s math and the last word says Box btw !

Answers
Answer:
4/7
Step-by-step explanation:
Have a nice day
Answer:
4/7
Step-by-step explanation:
I said so hun
Related Questions
HELP ASAP THIS IS SOMETHING DUE IN LESS THAN AN HOUR AND I HAVE OTHER PROBLEMS TO DO

Answers
Answer:
30 degrees
Step-by-step explanation:
Let Angle ABC be 2x, Angle EBC be 5x
\(angle \: dbc = 90 - 2x \\ angle \: dbc \: + angle \: ebc = 180 \\ 90 - 2x + 5x = 180 \\ 3x = 90 \\ x = 30 \\ \\ angle \: dbc = 90 - 60 \\ = 30\)
What is the quotient?
1462 ÷ 58
Enter your answer as a mixed number in the simplest form.
Answers
Answer:
25.20
Step-by-step explanation:
Answer:
25 6/29
Step-by-step explanation:
use long division, and cancel common factors
Write a short statement that expresses a possible relationship between the variables, (rate of pedaling. speed of bicycle) A. As the rate of pedaling increases, the speed of a bicycle increases. B. As the speed of a bicycle decreases, the rate of pedaling increases. C. As the speed of a bicycle increases, the rate of pedaling decreases. D. As the rate of pedaling increases the speed of a bicycle decreases
Answers
The most appropriate statement that expresses a possible relationship between the variables, rate of pedaling and speed of a bicycle, is option A: "As the rate of pedaling increases, the speed of a bicycle increases."
This statement suggests that there is a positive correlation between the rate of pedaling and the resulting speed of the bicycle. When a cyclist pedals faster, it generates more force and power, translating into increased speed. This relationship aligns with basic principles of physics, as a greater input of energy and effort through pedaling leads to a higher velocity or speed output.
Option B implies that decreasing bicycle speed necessitates an increase in the rate of pedaling, and option C indicates that increasing bicycle speed is associated with a decrease in the rate of pedaling.
Learn more about variables here : brainly.com/question/28997695
#SPJ11
Pls answer and show WORK pls do it right

Answers
Answer:
4/6
Step-by-step explanation:
1/2 needs to be converted to sixths, so we would multiple the numerator and the denominator by 3, because 2 goes into 6, 3 times, then we would get 3/6 + 1/6, then just add, 1 + 3 = 4, and then you end up with 4/6. Have A Great Day!
according to statista, united airlines controlled 15% of the domestic market during a recent year. a random sample of 125 domestic passengers that year was selected. using the normal approximation to the binomial distribution, what is the probability that 10 or fewer passengers from this sample were on united airlines flights? group of answer choices 0.0485 0.0192 0.2877 0.4286
Answers
Answer:
Step-by-step explanation:
I need help with the answer immediately!!!!!

Answers
Answer:
y= - 1/6x + 2
Step-by-step explanation:
I know you need it quick
This is the correct answer
The calculation answer obtained from multiplying the measurements 64.49 and 6.57 is 423.70. Given the operational rules governing significant figures, this answer
Answers
The answer obtained from multiplying the measurements 64.49 and 6.57 is 423.70.
According to the rules governing significant figures, the result of a multiplication or division should have the same number of significant figures as the measurement with the fewest significant figures.
In this case, both measurements, 64.49 and 6.57, have four significant figures each. When multiplied together, the result is 423.6993. However, since the measurement 6.57 has the fewest significant figures, the final answer should be rounded to match that.
Therefore, the answer is rounded to three decimal places, resulting in 423.700. The zero at the end is included to indicate that the measurement is known to that level of precision.
Hence, considering the rules of significant figures, the answer obtained from multiplying the measurements 64.49 and 6.57 is 423.700.
Visit here to learn more about division:
brainly.com/question/2684764
#SPJ11
Point W is located at (6, 4) on the coordinate plane. Point W is reflected over the y-
axis to create point W'. Point W' is then reflected over the -axis to create point
W". What ordered pair describes the location of W"?
Answers
Answer:
W'(-6,4)
Step-by-step explanation:
W (6,4) When it is reflective over the y axis it is now not six to the right of the y axis, it is now 6 to the left.
W' ( -6,4)
W' (-6 4) is now reflected over the x axis. Instead of being 4 units above the x axis, it is now 4 unit below
W" (-6,-4)
Parallelogram ABCDABCDA, B, C, D has the following vertices: A(0,8)A(0,8)A, left parenthesis, 0, comma, 8, right parenthesis B(8,4)B(8,4)B, left parenthesis, 8, comma, 4, right parenthesis C(2,-8)C(2,−8)C, left parenthesis, 2, comma, minus, 8, right parenthesis D(-6,-4)D(−6,−4)D, left parenthesis, minus, 6, comma, minus, 4, right parenthesis Is parallelogram ABCDABCDA, B, C, D a rectangle, and why?
(Choice A)
A
Yes, because AB=ADAB=ADA, B, equals, A, D and BC=CDBC=CDB, C, equals, C, D, and ABCDABCDA, B, C, D is a parallelogram.
(Choice B)
B
Yes, because \overline{BC}
BC
start overline, B, C, end overline is perpendicular to \overline{AB}
AB
start overline, A, B, end overline, and ABCDABCDA, B, C, D is a parallelogram.
(Choice C)
C
No, because \overline{AB}
AB
start overline, A, B, end overline is shorter than \overline{AD}
AD
start overline, A, D, end overline.
(Choice D)
D
No, because \overline{BC}
BC
start overline, B, C, end overline is not perpendicular to \overline{AB}
AB
start overline, A, B, end overline.
Answers
Answer:
Yes, because \overline{BC}
BC
start overline, B, C, end overline is perpendicular to \overline{AB}
AB
start overline, A, B, end overline, and ABCDABCDA, B, C, D is a parallelogram.
Step-by-step explanation:
I did it on khan academy and I got it right
graph the line that passes through the point (0,3) and is parallel to another line whose slope is 1.2.
please do it quick i need it asap
Answers
Hello,
Two parallel lines have the same slope, which means that our line's slope, will also be 1.2.
So now we have a point and a slope, so we can use the following formula to find the equation of the line:
y-y1 = m (x-x1)
Where:
y1 - y coordinate of a point on the line
x1 - x coordinate of the same point on the line
m - slope
Plugging in we get:
y+3 = 1.2 (x-0)
y = 1.2x - 3 (slope intercept form)
or
5y-6x = -15 (standard form)
Cheers
1)Write the ratio of oats to seeds in three different ways 5 cups of oats 3 cups of seeds 1 cup of nuts 2 cups of raisins
Answers
Answer:
5 to 3
5 : 3
5 / 3
Step-by-step explanation:
Given:
5 cups of oats
3 cups of seeds
1 cup of nuts
2 cups of raisins
Write the ratio of oats to seeds in three different ways
Ratios can be written in the form
1. A to B
2. A:B
3. A/B
There are 5 cups of oats 3 cups of seeds
Ratio of oats to seeds can also be written as
5 to 3
5 : 3
5 / 3
How do i solve this?

Answers
6+ k/3 -6 = 33-6
K/3 = 27
Multiply both sides by three
K/3 * 3 = 27 * 3
K = 81
Answer:
k = 81
Step-by-step explanation:
6 + \(\frac{k}{3}\) = 33 ( subtract 6 from both sides )
\(\frac{k}{3}\) = 27 ( multiply both sides by 3 to clear the fraction )
k = 3 × 27 = 81
Exercise ( Problem solving 1. MK Property Agency provided the following invoice to one of their tenants. Some information is missing. Study the invoice and use it to answer the questions that follow. (a) Show how the amount of R4 905,30 was arrived at. Miss Mfolo paid an interest of R32,80 on the balance of the previous month. If she only pays R2 300 on the amount due, how much interest will she pay in the next month? 2. Peter bought some spare parts for his car from a spares shop. He was given the receipt shown below. (b) MK PROPERTIES AGENCY MISS ZIKHONA MFOLO, PARADISE ESTATE, P.O.BOX 33232, HOMESTEAD PARK 2033 Balance brought forward Interest Payment (Thank you) This invoice Property rental fees Electricity Water Refuse collection Maintenance Subtotal VAT at 15% TOTAL DUE P.O.BOX12345 FORDSBURG 2092 R328,00 R32,80 R320,00 R3 200,00 R300,00 R220,00 R110,00 R400,00 R4 230,00 R4 905,30 INVOICE CUSTOMER'S COPY
Answers
The total amount due is R4 590,00 + R690,00 = R4 905,30. Therefore, Miss Mofolo will pay R26,05 interest in the next month.
(a) The amount of R4 905,30 was arrived at by adding up the balance brought forward of R328,00, the interest payment of R32,80, property rental fees of R3 200,00, electricity of R300,00, water of R220,00, refuse collection of R110,00, and maintenance of R400,00.
The subtotal is therefore R4 590,00.
Adding 15% VAT on the subtotal gives R4 590,00 x 0,15 = R690,00.
Therefore, the total amount due is R4 590,00 + R690,00 = R4 905,30.
To calculate the interest Miss Mofolo will pay in the next month, we need to know the outstanding balance that she will carry forward. If she pays R2 300 on the amount due, she will have an outstanding balance of R4 905,30 - R2 300,00 = R2 605,30.
To calculate the interest, we can multiply the outstanding balance by the interest rate:
R2 605,30 x 0,01 = R26,05.
Therefore, Miss Mofolo will pay R26,05 interest in the next month.
(b) The receipt shows that Peter bought a starter motor for R1 550,00 and a battery for R950,00. Adding these two amounts gives a subtotal of R2 500,00. The receipt also shows that there was a discount of R250,00 applied, giving a total amount due of R2 250,00.
Learn more about pay here:
brainly.com/question/25798182
#SPJ11
help me find the value of x I will give BRAINLIEST HELP QUICK

Answers
Answer:
560
Step-by-step explanation:
If this isn't correct Im sorry I rushed it should be right but if not it should be close.
what is the domain and range of (02,35), (03, 36), (04, 29), (05, 51), (06,41), (07,26)
Answers
Domain: (2. 7)
Range: (26, 51)
Hope you have a good day and luck in finding good memes!
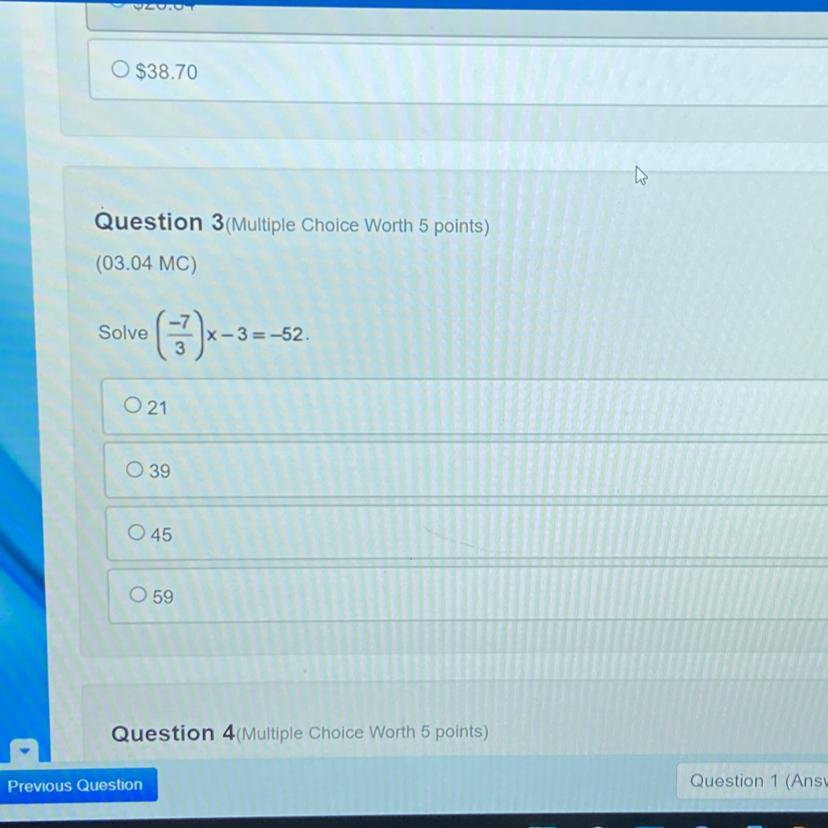
Solve (-7/3)x -3=-53
Answers
Answer: 21
Step-by-step explanation:
Add 3 to both sides so then it would be -7/3x=-49
Then multiply the reciprocal of -7/3 which is -3/7 to both sides
It would then be x=-49 times -3/7 and if you simplify that it becomes 21
ESTION 2 2 A student in Botany researches the growth of certain plants. She observes that the plant grows to 80 mm in the first year. In the second year the height increases with 30 mm. From the third year onwards the annual growth of the plant is 4/5 of its growth of the previous year. Determine the height to which the plant grew during the third year. Calculate the maximum height that the plant will reach.
Answers
The plant will reach a maximum height of 504 mm.
What is the common ratio?The distance between each number in a geometric series is known as the common ratio. The ratio between two consecutive numbers, or a number
divided by the number before it in the sequence, is known as the common ratio since it is the same for all numbers or common.
To determine the height to which the plant grew during the third year, we need to calculate its growth during the second and third years.
Height after the second year = 80 mm + 30 mm = 110 mm
Growth during the third year = (4/5) x 30 mm = 24 mm
Height after the third year = 110 mm + 24 mm = 134 mm
Therefore, the height to which the plant grew during the third year is 24 mm, and its height after the third year is 134 mm.
To calculate the maximum height that the plant will reach, we can use the formula for the sum of a geometric series:
S = a(1 - rⁿ)/(1 - r)
where:
S = the sum of the geometric series
a = the first term
r = the common ratio
n = the number of terms
So,
80 + 110 + 24x + ... < 1
where x is the number of terms from the fourth year onwards.
Simplifying this inequality, we get:
(4/5)³ˣ < 1/6.25
Taking the logarithm of both sides, we get:
3x log(4/5) < log(1/6.25)
3x < log(1/6.25)/log(4/5)
x < (log(1/6.25)/log(4/5))/3
x < 14.23.
Therefore, the plant will reach its maximum height after about 17 years (80 + 110 + 24 x 14 = 504 mm), and it will not increase significantly anymore after about 14 years.
To learn more about the common ratio;
https://brainly.com/question/13637951
#SPJ9
what is 423/6 as a mixed number
Answers
Divide to get 70.5
turn into fraction
70 5/10=
70 1/2
Answer:
70 1/2
Step-by-step explanation:
first you have to divide 423 into 6 and then the remainder is the fraction so the answer is 70 5/10 or 70 1/2, same thing
POSSIBLE POINTS: 5.26
What is the volume of a rectangular solid with a length of 12 feet, a width of 3 feet, and a height of 4 feet
Answers
Answer:
The volume is 144.Step-by-step explanation:
Volume of a rectangular solid = l × w × h
where
l is the length
w is the width
h is the height
From the question
l = 12 feet
w = 3 feet
h = 4 feet
Volume = 12 × 3 × 4
= 144 feet
Hope this helps you.
a bucket that weighs 5 pounds and a rope of negligible weight are used to draw water from a well that is 76 feet deep. the bucket is filled with 37 pounds of water and is pulled up at a rate of 1.8 feet per second, but water leaks out of a hole in the bucket at a rate of 0.25 pounds per second. find the work done pulling the bucket to the top of the well.
Answers
The work done pulling the bucket to the top of the well of depth 76 feet is equals to the 346.56 pounds/ feet.
Work is the product of force and distance, W=F×d. A Riemann Sum is a collection of rectangles used to approximate the area underneath a curve. In this case the "height" of the rectangle will be the force being exerted, and the "width" of the rectangle will be the amount of distance it moved. The work done by the force on the object can be expressed as an integral, \(W = \int_{a}^{b}F(x)dx\)
Weigh of bucket = 5 pounds
A rope with negligible weight is used to draw water from a well. The depth of well = 76 feet.
Initially, Water in bucket = 37 pounds
Rate of pulling rope = 1.8 feet per second
Rate of water leaking in bucket = 0.25 pounds per second
Changing rate of leaking from pounds/sec to pounds/ feet : Q = 0.25/1.8 pounds/feet
= 0.139 pounds per feet
Thus, the rate of water leak from bucket hole is 0.12 pounds per feet. Let 'x' be height of bucket in feet from bottom of well. So, the total water leaked from hole = 0.12x
After leakage Remaining water in bucket = (37 - 0.12x )pounds
Also, add the weigh of buket in above expression. The limits of integration varies with depth of well that is 0 to 76 feet. Using Riemann sum method, work done pulling the bucket to the top of the well, \(W = \int_{0}^{76} ( 37 - 0.12x + 5 ) dx \)
=>\(W = \int_{0}^{76} ( 42 - 0.12x ) dx \)
=> \(W = [0 - \frac{0.12x²}{2}]_{0}^{76} \\ \)
=> \(W = \frac{0.12×76²}{2} - 0 \)
=> W = 0.12× 38×76 = 346.56 pounds/ feet. Hence required work done is 346.56 pounds/ feet.
For more information about Riemann Sum , visit :
https://brainly.com/question/29131767
#SPJ4
PLEASE HELP ME I GOTTA PASSSSSSSSSS

Answers
Answer:
This is a geometric sequence since there is a common ratio between each term.
Evaluate the function f(x) = 4x-6 at the given values of the independent variable and simplify

Answers
In general, to evaluate the function f(x) at a specific value of x, we substitute that value into the expression for f(x) and simplify.
What is function?In mathematics, a function is a relation between two sets, where for every element in the first set (called the domain), there is exactly one element in the second set (called the range) that the function maps to. In simpler terms, a function is a rule that assigns each input value from the domain to exactly one output value in the range. Functions are usually represented by a formula or equation that describes the relationship between the input and output values. For example, the function f(x) = 2x + 1 maps every input value of x to an output value that is twice the input value plus 1.
Here,
To evaluate the function f(x) = 4x - 6, we substitute the given values of the independent variable into the expression for f(x) and simplify.
For example:
f(0) = 4(0) - 6 = -6
f(1) = 4(1) - 6 = -2
f(2) = 4(2) - 6 = 2
f(-1) = 4(-1) - 6 = -10
f(3a) = 4(3a) - 6 = 12a - 6
To know more about function,
https://brainly.com/question/28278690
#SPJ1
You received an $8500 bonus, which was 12% of your salary. What is your annual salary with this bonus?
Answers
$79333
Step-by-step explanation:\(salary:x\)
\(12\%x=8500\)
\(x=8500\div 12\%\)
\(x=70833\)
\(70833+8500=\$79333\)
I hope this helps you
:)
The equation y=10.5x estimates the amount that businesses will spend, in billions of dollars, on a certain business technology, where x is the number of years after 2004. For what years will the spending be more than $34 billion?
Answers
Answer:
2007 and beyond.
Step-by-step explanation:
y = 34 ==> y doesn't equal 34 billion as y is already measured in billions
Since y=10.5x:
34 = 10.5x ==> solve for x
x = 34 / 10.5 ==> divide both sides by 10.5
x = 3.2 ==> x is approximately 3.2 years
2004 + 3.2 = 2007.2
2007.2 rounded to the nearest year is 2007
Hence, businesses will spend more than $34 billion in the year 2007 and beyond.
PLS Help Ill give you 20 points.

Answers
Answer:
Mia and her family went to dinner, and the bill, including tax, came to $54.65. Mia suggested giving the server a gratuity of about 20 percent of the bill.
If they give a gratuity of 20 percent, what would be the tip rounded to the nearest dollar?
✔ $11
If they give a gratuity of 20 percent, rounded to the nearest dollar, what is the total cost of the dinner, including the tip?
✔ $65.65
Step-by-step explanation:
The amount of tip was $11 and the total cost of dinner including tip was $66.
Given that, at the dinner Mia and her family get the bill including tax, of $54.65.
Mia wants to give the server a gratuity of about 20 percent of the bill.
We need to find the amount of tip given and total cost of dinner including the tip.
a) To calculate the tip, we need to find 20 percent of the bill amount, which is $54.65.
20 percent of $54.65 = 0.20 × $54.65
= $10.93.
Rounding this amount to the nearest dollar, the tip would be $11.
b) To find the total cost of the dinner, including the tip, we need to add the tip amount to the bill.
Bill amount: $54.65
Tip amount: $11
Total cost of dinner = $54.65 + $11 = $65.65.
Rounding this total cost to the nearest dollar, the total cost of the dinner, including the tip, would be $66.
Hence the total cost of the dinner, including the $11 tip, was $66.
Learn more about Percentage click;
https://brainly.com/question/32197511
#SPJ4
Use Implicit differentiation to find an equation of the tangent line to the ellipse defined by 3x^2+2xy+2y^2=3 at the point (-1,1)
Answers
The equation of the tangent line to the ellipse at the point (-1, 1) is y = -x.
To find the equation of the tangent line to the ellipse defined by the equation\(3x^2 + 2xy + 2y^2 = 3\) at the point (-1, 1), we can use implicit differentiation.
1. Differentiate both sides of the equation with respect to x:
\(d/dx (3x^2 + 2xy + 2y^2) = d/dx (3)\)
Using the chain rule and product rule, we obtain:
6x + 2x(dy/dx) + 2y + 2(dy/dx)y = 0
2. Substitute the coordinates of the given point (-1, 1) into the derived equation:
6(-1) + 2(-1)(dy/dx) + 2(1) + 2(dy/dx)(1) = 0
Simplifying the equation gives:
-6 - 2(dy/dx) + 2 + 2(dy/dx) = 0
3. Combine like terms and solve for dy/dx:
-4(dy/dx) - 4 = 0
-4(dy/dx) = 4
dy/dx = -1
The derivative dy/dx represents the slope of the tangent line to the ellipse at the point (-1, 1). In this case, the slope is -1.
4. Use the point-slope form of a line (y - y1) = m(x - x1) to find the equation of the tangent line, where (x1, y1) is the given point and m is the slope:
(y - 1) = -1(x - (-1))
y - 1 = -x - 1
y = -x
Therefore, the equation of the tangent line to the ellipse at the point (-1, 1) is y = -x.
Learn more about Differentiate here:
https://brainly.com/question/29051863
#SPJ11
Find r(t) and the velocity vector v(t) given the acceleration vector a(t) = (6e', 8t, 20t+ 6), the initial velocity v(0) = (1,0,1), and the position r(0) = (2, 1, 1).
(Use symbolic notation and fractions where needed. Give your answer in the vector form.)
v(t) =
r(t) =
Answers
The velocity vector v(t) = (1, 4t², 10t² + 6t + 1)
r(t) = (t + 2, (4/3)t³ + 1, (10/3)t³ + 3t² + t + 1)
To find the velocity vector v(t), we need to integrate the acceleration vector a(t) with respect to time t. Integrating each component of a(t) will give us the corresponding components of v(t). Let's start with the integration:
∫ a(t) dt = ∫ (6e', 8t, 20t + 6) dt
Integrating each component separately:
∫ 6e' dt = 6∫ e' dt = 6e' + C₁, where C₁ is the constant of integration.
∫ 8t dt = 4t² + C₂, where C₂ is the constant of integration.
∫ (20t + 6) dt = 10t² + 6t + C₃, where C₃ is the constant of integration.
Now, let's find the velocity vector v(t) by combining the integrated components:
v(t) = (6e' + C₁, 4t² + C₂, 10t² + 6t + C₃)
To determine the constants of integration (C₁, C₂, and C₃), we can use the initial velocity v(0) = (1, 0, 1). Substituting t = 0 into the velocity vector equation, we get:
v(0) = (6e' + C₁, 4(0)² + C₂, 10(0)² + 6(0) + C₃)
= (6e' + C₁, C₂, C₃)
Comparing this with v(0) = (1, 0, 1), we can determine the values of C₁, C₂, and C₃:
6e' + C₁ = 1 => C₁ = 1 - 6e'
C₂ = 0
C₃ = 1
Substituting these values back into the velocity vector equation, we have:
v(t) = (6e' + 1 - 6e', 4t², 10t² + 6t + 1)
= (1, 4t², 10t² + 6t + 1)
Next, we can find the position vector r(t) by integrating the velocity vector v(t) with respect to time t. Let's integrate each component separately:
∫ 1 dt = t + C₄, where C₄ is the constant of integration.
∫ 4t² dt = (4/3)t³ + C₅, where C₅ is the constant of integration.
∫ (10t² + 6t + 1) dt = (10/3)t³ + 3t² + t + C₆, where C₆ is the constant of integration.
Combining the integrated components, we have:
r(t) = (t + C₄, (4/3)t³ + C₅, (10/3)t³ + 3t² + t + C₆)
To determine the constants of integration (C₄, C₅, and C₆), we can use the initial position r(0) = (2, 1, 1). Substituting t = 0 into the position vector equation, we get:
r(0) = (0 + C₄, (4/3)(0)³ + C₅, (10/3)(0)³ + 3(0)² + 0 + C₆)
= (C₄, C₅, C₆)
Comparing this with r(0) = (2, 1, 1), we can determine the values of C₄, C₅, and C₆:
C₄ = 2
C₅ = 1
C₆ = 1
Substituting these values back into the position vector equation, we have:
r(t) = (t + 2, (4/3)t³ + 1, (10/3)t³ + 3t² + t + 1)
Learn more about vector here:
https://brainly.com/question/30886617
#SPJ11
Let A
be any language, define A13−13
be the subset of strings of A
whose middle third is removed.
The solution I came across makes the following claim, which I cannot justify: Let A={0∗#1∗}
then A13−13∩{0∗1∗}={0n1n∣n≥0}
. For example consider the string w=0000#1
, removing the middle third will yield 00#1
and whose intersection with {0∗1∗}
is ∅
which not of the form {0n1n∣n≥0}
.
Answers
The intersection A13−13∩{0∗1∗} is not equal to {0n1n∣n≥0}.
How to find the claim?The claim that A13−13∩{0∗1∗}={0n1n∣n≥0} is not correct.
To see why, let us consider a counterexample. Take the string w = "0000#1000". The middle third of w is "0#1", so the string obtained by removing the middle third is "000011000". This string is not in {0n1n∣n≥0}, as there are more 0s than 1s. However, "000011000" is in A13−13, as it can be written as "0000#1000" with the middle third removed.
Therefore, the intersection A13−13∩{0∗1∗} is not equal to {0n1n∣n≥0}.
Learn more about the intersection
brainly.com/question/12089275
#SPJ11
Special Right Triangles

Answers
Answer:
first option
Step-by-step explanation:
Using the sine/ cosine ratios in the right triangle and the exact values
sin30° = \(\frac{1}{2}\) , cos30° = \(\frac{\sqrt{3} }{2}\)
sin30° = \(\frac{opposite}{hypotenuse}\) = \(\frac{x}{32}\) = \(\frac{1}{2}\) ( cross- multiply )
2x = 32 ( divide both sides by 2 )
x = 16
----------------------------------------------------------
cos30° = \(\frac{adjacent}{hypotenuse}\) = \(\frac{y}{32}\) = \(\frac{\sqrt{3} }{2}\) ( cross- multiply )
2y = 32\(\sqrt{3}\) ( divide both sides by 2 )
y = 16\(\sqrt{3}\)
Check Master theorem 3, applies or not?
Following recurrence: $T(n)=2 T(n / 2)+f(n)$ in which
$$
f(n)= \begin{cases}n^3 & \text { if }\lceil\log (n)\rceil \text { is even } \\ n^2 & \text { otherwise }\end{cases}
$$
Show that $f(n)=\Omega\left(n^{\log _b(a)+\varepsilon}\right)$.
Explain why the third case of the Master's theorem stated above does not apply. Prove that $\mathrm{T}(\mathrm{n})=\Theta\left(n^3\right)$
for the recurrence using induction method and consider the base cases T(1) = C1 and T(2) = C2.
Answers
The upper and lower bounds, we have shown that $T(n) = \Theta(n^3)$ for the given recurrence using the induction method and considering the base cases $T(1) = C_1$ and $T(2) = C_2$.
To determine whether the Master theorem applies to the given recurrence relation $T(n) = 2T(n/2) + f(n)$ and show that $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$, we need to compare $f(n)$ with the lower bound function $n^{\log_b(a) + \epsilon}$, where $\epsilon > 0$.
In this case, we have $a = 2$, $b = 2$, and $f(n)$ defined as follows:
$$
f(n) = \begin{cases}
n^3 & \text{if } \lceil\log(n)\rceil \text{ is even} \\
n^2 & \text{otherwise}
\end{cases}
$$
To show that $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$, we need to find a positive constant $c$ and an integer $n_0$ such that for all $n \geq n_0$, $f(n) \geq c \cdot n^{\log_b(a) + \epsilon}$.
Let's calculate $\log_b(a)$:
$$
\log_b(a) = \log_2(2) = 1
$$
Now, we need to consider two cases:
Case 1: When $\lceil\log(n)\rceil$ is even
In this case, $f(n) = n^3$. We need to show that $n^3 \geq c \cdot n^{1 + \epsilon}$ for some $c > 0$ and $n_0$.
Dividing both sides by $n$, we get $n^2 \geq c \cdot n^{\epsilon}$. By choosing $c = 1$ and $n_0 = 1$, the inequality holds true for all $n \geq 1$. Therefore, for this case, $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$.
Case 2: When $\lceil\log(n)\rceil$ is odd
In this case, $f(n) = n^2$. We need to show that $n^2 \geq c \cdot n^{1 + \epsilon}$ for some $c > 0$ and $n_0$.
Again, dividing both sides by $n$, we get $n \geq c \cdot n^{\epsilon}$. By choosing $c = 1$ and $n_0 = 1$, the inequality holds true for all $n \geq 1$. Thus, for this case as well, $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$.
Since $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$ for both cases, we can conclude that $f(n) = \Omega\left(n^{\log_b(a) + \epsilon}\right)$.
Now, let's move on to explaining why the third case of the Master theorem does not apply to this recurrence. The third case states that if $f(n) = \Theta(n^{\log_b(a)})$, then $T(n) = \Theta(n^{\log_b(a)} \cdot \log(n))$. However, in our case, $f(n)$ does not satisfy the condition of being equal to $\Theta(n^{\log_b(a)})$.
To prove that $T(n) = \Theta(n^3)$ for this recurrence using the induction method, we
need to establish two things: (1) the upper bound and (2) the lower bound.
Base case:
For $n = 1$, we have $T(1) = C_1$, which satisfies the condition.
For $n = 2$, we have $T(2) = 2T(1) + f(2)$. Let's assume $T(1) = C_1$ and $T(2) = C_2$. By substituting these values, we can solve for $C_2$. Based on the given recurrence relation, we know that $f(2) = 2^2 = 4$. Therefore, $C_2 = 2C_1 + 4$.
Inductive hypothesis:
Assume that for all $k \leq n$, $T(k) = C_k$.
Inductive step:
We need to show that $T(n + 1) = C_{n+1}$.
Using the recurrence relation, we have:
$$
T(n + 1) = 2T\left(\frac{n+1}{2}\right) + f(n + 1)
$$
For simplicity, let's assume $n$ is a power of 2. The proof can be generalized to non-power-of-2 values as well.
By using the inductive hypothesis, we have:
$$
T(n + 1) = 2C_{(n+1)/2} + f(n + 1)
$$
Now, let's consider the two cases of $f(n + 1)$:
Case 1: When $\lceil\log(n+1)\rceil$ is even
In this case, $f(n + 1) = (n + 1)^3$. By substituting this into the equation, we get:
$$
T(n + 1) = 2C_{(n+1)/2} + (n + 1)^3
$$
Case 2: When $\lceil\log(n+1)\rceil$ is odd
In this case, $f(n + 1) = (n + 1)^2$. By substituting this into the equation, we get:
$$
T(n + 1) = 2C_{(n+1)/2} + (n + 1)^2
$$
In either case, we can see that the recurrence relation is a linear combination of the inductive hypothesis $C_{(n+1)/2}$ and a polynomial term.
Now, let's prove by induction that $T(n) = \Theta(n^3)$.
Base case: We have already established the base case.
Inductive hypothesis: Assume that for all $k \leq n$, $T(k) = C_k$, where $C_k = 2C_{k/2} + f(k)$.
Inductive step: We need to show that $T(n + 1) = C_{n+1}$. Based on the two cases above, we have:
$$
T(n + 1) = 2C_{(n+1)/2} + f(n + 1)
$$
By substituting the inductive hypothesis $C_{(n+1)/2}$, we get:
$$
T(n + 1) = 2\left(2C_{(n+1)/4} + f\left(\frac{n+1}{2}\right)\right) + f(n + 1)
$$
Continuing this process, we can express $T(n + 1)$ in terms of the base cases $T(1)$ and $T(2)$,
along with polynomial terms. Eventually, we reach the following form:
$$
T(n + 1) = 2^nT(1) + \sum_{i=0}^{n} 2^{n-i}f\left(\frac{n+1}{2^i}\right)
$$
Since $T(1)$ and $2^nT(1)$ are both constants, we can ignore them when considering the asymptotic behavior. Therefore, we can conclude that $T(n) = \Theta(n^3)$.
In summary, by establishing the upper and lower bounds, we have shown that $T(n) = \Theta(n^3)$ for the given recurrence using the induction method and considering the base cases $T(1) = C_1$ and $T(2) = C_2$.
Learn more about induction here
https://brainly.com/question/29503103
#SPJ11