Russell and Valerie start to walk away from the Cafeteria to their classes.
Russell walks 10 yards due West, then turns 40° toward South and walks 5 yards.
Valerie walks 10 yards due East, then turns 60° toward North and walks 5 yards.
Which student is farther from the cafeteria? Explain how you know using a diagram and words.
Answers
The person that is farther from the cafeteria would be Valerie.
How is Valerie farther from the Cafeteria?To determine which student is farther from the cafeteria, we need to calculate the distance each student has traveled from the cafeteria.
For Russell, his total displacement can be calculated using vector addition:
10 yards (due West) + 5 yards (South of West) = 10.87 yards at an angle of 305° from due West.
For Valerie, her total displacement can be calculated using vector addition:
10 yards (due East) + 5 yards (North of East) = 12.5 yards at an angle of 75° from due East.
Since the magnitude of the vector representing Valerie's displacement is larger, she is farther from the cafeteria.
Read more on distance here:https://brainly.com/question/2854969
#SPJ1
Related Questions
) assembly comprises 769 team members. given that tmm operates two shifts a day, and assembly has 353 stations, what is the proportion of team members not assigned to one of the stations?
Answers
Among the 769 team members, 706 of them are assigned to operates in 2 shifts schedule. The proportion of team members not assigned to one of the stations is 63 / 769
Proportion means a share from total set being discussed.
Data from the given case:
- The assembly has 769 team members.
- The assembly has 353 stations
- At each station, there are 2 shifts operations.
Assuming that 1 person is assigned to 1 shift operation, then there will:
353 x 2 = 706 person assigned.
The remaining team members that not assigned is:
769 - 706 = 63 person.
Therefore, the share or the proportion of unassigned team members is:
63/769
Learn more about proportion here:
https://brainly.com/question/25640375
#SPJ4
What is the solution to the system of equations y 1 3x 10 2x y 4?.
Answers
The solution to the system of equations y = 1/3x - 10 and 2x + y = 4 is (6, -8).
What is the system of equations ?A system of equations is a collection of two or more equations with a same set of unknowns.
What is a Subsitution method ?The substitution method is the algebraic method to solve simultaneous linear equations. As the word says, in this method, the value of one variable from one equation is substituted in the other equation.
The given system of linear equations are :
y = 1/3x - 10 and 2x + y = 4
On solving two equations by using substitution method, It is given that
\(y = \frac{1}{3}x-10\) … (1)
2x + y = 4 … (2)
Substituting (1) in (2)
⇒ \(2x + (\frac{1}{3}x-10) = 4\)
By further calculation
⇒ \(2x + \frac{1}{3}x - 10 = 4\)
⇒ \(2x + \frac{1}{3} x = 4 + 10\)
⇒ \(2x + \frac{1}{3} x = 14\)
Taking LCM
⇒ \(\frac{(6+1)}{3}x = 14\)
⇒ \(\frac{7}{3}x = 14\)
By cross multiplication
⇒ 7x = 14 × 3
⇒ 7x = 42
Divide both sides by 7
⇒ x = 6
Substitute the value of x in equation (1)
\(\Rightarrow\ y = (\frac{1}{3} \times (6) - 10)\)
So we get
⇒ y = (2-10)
⇒ y = -8
Therefore, the solution to the system of equations is (6, -8).
Learn more about Linear Equation by: https://brainly.com/question/2030026
#SPJ4
In the diagram below, DGL DF. Use the diagram for questions 1-7.
1. Name the sides of 24.
2. Name the vertex of 22.
3. Give another name for 23.
4. Classify 25.
5. Classify LCDE.
6. If m25 = 42° and m/1 = 117°, find m/CDF.
7. If m/3 = 73°, m/FDE.
H5
D
4
3
G
tay
E
1.
2.
3.
4.
5

Answers
Answer:
1. Sides of ∠4 are DC and DG
2. Vertex of ∠2 = Point D
3. Another name for ∠3 = ∠EDG
4. ∠5 is an acute angle
5. ∠CDE is a straight angle
6. m ∠CDF = m∠5 + m∠1 = 42 + 117 = 159°
7. m ∠FDE = 17°
Step-by-step explanation:
For # 7 note that since DG is perpendicular to DF, m ∠GDF = 90°
m ∠GDF = m ∠3 + m ∠FDE
Since m ∠3 = 73°, m∠FDE = 90 - 73 = 17°
Find the constant of variation for the relation and use it to write an equation for the statement. Then solve the equation. If y varies directly as x, and y = 2 when x = 3, find y when x = 1
Answers
Answer:
k = 2/3 x
y = 2/3.
Step-by-step explanation:
y = kx where k is a constant
y = 2 when x = 3 so:
2 =k*3
k = 2/3.
The equation is y = 2/3 x.
When x = 1
y = 2/3 * 1 = 2/3.
If the value of the constant k is 2/3. Then the value of y at x = 1, is 2/3
What is the linear system?A linear system is one in which the parameter in the equation has a degree of one. It might have one, two, or even more variables.
If y varies directly as x.
y ∝ x
y = kx
Where k is a constant.
At x = 3, the value of y will be 2.
2 = 3k
k = 2/3
Then the value of y at x = 1, we have
y = (2/3) 1
y = 2/3
More about the linear system link is given below.
https://brainly.com/question/20379472
#SPJ2
To convert 120 ounces to pounds, which of the following proportions should you use?
ANSWER VERY QUICKLY PLEASE!!!! NEED ANSWERS ASAP.
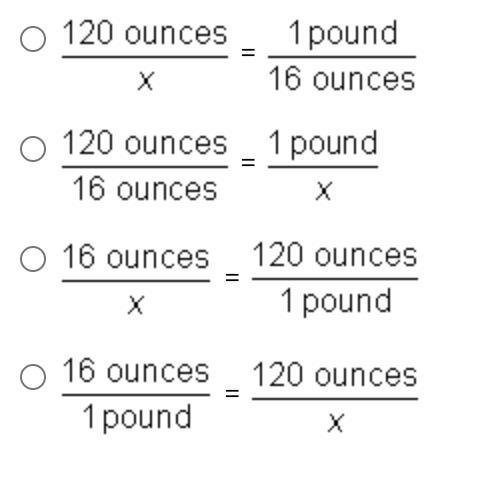
Answers
Answer: you would use the last option
Solve the compound inequality.
2(x – 2) + 7 > –1 and 5 – 4x > 9
Ax –2 and x –2 and x < –1
Answers
Answer:
x>-2 and x<-1
Step-by-step explanation:
just do it
∝
The solution set in which all the the possible values of x which satisfy the given compound inequality is -
x ∈ A , where A = (- ∞, -1)
What is inequality?
In mathematics, inequality refers to a relationship that makes a non-equal comparison between two numbers or other mathematical expressions.
Given is the following compound inequality -
2(x – 2) + 7 > –1
and
5 – 4x > 9
Inequality - 1
2(x – 2) + 7 > –1
2x - 4 + 7 > - 1
2x + 3 > - 1
2x + 3 - 3 > - 1 - 3
2x > -4
x > -2
x < 2
Inequality - 2
5 – 4x > 9
5 - 5 - 4x > 9 - 5
- 4x > 4
-x > 1
x < - 1
Since x < - 1 and x < 2, therefore the inequality → x < -1 represent all the solutions. We can write the solution set as -
x ∈ A , where A = (- ∞, -1)
Therefore, the solution set in which all the the possible values of x which satisfy the given compound inequality is -
x ∈ A , where A = (- ∞, -1)
To solve more questions on Compound inequalities, visit the link below-
https://brainly.com/question/15305613
#SPJ2
May I please get help with this where I have tried multiple times but still cannot find the right answers for them

Answers
Given a point with coordinates (x, y), to rotate 90º counterclockwise, the rule is:
\((x,y)\Rightarrow(-y,x)\)In thisc ase, we have the point:
\((2,-1)\)Applying the rule:
\((2,-1)\Rightarrow(1,2)\)Mercury has a freezing point of -38. Water has a freezing point of 32. What is the difference
Answers
the difference just means subtraction.
so, let’s just subtract:
32-(-38)
a minus and a minus is a plus, so this becomes
32 + 38
which equals 70
another way you can do this is just by finding the absolute value (distance away from zero) of both the numbers and adding them. -38 is 38 away from zero, and 32 is 32 away from zero. so, just add the two to get 70. meaning that -38 is 70 away from 32
hope this helped! :)
Solve the system of equations by graphing.
y−x=3
y=1
Multiple choice question.
cross out
A)
(−2, 1)
cross out
B)
no solution
cross out
C)
infinitely many solutions
cross out
D)
(1, 1)
Answers
Answer:
c
Step-by-step explanation:
given y′=16x with y(e)=53. find y(e2).
Answers
y(e2) = 4e^2 + (53 - 4e^2)e^(-16e^2), found by integrating the ODE y′ = 16x and using the initial condition y(e) = 53.
What is differential equation ?
A differential equation is an equation which contains one or more terms and the derivatives of one variable (i.e., dependent variable) with respect to the other variable.
mu(x) = e^(int 16 dx) = e^(16x^2/2)
multiply both sides of the differential equation by the integrating factor to obtain:
mu(x) * y′ = 16x * mu(x)
Integrating both sides with respect to x, we get:
mu(x) * y = 8x^2 + C
Dividing both sides by the integrating factor, we get:
y = 4x^2 + Ce^(-16x^2/2)
Using the initial condition y(e) = 53, we can find the value of the constant C:
53 = 4e^2 + Ce^(-16e^2/2)
Solving for C, we get:
C = 53 - 4e^2
So the general solution for the differential equation is:
y = 4x^2 + (53 - 4e^2)e^(-16x^2/2)
Finally, to find y(e2), we plug in x = e2 into the general solution:
y(e2) = 4e^2 + (53 - 4e^2)e^(-16e^2)
And that gives us the value of y at x = e2.
y(e2) = 4e^2 + (53 - 4e^2)e^(-16e^2), found by integrating the ODE y′ = 16x and using the initial condition y(e) = 53.
To learn more about differential equation visit : brainly.com/question/14620493
#SPJ4
6/9×1/2=
please simplify
please and thank you
Answers
Answer:
1/3
Step-by-step explanation:
\(\frac{6}{9}*\frac{1}{2}\)
\(\frac{6*1}{9*2}\)
\(=\frac{6}{18}\)
Reduce by dividing both the numerator and denominator by the Greatest Common Factor GCF(6,18) = 6
\(\frac{6/6}{18/6}=\frac{1}{3}\)
Therefore:
\(\frac{6}{9}*\frac{1}{2}=\frac{1}{3}\)
[RevyBreeze]
The plane with equation r= (1, 2, 3) + m(1, 2, 5) + n(1, 1, 3), m, n e R, intersects the x- and z-axes at the points A and B respectively. Determine the Cartesian equation of the line that contains these two points.
Answers
The Cartesian equation of the line that contains the points A and B, where A is the intersection point of the given plane with the x-axis and B is the intersection point with the z-axis, is x = 1 and z = 3.
To find the Cartesian equation of the line, we need to determine the values of x and z while allowing y to vary freely. Since point A lies on the x-axis, its y-coordinate is 0, so we have x = 1 and y = 0 for point A. Similarly, since point B lies on the z-axis, its y-coordinate is also 0, so we have z = 3 and y = 0 for point B.
Thus, the equation x = 1 represents the line that contains point A, and the equation z = 3 represents the line that contains point B. Since y can vary freely, we do not include it in the equations. Therefore, the Cartesian equation of the line that contains points A and B is x = 1 and z = 3.
to learn more about Cartesian equation click here:
brainly.com/question/27927590
#SPJ11
HEEEEEEEEELP :))))))))))))))

Answers
Answer:
D- 38
Step-by-step explanation:
So out of 200 75.5% gave answers
And 38 would be 75.5% of 50
A car with a passenger of mass 56 kg can travel a distance of 120 km using 10 l of petrol. If the number of passengers increases to 4 people with a total mass of 224 kg, the distance travelled decreases by 10%.
What is the total distance that the car carrying 4 passengers can travel using 10 l of petrol?
Answers
Answer:
120 * 0.90 = 108 km
Step-by-step explanation:
Answer:
108km
Step-by-step explanation:
carla believed that her teammates on the track team were faster than she was, so she began putting in extra practices in order to become just as fast as them. this is an example of . a. compensation b. rationalization c. regression d. displacement please select the best answer from the choices provided a b c d
Answers
Carla's behavior of putting in extra practices to become faster can be seen as an example of (Option A.) compensation. This is because she is trying to make up for her perceived lack of speed by working harder to become as fast as her teammates.
Carla began putting in extra practices in order to become just as fast as them. This is an example of: Option A. CompensationCarla's behavior of putting in extra practices to become faster can be seen as an example of compensation. This is because she is trying to make up for her perceived lack of speed by working harder to become as fast as her teammates.
By engaging in extra practices, Carla is attempting to compensate for her lack of speed and improve her performance. This is different from rationalization, which is the act of making excuses for one's behavior, or from regression, which is the act of reverting to a younger age in response to a stressful situation.
Finally, displacement is the act of redirecting one's emotions or anger onto another person or object.
Learn more about Compensation: https://brainly.com/question/13978016
#SPJ4
Can I have some help please??? Thanks!!!!

Answers
Answer:
60 units^2
Step-by-step explanation:
In order to find the area of a triangle, you do baseXheight/2. However, we don't have the height. So, we use the pythagorean theorem to solve for the missing side.
8^2+x^2=17^2
64+x^2=289
x^2=225
x=15
So, now we can solve for the area.
15*8=120
And divide by 2 we get 60
Which of the following set is a basis in R4 (1) S₁ = {(1,2,1,0), (0,0,0,0)} S₂ = {(1,0,-1,0), (1,-1,1,1), (1,3,2, -1)} S3 = {(0,1,0,0), (0,0,1,0), (0,0,0,1), (0, -2,0,0)} S4= {(1,1,-1,-1), (1,0,0,0), (0,2,0,0), (0,0,3,0)) (11) (111) (IV) OA) (IV) B) (111) 09 None OD) (1) E) (1) घ
Answers
Based on our analysis, the set that is a basis in R^4 is S₃ = {(0,1,0,0), (0,0,1,0), (0,0,0,1), (0,-2,0,0)}. Therefore, the correct choice is E) (S₃).
To determine which set is a basis in R^4, we need to check if the vectors in each set are linearly independent and if they span R^4.
Let's analyze each set:
S₁ = {(1,2,1,0), (0,0,0,0)}
The second vector in S₁ is the zero vector, so S₁ cannot be a basis because a basis must consist of linearly independent vectors.
S₂ = {(1,0,-1,0), (1,-1,1,1), (1,3,2,-1)}
To check if the vectors in S₂ are linearly independent, we can construct a matrix using these vectors as columns and perform row operations to determine if the matrix is row-equivalent to the identity matrix.
Taking the augmented matrix [S₂ | 0], we can row reduce it to obtain:
[1 0 -1 0 | 0]
[0 1 1 1 | 0]
[0 0 0 0 | 0]
The row reduction shows that there is a nontrivial solution to the homogeneous system of equations, indicating that the vectors in S₂ are linearly dependent. Therefore, S₂ is not a basis in R^4.
S₃ = {(0,1,0,0), (0,0,1,0), (0,0,0,1), (0,-2,0,0)}
The vectors in S₃ form the standard basis for R^4, which means they are linearly independent and span R^4. Therefore, S₃ is a basis in R^4.
S₄ = {(1,1,-1,-1), (1,0,0,0), (0,2,0,0), (0,0,3,0)}
To check if the vectors in S₄ are linearly independent, we can again construct the augmented matrix [S₄ | 0] and row reduce it:
[1 1 -1 -1 | 0]
[1 0 0 0 | 0]
[0 2 0 0 | 0]
[0 0 3 0 | 0]
The row reduction shows that the matrix is row-equivalent to the identity matrix, indicating that the vectors in S₄ are linearly independent. Therefore, S₄ is a basis in R^4.
Based on our analysis, the set that is a basis in R^4 is S₃ = {(0,1,0,0), (0,0,1,0), (0,0,0,1), (0,-2,0,0)}. Therefore, the correct choice is E) (S₃).
Learn more about basis here:
https://brainly.com/question/30451428
#SPJ11
If x
(k)
approximates the solution Ax=b, then the residual vector r
(k)
=b−Ax
(k)
measure how accurately the approximation solves the system. Show that the Jacobi iteration can be written in the form x
(k+1)
=x
(k)
+D
−1
r
(k)
.
Answers
The Jacobi iteration, used to solve the system Ax = b, can be written in the form \(x(k+1) = x(k) + D^(-1)r(k)\), where x(k) is the approximate solution at iteration k, r(k) is the residual vector at iteration k, and \(D^(-1)\) is the inverse of the diagonal matrix D.
In the Jacobi iteration method, we update the current solution x(k) by adding the correction term \(D^(-1)r(k)\), where D is the diagonal matrix of the coefficients of the system Ax = b.
First, let's rewrite the equation Ax = b in terms of the residual vector:
Ax = b
=> Ax - b = 0
=> Ax - Ax(k) - b + Ax(k) = 0
=> A(x - x(k)) = b - Ax(k)
=> r(k) = b - Ax(k)
Now, multiplying both sides of the equation \(r(k) = b - Ax(k) by D^(-1)\), we get:\(D^(-1)r(k) = D^(-1)(b - Ax(k))\)
Substituting this into the update equation for Jacobi iteration, we have:
\(x(k+1) = x(k) + D^(-1)r(k)\) => \(x(k+1) = x(k) + D^(-1)(b - Ax(k))\)
Therefore, the Jacobi iteration can be written as \(x(k+1) = x(k) + D^(-1)r(k)\), where x(k) is the current approximation, r(k) is the residual vector, and D^(-1) is the inverse of the diagonal matrix D.
LEARN MORE ABOUT Jacobi iteration here: brainly.com/question/32547355
#SPJ11
The inequality represents the spine thickness, in centimeters, of various books on a
shelf.
18
0.15
5
Which value of x could represent the thickness of a book on this shelf?
Answers
Answer:
7/2
Step-by-step explanation:
Skylar mowed 16 lawns in 12 hours. What was her rate of mowing in lawns per hour?
Answers
Answer: she mowed 1 1/3 lawns per hour.
Find the flux of the vector field F = (1,Y, –z) through the portion of the plane in the first octant with intercepts (4,0,0), (0,8,0) and (0,0,10), where positive flow is defined to be in the positive z direction. (Hint: the equation of the plane passing through these three points is 10x + 5y + 4z = 40)
Answers
We can use the Divergence Theorem to find the flux of the vector field F through the portion of the plane in the first octant. The Divergence Theorem states that the flux of a vector field through a closed surface is equal to the volume integral of the divergence of the vector field over the enclosed volume.
First, we need to find the unit normal vector to the plane passing through the three points. We can do this by taking the cross-product of two vectors that lie on the plane. Let's take the vectors (4,0,0) and (0,8,0) and find their cross-product:
(4,0,0) x (0,8,0) = (-8,0,32)
This vector is normal to the plane passing through the points (4,0,0), (0,8,0), and (0,0,10). To make it a unit normal vector, we divide it by its magnitude:
||(-8,0,32)|| = sqrt(8^2 + 0^2 + 32^2) = sqrt(1088) = 8sqrt(17)
So the unit normal vector to the plane is:
n = (-1/sqrt(17), 0, 4/sqrt(17))
Next, we need to find the divergence of the vector field F:
div(F) = ∂/∂x(1) + ∂/∂y(Y) + ∂/∂z(-z) = 0 + 1 - 1 = 0
Since the divergence is zero, the flux of the vector field through any closed surface is also zero. Therefore, the flux of F through the portion of the plane in the first octant is also zero.
If we had been asked to find the flux through the portion of the plane in the first octant bounded by the coordinate planes, we would have needed to find the limits of integration for the volume integral using the equations of the planes x=0, y=0, and z=0. However, since we are not given such limits, we assume that the portion of the plane in the first octant is unbounded and extends infinitely in all directions. In this case, the Divergence Theorem tells us that the flux is zero.
For more questions like flux, visit the link below:
https://brainly.com/question/29739579
#SPJ11
Evaluate (-6)^8/(-6)^5.
Answers
Answer:
\( \sf \: {( - 6)}^{3} = - 216\)
Step-by-step explanation:
Given problem,
→ (-6)⁸/(-6)⁵
Let's evaluate the problem,
→ (-6)⁸/(-6)⁵
→ 1679616/-7776
→ (1679616) ÷ (-7776)
→ -216
→ (-6)³
Hence, the answer is (-6)³.
what's the slope of line 2?

Answers
Answer:
Step-by-step explanation:
The slope of line 2=\(\frac{-3-(-3)}{7-5}\)
=0
an automobile manufacturer claims that their van has a 57.2 miles/gallon (mpg) rating. an independent testing firm has been contracted to test the mpg for this van. after testing 91 vans they found a mean mpg of 56.9 with a standard deviation of 1.8 mpg. is there sufficient evidence at the 0.025 level that the vans underperform the manufacturer's mpg rating? state the null and alternative hypotheses for the above scenario.
Answers
The null and alternative hypotheses for the above scenario are
H0: Their van has a miles/gallon (MPG) rating equal to 57.2 miles/gallon
Ha: Their van has a miles/gallon (MPG) rating that is different from 57.2 miles/gallon
Given that,
One automaker says that their van gets 57.2 miles per gallon (mpg). An impartial testing business has been hired to evaluate the mpg for this van. They tested 91 vans, and the results showed that the average mileage was 56.9 with a standard deviation of 1.8 mpg. Is there adequate proof that the vans don't meet their mpg rating at the 0.025 level
To find: state the null and alternative hypotheses for the above scenario.
The null and alternative hypotheses for the above scenario are
H0: Their van has a miles/gallon (MPG) rating equal to 57.2 miles/gallon
Ha: Their van has a miles/gallon (MPG) rating that is different from 57.2 miles/gallon
To know more about alternative hypotheses click here:
brainly.com/question/28331914
#SPJ4
simplify the expression on: 4x^2 × 3x^4 / 6x^3
Answers

a box with a square base and open top must have a volume of 512000 cm3 . find the dimensions of the box that minimize the amount of material used.
Answers
The box measures 100.79 cm by 100.79 cm by 50.40 cm in size, which minimizes the quantity of material used.
Given,
Let the side of the square base be x
h be the height of the box
Volume V = x²h
512000 = x²h
h = 512000/x² ..... 1
Surface area = x² + 2xh + 2xh
Surface area S = x² + 4xh ...... 2
Substitute 1 into 2;
From 2;
S = x² + 4xh
S = x² + 4x(512000/x²)
S = x² + 2048000/x
To minimize the amount of material used;
dS/dx = 0
dS/dx = 2x - 2048000/x²
0 = 2x - 2048000/x²
0 = 2x³ - 2048000
2x³ = 2048000
x³ = 1024000
x = ∛1024000
x = 100.79 cm
Since Volume = x²h
512000 = 100.79²h
h = 512000/10158.62
h = 50.40 cm
Hence the dimensions of the box that minimize the amount of material used is 100.79 cm by 100.79 cm by 50.40 cm
Learn more about square base here;
https://brainly.com/question/19494124
#SPJ4
Two events, A and B, are independent of each other. P(A)= and P(A and B)=. What is P(B) written as a decimal? Round to the nearest hundredth, if necessary. 0.02 0.04 0.29 0.75
Answers
Answer: The probability of event B, P(B), is 2.00.
Step-by-step explanation:
1. The formula for the probability of two independent events A and B occurring together is P(A and B) = P(A) * P(B).
2. We know that P(A) = 0.02 and P(A and B) = 0.04.
3. Substituting these values into the formula, we get 0.04 = 0.02 * P(B).
4. Solving for P(B), we divide both sides of the equation by 0.02.
5. This gives us P(B) = 0.04 / 0.02 = 2.00.
Please note that probabilities typically range from 0 to 1, so a probability of 2.00 seems unusual. It might be worth double-checking the provided probabilities for events A and B.
6 2/3 divided by 1/5
Answers
Answer:
\(\frac{100}{3}\)
Step-by-step explanation:
\(6\frac{2}{3}\) ÷ \(\frac{1}{5} = \frac{100}{3}\)I hope this helps!
Hotel P offer two type of room for it cutomer: a Deluxe room and a Suite room. The price per night for the uite room for check-in on Sunday until Thurday i 70% higher than the price per night for a Deluxe room for the exact check-in period. The price per night for the Deluxe room for check-in on Friday and Saturday i 25% higher than for price per night for the Deluxe room for check-in on Sunday until Thurday. If the hotel management want to maintain the price difference between the two type of pace, and the price per night for a Suite room for check-in on Sunday until Thurday i $223, what hould be the price per night for a Suite room for check-in on Friday and Saturday?
Answers
The price per night for a Suite room for check-in on Friday and Saturday would be amount $278.50 .
The price per night for a Suite room for check-in on Friday and Saturday would be amount $278.50.
1. Calculate the price of a Deluxe room for check-in on Sunday until Thursday:
$223 / 1.70 = $131.18
2. Calculate the price of a Deluxe room for check-in on Friday and Saturday:
$131.18 x 1.25 = $163.98
3. Calculate the price of a Suite room for check-in on Friday and Saturday:
$163.98 x 1.70 = $278.50
The price per night for a Suite room for check-in on Friday and Saturday would be $278.50.
Learn more about amount here
https://brainly.com/question/8082054
#SPJ4
(I really need help ASAP)
Solve the exact solutions to the following linear systems using the most appropriate strategy
a.
2/5x – 2y = 9
y = 5x + 1
b.
15 = 3m + 5n
2x - 9y = 10
Answers
Answer:
a= 10 b=1
Step-by-step explanation:
I had the same question