Seventy-five increased by a number - What Mathematical operation is used?
A- Multiplication
B-Division
C-Subtraction
Answers
Answer:
a
Step-by-step explanation:
I think it's A so I said A
Answer:
the correct answer is C
Step-by-step explanation:
Related Questions
What's the total charge for a meal that cost $47.5 if the tax it 8%?
Answers
To determine the total charge we first need to calculate how much we pay of taxes. This can be done by using a rule of three:
\(\begin{gathered} 47.5\rightarrow100 \\ x\rightarrow8 \end{gathered}\)Then we have:
\(x=\frac{8\cdot47.5}{100}=3.8\)Hence charge for the tax is $3.8.
Therefore the total charge of the meal is $51.3
Help need it ASAP) In a survey of 2,900 people who owned a certain type of car, 580 said they would buy that type of car again. What percent of the people surveyed were satisfied with the car?
Answers
Answer:
20%
Step-by-step explanation:
580 /2,900 x 100% = 20%
Hope this helps! If not then sorry. :)
our friend sings every saturday night at your favorite bar in san diego. during his show, people show up at the rate of 1 person every minute. a) [4 points] what is the probability that on a random minute, at least 3 people show up?
Answers
If people show up at rate of 1 person every minute , then the probability that on a random minute , at least 3 people show up is 0.084 .
The number of people showing up at bar in San Diego during any random minute is a Poisson process with an average rate of 1 person per minute.
Let X = number of people who show up during a random minute.
Then X will follows a Poisson distribution with parameter λ = 1.
So , probability of at least 3 people showing up in a random minute is:
⇒ P(X ≥ 3) = 1 - P(X < 3)
⇒ 1 - (P(X = 0) + P(X = 1) + P(X = 2))
By using Poisson probability mass function for finding P(X = k) for k = 0,1,2 ;
⇒ P(X = k) = (\(e^{-\lambda}\))×(\(\lambda ^{k}\))/k!
Substituting in λ = 1, we get ;
⇒ P(X = 0) = (\(e^{-1}\))×(\(1 ^{0}\))/0! = 1/e ;
⇒ P(X = 1) = (\(e^{-1}\))×(\(1 ^{1}\))/1! = 1/e ;
⇒ P(X = 2) = (\(e^{-1}\))×(\(1 ^{2}\))/2! = 1/2e ;
So, the probability of at least 3 people showing up in a random minute is:
⇒ P(X ≥ 3) = 1 - (P(X = 0) + P(X = 1) + P(X = 2))
= 1 - (1/e + 1/e + 1/2e) = 1 - (2/e + 1/2e)
= 1 - (5/2e)
= 0.083019 ≈ 0.084 .
Therefore , the required probability is 0.084 .
Learn more about Probability here
https://brainly.com/question/29251004
#SPJ4
The given question is incomplete , the complete question is
Your friend sings every Saturday night at your favorite bar in San Diego. During his show, people show up at the rate of 1 person every minute.
What is the probability that on a random minute, at least 3 people show up ?
The mean cost of a box of Cheerios Oat Crunch is $4.00 and the variance is .35. If the price increases by 25 cents a box, what is the new variance?
Answers
The new variance is 0.35.
The variance of a random variable measures how spread out the values are from the mean.
The formula for the variance is Var(X) = E[(X - E[X])²], where E[X] is the expected value (or mean) of X.
If the price of a box of Cheerios Oat Crunch increases by 25 cents, the new mean cost would be $4.25. To find the new variance, we can use the formula Var(aX + b) = a²Var(X), where a is a constant and b is a constant. In this case, a = 1 (because we are not changing the units of measurement) and b = 0.25 (because we are adding 25 cents to the price).
Therefore, the new variance is Var(X + 0.25) = Var(X) = Var(X) x 1² = Var(X) = 0.35.
This means that the variance remains the same even though the price has increased. This is because the variance is a measure of how spread out the values are from the mean, and the mean has shifted by the same amount as the price increase. Therefore, the distance between the values and the new mean is the same as the distance between the values and the old mean, so the spread is unchanged.
The variance is a measure of how spread out a set of data is from its mean. It is calculated as the average of the squared differences between each value and the mean. The formula for variance is Var(X) = E[(X - E(X))²], where E is the expected value or mean of X.
In this case, we are given that the mean cost of a box of Cheerios Oat Crunch is $4.00 and the variance is 0.35. This means that the average squared difference between each box's cost and the mean cost is 0.35.
Now, if the price of each box increases by 25 cents, this means that the new mean cost will be $4.25. However, this does not affect the variance directly. This is because the variance only measures how spread out the data is from the mean, and the mean has shifted by the same amount as the price increase.
To see this more clearly, imagine a set of data that has a mean of 4 and a variance of 0.35. If we add 0.25 to each value, we get a new set of data that has a mean of 4.25. However, the spread of the data is still the same; that is, the distance between each value and the new mean is the same as the distance between each value and the old mean. Therefore, the variance remains the same even though the price has increased.
In summary, the variance measures how spread out a set of data is from its mean, and a shift in the mean does not affect the variance directly.
To know more about variance click here:
brainly.com/question/16023611
#SPJ4
What does congruent mean square?
Answers
Answer:
All the sides of the square are equalso the squares are congruent.. Are congruent shapes? Two shapes that are the same size and the same shape are congruent.
hope this helps
mark me brainliest
Step-by-step explanation:
Factorise the following:
x2 + 7x + 12
Answers
Answer:
(x+4)(x+3)
Step-by-step explanation:
x^2+4x+3x+12
take x out of each
x(x+4)+3x+12
take 3 out
x(x+4)+3(x+4)
(x+3)(x+4)
Give an example for following statements. (1)Give a 4 x 4 matrix which is not diagonalizable. (2) Find a 3 x 3 diagonalizable matrix with X = 1 is an eigenvalue of multiplicity larger (or equal) than 2. • (3)Find a 2 × 2 nondiagonalizble matrix with λ = -1 be the only eigenvalue.
Answers
The elements of a square matrix that do not sit on the leading diagonal are known as the matrix's non-diagonal elements. These elements are positioned off the matrix's main diagonal.
(1)An example of a 4 x 4 matrix that is not diagonalizable is [0, 1, 0, 0; 0, 0, 1, 0; 0, 0, 0, 1; 0, 0, 0, 1]. This matrix has an eigenvalue of 1 with an algebraic multiplicity of 3 and a geometric multiplicity of 2.
(2) An example of a 3 x 3 diagonalizable matrix with X = 1 is an eigenvalue of multiplicity larger (or equal) than 2 is[1, 0, 0; 1, 1, 0; 0, 1, 1]. The characteristic polynomial of this matrix is given by (λ − 1)^3, hence the eigenvalue 1 has algebraic multiplicity 3. We can see that the eigenspace corresponding to the eigenvalue 1 has dimension 2, meaning that the matrix is diagonalizable and that the eigenvectors are given by [1; 0; 0], [0; 1; 0], and the linear combination of these two vectors [1; 1; 1].
(3) An example of a 2 × 2 non-diagonalizable matrix with λ = -1 be the only eigenvalue is [1, 1; 0, 1]. This matrix has an algebraic multiplicity of -1 with a geometric multiplicity of 1.
To know more about the diagonalizable visit:
https://brainly.com/question/30481023
#SPJ11
how many degrees of freedom are there in a real symmetric matrix, a real diagonal matrix, and a real orthogonal matrix? (hint: the first answer is the sum of the other two, because a
Answers
The number of degrees of freedom in a real symmetric matrix is equal to the number of unique entries in the matrix.
If a matrix is n x n, then it has n^2 total entries. However, since it is symmetric, the diagonal entries are repeated, leaving (n^2 + n) / 2 unique entries. Therefore, the number of degrees of freedom in a real symmetric matrix is (n^2 + n) / 2.
The number of degrees of freedom in a real diagonal matrix is simply the number of diagonal entries, which is equal to the dimension of the matrix. Therefore, if the matrix is n x n, the number of degrees of freedom is n.
Finally, the number of degrees of freedom in a real orthogonal matrix is equal to the number of independent variables needed to specify the matrix. An orthogonal matrix is defined as a matrix whose columns are orthonormal, which means that they are unit vectors that are orthogonal (perpendicular) to each other. Since each column must be a unit vector, there are n independent variables needed to specify the first column (the magnitude and direction), n-1 independent variables needed to specify the second column (since it must be orthogonal to the first), and so on. Therefore, the total number of degrees of freedom in a real orthogonal matrix is the sum of the integers from 1 to n-1, which is (n-1)n/2.
Using the hint given in the question, we can add up the number of degrees of freedom in a real diagonal matrix and a real orthogonal matrix to get (n + (n-1)n/2), which simplifies to (n^2 + n)/2, which is the same as the number of degrees of freedom in a real symmetric matrix.
To learn more about symmetric matrix, click here:
brainly.com/question/14405062
#SPJ11
Pam and Erin are both planting gardens for their backyards. They want their gardens to be proportional. If pams garden has a length of 16 feet and a width of 12 feet, what length does Erin need if the width of her garden is 21 feet?
Answers
Step-by-step explanation:
Pam and Erin want the gardens to be proportional.
proprtional- equal
Pams garden has a length of 16 and width of 12. To find the area of Pam's garden multiply the length by the width.
16×12= 192
Now that we know the area of Pam's garden, we can set up and equation for Erin's garden. Since they have to be equal we know that Erin's garden should equal 192. Thus we solve for the length by dividing the width from the total.
192÷21=9.14
37. Watering a lawn requires 623 gallons of water forevery 1000 sq. ft. What is the rate of gallons per squarefoot?
Answers
The Solution:
Given:
\(1000\text{ sq ft = 623 gallons}\)We are asked to find the rate of gallons per square foot.
\(\begin{gathered} 1000\text{ sq ft = 623 gallons} \\ \text{ Dividing both sides by 1000, we get} \\ 1\text{ sq ft=}\frac{623}{1000}\text{gallons}=0.623\text{ gallon} \end{gathered}\)Therefore, the correct answer is 0.623 gallon.
A license plate consists of two letters followed by two digits; for example, $MP78$. Neither the digits nor the letters may be repeated, and neither the letter $O$ nor the digit $0$ may be used. When reading from left to right, the letters must be in alphabetical order and the digits must be in increasing order. How many different license plate combinations are possible
Answers
If in a number plate two different alphabets need to be selected then there are 650 such number plates that can be formed in such a way that the alphabets come in increasing order.
Given that a number plate can be formed using 2 alphabets which must come in increasing order.
We are required to find the number of plates that can be formed.
Number of total alphabets=26.
The number of plates will be equal to the number of ways in which two alphabets can be arranged.
Combination is the number of ways in which some combinations can be formed. It is expressed as n\(C_{r}\)=n!/r!(n-r)!
Number of license plates=26\(C_{1}\)*25\(C_{1}\)
=26*25
=650 plates
Hence If in a number plate two different alphabets need to be selected then there are 650 such number plates that can be formed in such a way that the alphabets come in increasing order.
Learn more about combinations at https://brainly.com/question/11732255
#SPJ4
Please answer! thankss

Answers
Answer:
48
Step-by-step explanation:
since this is a linear Angle, the total sum will be 180. Given Angle is 132. so answer will be:
180-132= 48
Answer:
n = 48°Step-by-step explanation:
The sum of adjacent angles on a straight line is 180°. i.e. In the figure, we are given is a straight line, then n and 132° are the adjacent angles.
n + 132° = 180
n = 180 - 132
n = 48
Hence, the measure of the unknown angle is 48°.
what is the quotient of 5/8 divided by 1/4
Answers
Answer:
20/8 or 5/2
Step-by-step explanation:
5/8 times 4/1
Find the value of t and u

Answers
Answer:
option b , t = 4 \(\sqrt{2}\) , u= 7
Step-by-step explanation:
sin 45 = 1/\(\sqrt{2}\)
4 / t =1/\(\sqrt{2}\)
t = 4\(\sqrt{2}\)
u = 4+3 = 7
Find the function f(x) = (x^2 - 2)(x^2 - √2) find the value(s) of x in which f’(x) = 0. to the hundredths place.
Answers
The value(s) of x in which f’(x) = 0 are x = 0 and \(x= ^+_-\sqrt{2+\sqrt2}\) to the hundredth place.
First we need to find the derivative of f(x) for that we can use the product rule:
we know that \(f(x) = (x^2 - 2)(x^2 - \sqrt2)\) so the first derivative f'(x) is equal to:
\(f'(x) = [(x^2 - 2)(2x)] + [(x^2 - \sqrt2)(2x)]\)
after simplifying the derivative further, we get:
\(f'(x) = 2x(x^2 - \sqrt2 - 2)\)
We need to find the value of x for which the function f(x) =0:
So we can set f'(x) to zero and solve for x to find what is the value of x that satisfies the given equation.
\(2x(x^2 - \sqrt2 - 2) = 0\)
Therefore, either 2x = 0 (i.e., x = 0) or \(x^2 - \sqrt2 - 2\) = 0.
To solve for x in the second equation we can add 2 and \(\sqrt2\) to both sides and then take the square root of both sides:
\(x^2 = 2 + \sqrt2\\x = ^+_- \sqrt{2 + \sqrt2}\)
Therefore, the value(s) of x in which f’(x) = 0 are x = 0 and \(x= ^+_-\sqrt{2+\sqrt2}\) to the hundredth place.
Learn more about derivatives at:
https://brainly.com/question/28376218
#SPJ1
Pls help ill give brainliest.
Do all 3 to get brainliest pls

Answers
Problem 1
A = length times width
A = w(w + 0.6)
A = w^2 + 0.6w
Answer choice D.
Problem 2
16/9 = 42/x
9(42) = 16x
378 = 16x
378/16 = x
23.625 = x
Answer choice C.
Problem 3
f(-5) = 4(-5) + 3
f(-5) = -20 + 3
f(-5) = -17
Answer choice B.
Solve for x. Round to the nearest tenth.
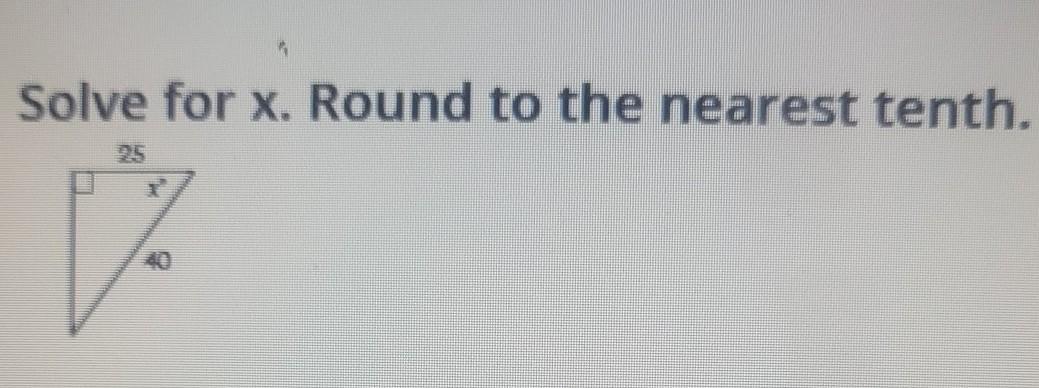
Answers
Using trigonometric function the value of x is 51.3°.
What is trigonometric function?
The functions of an angle in a triangle are known as trigonometric functions, commonly referred to as circular functions. In other words, these trig functions provide the relationship between a triangle's angles and sides. There are five fundamental trigonometric functions: sine, cosine, tangent, cotangent, secant, and cosecant.
Here Let us take the given right triangle as ABC.
∠A = x , ∠B = 90° and AB = 25 , AC= 40
Now using Cosine ratio then
Cos A = \(\frac{adjacent}{hypotenuse}\)
=> cos x = \(\frac{25}{40}\)
=> cos x = \(\frac{5}{8}\)
=> x = \(cos^{-1}\frac{5}{8}\)
=> x = 51.3°
Hence the value of x is 51.3°.
To learn more about trigonometric function refer the below link
https://brainly.com/question/25618616
#SPJ1
On one day at a local minigolf course, there were 320 customers who paid a total of $2,900. If the cost for a child is $7 per game and the cost for an adult is $10 per game, write a system of equations to model this scenario, where x represents the number of children and y represents the number of adults who played that day.
Answers
Answer: We can write a system of equations to model this scenario using the information given.
Let x represent the number of children who played minigolf that day, and let y represent the number of adults who played minigolf that day.
The first equation represents the total number of customers, which is the sum of the number of children and the number of adults.
x + y = 320
The second equation represents the total amount of money earned, which is the product of the number of children playing and the cost per child's game plus the product of the number of adults playing and the cost per adult's game.
7x + 10y = 2900
So the system of equations that models this scenario is:
x + y = 320
7x + 10y = 2900
We can use this system of equations to find the number of children and adults who played minigolf that day, given the total number of customers and total amount of money earned. By solving this system of equations we can find the values for x and y.
Step-by-step explanation:
What is the image of the point (-9,3) after a rotation of 180° counterclockwise
about the origin?
Answers
Answer:
(9, - 3 )
Step-by-step explanation:
Under a counterclockwise rotation about the origin of 180°
a point (x, y ) → (- x, - y ) , thus
(- 9, 3 )→ (9, - 3 )
You are 12.00 m to the North (+y) of and −5.00 m to the East (+x) of the center of town. i. How far are you from the center of town, and ii. at what angle?
Answers
You are located 12.00 m north and 5.00 m east of the center of town. Using Pythagorean theorem, your distance from the center of town is 13.00 m, and your angle is 157.38 degrees.
We can use the Pythagorean theorem to find the distance (d) of your location from the center of town:
d = sqrt((12.00 m)^2 + (-5.00 m)^2)
d = sqrt(144.00 m^2 + 25.00 m^2)
d = sqrt(169.00 m^2)
d = 13.00 m
Therefore, you are 13.00 meters away from the center of town.
To find the angle (theta) between the line connecting your location to the center of town and the positive x-axis, we can use the inverse tangent function (tan^-1) as follows:
theta = tan^-1(opp/adj)
theta = tan^-1((-5.00 m)/(12.00 m))
theta = -22.62 degrees
However, since your location is in the second quadrant (negative x and positive y), the angle must be measured from the positive y-axis, not the positive x-axis. Therefore, the actual angle between the line connecting your location to the center of town and the positive y-axis is:
theta = 180 degrees - 22.62 degrees
theta = 157.38 degrees
Therefore, you are 13.00 meters away from the center of town, at an angle of 157.38 degrees from the positive y-axis.
know more about Pythagorean theorem here: brainly.com/question/14930619
#SPJ11
order from the least to the greatest 5 -13 _19 -8 _14 -12
Answers
If you mean for the numbers with _ to be positive, the order is -13, -12, -8, 5, 14, 19
I need to know the area in square centimeters of sector ABC?

Answers
Answer:
75π / 2
Step-by-step explanation:
a = (∅ / 2π) * πr²
a = (3π/4 / 2π) * π(100)
a = (3/8) * 100π
a = 300/8 * π
a = 75/2 * π
a = 75π / 2
Which of the following is a perfect square?
A.) 115
B.) 169
C.) 154
D.) 279
Answers
Answer:
B (169)
Step-by-step explanation:
Helppp meeee please

Answers
Answer:
The Equation for lyft is:
4.50x
The Equation for uber is
2.50x+5
Step-by-step explanation:
If you want to determine which one is better then you can just plug in the distance in place of x. For example if it asked you which one was better for 5 miles, uber or lyft you could plug 5 into the equation.
It would look like this
Lyft: 4.50 (5)= 22.5
Uber
2.50 (5) +5= 17.5
If the distance were 5 miles uber would be the better option.
Use the following scenario to solve the following problems: A bag contains 4 white cards, 3 black cards, and 6 green cards. Find the probability of each event for one draw. Hint: Use the formula for theoretical probability. a number greater than 6 A) 0 B) 1/6 Fundamental Counting Principle Find the number of possible passwords (with no letters or digits excluded) for the conditions in the following problems. Hint: There are 10 choices for the digits and 26 choices for the letters 2 digits followed by 3 letters followed by 1 digit A) 17,576,000 B) 6,760,000 2 letters followed by 4 digits A) 17,576,000 B) 6,760,000
The local pizza shop offers 4 sizes of pizza, three types of crust, and 10 toppings. How many different pizzas can be ordered with one topping? A) 60 B) 120
Answers
a) Probability of drawing a number greater than 6. b) (i) 10 × 10 × 26 × 26 × 26 × 10 = 17,576,000 (option A). (ii) 26 × 26 × 10 × 10 × 10 × 10 = 17,576,000 (option A). c) there are 120 different pizzas that can be ordered with one topping.
a) Probability of drawing a number greater than 6: Since there are no numbers greater than 6 in the bag, the probability of drawing a number greater than 6 is 0 (option A).
b) Number of possible passwords for the conditions:
(i) 2 digits followed by 3 letters followed by 1 digit: For this password, we have 10 choices for the first digit, 10 choices for the second digit, 26 choices for each of the three letters, and 10 choices for the last digit. By applying the fundamental counting principle, we multiply these choices together: 10 × 10 × 26 × 26 × 26 × 10 = 17,576,000 (option A).
(ii) 2 letters followed by 4 digits: For this password, we have 26 choices for each of the two letters and 10 choices for each of the four digits. Using the fundamental counting principle, we multiply these choices together: 26 × 26 × 10 × 10 × 10 × 10 = 17,576,000 (option A).
c) Number of different pizzas that can be ordered with one topping: We have 4 sizes of pizza, 3 types of crust, and 10 toppings. To find the number of different pizzas, we multiply the number of choices for each category together: 4 (sizes) × 3 (crusts) × 10 (toppings) = 120 (option B). Therefore, there are 120 different pizzas that can be ordered with one topping.
learn more about fundamental counting principle here: brainly.com/question/30869387
#SPJ11
Write an
equivalent expression by distributing the "-" sign outside the parentheses:
-(0.39 + 75 – 5)
Answers
Answer:
(-0.39 - 75 + 5)
Step-by-step explanation:
If you multiply a positive by a negative, it becomes a negative. If you multiply a negative by a negative, it becomes positive. Remember that, it will help you later on.
Answer:-70.39
Step-by-step explanation: im in college
w/4 + 12.5 - 7z what term has a coefficient
Pls help me.

Answers
This expression contains 3 terms.
7z is the term with a coefficient.
12.5 is the constant term in the expression.
What is an equation?An equation is written in the form of variables and constants separated by the operation of multiplication and division,
An equation states that terms in different forms on both sides of the equality sign are equal.
Multiplication and division do not separate the terms of an equation.
Given, w/4 + 12.5 - 7z.
There are 3 terms in this expression.
The term that has a coefficient is 7z.
The constant term in the expression is 12.5.
learn more about equations here :
https://brainly.com/question/14686792
#SPJ2
Use goal seek to answer this question. All else equals, to have a net income of 20,000, the cogs margin percentage must be ______, and the gross profit must be ______.
Answers
The COGS margin percentage must be 40%, and the gross profit must be $17,250.
What is COGS margin?
Net sales less cost of goods sold equals gross margin (COGS). In other words, it is the amount of money a company keeps after deducting the direct costs of producing the goods and services it sells.
The income statement is missing, so the given information was:
Revenue = 100,000
COGS = 40,000
Gross Profit = 60,000
Salaries
Marketing
Rent
Earnings Before Tax 23,000
Income Tax 25%
We have to find the net income.
Since COGS are $40,000 and total sales are $100,000, the COGS margin percentage = 40,000 / 100,000 = 40%
Then earnings before taxes are $23,000 and taxes are 25%, then net income = $23,000 x (1 - 25%) = $23,000 x 75% = $17,250
Hence, the COGS margin percentage must be 40%, and the gross profit must be $17,250.
To learn more about COGS margin, visit;
https://brainly.com/question/4177260
#SPJ4
How many questions are in ATI Capstone Comprehensive Assessment B?
Answers
The ATI Capstone Comprehensive Assessment evaluation normally comprises of multiple-choice questions, with 90 to 130 possible answers. Certain versions may also include extra alternative questions kinds, such as fill-in-the-blank or select-all-that-apply
Depending on whatever version of the test you are taking, the ATI Capstone Comprehensive Assessment B may have fewer or more questions.The purpose of the ATI Capstone Comprehensive Assessment B is to assess the knowledge and critical-thinking abilities of nursing students in a range of areas, such as pharmacology, leadership, and patient care.The duration of the test and the precise number of questions it contains may also vary according on the institution or nursing programme that is giving it.For more questions on ATI Capstone Comprehensive Assessment
https://brainly.com/question/27755378
#SPJ4
Write an equation to represent the following statement.
24 is 4 times as great as k.
Answers
Answer:
24 x 4 = k x 4
Step-by-step explanation: