Simplify (3xy)^5/x^3
Answers
Answer:
\(243 {x}^{2} {y}^{5} \)
Step-by-step explanation:
\( \frac{(3xy {)}^{5} }{ {x}^{3} } \)
\( \frac{243 {x}^{5} {y}^{5} }{ {x}^{3} } \)
simplify:
\(243 {x}^{2} {y}^{5} \) ✅
Related Questions
need help with this ASAP
!!!!!!!!!!!!!!!!!!!!!!!!

Answers
The fence in dead center is about 399 feet from the third base.
What is the Pythagorean Theorem?The Pythagorean Theorem states that in the case of a right triangle, the square of the length of the hypotenuse, which is the longest side, is equals to the sum of the squares of the lengths of the other two sides.
Hence the equation for the theorem is given as follows:
c² = a² + b².
In which:
c > a and c > b is the length of the hypotenuse.a and b are the lengths of the other two sides (the legs) of the right-angled triangle.For the triangle in this problem, we have that:
The sides are d ft and 90 ft.The hypotenuse is of 409 ft.Hence the distance is obtained as follows:
d² + 90² = 409²
\(d = \sqrt{409^2 - 90^2}\)
d = 399 ft.
More can be learned about the Pythagorean Theorem at brainly.com/question/30203256
#SPJ1
Please help me with this problem so that my son can understand better. Let f(x)=−0.5(x−8)^2−2.What is the average rate of change for the quadratic function from x=−4 to x = 8? Enter your answer in the box.

Answers
The function is given to be:
\(f(x)=-0.5(x-8)^2-2\)To calculate the average rate of change between two points, we can use the formula:
\(\Rightarrow\frac{f(a)-f(b)}{a-b}\)where a and b are the points.
For the function provided, we are to find the rate of change between -4 and 8. Hence, our formula will be:
\(\frac{f(-4)-f(8)}{-4-8}=\frac{f(-4)-f(8)}{-12}\)We can evaluate f(-4) and f(8) to be:
\(\begin{gathered} f(-4)=-0.5(-4-8)^2-2=-0.5(-12)^2-2=-0.5(144)-2=-72-2=-74 \\ f(8)=-0.5(8-8)^2-2=-2 \end{gathered}\)Therefore, the average rate of change is calculated to be:
\(\Rightarrow\frac{-74-(-2)}{-12}=\frac{-74+2}{-12}=\frac{-72}{-12}=6\)The average rate of change is 6.
please help ALGRBRA II 10 points

Answers
A square pyramid has a base edge of 1 meter. The height of each triangular face is 1 meter. What is the pyramid's surface area?
Answers
Answer:
A square pyramid has 5 faces: 1 square base and 4 triangular faces.
The area of the base is:
A = s^2
where s is the length of the base edge.
In this case, s = 1 m, so:
A = 1^2 = 1 m^2
The area of each triangular face is:
A = 1/2 * b * h
where b is the base of the triangle (which is equal to the length of one side of the square base) and h is the height of the triangle (which is given as 1 m).
In this case, b = 1 m and h = 1 m, so:
A = 1/2 * 1 * 1 = 0.5 m^2
The total surface area of the pyramid is the sum of the area of the base and the area of the four triangular faces:
SA = A_base + 4 * A_triangles
SA = 1 + 4(0.5)
SA = 1 + 2
SA = 3 m^2
Therefore, the surface area of the pyramid is 3 square meters.
7-3: MathXL for School: Additional Practice
7.3.AP-2
4 cm
Find the area of the shape.
7 cm
7 cm
13 cm
19 cm
The area is
(Type a whole number or a decimal.)
Answers
Answer:
1- 7cm × 7 cm - 49 cm squ.
13cm × 13 cm -169. cm square
19×19-361 cm square
The area of the shape 1 is 49 square cm, area of the shape 2 is 169 square cm and area of the shape 3 is 361 square cm.
We have to find the area of the shapes
(1) who's all dimensions are 7 cm.
(2) who's all dimensions are 13 cm.
(3) whose dimensions are 19 cm.
All the shapes will form a square because their both sides are equal.
We know the area of the square is "a x a" where 'a' is the side of the square.
Thus, the area for 1st figure is
a x a = 7 x 7
area = 49 square cm.
Area for the 2nd figure is
a x a = 13 x 13
area = 169 square cm.
Similarly, the area of 3rd figure is:
a x a = 19 x 19
area = 361 square cm.
Hence, the area of all the given shapes are 49square cm, 169 square cm and 361 square cm.
Learn more about area of the shapes here:
https://brainly.com/question/28337394
#SPJ6
Write an equation to describe the linear function that fits the given characteristics.
Passes through the points (1,2) and (3,-4)
Parallel to y=1/4x+5 and passes through the point (4,8).
Answers
1/4x+7 passes through (4,8) but to make it pass through (1,2) and (3,-4) would be better for perpendicular. perpendicular is -4x+6 for (1,2) and (3,-4) which is kind of the closes i had time to play with it.
Harry and Tim both made New Year’s Resolution. Harry made 5 more resolutions than Tim. Together they made 13 resolutions. How many resolutions did Harry make?
Answers
Answer:
Harry made 9 resolutions.
Step-by-step explanation:
Tim made x resolutions.
Harry made five more, x + 5.
Harry and Tim's together was 13.
x + x + 5 = 13
combine like terms.
2x + 5 = 13
subtract 5
2x = 8
divide by 2
x = 4
Tim made 4 resolutions.
Harry made 4 + 5, that is, 9 resolutions.
Check:
Harry and Tim together is 13.
4 + 9 = 13
Harry made 9 resolutions.
p^q is logically equivalent to
Answers
The logically equivalent statement to p → q is:
~q → ~p
How to find the logically equivalent statement?The conditional statement:
p → q
Assuming p and q are propositions, the conditional statement may be expressed as:
"If p holds true, then q follows suit. "
'
Whenever p is true, q is true as well. This implies that if q is false, then p must also be false.
We can rephrase this statement cleverly using the negative propositions, which include the opposite or contradictory statements.
~p and ~q
These mean:
Not p and Not q respectively.
Then the statement:
"If q is not true, then p is not true"
Is written as:
~q → ~p
So this is the logically equivalent statement.
Read more about conditional statements here:
https://brainly.com/question/27839142
#SPJ1
The Complete Question
Given a conditional statement p → q, which statement is logically equivalent?
~p → ~q
~q → ~p
q → p
p → ~q
Answer for brainliest

Answers
x has to be less than 6
According to a random sample taken at 12 A.M., body temperatures of healthy adults have a bell-shaped distribution with a mean of 98.07°F and a standard deviation of 0.58°F. Using Chebyshev's theorem, what do we know about the percentage of healthy adults with body temperatures that are within 3 standard deviations of the mean? What are the minimum and maximum possible body temperatures that are within 3 standard deviations of the mean?

Answers
SOLUTION;
Using Chebyshev's theorem the percentage of healthy adults with body temperatures that are within 3 standard deviations of the mean make up at least 89% of the population.
Thus;
The maximum and minimum possible body temperatures that are within 3 standard deviations of the mean are;
\(\begin{gathered} 98.07+3(0.58)\text{ }and\text{ }98.07-3(0.58) \\ Maximum=99.81^oF \\ Minimum=96.33^oF \end{gathered}\)between what two consecutive integers must log2(17) lie
Answers
Answer:
4 and 5
Step-by-step explanation:
For answering questions like this, it can be useful to remember a few of the powers of small integers:
2^4 = 16
2^5 = 32
Exponents and logarithmsA logarithm can be considered to be an exponent of the base.
\(\log_b(x) = a \ \Longleftrightarrow\ b^a=x\)
The ordering of powers of 2 relative to the number of interest (17) is ...
16 < 17 < 32
2⁴ < 17 < 2⁵ . . . . . . . . . . . . . . . . . . . expressed as powers of 2
log₂(2⁴) < log₂(17) < log₂(2⁵) . . . . . log₂ of the above inequality
4 < log₂(17) < 5 . . . . . . . . . . . . . . . . showing the values of the logs
Log₂(17) lies between 4 and 5.
__
Additional comment
Using the "change of base" formula, you can use a calculator to find the value of log₂(17). It shows you the value is between 4 and 5.
log₂(17) = log(17)/log(2) . . . . . . using logs to the same base

b) A car priced at $3600 is reduced by 25%.
What is the reduced price?
Answers
3600:25 =144
reduced price is 144$
15)
136⁰
2
S
?
R
Find the measure of the arc or angle indicated
Answers
Answer:
224
Step-by-step explanation:
360.-136.
which of the following is an example of conditional probability?

Answers
Answer:
the answer is (a) the probability that a person will order pie
A bowl contains blueberries and strawberries. There are a total of 10 berries in the bowl. The ratio of blueberries to strawberries is 2 for every
3. How many of each berry are in the bowl?
Answers
Answer:
8 strawberries and 2 blueberries
Step-by-step explanation:
a simplifie 6 × ( - 24 ) ÷ ( -4 )
Answers
\(\huge\textbf{Hey there!}\)
\(\huge\boxed{\mathsf{6\times(-24)\div (-4)}}\\\huge\boxed{= \mathsf{\dfrac{6(-24)}{-4}}}\\\huge\boxed{\mathsf{= \dfrac{\bold{-144}}{-4}}}\\\huge\boxed{\mathsf{\dfrac{-144\div-4}{-4\div-4}}}\\\huge\boxed{= \mathsf{\dfrac{\bold{36}}{1}}}\\\huge\boxed{\mathsf{= 36\div1}}\\\huge\boxed{\mathsf{= 36}}\\\\\\\huge\text{\bf Therefore, your answer is: \boxed{\mathsf{36}}}\huge\checkmark\)
\(\huge\text{\bf Good luck on your assignment and enjoy}\\\huge\text{\bf your day!}\)
~\(\frak{Amphitrite1040:)}\)
Two submarines began dives in the same vertical position to meet at a designated point.
If one submarine was on a course approximated by the equation x + 4y = -14 and
the other was on a course approximated by the equation x + 3y = -8, at what location would they meet?
Answers
Answer:
(10, -6)
Step-by-step explanation:
The solution of the system of the equations given would be the location that they would both meet.
Let's solve:
x + 4y = -14 ----› Eqn. 1
x + 3y = -8 -----› Eqn. 2
Subtract Eqn. 2 from Eqn. 1
y = -6
Substitute y = -6 into Eqn. 1.
x + 4y = -14 ----› Eqn. 1
x + 4(-6) = -14
x - 24 = -14
x = -14 + 24 (addition property of equality)
x = 10.
The answer is: (10, -6)
HELP i need the answer 20 POINTS
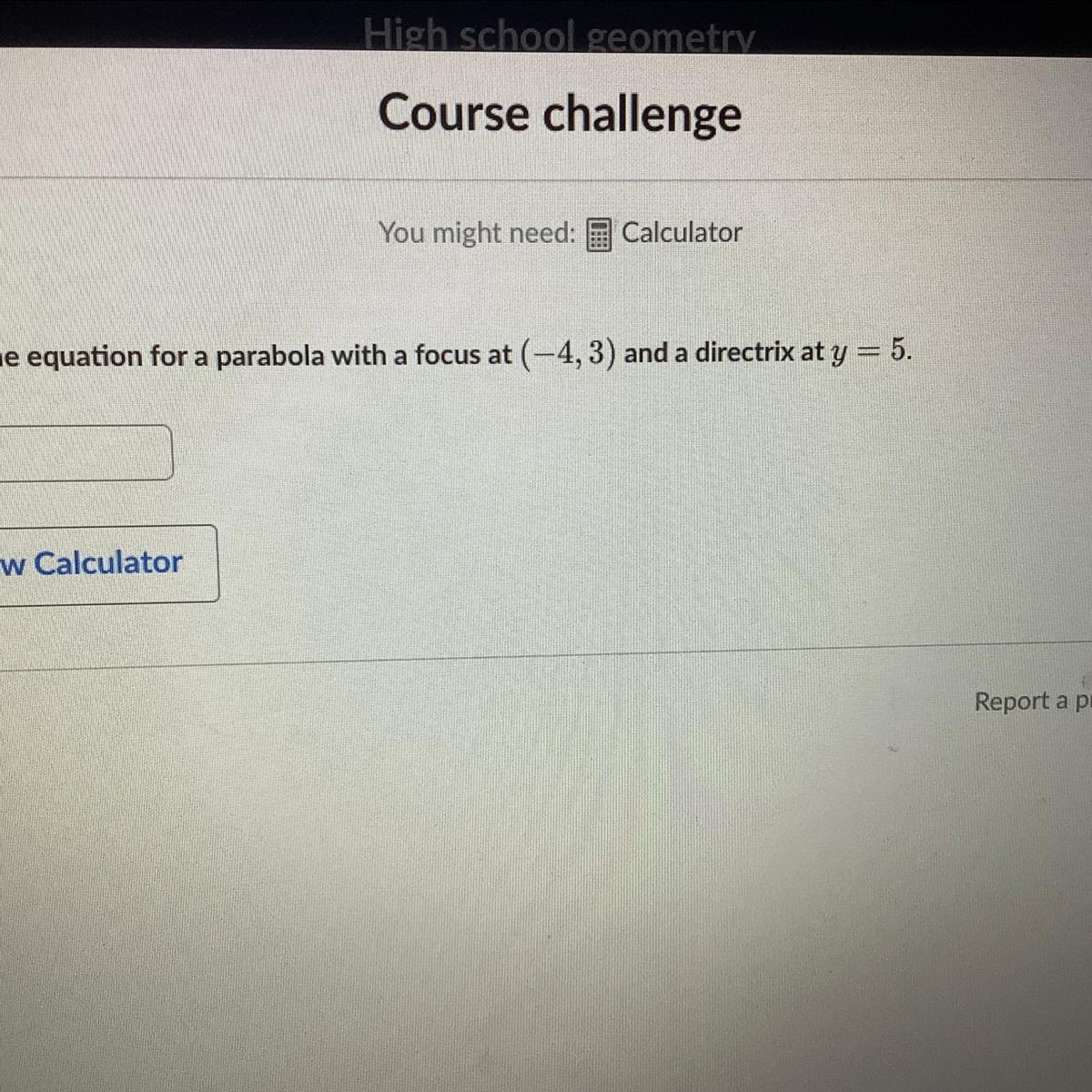
Answers
Answer:
y = -1/4(x +4)² +4
Step-by-step explanation:
The equation of a parabola with focus (a, b) and directrix y=d can be written using the form ...
y = 1/(2(b-d))(x -a)² +(b+d)/2
ApplicationWe are given (a, b) = (-4, 3) and d=5. Using these values in the above form gives the equation ...
y = 1/(2(3-5))(x -(-4))² +(3+5)/2
y = -1/4(x +4)² +4
Please I’ll give u brainliest

Answers
The correct statements are;
Option B, E
How to determine the statements
The formula for perimeter of a quarter circle is P = (1/4 x 2 x pi x r) + (2 x r)
From the diagram, we have that;
r = 5 meters
Substitute the value, we have;
Perimeter = 1/4× 2 ×3.14 × 5 + 2(5)
expand the bracket, we have;
Perimeter = 1.57 + 10
Perimeter = 11.57 meters
Area = 1/4 πr²
Substitute the values, we have;
Area = 1/4 × 3.14 × (5)²
expand the bracket, we have;
Area =19.63 square meters
Learn more about circles at: https://brainly.com/question/25292087
#SPJ1
Please help me!!!! Find the 56th term of an arithmetic sequence if the common difference is

Answers
Answer:
7 + 55\(\sqrt{11}\)
Step-by-step explanation:
term one is 7
so term 2 is 7 + \(\sqrt{11}\)
and term 3 is 7 + 2\(\sqrt{11}\)
common pattern here: 7 + (n-1)\(\sqrt{11}\)
when n = 56
7 + 55\(\sqrt{11}\)
Determine the minimum sample size needed if we want to estimate the population percent of students who LOVE statistics. We want the estimate to be within 5% of the true proportion, with 99% confidence. Assume a previous study estimated that percent to be twenty percent. 425 43 101 664
Answers
425 is the smallest sample size required when the percentage of pupils in the population who LOVE statistics.
Given that,
We have to find the smallest sample size required to determine the percentage of pupils in the population who LOVE statistics. With 99% confidence, the estimate should be within 5% of the true proportion. Assume that past research pegged that percentage at 20%.
p = 0.20
1 - p = 0.80
Margin of error = E = 0.05
At 99% confidence level
α= 1 - 99%
α = 1 - 0.99 =0.01
α/2 = 0.005
Zα/2 = Z0.005 = 2.576
Sample size = n = (Zα / 2 / E )2 × p × (1 - p)
= (2.576 / 0.05)2 × 0.20 × 0.80
= 424.68
Sample size = n = 425
Therefore, 425 is the smallest sample size required when the percentage of pupils in the population who LOVE statistics.
To learn more about size visit: https://brainly.com/question/18587054
#SPJ4
is -3 an even number?
Answers
Answer:
I don't think so....but then again you never know... but I'm 99.99% sure. I'm so sorry if I'm wrong.
Step-by-step explanation:
Good Luck! Have an amazing day/ night!
God bless all <33
Consider a 3 x 3 matrix 0.000 0.000 0.000
A= 3.000 3.000 -3.000
0.000 0.000 0.000 Find three linearly independent eigenvectors 0.000 0.000 0.000 v1, v2, v3 and their eigenvalues λ1, λ2, λ3. In order to be accepted as correct, all entries of the vector Av; λivi must have absolute value smaller than 0.05. Your eigenvalues will only be correct if the corresponding vectors are eigenvectors with these eigenvalues.
v1 = -1 is an eigenvector of A to the eigenvalue λi = 0 1
0
v2 = -1 is an eigenvector of A to the eigenvalue λ2= 0
0
1
v2 = 0 is an eigenvector of A to the eigenvalue λ3= 0
1
0
Answers
As per the matrix, the three linearly independent eigenvectors are 0, 0.05 and 1.
Now let's consider the given matrix A. We are asked to find three linearly independent eigenvectors and their corresponding eigenvalues. Linearly independent eigenvectors are important because they allow us to represent any vector in the space as a linear combination of these eigenvectors.
The first eigenvector v1 is -1, and it corresponds to the eigenvalue λ1 = 0. To check if this is indeed an eigenvector, we multiply it by A and check if the resulting vector is a scalar multiple of v1. In this case, Av1 = 0v1, which means that v1 is indeed an eigenvector with eigenvalue λ1 = 0.
The second eigenvector v2 is also -1, and it corresponds to the eigenvalue λ2 = 0. Again, we multiply it by A and check if the resulting vector is a scalar multiple of v2. Av2 = 0v2, which means that v2 is an eigenvector with eigenvalue λ2 = 0.
The third eigenvector v3 is 0, and it corresponds to the eigenvalue λ3 = 1. We repeat the same process and check if Av3 is a scalar multiple of v3. In this case, Av3 = 0.05v3, which satisfies the given condition of having all entries with absolute value smaller than 0.05. Therefore, v3 is an eigenvector with eigenvalue λ3 = 1.
To know more about matrix here
https://brainly.com/question/28180105
#SPJ4
When two lines intsecteach other in one pointand they make 90 degree angle And if the slope of the 1st line is M1 and the slope of the 2nd line ts we can say: MIXM2=1
True or False
Answers
A couple plans to purchase a house. The bank requires a 20% down payment on the $240,000 house. The couple will finance the rest of the cost with a fixed- rate mortgage at 8.5% annual interest with monthly payments over 30 years.
Complete the parts below. Do not round any intermediate computations. Round your final answers to the nearest cent if necessary. If necessary, refer to the list of financial formulas.
(a) Find the required down payment.
(b) Find the amount of the mortgage.
(c) Find the monthly payment.

Answers
(A) The required down payment is $48,000.
(B) The amount of the mortgage is $192,000.
(C) Monthly payment = $192,000 * (0.085/12) * (1 + (0.085/12))^(3012) / (((1 + (0.085/12))^(3012)) - 1)
(a) To find the required down payment, we need to calculate 20% of the house price.
Down payment = 20% of $240,000
Down payment = 0.2 * $240,000
Down payment = $48,000
The required down payment is $48,000.
(b) The amount of the mortgage is equal to the total cost of the house minus the down payment.
Mortgage amount = Total cost of the house - Down payment
Mortgage amount = $240,000 - $48,000
Mortgage amount = $192,000
The amount of the mortgage is $192,000.
(c) To find the monthly payment for the mortgage, we can use the formula for the monthly payment on a fixed-rate mortgage:
Monthly payment = P * r * (1 + r)^n / ((1 + r)^n - 1)
Where:
P = Principal amount (mortgage amount)
r = Monthly interest rate (8.5% annual interest divided by 12 months and converted to a decimal)
n = Total number of monthly payments (30 years multiplied by 12 months)
Monthly payment = $192,000 * (0.085/12) * (1 + (0.085/12))^(3012) / (((1 + (0.085/12))^(3012)) - 1)
Using this formula and performing the calculation will give you the monthly payment amount.
for more questions on down payment
https://brainly.com/question/1698287
#SPJ8
? Question
Drag the tiles to the correct boxes to complete the pairs.
Solve each equation using the square root property. Then, match the solutions to the equatio
Tiles
Help please

Answers
The solutions for the given equations are x = 9i , -9i , x = 2i,-2i ,
x = \(\sqrt{13}\) , - \(\sqrt{13}\) , x = \(\sqrt{14}\)i , - \(\sqrt{14}\)i .
Given equations ,
\(x^{2}\) + 81 = 0 ,\(x^{2}\)-22 = -26 , 3\(x^{2}\)-18=21 ,2\(x^{2}\) +15 = -13
What is Quadratic equation ?
quadratic equation can be defined as the equation which is in the form of a\(x^{2}\)+bx + c = 0 .
where a,b,c are constants .
By solving we get ,
\(x^{2}\) +81 = 0
\(x^{2}\) = -81
x = 9i , -9i
\(x^{2}\) - 22 = -26
\(x^{2}\) = -26 + 22
\(x^{2}\) = -4
x = 2i,-2i
3\(x^{2}\) - 18 = 21
3\(x^{2}\) = 18+21
3\(x^{2}\) = 39
\(x^{2}\) = 13
x = \(\sqrt{13}\) , - \(\sqrt{13}\)
2\(x^{2}\) +15 = -13
2\(x^{2}\) = -13-15
2\(x^{2}\) = -28
\(x^{2}\) = -14
x = \(\sqrt{14}\)i , - \(\sqrt{14}\)i
Hence the solutions for the given equations are x = 9i , -9i , x = 2i,-2i ,
x = \(\sqrt{13}\) , - \(\sqrt{13}\) , x = \(\sqrt{14}\)i , - \(\sqrt{14}\)i .
To learn more about Quadratic equations from the given link.
https://brainly.com/question/30098550
#SPJ1
PLEASE HELP PLEASE PLEASE HELP


Answers
Answer:
D. is correct.
Look at 2 × \(\frac{1}{3}\) as 2 × 1 for now.\(2 * 1 = 2\)
Multiply 4 by 1(third)\(4 * 1=4\)
Now, add.\(4 + 2 = 6\)
________________________________________________________
The product of answer choice D.(6) is not equal to the product of \(\frac{2}{3}\) × 4, because \(\frac{2}{3}\) × 4 is actually \(2\frac{2}{3}\)
________________________________________________________
What have we learned?We learned how to find whole numbers through an expression with fractions.
Questions related to the topic? Ask me in the comments box.
Need help ASAP!!!!!!!!!!!!!!!!!

Answers
Slope = (y2-y1)/(x2-x1) = (3-0)/(3-2) = 3/1 =3
The general equation of a line is represented as y = mx + b, where m is the slope and b is the y-intercept.
We know the slope is 3, so we have
y = 3x + b
To find b, we choose one coordinate and we substitute in the equation and solve for b.
Let’s substitute (2,0) in the equation; we have:
0 = 3(2) + b
0 = 6 + b
b = -6
So the equation of the line is:
y = 3x - 6
Write an equation in point-slope from for a line that passes through the given set of points. (3,5) and (-6,-4)
Answers
does this help or do you need help plugging in and finding slope?
how do i solve this Please give me a advise

Answers
The probability of spinning an even number, flipping tails, then spinning an odd number is:
1/9 (as fraction) or 11.11% (as percent)
How to find the probability of spinning an even number, flipping tails, then spinning an odd number?
To find the probability of spinning an even number, flipping tails, then spinning an odd number. We need to consider the individual probabilities. That is:
We have only one even number in the spinner, and that is 2. Thus,
P(even number) = 1/3
For a coin, the probability of head or tail is 1/2. Thus,
P(tail) = 1/2
We have two odd number in the spinner, and that is 1 and 3. Thus,
P(odd number) = 2/3
Therefore, the probability of spinning an even number, flipping tails, then spinning an odd number will be:
P(even, tail, odd) = 1/3 * 1/2 * 2/3
= 2/18
= 1/9 (as fraction)
In percent:
P(even, tail, odd) = 1/9 * 100
= 11.11%
Learn more about probability on:
https://brainly.com/question/24756209
#SPJ1