Simplify the following expression: 3(2x+7) *
O 6x + 7
O 6x + 10
O 6x + 21
O 5x + 10
Answers
3 x 2x = 6x
3 x 7 = 21
6x+21
Related Questions
Solve for length of segment c.
11 cm
10 cm
8.8 cm
c = [?] cm
If two segments intersect inside
or outside a circle: ab = cd
![Solve for length of segment c.11 cm10 cm8.8 cmc = [?] cmIf two segments intersect insideor outside a](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/6JIAngrmaJS5xiD3lMj7AnGQ5XEMOjDN.png)
Answers
Answer:
c = 8
Step-by-step explanation:
Using the Intersecting Chords Theorem, we can form the following equation and solve for c:
\(ab=cd\\(10)(8.8)=11c\\88=11c\\c=8\)
Is the Mean Value Theorem applicable to the function f(x) = |x - 1| on the interval [0, 2]?
Why or why not?
Answers
Carly has $50.84 in a bank account. She writes a check for $66.12 from the account. What is the balance of Carly’s account after writing the check?
Answers
Answer:
66.12 - 50.84 = -15.28
carly has -15.28$ after writing that check.
Which pair of terms can be used to represent any two consecutive odd numbers?
x and x + 1
x and x + 2
x and 2 x + 1
2 x and 2 x + 1
Answers
Answer:
x and 2x+1
Step-by-step explanation:
7Ь — 9x + За – 12
Help please
Answers
Answer:
−9x+3a+7b−12
Step-by-step explanation:
Hope this helps
For the question of total area of the cuboid is 200cm^.
I understand where we divide 150 by 4.
But why do I need to multiply by 5, when there are 6 faces.
Answers
You need to multiply by 5 instead of 6 because each pair of opposite faces on a cuboid has the same area, so by considering one face from each pair, you ensure that you don't count any face twice.
When calculating the total surface area of a cuboid, you need to understand the concept of face pairs.
A cuboid has six faces, but each face has a pair that is identical in size and shape.
Let's break down the reasoning behind multiplying by 5 instead of 6 in the given scenario.
To find the surface area of a cuboid, you can add up the areas of all its faces.
However, each pair of opposite faces has the same area, so you avoid double-counting by only considering one face from each pair. In this case, you have five pairs of faces:
(1) top and bottom, (2) front and back, (3) left and right, (4) left and back, and (5) right and front.
By multiplying the average area of a pair of faces by 5, you account for all the distinct face pairs.
Essentially, you are considering one face from each pair and then summing their areas.
Since all the pairs have the same area, multiplying the average area by 5 gives you the total surface area.
When dividing 150 by 4 (to find the average area of a pair of faces), you are essentially finding the area of a single face.
Then, by multiplying this average area by 5, you ensure that you account for all five pairs of faces, providing the total surface area of the cuboid.
Thus, multiplying by 5 is necessary to correctly calculate the total surface area of the cuboid by accounting for the face pairs while avoiding double-counting.
For similar question on cuboid.
https://brainly.com/question/29568631
#SPJ8
please help! I'm almost out of time on my assignment

Answers
If 4+h=7 what is the value of 5h?
Answers
Answer
5h=15
Step-by-step explanation:
h = 7 - 4 = 3 therefore 5(3)=15
Answer:
Here's my work for it
:)

Domain or range function or not And explain

Answers
if sin 15° =x and sin 75° =y then which one of the following correct
Tan 15°=x/y
Tan 75° =x/y
Cot 15°=x/y
75°=y/x
Answers
Answer:
tan 75 =x/you is the my answer
Which equation is equivalent to 2 Superscript 4 x Baseline = 8 Superscript x minus 3?
2 Superscript 4 x Baseline = 2 Superscript 2 x minus 3
2 Superscript 4 x Baseline = 2 Superscript 2 x minus 6
2 Superscript 4 x Baseline = 2 Superscript 3 x minus 3
Answers
The equivalent exponential equations are given as follows:
\(2^{4x} = 2^{3(x - 3)}\)
How to obtain the equivalent exponential expression?The exponential expression for this problem is defined as follows:
\(2^{4x} = 8^{x - 3}\)
The number eight is the third power of 3, that is:
8 = 2³.
The power of a power rule is used when a single base is elevated to multiple exponents.
Then the simplified expression is obtained keeping the base, while the exponents are multiplied.
Meaning that the equivalent expression on the right side of the equality in this problem is given as follows:
\(8^{x - 3} = (2^3)^{x - 3} = 2^{3(x - 3)}\)
Meaning that the correct option is given by the fourth option.
More can be learned about the power of a power property at brainly.com/question/11975096
#SPJ1
Find C Find A and find ab=21 c=26 B=34 degrees
Answers
The Law of Sines states that:
\(\frac{\sin A}{a}=\frac{\sin B}{b}=\frac{\sin C}{c}\)Procedure
0. Using the Law of Sines to ,find C
\(\frac{\sin B}{b}=\frac{\sin C}{c}\)As we have the values of all variables but C, we isolate for it:
\(\sin C=\frac{\sin B}{b}\)\(C=\sin ^{-1}(\frac{\sin B}{b}\cdot c)\)Replacing the values:
\(C=\sin ^{-1}(\frac{\sin34}{21}\cdot26)\)\(C=\sin ^{-1}(0.6923)\)\(C=43.82\)2. Calculating A
Then, knowing that all the interior angles of a triangle add up to 180°...
\(A+B+C=180\)...we can isolate for A:
\(A=180-B-C\)Replacing the values given for B and the value of C:
\(A=180-34-43.82\)\(A=102.18\)3. Calculating a
Now that we know A, we can find a using the Law of Sines:
\(\frac{\sin A}{a}=\frac{\sin B}{b}\)Isolating for a we get:
\(a=\frac{\sin A}{\frac{\sin B}{b}}\)Replacing the values:
\(a=\frac{\sin 102.18}{\frac{\sin 34}{21}}\)\(a=36.71\)Answer:
• C = 43.82°
,• A = 102.18°
,• a = 36.71
You are riding the bus to school and you realize it is taking longer because of all the stops you are making. The time it takes to get to school, measured in minutes, is modeled
using the function (0) - ** - 3x' . dx - 6, where x is the number of stops the bus makos. If the bus makes 2 stops after you board, how long does it take you to get to
school?
Answers
Answer:
7 minutes
Step-by-step explanation:
what is 750× 2
what is the square root of 90
Answers
Square root of 90 can be written as 3 multiplied by Square root of 10
¡Ayuda, por favor!
La fórmula de Lorentz para la contracción en la teoría de la relatividad relaciona la longitud L de un objeto, que se mueve a una velocidad de V con respecto a un observador, con su longitud L0 en reposo. Si c es la velocidad de la luz. entonces:
L²=L0 ² (1- V²/C²)
¿Para qué velocidades L será menor que 1/2 L0? Exprese su respuesta en términos de c.
Answers
Answer:
I don't understand.............
PLSS HElP WITH THIS !!!! MARKING BRAINLIST!!!!
NO ONE WILL HELP:(
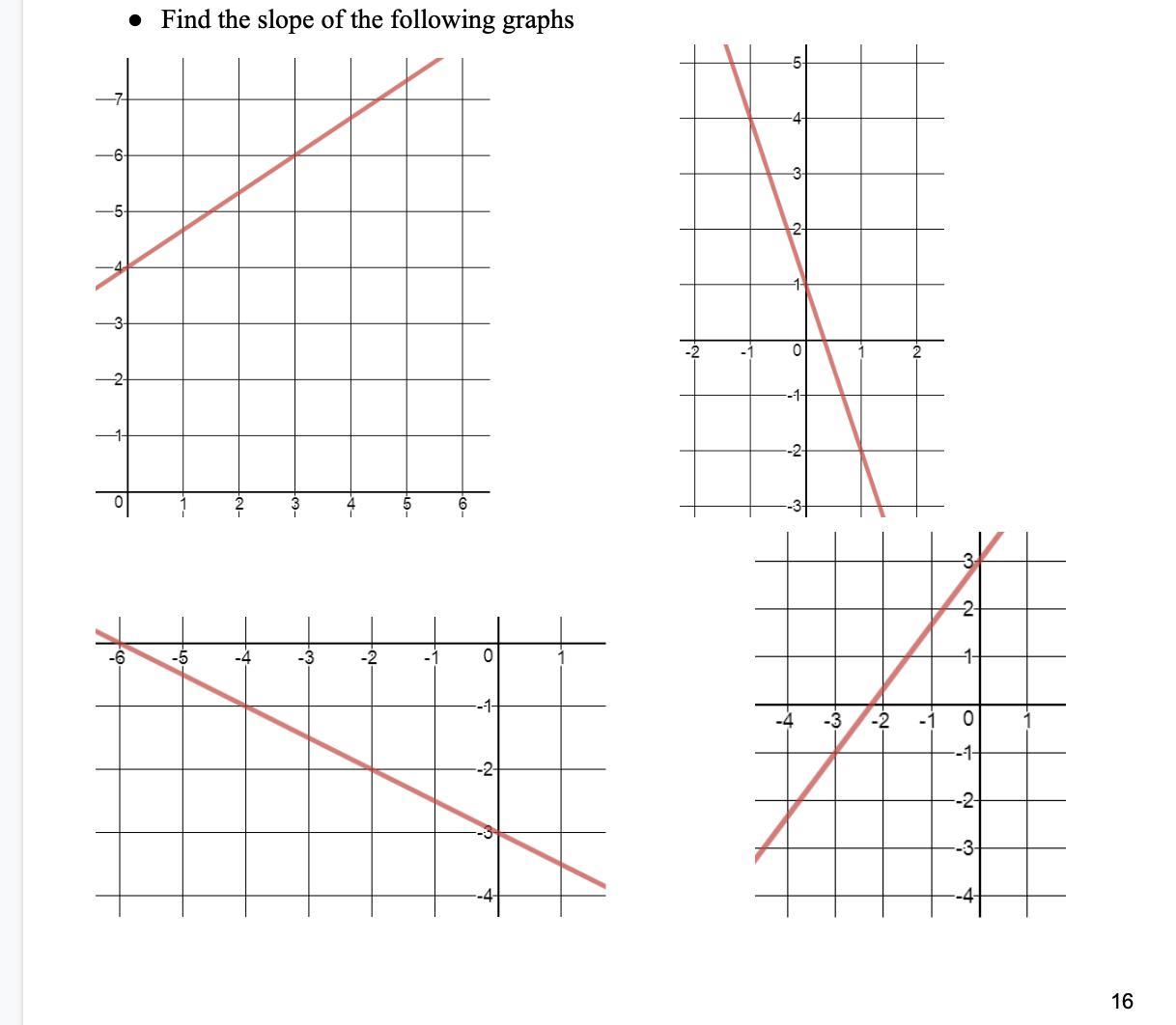
Answers
Answer:
1. 2/3
2. -3
3. -1/2
4. 4/3
Millions of Americans rely on caffeine to get them up in the morning. Here's nutritional data on some popular
drinks at Ben's Beans coffee shop:
Drink
Type
Calories
Sugars (g)
Caffeine (mg)
Brewed coffee
Hot
4
0
260
Caffé latte
Hot
100
14
75
Caffè mocha
Hot
170
27
95
Cappuccino
Hot
60
8
75
Iced brewed coffee
Cold
60
15
120
Chai latte
Hot
120
25
60
The individuals in this data set are:
Choose 1 answer:
Answers
Answer:
Brewed Coffee
4
Step-by-step explanation:
Answer:
Ben's Beans drinks is the correct answer
find the x of these multiplication
6) 3x = 27
7) 5x = 35
8) 2x = 24
Answers
X = 9
7) do the same process, X = 7
8) do the same process, X = 12
Hey there!
QUESTION #6.
3x = 27
DIVIDE 3 to BOTH SIDES
3x/3 =27/3
SIMPLIFY IT!
x = 27/3
x = 9
Therefore, the answer should be:
x = 9
QUESTION #7.
5x = 35
DIVIDE 5 to BOTH SIDES
5x/5 = 35/5
SIMPLIFY IT!
x = 35/5
x = 7
Therefore, the answer should be:
x = 7
QUESTION #9.
2x = 24
DIVIDE 2 to BOTH SIDES
2x/2 = 24/2
SIMPLIFY IT!
x = 24/2
x = 12
Therefore, the answer should be:
x = 12
Good luck on your assignment & enjoy your day!
~Amphitrite1040:)
The following graph describes function 1, and the equation below it describes function 2. Determine which function has a greater maximum value, and provide the ordered pair. (1 point)
Function 1
(look at the graph below)
Function 2
f(x) = −x2 + 2x − 15
Function 1 has the larger maximum at (4, 1).
Function 1 has the larger maximum at (1, 4).
Function 2 has the larger maximum at (−14, 1).
Function 2 has the larger maximum at (1, −14).

Answers
A function that has a greater maximum include the following: A. Function 1 has a larger maximum at (4, 1).
How to determine the function that has a larger maximum?In order to determine the maximum value of function 2, we would have to take the first derivative with respect to x and then, substitute this x-value into the original function while equating it to zero (0), and then evaluate as follows;
f(x) = −x² + 2x - 15
f(x) = −2x + 2
0 = −2x + 2
2x = 2
x = 2/2
x = 1
For the maximum value of function 2, we have:
f(1) = −(1)² + 2(1) - 15
f(1) = -14
For the maximum value of function 1, we can logically deduce that it is equal to 1 based on the graph in image attached above.
Read more on maximum and function here: https://brainly.com/question/1800006
#SPJ1
Test the claim that the proportion of people who own cats is significantly different than 80% at the 0.2 significance level. The null and alternative hypothesis would be:______.
A. H0 : μ = 0.8 H 1 : μ ≠ 0.8
B. H0 : p ≤ 0.8 H 1 : p > 0.8
C. H0 : p = 0.8 H 1 : p ≠ 0.8
D. H0 : μ ≤ 0.8 H 1 : μ > 0.8
E. H0 : p ≥ 0.8 H 1 : p < 0.8
F. H0 : μ ≥ 0.8 H 1 : μ < 0.8
The test is:_____.
a. left-tailed
b. right-tailed
c. two-tailed
Based on a sample of 200 people, 79% owned cats.
The test statistic is:______.
The p-value is:_____.
Based on this we:_____.
A. Fail to reject the null hypothesis.
B. Reject the null hypothesis.
Answers
Answer:
C. H0 : p = 0.8 H 1 : p ≠ 0.8
The test is:_____.
c. two-tailed
The test statistic is:______p ± z (base alpha by 2) \(\sqrt{\frac{pq}{n} }\)
The p-value is:_____. 0.09887
Based on this we:_____.
B. Reject the null hypothesis.
Step-by-step explanation:
We formulate null and alternative hypotheses as proportion of people who own cats is significantly different than 80%.
H0 : p = 0.8 H 1 : p ≠ 0.8
The alternative hypothesis H1 is that the 80% of the proportion is different and null hypothesis is , it is same.
For a two tailed test for significance level = 0.2 we have critical value ± 1.28.
We have alpha equal to 0.2 for a two tailed test . We divided alpha with 2 to get the answer for a two tailed test. When divided by two it gives 0.1 and the corresponding value is ± 1.28
The test statistic is
p ± z (base alpha by 2) \(\sqrt{\frac{pq}{n} }\)
Where p = 0.8 , q = 1-p= 1-0.8= 0.2
n= 200
Putting the values
0.8 ± 1.28 \(\sqrt{\frac{0.8*0.2}{200} }\)
0.8 ± 0.03620
0.8362, 0.7638
As the calculated value of z lies within the critical region we reject the null hypothesis.
The slope of EF is -3/2
Which segments are perpendicular to EF?
Select each correct answer.
LM, where L is at (1, 8) and M is at (3, 11)
JK, where J is at (14, -1) and K is at (5, -7)
NP, where N is at (0, -3) and P is at (2, - 6)
GH, where G is at (1, 7) and His at (4, 9)
Answers
Segment JK is perpendicular to segment EF having slope -3/2 i.e option (b) is correct answer.
What is slope?A value known as slope can be used to define a line's direction and steepness. It is frequently represented by the letter 'm' in variable format. The gradient or rate of change of a line is another name for its slope.
When two points on a line are P(x1,y2) and Q(x2,y2), the slope formula is as follows:
Slope, m = Change in y-coordinates/Change in x-coordinates
m= \(\frac{y_{2} -y_{1} }{x_{2} -x_{1} }\)
Thus, using the a fore mentioned method, we can quickly determine the slope of a line connecting two points.
In other words, the increase of the line between two points (along the y-axis) over the run is referred to as the slope of a line between two points (along x-axis). Therefore,
m = Rise/Run for Slope
In terms of their slopes, lines must be perpendicular.
Let the slopes of two lines be m1 and m2.
The product of the slopes of the two lines is equal to -1 if they are perpendicular.
that is \(m_{1} m_{2} = -1\)
Slope of EF = -3/2
Therefore we can find the slope of the segment which is perpendicular to the segment EF
Put the value of slope of EF in place of \(m_{1}\)
\(m_{1}\) = -3/2
(-3/2)×\(m_{2}\) = -1
\(m_{2}\) = 2/3
As segment JK is formed by J (14,-1) and K (5,-7)
We can find the slope of it by
m = (y2-y1)/(x2-x1)
m = (-7+1)/(5-14)
m = 6/9
m = 2/3
As we got slope of JK equal to \(m_{2}\)
Therefore, Segment JK is perpendicular to the EF
Learn more about perpendicular lines here:
https://brainly.com/question/1202004
#SPJ1
Eumin pays $3.56 for 4 juice boxes.
How much would Eumin pay for 7 juice boxes?
Enter your answer in the box.
Answers
Answer:
No, You divide 3.56/4 and you get 0.89 and then after that you multiply it by 7 so you do0.89x7=6.23
Step-by-step explanation:
How did astronomers determine where Earth is located within the Milky Way?
Answers
answer: The Earth's place in the galaxy was determined largely by an astronomer named Harlow Shapley. Thanks to the regular periods of these stars and their presence in globular clusters, Shapley was able to map the distances to a number of clusters.
Step-by-step explanation:
Answer:
An astronomer Named Harlow Shapley was the one who announced where we are in space ( Earth ). How? with the help with stars brightness ( Absolute Magnitude ) whereas to how bright a stat appears to be ( magnitude ) they use that calculation to determine the distance from Earth. Harlow Shapley couldn't do this on his own as he needed help with another one's work named Henrietta Swan Leavitt. ( Who established that "Variable Stars" could determine the "astronomical distance " )
what is -0.3 x -10/11
Answers
Answer:
-0.3x-10/11=3/11
help with 6 please i need to graduate
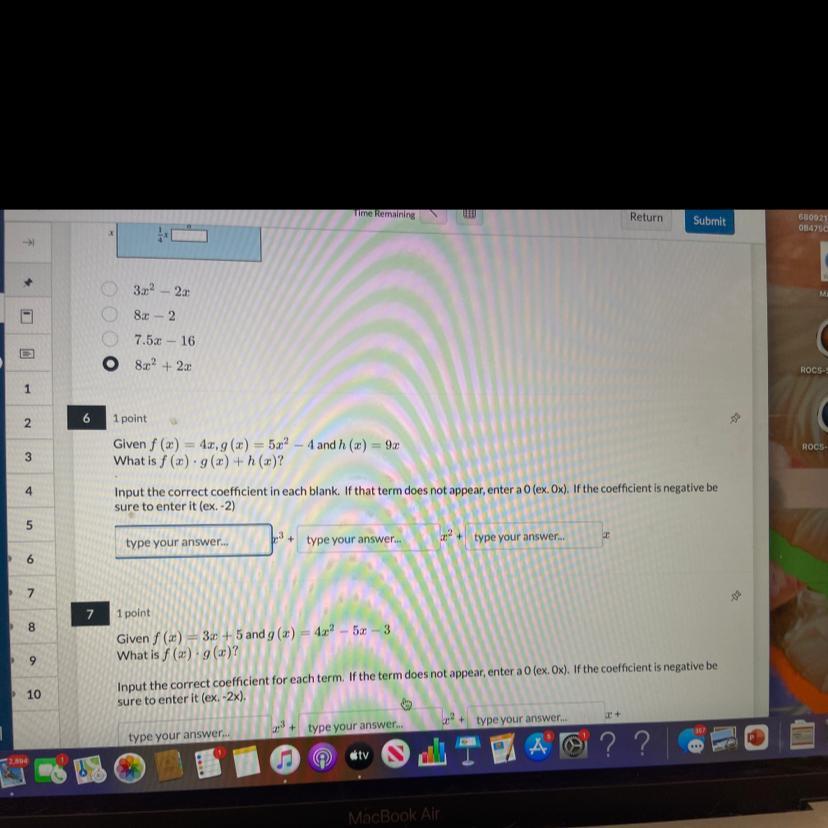
Answers
The expression with the correct coefficients in each block is given as follows:
20x³ + 0x² - 7x.
How to obtain the resultant function?The functions in the context of this problem are defined as follows:
f(x) = 4x.g(x) = 5x² - 4.h(x) = 9x.The operation is given as follows:
f(x)g(x) + h(x).
Hence, to obtain the resultant function, we apply the definitions into the operation and the simplify, applying the distributive property and combining the like terms, as follows:
4x(5x² - 4) + 9x = 20x³ - 16x + 9x = 20x³ - 7x.
As there is no x² term, the coefficient of zero multiplies the term x².
More can be learned about functions at https://brainly.com/question/31895757
#SPJ1
Eggs $8.00 per dozen.
Mrs. Harris bought 5 dozens eggs and sold them at $1.00 each. Calculate her gain or loss percent.
Answers
Subtract. Write your answer in simplest form.
5 1/2- 1 2/7
Answers
Answer:
5 1/2- 1 2/7
11/2-9/7
(11×7-9×2)/14
59/14
4 3/14
A store is having a sale on jelly beans and almonds. For 3 pounds of jelly beans and 5 pounds of almonds, the total cost is $27. For 9 pounds of jelly beans and
7 pounds of almonds, the total cost is $51. Find the cost for each pound of jelly beans and each pound of almonds.
Cost for each pound of jelly beans:
Cost for each pound of almonds:
Answers
Answer:
Cost for each pound of jelly beans: $2.75
Cost for each pound of almonds: $3.75
Step-by-step explanation:
Let J be the cost of one pound of jelly beans.
Let A be the cost of one pound of almonds.
Using the given information, we can create a system of equations.
Given 3 pounds of jelly beans and 5 pounds of almonds cost $27:
\(\implies 3J + 5A = 27\)
Given 9 pounds of jelly beans and 7 pounds of almonds cost $51:
\(\implies 9J + 7A = 51\)
Therefore, the system of equations is:
\(\begin{cases}3J+5A=27\\9J+7A=51\end{cases}\)
To solve the system of equations, multiply the first equation by 3 to create a third equation:
\(3J \cdot 3+5A \cdot 3=27 \cdot 3\)
\(9J+15A=81\)
Subtract the second equation from the third equation to eliminate the J term.
\(\begin{array}{crcrcl}&9J & + & 15A & = & 81\\\vphantom{\dfrac12}- & (9J & + & 7A & = & 51)\\\cline{2-6}\vphantom{\dfrac12} &&&8A&=&30\end{array}\)
Solve the equation for A by dividing both sides by 8:
\(\dfrac{8A}{8}=\dfrac{30}{8}\)
\(A=3.75\)
Therefore, the cost of one pound of almonds is $3.75.
Now that we know the cost of one pound of almonds, we can substitute this value into one of the original equations to solve for J.
Using the first equation:
\(3J+5(3.75)=27\)
\(3J+18.75=27\)
\(3J+18.75-18/75=27-18.75\)
\(3J=8.25\)
\(\dfrac{3J}{3}=\dfrac{8.25}{3}\)
\(J=2.75\)
Therefore, the cost of one pound of jelly beans is $2.75.
Please answer this fast!!
Options:
- SSS
- SAS
- ASA
- AAS
- HL
- Not Possible

Answers
Answer:
SAS
Step-by-step explanation:
Only two sides are proven to be congruent and the angle in between the two is a corresponding angle, making them congruent.
is y=x-3 a function or an equation