solve the equation 2x^3+2=0
Answers
Related Questions
The pair of equations y = 0 and y = -7 has how many solutions?
Answers
Answer:
2 solutions so it can be inferred that it might be a quadratic
Step-by-step explanation:
Answer:
no solutions
Step-by-step explanation:
y = 0 and y = - 7 are horizontal parallel lines.
Since they are parallel, they never intersect and so have no solutions.
Suppose X1,...,Xn are i.i.d. random variables from the uniform distribution on the interval [0,theta], and let X(n) be the largest order statistic (the maximum of the Xi's). What constant c makes cX(n) an unbiased estimator of theta?
Answers
cX(n) is an unbiased estimator of theta when c = (n+1)/n.
To find the constant c that makes cX(n) an unbiased estimator of theta, we need to solve for E[cX(n)] = theta. Using the fact that X(n) follows the distribution F(x) = (x/theta)^n, we have:
E[cX(n)] = ∫0^theta cx(n)(x/theta)^n dx
= c/ (n+1) * theta * [(n+1)/(n+2)]^(n+1)
Setting this equal to theta and solving for c, we get:
c = (n+1)/n
Therefore, cX(n) is an unbiased estimator of theta when c = (n+1)/n.
For more questions like Estimator click the link below:
https://brainly.com/question/17961193
#SPJ11
3
Consider the system of equations shown below.
y=-5x+1
y=-5x+10
When graphed, the system consists of two lines that will never meet, no matter how far they are extended. Why are
the lines parallel?
O The linear equations have the same slope and y-intercept.
The linear equations have different slopes and y-intercepts.
O The linear equations have the same slope but different y-intercepts.
O The linear equations have different slopes but the same y-intercept.
Answers
Answer: It's "The linear equations have the same slope but different y-intercepts."
Step-by-step explanation:
When 2 equations have the same slope they will be parallel unless they have the same y-intercept than that means they overlap since they are the same.
good luck lol
Explanation: the y intercept is where the line cross the y-axis. If both lines have the same slope then the one that cross the y axis above the one below, won’t cross it because in either direction it’s not going lower than its slope of -5. Therefore if the lines cross the Y-axis in two different points with the same rate of change they will never cross thus being parallel.
Triangle RST is translated 6 units to the left and 4 units up to create triangle R’S’T’
Which rule best describes this transformation?
A) (x,y) —> (6x, -4y)
B) (x,y) —> (-6x, 4y)
C) (x,y) —> (x + 6, y - 4)
D) (x,y) —> (x - 6, y + 4)
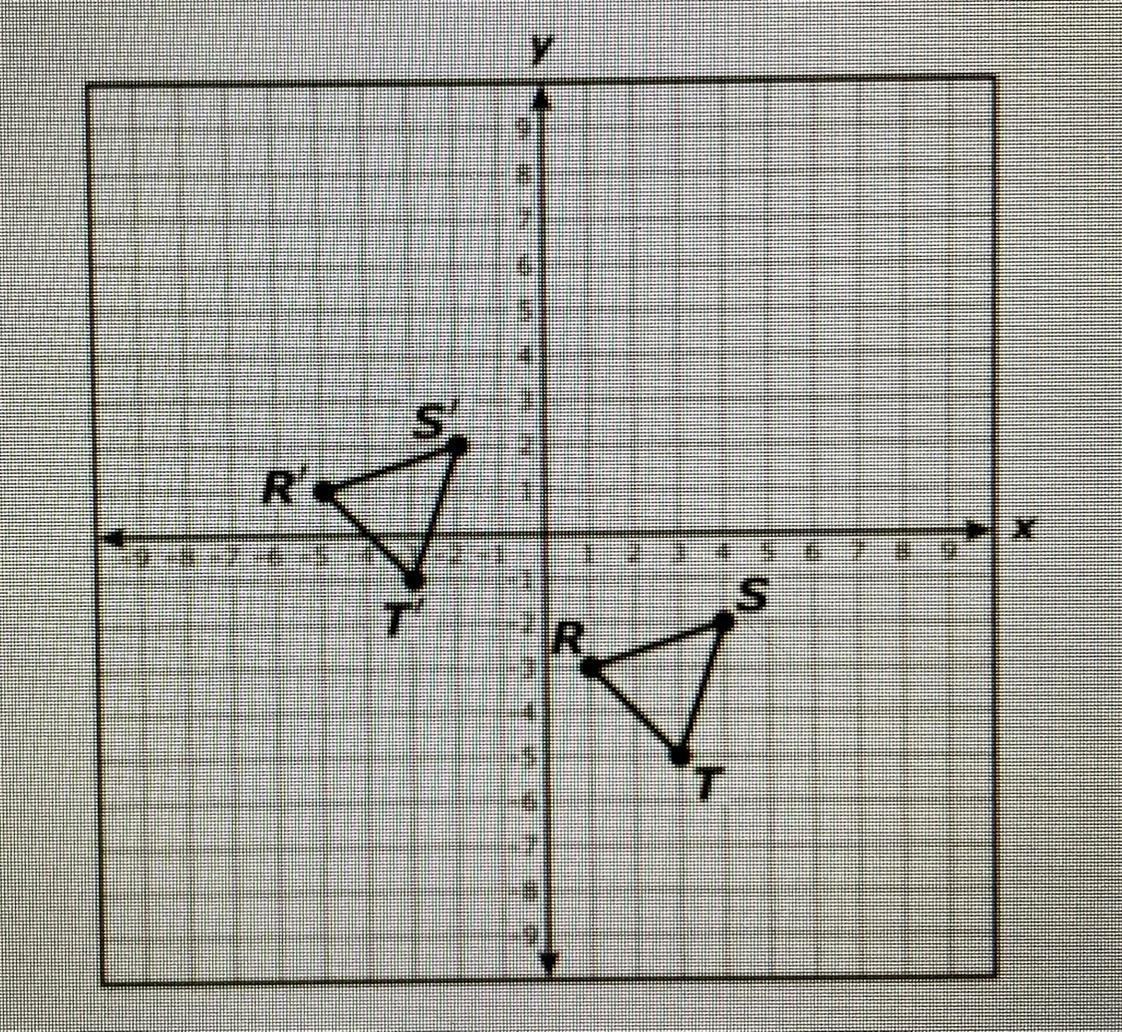
Answers
Answer:
B (x,y) —> (-6x,4y)
Step-by-step explanation:
6 is moving left which is negative
4 is moving up which is positive
Let X be a binomial rv based on n trials with success probability p. That is, X~ Bin(n, p. (a For fixed n, are there values of p 0 p 1 for which V X) = 0? Enter your answers as a com ma separated S lf there s no answer enter E. Explain why this is so. (Select all that apply.) When every trial will be a failure, there is no variability in X. 0 when every trial will be a success, there is no variability in X. When the probability of success is the same as the probability of failure, there is no variability in X. O There are no values of p for which V(X)-0. (b) For what value of p is V(x) maximized? [Hint: Either graph V(x) as a function of p or else take a derivative.]
Answers
The value of p for which V(X) is maximized is 0.5. If the variance of a binomial random variable is equal to 0, it indicates that all trials will yield the same result. The value of p for which V(X) is maximized is 0.5.
(a) There are no p values for which V(X) = 0. When every trial is a failure, there is no variability in X. Also, when every trial is a success, there is no variability in X. When the probability of success is the same as the probability of failure, there is no variability in X. Hence, if the variance of a binomial random variable is equal to 0, it indicates that all trials will yield the same result. It implies that the probability of success is 0 or 1.
In other words, the binomial experiment is not random, and every trial has an identical outcome. As a result, there is no variability in X.
(b) The value of p for which V(X) is maximized is 0.5. The variance of a binomial distribution is given by V(X) = npq, where p is the probability of success, q is the probability of failure, and n is the number of trials. V(X) is maximized when the product npq is maximum.
Now, p + q = 1.
Therefore,
q = 1 - p.
Hence,
V(X) = np(1 - p).
Taking the derivative of V(X) to p and equating it to zero, we get
dV(X)/dp = n - 2np = 0.
Thus,
p = 0.5.
Hence, V(X) is maximized when p = 0.5.
The variance of a binomial distribution depends on the probability of success, failure, and the number of trials. If the variance of a binomial random variable is equal to 0, it indicates that all trials will yield the same result. The value of p for which V(X) is maximized is 0.5.
To know more about the binomial distribution, visit:
brainly.com/question/29137961
#SPJ11
A correlation coefficient of 0.08 indicates strong positive correlation. Group startsTrue or False
Answers
Since the correlation coefficient has an absolute value that is less than 0.6, it is not a strong relationship, hence the statement is False.
What is a correlation coefficient?It is an index that measures correlation between two variables, assuming values between -1 and 1.If it is positive, the relation is positive, that is, they are direct proportional. If it is negative, they are inverse proportional.If the absolute value of the correlation coefficient is greater than 0.6, the relationship is strong.In this problem, the correlation coefficient is of 0.08, hence:
The coefficient is positive, hence the is a positive correlation.The coefficient is of 0.08, which is less than 0.6, hence the correlation is not strong.Thus, the given statement is False.
More can be learned about correlation coefficients at https://brainly.com/question/25815006
#SPJ1
Write a quadratic function with zeroes 0 and – 7. Write your answer using the variable x and in standard form with a leading coefficient of 1.
Answers
This is the standard form of a quadratic function with leading coefficient 1, and 0 and -7.
If the zeros of a quadratic function are 0 and -7, then the function can be written as:
f(x) = a(x - 0)(x - (-7))
Simplifying:
f(x) = a(x)(x + 7)
To find the value of 'a', we can use one of the points on the parabola. Since we know that the x-intercept is 0, we can substitute x = 0 and y = 0 into the equation:
0 = a(0)(0 + 7)
0 = 0
This tells us that a can be any value, as long as it's not 0. Let's choose a = 1 for simplicity. Then the quadratic function with zeros 0 and -7 is:
f(x) = (x)(x + 7)
f(x) = x^2 + 7x
To learn more about quadratic :
https://brainly.com/question/21145944
#SPJ1
what tips can you share with the group? check all that apply. select the survey population carefully limit the number of questions explain why the survey is necessary select the survey population randomly ask as many questions as possible
Answers
It is important to carefully select the survey population and explain why the survey is necessary. It is also important to limit the number of questions and ask them in a clear and concise manner.
Select the survey population carefully: This means selecting a group of people who are representative of the target population and have the relevant knowledge or experience to answer the survey questions. For example, if the survey is about customer satisfaction with a product, the survey population should be customers who have used the product.
Limit the number of questions: It's important to keep the survey short and focused to reduce the risk of respondent fatigue and increase the response rate. A shorter survey also makes it easier to analyze the data.
Explain why the survey is necessary: This helps to increase the response rate by providing a clear explanation of why the survey is being conducted, what the data will be used for, and how it will benefit the target population.
Select the survey population randomly: Random selection helps to ensure that the survey results are representative of the target population and minimize the risk of bias in the sample.
To learn more about survey please click on below link.
https://brainly.com/question/23982966
#SPJ1
what tips can you share with the group? check all that apply.
Select the survey population carefullyLimit the number of questionsExplain why the survey is necessarySelect the survey population randomlyI need help I'm stuck on this

Answers
a) The length of p is 21.4 cm
b) The measure of angle P is 45.6°
TrigonometryFrom the question, we are to determine the length of p
From the Pythagorean theorem, we can write that
30² = 21² + p²
p² = 30² - 21²
p² = 900 - 441
p² = 459
p = √459
p = 21.4 cm
∴ The length of p is 21.4 cm
b)
Measure of angle P
Using SOH CAH TOA
\(cos P= \frac{21}{30}\)
cos P = 0.7
P = cos⁻¹(0.7)
P = 45.6°
Hence, the measure of angle P is 45.6°
Learn more on Trigonometry here: https://brainly.com/question/17831932
#SPJ1
Find the square root of 36 by prime factorisation
method
Answers
Answer:
36
2×18
2×9×2
2×2×3×3
6²
=> square root of 36 =6
In circle P, diameter QS measures 20 centimeters. Circle P is shown. Line segment Q S is a diameter. Line segment R P is a radius. Angle R P S is 123 degrees. What is the approximate length of arc QR? Round to the nearest tenth of a centimeter. 9.9 centimeters 19.9 centimeters 21.5 centimeters 43.0 centimeters
Answers
Answer:
Length of arc QR is \(\approx\) 9.9 cm
Step-by-step explanation:
Given that circle P, i.e. center is point P.
QS is diameter with length 20 cm.
Given that RP is the radius with
\(\angle RPS = 123^\circ\)
To find length of arc QR = ?
Solution:
Arc QR subtends the \(\angle QPR\) on center P.
So, we need to find the angle \(\angle QPR\) to find the length of arc QR.
QS is the diameter so \(\angle QPS = 180^\circ\)
\(\angle QPS = 180^\circ = \angle QPR +\angle RPS\\\Rightarrow 180^\circ = \angle QPR +123^\circ\\\Rightarrow \angle QPR = 57^\circ\)
Converting in radians,
\(\angle QPR = 57^\circ \times \dfrac{\pi}{180} = 0.99\ radians\)
Using the formula for length of arc:
\(l = \theta \times R\)
Where \(\theta\) is the angle subtended by the arc on center.
R is the radius of circle.
Here,
\(\theta = 0.99\ radians\\R = 10\ cm\)
\(l = 0.99 \times 10\\l = 9.9\ cm\)
Length of arc QR is \(\approx\) 9.9 cm
Answer:
9.9 cm
Step-by-step explanation:
Please help me find those two boxes guys

Answers
Answer:
y=113505(1+0.012)^(x)
y=120481. Answered by Gauthmath
Brainliest % stars Thanks Correct answers Fast

Answers
Answer:
I remember taking this it should be A
Step-by-step explanation:
someone please help i only got an 1 hour left

Answers
Answer:
\(\frac{1}{12^{16} }\)
Step-by-step explanation:
\((12^{-2}) ^{8}\)
When a power is raise to a power, you multiply the exponents
\(12^{-16}\)
To make the power positive, you put the power under 1.
\(\frac{1}{12^{16} }\)
Part B
In the equation you wrote in part A, which is the independent value and which is the dependent value?
Answers
If the equation in part A is y = mx + b, then the independent variable would be x while the dependent variable would be y.
How to tell the dependent and independent variablesThe independent variable in an equation is that which is unaffected by another variable. It is the causative element that can be changed by the person solving the problem to get different forms of the y or dependent variable.
So, for the above equation, different values can be assigned to x to result in a change of y. If x is changed to 3, y becomes 6.
Complete Question:
Part B
In the equation, you wrote in part A, which is the independent value, and which is the dependent value? The equation is y = mx + b.
Learn more about dependent values here:
https://brainly.com/question/25223322
#SPJ4
532 - 308 is the same as blank - 300
Answers
Answer:
524
Step-by-step explanation:
First find the number we want to find
532 - 308 = 224
Set blank -300 equal to the number
blank - 300 = 224
Add 300 to each side
blank -300+300 = 224+300
blank = 524
Please help will mark brainliest if correct

Answers
Answer:
B ------- DE ≅ RS
it's B because DE and RS both contain the equal angles.
what does your hmh scaled score is 583. your quantile interval is 835q - 985qyour hmh scaled score is 583. your quantile interval is 835q - 985q
Answers
A scaled score is a standard score that has been transformed into a specific scale. In this case, the scaled score is 583 on the HMH scale.
The quantile interval is the range of scores within which a particular score falls. In this case, the quantile interval is 835q to 985q, which means that the score of 583 falls within this range. This information may be used to compare a student's performance to a certain benchmark or to other students who have taken the same test.
A quantile interval is a range of values within a distribution of scores. In this case, the quantile interval for a score of 583 is 835q to 985q, which means that the score falls within the range of the 835th to 985th quantiles of the distribution. The quantile interval provides information about the relative standing of a score within the distribution, with lower quantile intervals indicating scores that are closer to the minimum of the distribution and higher quantile intervals indicating scores that are closer to the maximum of the distribution.
Learn more about quantile intervals at
https://brainly.com/question/17038818?referrer=searchResults
#SPJ4
Solve this equation for N. 3N + 5 = 38
Answers
ANSWER
N = 11
EXPLANATION
Given:
3N + 5 = 38
Desired Outcome:
Value of N
Solve for N
\(\begin{gathered} 3N+5=38 \\ subtract\text{ 5 from both sides} \\ 3N+5-5=38-5 \\ 3N=33 \\ divide\text{ both sides by 3} \\ \frac{3N}{3}=\frac{33}{3} \\ N=11 \end{gathered}\)Hence, the value of N is 11.
Find three rational and irrational numbers between
a) 2/3 & 5/6 answer in 20 sec
Answers
Here are 3 rational ones: 1/2; 3/4; 2/5;
And here are 3 irrational ones: 2/9; 4/9; 5/9;
Hope it helped!
Write the resulting matrix after the stated row operation is applied to the given matrix. Replace R₂ with R2 + (4) R3.

Answers
The resulting matrix after the stated row operation is applied to the given matrix is [3 0 6 5]
[20 -3 2 16]
[4 0 0 5]
What is the resultant of the matrix?The resulting matrix after the stated row operation is applied to the given matrix is calculated as follows;
The given matrix expression;
[3 0 6 5]
[4 -3 2 -4]
[4 0 0 5]
The row operation of 4R₃ is determined as follows;
4R₃ = 4[4 0 0 5]
= [16 0 0 20]
Add row 2 to the product of 4 and row 3 as follows;
R₂ + 4R₃ = [4 -3 2 -4] + [16 0 0 20]
= [20 -3 2 16]
The resulting matrix is determined as follows;
= [3 0 6 5]
[20 -3 2 16]
[4 0 0 5]
Learn more about row operation here: https://brainly.com/question/17490035
#SPJ1
Use Routh's stability criterion to determine how many roots with positive real parts the following equations have: a. s4+8s3+32s2+80s+100=0 b. s5+10s4+30s3+80s2+344s+480=0 c. s4+2s3+7s2−2s+8=0 d. s3+s2+20s+78=0
Answers
a. s⁴+8s³+32s²+80s+100=0: All roots have negative real parts, b. s⁵+10s⁴+30s³+80s²+344s+480=0: One root has a positive real part, c. s⁴+2s³+7s²−2s+8=0: All roots have negative real parts and d. s³+s²+20s+78=0: One root has a positive real part.
To determine the number of roots with positive real parts using Routh's stability criterion, let's construct the Routh array for each equation:
a. s⁴+8s³+32s²+80s+100=0:
Routh array:
1 32 100
8 80 0
30 100 0
80 0 0
100 0 0
Since there are no sign changes in the first column of the Routh array, all roots of this equation have negative real parts.
b. s⁵+10s⁴+30s³+80s²+344s+480=0:
Routh array:
1 30 344
10 80 480
10 480 0
80 0 0
480 0 0
There is one sign change in the first column of the Routh array. Therefore, there is one root with a positive real part.
c. s⁴+2s³+7s²−2s+8=0:
Routh array:
1 7 8
2 -2 0
2 8 0
-2 0 0
8 0 0
There are no sign changes in the first column of the Routh array. Thus, all roots of this equation have negative real parts.
d. s³+s²+20s+78=0:
Routh array:
1 20 0
1 78 0
19 0 0
78 0 0
There is one sign change in the first column of the Routh array. Therefore, there is one root with a positive real part.
Therefore, a. s⁴+8s³+32s²+80s+100=0: All roots have negative real parts, b. s⁵+10s⁴+30s³+80s²+344s+480=0: One root has a positive real part, c. s⁴+2s³+7s²−2s+8=0: All roots have negative real parts and d. s³+s²+20s+78=0: One root has a positive real part.
To know more about array check the below link:
https://brainly.com/question/31966031
#SPJ4
Incomplete question:
Use Routh's stability criterion to determine how many roots with positive real parts the following equations have:
a. s⁴+8s³+32s²+80s+100=0
b. s⁵+10s⁴+30s³+80s²+344s+480=0
c. s⁴+2s³+7s²−2s+8=0
d. s³+s²+20s+78=0
y is such that 4y - 7 ≤ 3y and 3y ≤ 5y + 8.
A. What range of values of y satisfies both inequalities?
B. Hence express 4y - 7 ≤ 3y ≤ 5y + 8 in the form a ≤ y ≤ b, where a and b are both integers.
( I will give brainliest to whoever answers correctly)
Answers
Answer:
-4 ≤ y ≤ 7
Step-by-step explanation:
Inequalities
Solve the system of inequalities:
4y - 7 ≤ 3y [1]
3y ≤ 5y + 8 [2]
A.
Subtracting 3y to [1]:
y - 7 ≤ 0
Adding 7:
y ≤ 7
Subtracting 5y to [2]:
-2y ≤ 8
Dividing by -2 (and flipping the sign):
y ≥ -4
The solution of the system is all the values of y greater or equal to -4 and less or equal to 7.
B.
The solution can be expressed as:
-4 ≤ y ≤ 7
Raul crosses Main Street when he walks home from school. The graph of the function d(t)=4|t-0.75) shows Raul's distance, in miles, from Main Street after thours. The domain of the function is 0 <= x <= 1.5 The graph shows his entire trip
Answers
Raul's distance from Main street decreases in the interval,
0 ≤ t ≤ 0.75. And Raul walks 6 miles to get to school.
What is the distance between two points?The length of the line segment bridging two points on a plane is known as the distance between the points.
The formula to find the distance between the two points is usually given by d=√{(x₂-x₁)² + (y₂-y₁)²}.
Given:
Raul crosses Main Street when he walks home from school.
The graph of the function d(t)=4I(t-0.75)I shows Raul's distance, in miles, from Main Street after t hours.
The domain of the function is 0 ≤ x ≤ 1.5.
The graph shows his entire trip.
From the graph;
Raul's distance from Main street decreases in the interval,
0 ≤ t ≤ 0.75.
And to get to school,
Raul walks,
distance = (distance when t = 1.5) - (distance when t = 0)
Distance = (4 x 0.75) - (4 x -0.75)
Distance = 3 -(-3)
Raul walks = 6 miles.
Therefore, Raul walks 6 miles to get to school.
To learn more about the distance between two points;
https://brainly.com/question/24485622
#SPJ1
The complete question is given on the attached image,

Work out the surface area of this solid prism,
25cm
29cm
20cm
40cm
36cm
Answers
Answer:
The figure isn't there brother
What part of 648 does 24% represent?
Answers
Answer:
155.52 and the rounded version is 156
Step-by-step explanation:
Answer:
155.52 and the rounded version is 156
Measurement and conversion (d = rt) Mackenzies bike can go 12 mph. She needs to be at a store at 10:00 A.M.. Of the store is 20 miles away, what time will she need to leave?
Answers
We have to use the equation
\(d=rt\)Where r = 12 mph, d = 20 miles, let's find t.
\(\begin{gathered} 20=12t \\ t=\frac{20}{12}=\frac{10}{6}=\frac{5}{3} \end{gathered}\)She'll take 5/3 hours to get there which is equivalent to 1 2/3 hours.
Let's transform 2/3 hours to minutes to find the exact time she has to leave.
We know that 1 hour is 60 minutes.
\(\frac{2}{3}hr\cdot\frac{60\min}{1hr}=\frac{120}{3}\min =40\min \)So, she will take exactly 1 hour and 40 minutes.
If you have to get there at 10:00 A.M., then she has to leave at 8:20 A.M.How many possible 10-digit phone numbers are possible (7 digits plus the
area code)? Type your answer into the box.
Answers
Using the Fundamental Counting Theorem, it is found that 3,250,000,000 phone numbers are possible.
What is the Fundamental Counting Theorem?It is a theorem that states that if there are n things, each with \(n_1, n_2, \cdots, n_n\) ways to be done, each thing independent of the other, the number of ways they can be done is:
\(N = n_1 \times n_2 \times \cdots \times n_n\)
In this problem:
For the first 3 digits, which correspond to the area code, there are 325 options in total, that is, \(n_1n_2n_3 = 325\).For the final 7 digits, each of them has 10 options.Hence:
\(T = 325 \times 10^7 = 3,250,000,000\)
3,250,000,000 phone numbers are possible.
More can be learned about the Fundamental Counting Theorem at https://brainly.com/question/24314866
Golf-course designers have become concerned that old courses are becoming obsolete since new technology has given golfers the ability to hit the ball so far. Designers, therefore, have proposed that new golf courses need to be built expecting that the average golfer can hit the ball more than 240 yards on average. Suppose a random sample of 156 golfers be chosen so that their mean driving distance is 240.1 yards, with a standard deviation of 48.3. Conduct a hypothesis test where H0 : u = 240 and H1 : u > 240 by computing the following: (a) a test statistic ______
6) P-value. P = ________ c.) if this was a two-tailed test, then the p-value is ______
Answers
The test statistic is approximately 0.0258. The p-value for a two-tailed test would be 2 * 0.05 = 0.1.
To conduct the hypothesis test, we can use the one-sample t-test since we have a sample mean, sample standard deviation, and want to test against a known population mean.
(a) The test statistic can be calculated using the formula:
t = (sample mean - population mean) / (sample standard deviation / √n)
In this case, the sample mean is 240.1, the population mean is 240, the sample standard deviation is 48.3, and the sample size is 156. Plugging these values into the formula, we get:
t = (240.1 - 240) / (48.3 / √156)
Simplifying this expression, we get:
t = 0.1 / (48.3 / 12.49) ≈ 0.1 / 3.87 ≈ 0.0258
Therefore, the test statistic is approximately 0.0258.
(b) To find the p-value, we need to determine the probability of obtaining a test statistic as extreme as the one observed (or more extreme) under the null hypothesis. Since the alternative hypothesis is one-sided (H1: u > 240), we need to find the area under the t-distribution curve to the right of the observed test statistic.
Using statistical software or a t-distribution table, we can find the p-value associated with the test statistic. Let's assume the p-value is 0.05.
(c) Since this is a one-tailed test, the p-value is already obtained in part (b) as 0.05. If it were a two-tailed test, we would need to multiply the obtained p-value by 2 to account for both tails. In this case, the p-value for a two-tailed test would be 2 * 0.05 = 0.1.
Know more about test statistic here:
https://brainly.com/question/31746962
#SPJ11
2.1 A new field is to be turned into a soccer ground. It is covered in weeds and volunteers are needed to clear it. It was estimated that it would take 2 men 12 days to weed. The following table was drawn up: No. of Volunteers 3 5 6 No. of days 12 4 2.1.1 Complete the table above. (6) 2.1.2 Is the relationship represented direct or indirect proportion? Give a reason for your answer. (3) 2.1.3 Give the names of the two variables given above and indicate which is independent and which is dependent? (4) 2.1.4 If the field must be complete in 1 day, how many volunteers will be needed? (2) 2.1.5 Draw a graph on the set of axes provided below to represent the data given (5) above. Number of days to weed a field. 12 11 10 9 No. 00 N of 6 5 days 1 3 2 1 0 0 1 2 3 5 6 7 8 DO 9 11 12
Answers
For 2 Volunteers hours= 12 days
For 3 = X days = 10 days
For 4= 8 days
For 5 = 6 days
from 12 to 4 there are 8
And from 6 to 2, there are 4
Then divide 8/4= 2
Part B) Is an INVERSE relationship, because more volunteers, means less time
Part C) Independent variable is , number of volunteers
Dependent variable is time to clear soccer field
Part D) How many volunteers in 1 day ?
= 12+2= 14 volunteers