Solve the proportion
8/3 = g/3
Answers
Answer:
g=8
that is
I hope to be helpful
Related Questions
estimate the sum 1.37+0.9. betwen answers like bettwen 3
Answers
,y) mean that student x is enrolled in class y, where the domain for x consists of all students in your school and the domain for y consists of all classes being
Answers
C(X,Y) is a statement that means a student X from the school is enrolled in class Y which is one of the classes offered by the school.
C(X,Y) is a statement that means a student X from the school is enrolled in class Y which is one of the classes offered by the school. The domain for X consists of all students in the school and the domain for Y consists of all classes being offered. To explain this statement further, firstly it should be noted that the statement is saying that a student (X) is enrolled in a class (Y). Secondly, the term “domain” refers to the set of all possible values that can be used for a given variable. In this case, the domain for X consists of all students in the school, while the domain for Y consists of all classes being offered at the school. Therefore, the statement C(X,y) is saying that a student from the school is enrolled in one of the classes offered by the school.
The complete question: Let C(X,Y) mean that student x is enrolled in class y, where the domain for x consists of all students in your school and the domain for y consists of all classes being
Learn more about statement here
https://brainly.com/question/2370460
#SPJ4
What is the slope of the line represented by the equation y= -1/2x+ 1/4
Answers
Answer:
-1/2
Step-by-step explanation:
y=mx+b is used to find the slope. The m part of the equation is the slope
Answer:
The slope of the line is -1/2 because the standard form is y=mx+b where m represents the slope and b represents the y-intercept.
Step-by-step explanation:
Question 2 of 10 A tessellation could be created using circles like the one shown below. A. True B. False

Answers
Answer:
B. False
Step-by-step explanation:
You want to know if it is true that a tessellation can be created from circles.
TessellationA tessellation is the covering of a plane, using one or more geometric shapes with no overlaps and no gaps.
As the attachment shows, it is not possible to cover a plane with circles, leaving no gaps.
A tessellation cannot be created using circles. The proposition is False.

Identify the property that justifies the following statement:
If ∠1 ≅ ∠2, then ∠2 ≅ ∠1
a. Reflexive Property of Congruence
b. Reflexive Property of Similarity
c. Symmetric Property of Congruence
d. Symmetric Property of Similarity

Answers
If it's not Symmetric Property of Similarity, my bet's on possibly Symmetric Property of Congruence.
Solve: 2m³-5m² - 7m = 0
Answers
Answer:
m = - 1 , m = 0 , m = \(\frac{7}{2}\)
Step-by-step explanation:
2m³ - 5m² - 7m = 0 ← factor out common factor m from each term
m(2m² - 5m - 7) = 0
factorise the quadratic 2m² - 5m - 7
consider the factors of the product of the coefficient of the m² term and the constant term which sum to give the coefficient of the m- term
product = 2 × - 7 = - 14 and sum = - 5
the factors are + 2 and - 7
use these factors to split the m- term
2m² + 2m - 7m - 7 ( factor the first/second and third/fourth terms )
2m(m + 1) - 7(m + 1) ← factor out (m + 1) from each term
(m + 1)(2m - 7)
then
2m³ - 5m² - 7m = 0
m(m + 1)(2m - 7) = 0 ← in factored form
equate each factor to zero and solve for m
m = 0
m + 1 = 0 ( subtract 1 from both sides )
m = - 1
2m - 7 = 0 ( add 7 to both sides )
2m = 7 ( divide both sides by 2 )
m = \(\frac{7}{2}\)
solutions are m = - 1 , m = 0 , m = \(\frac{7}{2}\)
Find the values of x,y, and x in a circle

Answers
Answer: x=20, y=z=160
Step-by-step explanation:

what is the property of 3x(5x7)=(3x5)7
Answers
The property you are referring to is called the associative property of multiplication. According to this property, when multiplying three numbers, the grouping of the numbers does not affect the result. In other words, you can change the grouping of the factors without changing the product.
In the equation you provided: 3x(5x7) = (3x5)7
The associative property allows us to group the factors in different ways without changing the result. So, whether we multiply 5 and 7 first, or multiply 3 and 5 first, the final product will be the same.
pls help me waaaaaaa
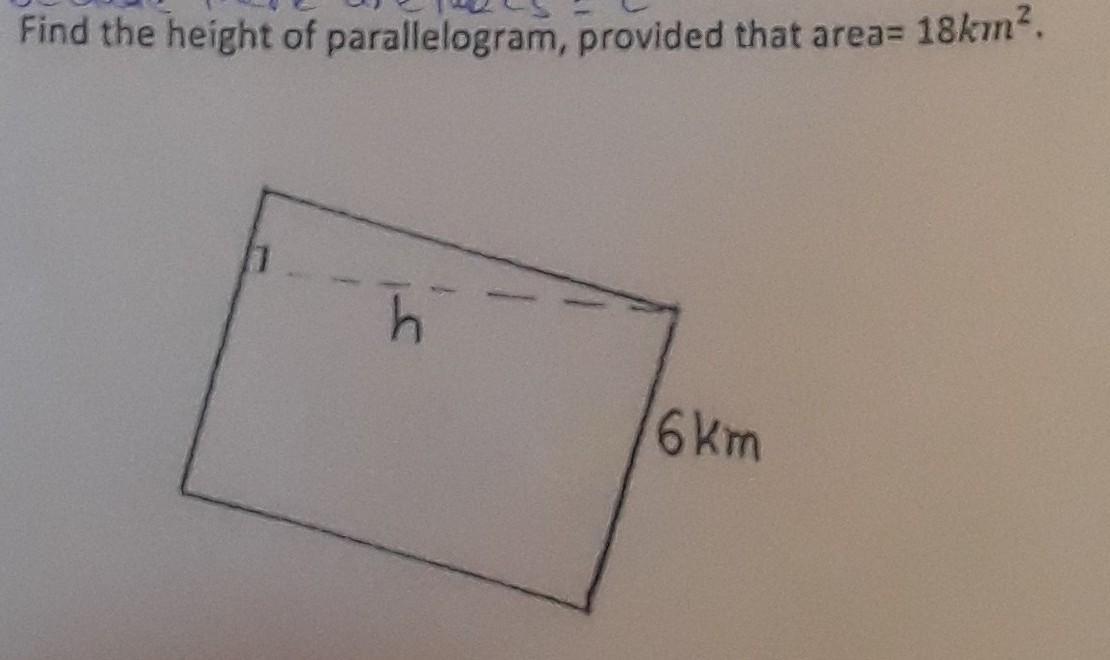
Answers
Answer:
lol lol lol lol lol lol lol lol
Step-by-step explanation:
When Ibuprofen is given for fever to
children 6 months of age up to 2 years, the
usual dose is 5 milligrams (mg) per kilogram
(kg) of body weight when the fever is under
102.5 degrees Fahrenheit. How much
medicine would be usual dose for a 18
month old weighing 21 pounds?
milligrams
Round your answer to the nearest milligram.
Answers
Answer: The usual dose for an 18-month-old weighing 21 pounds is 48 mg of ibuprofen.
Step-by-step explanation: To find the usual dose of ibuprofen for a child, we need to follow these steps:
Convert the child’s weight from pounds to kilograms. One pound is equal to 0.4536 kilograms, so we multiply 21 by 0.4536 to get 9.5256 kilograms.Multiply the child’s weight in kilograms by the dose per kilogram. The dose per kilogram is 5 mg when the fever is under 102.5 degrees Fahrenheit, so we multiply 9.5256 by 5 to get 47.628 mg.Round the result to the nearest milligram. To round a number to the nearest milligram, we look at the digit after the decimal point. If it is 5 or more, we add one to the digit before the decimal point and drop the rest. If it is less than 5, we keep the digit before the decimal point and drop the rest. In this case, the digit after the decimal point is 6, which is more than 5, so we add one to the digit before the decimal point and drop the rest. The result is 48 mg.Therefore, the usual dose for an 18-month-old weighing 21 pounds is 48 mg of ibuprofen. Hope this helps, and have a great day! =)
(Due in 20 minutes)
The three side lengths of four triangles are given. Which triangle is a right triangle?
Triangle 1: √13, 6, 7
Triangle 2: 7, 8, 13
Triangle 3: 10, 11, 12
Triangle 4: √10, 9, 8
Answers
Which expressions are less than 7/8? Choose2 answers 7/8 x 9/19 7/8 x 3/4 7/8 x 9/9 7/8 x 4/3
Answers
The two expressions that are less than 7/8 are 7/8 x 9/19 and 7/8 x 3/4.
To determine which expressions are less than 7/8, we can compare them individually to 7/8 and evaluate their values.
Let's consider each expression one by one:
7/8 x 9/19:
To compare this expression to 7/8, we multiply the fractions:
(7/8) x (9/19) = 63/152.
Since 63/152 is less than 7/8, this expression is less than 7/8.
7/8 x 3/4:
Multiplying the fractions:
(7/8) x (3/4) = 21/32.
Since 21/32 is less than 7/8, this expression is less than 7/8.
7/8 x 9/9:
The fraction 9/9 simplifies to 1, so the expression becomes:
(7/8) x 1 = 7/8.
Since 7/8 is equal to 7/8 (not less than), this expression is not less than 7/8.
7/8 x 4/3:
Multiplying the fractions:
(7/8) x (4/3) = 28/24.
We can simplify 28/24 to 7/6.
Since 7/6 is greater than 7/8, this expression is not less than 7/8.
Therefore, the two expressions that are less than 7/8 are 7/8 x 9/19 and 7/8 x 3/4.
for such more question on expressions
https://brainly.com/question/12270624
#SPJ8
i need help quick!!!

Answers
Answer: A,C, and D
Step-by-step explanation:
Answer:
the answer to this question may be option B, C and D
which inequality statement below is true?
7.745966… > √59
7.745966… < √59
Answers
The inequality statement 7.745966… < √59 is true.
The symbol √59 represents the square root of 59, which is approximately 7.681146. The value 7.745966… is a decimal representation of the square root of 59 that has been rounded to three decimal places. Because 7.681146 is less than 7.745966, the inequality statement 7.745966… < √59 is true.
In ΔGHI, \overline{GI} GI is extended through point I to point J, \text{m}\angle GHI = (3x+13)^{\circ}m∠GHI=(3x+13) ∘ , \text{m}\angle IGH = (x+8)^{\circ}m∠IGH=(x+8) ∘ , and \text{m}\angle HIJ = (6x-5)^{\circ}m∠HIJ=(6x−5) ∘ . Find \text{m}\angle GHI.m∠GHI.
Answers
Given:
In ΔGHI, GI is extended through point I to point J.
\(m\angle GHI=(3x+13)^\circ,m\angle IGH=(x+8)^\circ,m\angle HIJ=(6x-5)^\circ\)
To find:
The measure of angle GHI.
Step-by-step explanation:
According to exterior angle theorem, the measure of an exterior angle of a triangle is equal to the sum of measure of two opposite angles.
Using exterior angle theorem, we get
\(m\angle HIJ= m\angle GHI+m\angle IGH\)
\((6x-5)^\circ=(3x+13)^\circ+(x+8)^\circ\)
\((6x-5)^\circ=(4x+21)^\circ\)
\(6x-4x=21+5\)
\(2x=26\)
Divide both sides by 2.
\(x=13\)
Now,
\(m\angle GHI=(3x+13)^\circ\)
\(m\angle GHI=(3(13)+13)^\circ\)
\(m\angle GHI=(39+13)^\circ\)
\(m\angle GHI=52^\circ\)
Therefore, the measure of angle GHI is 52 degrees.

what is \(24 = 4s\)what is it?
Answers
24 = 4s
4 is multiplying on the rigth, then it will divide on the left
24/4 = s
6 = s
Answer:
\(\sf{6=s}\)Solution:
Hi! To solve this equation, divide both sides by 4.
\(\implies\rm24=4s\\\implies\rm{6=s}\)We come to the conclusion that the answer is 6=s.
Problem solved! It's been a pleasure helping you.
What are the new vertices?

Answers
Answer:
the answer is option c.
Step-by-step explanation:
please mark me brainliest.
jelp pls with 2 work pps brainly

Answers
Answer:
The underline one is 40.
Missing numbers. 35, 40 45, 50, 55, 60, 65, 70.
Step-by-step explanation:
Add 5 rule
Answer:
5. The tens place.
6. 35, 40, 45, 50, 55, 60, 65.... (And so on)
Step-by-step explanation:
Hoped this helped.
The graph of a sinusoidal function intersects its midline at ( 0 , − 6 ) (0,−6)left parenthesis, 0, comma, minus, 6, right parenthesis and then has a minimum point at ( 2.5 , − 9 ) (2.5,−9)left parenthesis, 2, point, 5, comma, minus, 9, right parenthesis. Write the formula of the function, where � xx is entered in radians. � ( � ) = f(x)=f, left parenthesis, x, right parenthesis, equals
Answers
The formula of the function, where x is entered in radians is y = -3sin(πx/5) -6
What is the sinusoidal function?The key components: amplitude, period, phase shift, and vertical shift.
Amplitude is the distance between the midline and max/min points. Midline at (0, -6), so distance to min point (2.5, -9) is 3. Amplitude: 3.
Vertical shift: Displacement along y-axis. Midline at y = -6, vertical shift is -6. Period: distance between max/min points. Graph intersects midline at (0, -6) and minimum point at (2.5, -9). Period is 5 (2 * 2.5). Freq: Reciprocal of period, 1/5.
Phase shift: Horizontal shift of graph. Graph intersects midline at x=0, no phase shift.
Hence:
Amplitude: 3Vertical shift: -6Period: 5Frequency: 1/5Phase shift: 0The general form of the sinusoidal function is y = A sin (B(x-C)) + D
So, Substituting the known values into the general formula:
y = 3 x sin((1/5)x - 0) - 6
Hence: Simplifying it will be:
y = 3 x sin(πx/5) - 6
Then, the formula of the function, where x is entered in radians, is: y = -3sin(πx/5) - 6
Based on the image attached, the first given point is one that informs one that the function is a sine (not a cosine) function, and that it is one that has its offset as -6.
Also, the second given point is one that informs you of the first peak is at x=2.5, hence the argument of the sine function is π/2 if x=2.5: (πx/5).
Based on the fact that the peak is 3 units smaller than the midline, the amplitude is said to be -3.
Therefore the formula for the function is seen as: y = -3·sin(πx/5) -6
Learn more about sinusoidal function from
https://brainly.com/question/16300816
#SPJ1

what is the image point of (-6, -6) after A translation right 2 units and down 3 units
Answers
What is the quotient of negative 210 over negative 15?
Answers
The quotient of A = - 210 and B = - 15 is 14.
What is Euclid's Division Lemma ?The Euclid's Division Lemma says when a number 'a' is divided by a number 'b' gives the quotient to be 'q' and the remainder to be 'r' then the following relation -> a = bq + r exists, where 0 ≤ r < b.
Dividend [a] = Divisor [b] × Quotient [q] + Remainder [r]
Given two numbers A = - 210 and B = - 15
The resultant quotient when A is divided by B can be calculated using Euclid's division lemma. Initially, we will assume remainder [r] = 0.
If the quotient is in decimals, we will change the remainder according to it else if the quotient is a whole number, the remainder will remain 0.
Using Euclid's division lemma -
Dividend [a] = Divisor [b] × Quotient [q] + Remainder [r]
- 210 = - 15 x q + 0
- 210 = - 15 x q
q = - 210 / - 15 = 14
Since, the quotient is a whole number, the remainder will remain zero.
Therefore, the quotient of A = - 210 and B = - 15 is 14.
To solve more questions on Divisions, visit the link below-
https://brainly.com/question/18052636
#SPJ1
In an experiment, the probability that event B occurs is , and the probability that event A occurs given that event B occurs is 3 7) What is the probability that events A and B both occur? Simplify any fractions.
Answers
We have to use the conditional probability formula:
\(P(A|B)=\frac{P(A\cap B)}{P(B)}\)Where P(A|B) is the probability that A occurs given that B occurs, P(B) is the probability that B occurs, and P(A∩B) is the probability that both events A and B occur.
In this case, since we are asked for the probability that events A and B both occur, we need to solve the equation for P(A∩B):
\(P(A\cap B)=P(A|B)\cdot P(B)\)And the information we have about the problem is:
\(\begin{gathered} P(A|B)=\frac{3}{7} \\ P(B)=\frac{2}{9} \end{gathered}\)We substitute this into the formula for P(A∩B):
\(P\mleft(A\cap B\mright)=\frac{3}{7}\cdot\frac{2}{9}\)Solving the multiplication of fractions:
\(\begin{gathered} P\mleft(A\cap B\mright)=\frac{3\cdot2}{7\cdot9} \\ P\mleft(A\cap B\mright)=\frac{6}{63} \end{gathered}\)And finally, we simplify the fraction by dividing both numbers in the fraction by 3:
\(P\mleft(A\cap B\mright)=\frac{2}{21}\)Answer: 2/21
what is the slope of -3 up to positive 3
Answers
The line or points has a slope value of -1
How to determine the slope of the point?From the question, we have the following statement that can be used in our computation:
The slope of -3 up to positive 3
For a start, we represent the above parameters using an ordered pair
The ordered pair is represented as
(x, y) = (-3, 3)
The slope of the point is then calculated using the following slope formula
Slope = (y₂ - y₁)/(x₂ - x₁)
Where
(x, y) = (-3, 3) and (0, 0)
The coordinate (0, 0) represents the origin
Substitute the known values in the above equation
So, we have the following equation
Slope = (3 - 0)/(-3 - 0)
Evaluate the difference
So, we have the following equation
Slope = (3)/(-3)
Evaluate the quotient
So, we have the following equation
Slope = -1
Hence, the slope is -1
Read more about slope at
https://brainly.com/question/29135291
#SPJ1
Which of the following shows the image T of triangle ABC under the transformation (x,y)→(x−4,y+1)?
Answers
The required image T of triangle ABC under the transformation (x,y) → (x-4, y+1) is formed by connecting the vertices A'(a-4, b+1), B'(c-4, d+1), and C'(e-4, f+1)
To find the image T of triangle ABC under the transformation (x,y) → (x-4, y+1), apply this transformation to each of the vertices of triangle ABC and then connect the new vertices to form the image T.
Let's assume that the coordinates of the vertices of triangle ABC are A(a,b), B(c,d), and C(e,f).
To find the image of vertex A under the transformation, we substitute x = a and y = b into the transformation equation:
(x,y) → (x-4, y+1)
(a,b) → (a-4, b+1)
Therefore, the image of vertex A is A'(a-4, b+1).
Similarly, we can find the images of vertices B and C:
B'(c-4, d+1)
C'(e-4, f+1)
Therefore, the image T of triangle ABC under the transformation (x,y) → (x-4, y+1) is formed by connecting the vertices A'(a-4, b+1), B'(c-4, d+1), and C'(e-4, f+1)
Learn more about transformation here:
https://brainly.com/question/27143173
#SPJ1
solve for r a= pi r squared
Answers
I need help please can somebody help me please


Answers
The values of the matrices operations are \(4G + 2F = \left[\begin{array}{ccccc}34&-4&-42&-18&48\\-42&-8&16&30&8\\24&32&4&34&34&-12&-32&-12&-42&-20\end{array}\right]\) and \(4D - 3B= \left[\begin{array}{ccccc}31&6&34&-14&-57\\-19&-27&-18&18&27\\47&14&-1&-40&9&10&12&-19&-15&-58&-14&-23&10&-8&10\end{array}\right]\)
Evaluating the matrices operationsFrom the question, we have the following parameters that can be used in our computation:
\(G = \left[\begin{array}{ccccc}8&-5&-7&-1&10\\-6&-7&1&9&2\\4&6&3&7&5&-4&-3&0&-10&-9\end{array}\right]\)
Also, we have
\(F = \left[\begin{array}{ccccc}1&8&-2&-5&9\\-9&10&6&-3&0\\4&5&-4&3&7&2&-10&-6&-1&-8\end{array}\right]\)
Using the above as a guide, we have
\(4G + 2F = 4\left[\begin{array}{ccccc}8&-5&-7&-1&10\\-6&-7&1&9&2\\4&6&3&7&5&-4&-3&0&-10&-9\end{array}\right] + 2 \left[\begin{array}{ccccc}1&8&-2&-5&9\\-9&10&6&-3&0\\4&5&-4&3&7&2&-10&-6&-1&-8\end{array}\right]\)
Evaluate the sum
\(4G + 2F = \left[\begin{array}{ccccc}34&-4&-42&-18&48\\-42&-8&16&30&8\\24&32&4&34&34&-12&-32&-12&-42&-20\end{array}\right]\)
Next, we have
\(D = \left[\begin{array}{ccccc}7&-6&3&-8&-9\\2&-9&-6&6&9\\8&2&5&-10&-3&10&-3&-4&3&-7&1&-2&4&-5&-2\end{array}\right]\)
Also, we have
\(B = \left[\begin{array}{ccccc}-1&-10&-8&-6&7\\9&-3&-2&2&3\\-5&-2&7&0&-7&10&-8&1&9&10&6&5&2&-4&-9\end{array}\right]\)
The matrix expression is then represented as
\(4D - 3B= 4\left[\begin{array}{ccccc}7&-6&3&-8&-9\\2&-9&-6&6&9\\8&2&5&-10&-3&10&-3&-4&3&-7&1&-2&4&-5&-2\end{array}\right] - 3\left[\begin{array}{ccccc}-1&-10&-8&-6&7\\9&-3&-2&2&3\\-5&-2&7&0&-7&10&-8&1&9&10&6&5&2&-4&-9\end{array}\right]\)
Evaluate
\(4D - 3B= \left[\begin{array}{ccccc}31&6&34&-14&-57\\-19&-27&-18&18&27\\47&14&-1&-40&9&10&12&-19&-15&-58&-14&-23&10&-8&10\end{array}\right]\)
Read more about matrix at
https://brainly.com/question/11989522
#SPJ1
Solve for a, b, and c

Answers
Answer:
a = 24(97√3 -168) ≈ 0.21428002b = 144(26 -15√3) ≈ 2.77025565c = 144(97 -56√3) ≈ 0.74228776Step-by-step explanation:
We can consider the horizontal line to be y=0, and define p, q, r to be the points on the top line left to right between c and 1 at the vertices of the 15° angles.
Then the y-value of r is 1 -tan(15°)². The y-value of q is the square of this, and the y-value of p is the cube (1 -tan(15°)²)³. That makes the value of c be ...
c = (1 -tan(15°)²)⁴
15° is half of 30°, so we can use the tangent half-angle identity to find its value:
tan(15°) = (1 -cos(30°))/sin(30°) = (1 -√3/2)/(1/2) = 2 -√3
This lets us find the exact value of c to be ...
c = (1 -(2 -√3)²)⁴ = (1 -(4 -4√3 +3))⁴ = (4√3 -6)⁴
= (4√3)⁴ -4·6·(4√3)³ +6·6²·(4√3)² -4·6³·(4√3) +6⁴
= 2304 -4608√3 +10368 -3456√3 +1296 = 13968 -8064√3
c = 144(97 -56√3)
__
The value of 'a' is ...
a = p·tan(15°) = (4√3 -6)³·(2 -√3) = (2 -√3)((4√3)³ -3·6·(4√3)² +3·6²(4√3) -6³)
= (2 -√3)(192√3 -864 +432√3 -216) = (2 -√3)(624√3 -1080)
= 1248√3 -2160 -1872 +1080√3 = 2328√3 -4032
a = 24(97√3 -168)
__
The value of b is ...
b = c/tan(15°) = (144)(97 -56√3)/(2 -√3) = (144)(2 +√3)(97 -56√3)/(4 -3)
= 144(194 -112√3 +97√3 -168)/1 = 144(26 -15√3)
b = 144(26 -15√3)
_____
Additional comment
The tangent of an angle in a right triangle is the ratio of the opposite to the adjacent side. For example, tan(15°) = c/b. Then the right-most short segment of the horizontal line is 1·tan(15°). The difference between the vertical at its right (1) and the vertical at its left (r) = tan(15°) times that segment length, or tan(15°)². Then r = 1-tan(15°)². Each vertical segment to the left of that is the previous vertical segment multiplied by this factor:
q = r²
p = r·q = r³
c = r·p = r⁴
__
The binomial expansions we used are ...
(a +b)⁴ = a⁴ +4a³b +6a²b² +4ab³ +b⁴
(a +b)³ = a³ +3a²b +3ab² +b³
Hello I need help with this math it’s negative fractions so -7 1/5 - (-2/5)
Answers
Answer:
-36/5
- . 2/5-=+2/5
-36/5+2/5
34/5 or 6 4/5
Name one pair of congruent sides. A. Segments PR and SV B. Segments QR and ST C. Segments RP and TS D. Segments PQ and VS
Answers
Answer:
Step-by-step explanation:Base on the diagram, and the following question, the following are the answers to your question.
#1 Congruent Angle
#2 Congruent Sides
#3 Side Angle Side
I hope you are satisfied with my answer and feel free to ask for more if you have question and further clarification
Factorise 4x³+8x guys plz
Answers
Answer:
4x (x²+2)
Hope that helps
Answer:
4x(x+2x)
Step-by-step explanation:
There is no further explanations on it