Suppose a conic section passes through the point (2, - 7). If the conic section has focus ( - 1, -3), directrix 2 = 3, then it is a ellipse parabola hyperbola cannot be determined.
Answers
The conic section has focus ( - 1, -3), directrix 2 = 3, then it is a parabola.
How to find if the conic section is a ellipse parabola hyperbola?For a parabola, the focus and directrix play important roles in determining its shape. The distance between a point on the parabola and the focus is equal to the perpendicular distance between that point and the directrix.
Let's calculate the distances and verify if they are equal:
Distance between the point (2, -7) and the focus (-1, -3):
d₁ = √[(2 - (-1))² + (-7 - (-3))²] = √[9 + 16] = √25 = 5
Distance between the point (2, -7) and the directrix 2 = 3:
d₂ = |y - directrix| = |(-7) - 3| = |-10| = 10
Since d₁ ≠ d₂, we can conclude that the conic section cannot be an ellipse.
In an ellipse, the sum of the distances from any point on the ellipse to the two foci is constant. However, in this case, the distances are not equal.
Similarly, for a hyperbola, the difference between the distances from any point on the hyperbola to the two foci is constant. Since the distances are not equal in this case, we can also rule out a hyperbola.
Therefore, based on the given information, the conic section is a parabola.
Learn more about conic section
brainly.com/question/22105866
#SPJ11
Related Questions
Please help it’s due today Solve x+2/3=9/10
Answers
Answer:
\(x=\frac{7}{30}\)
Step-by-step explanation:
Solve for x:
\(x+\frac{2}{3}=\frac{9}{10}\)
Subtract \(\frac{2}{3}\) on both sides
\(x=\frac{7}{30}\)
Answer:
X= 7/30
Step-by-step explanation:
subtract 2/3 from both sides.
X+ 2/3 = 9/10
X +2/3 -2/3 =9/10 -2/3
simplify the expression
X + 2/3 -2/3 =9/10 -2/3
X=7/30
c=180-a-b a=62 and b=33 please help me
Answers
Answer:
\(c=85\)
Step-by-step explanation:
\(c=180-a-b\) where \(a=62\) and \(b=33\)
Step 1. Substitute a for 62 and b for 33
\(c=180-(62)-(33)\)
Step 2. Subtract \((180 - 62)\)
\(c=118-33\)
Step 3. Subtract (again)
\(c=85\)
so c = 85
DUE ON FIDAY PLEASE HELP !!!!!!!!!!
,suppose you have a circle with an arc length of 30π units and a radius of 20 units, without calculating what do you think is the measure of the central angle and why?
Answers
Answer: The arc length, the radius, and the central angle of a circle are related by the formula:
arc length = radius x central angle
Therefore, we can find the central angle by dividing the arc length by the radius:
central angle = arc length / radius
In this case, the arc length is 30π units and the radius is 20 units, so:
central angle = (30π units) / (20 units) = 3π / 2 radians
This is the measure of the central angle in radians. In degrees, we can convert this angle by multiplying by 180/π:
central angle = (3π / 2 radians) x (180/π degrees per radian) ≈ 270 degrees
Therefore, without calculating, I think the measure of the central angle is approximately 270 degrees. This is because the arc length is more than three-quarters of the circumference of the circle (which has a circumference of 2πr = 40π units), so the central angle should be more than three-quarters of a full circle, which is 270 degrees.
Step-by-step explanation:
Help me pleaseeee no links

Answers

From a recent study, it was found that 10% of students travel outside of the country during spring break. Is this an example of descriptive statistics or inferential statistics?.
Answers
Using the definition of descriptive and inferential statistics, it is found that this is an example of descriptive statistics.
What is the definition of descriptive and inferential statistics?A descriptive statistic is one that uses a data-set to describe a population.An inferential statistic is one that makes inference or predictions about a population based on a sample.In this problem:
A recent study was taken from a sample, and from this sample, it was found that 10% of students travel outside of the country during spring break, that is, the sample(data-set) was used to describe a population, so it is an example of descriptive statistics.You can learn more about descriptive and inferential statistics at https://brainly.com/question/21777190
-12 / 3 * (-8 + (-4)^2 - 6) +2
simplified please !!
I need it soon please!
ILL GIVE BRAINIEST
i need the steps too
Answers
Answer:
-6
Step-by-step explanation:
use PEMDAS
start with the exponent: (-4)^2=16
-12 / 3 * (-8 + 16 - 6) +2
then the parentheses: (-8+16-6)=2
-12 / 3 * 2 +2
next is multiplication/division, division comes first so: -12/3=-4
-4 * 2 +2
then multiplication: -4*2=-8
-8+2=-6
Brainliest goes to whoever answers correctly try to show work ONLY if you can also if you want more points answer my other questions

Answers
Answer:
Hi, there for the graph it will be not a function
For the equation it is also not a function
Step-by-step explanation:
For the graph use vertical line test to see if the x value are the same if they are that's means it is not a function.
The X value can't be the same and can't be used twice.
The reason why because given an equation, there should be only one corresponding y value for any x value.
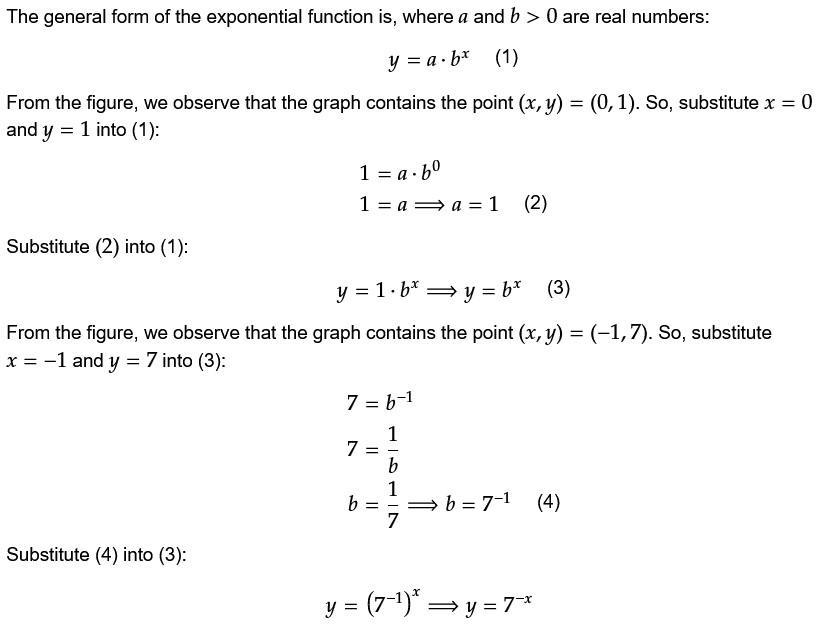
Select one of the following functions to match the graph.
A. y = (7) x
B. y = (7)-x
C. y = (-7) x
D. y = (-7)-x

Answers
Answer:
B
Step-by-step explanation:
The explanation is attached below.
Which of the following is the necessary condition for creating confidence intervals for the population mean? A. Known population parameter B. Normality of the population C. Known standard deviation of the estimator D. Normality of the estimator (e.g., sample mean)
Answers
Answer:
D. Normality of the estimator (e.g., sample mean)
To learn more about confidence intervals visit;
https://brainly.com/question/22556522
#SPJ4
The question was incomplete. Check below the complete question.
Which of the following is the necessary condition for creating confidence intervals for the population mean? A. Known population parameter B. Normality of the population C. Known standard deviation of the estimator D. Normality of the estimator (e.g., sample mean)
Find the volume of a cone with a base diameter of 9 and a height of 12. Write the exact volume in terms of pi , and be sure to include the correct unit in your answer.
Answers
81 is your volume

Answer:
81π cubic units
Step-by-step explanation:
The formula for volume of cone is given by:
V = 1/3πr^2h, where
V is the volume in cubic units,r is the radius of the circular base,and h is the height of the cone.Step 1: Find radius:
We know that the diameter, d, is simply twice the radius. Thus, we can find the radius of the circular base by dividing the given diameter by 2:
d = 2r
d/2 = r
9/2 = r
4.5 units = r
Thus, the radius of the circular base is 4.5 units.
Step 2: Find volume and leave in terms of pi:
We can find the volume in terms of pi by plugging in 4.5 for r and 12 for h and simplifying:
V = 1/3π(4.5)^2(12)
V = 1/3π(20.25)(12)
V = 1/3π(243)
V = 81π cubic units
Thus, the volume of the cone in terms of pi is 81π cubic units.
What 2 time 200000= because i dont know the question so can i get some help
Answers
Answer:
400000
Step-by-step explanation:
In 15 words or fewer, will dividing the two polynomials in the table produce another polynomial? Why or why not?
Answers
Dividing two polynomials may produce another polynomial only when the divisor has a lower degree than the dividend. Otherwise, it will generally result in a rational function.
No, dividing two polynomials may not result in another polynomial. It can produce a rational function.
A polynomial is an algebraic expression consisting of terms with non-negative integer exponents. When two polynomials are divided, the result is not always a polynomial.
If the degree of the polynomial being divided (dividend) is higher than the degree of the polynomial dividing (divisor), then the result can be a polynomial with a lower degree. However, if the degree of the divisor is equal to or greater than the degree of the dividend, the result will generally be a rational function, which is a ratio of two polynomials.
A rational function has the form P(x)/Q(x), where P(x) and Q(x) are polynomials and Q(x) is not equal to zero. The division can introduce terms with negative or fractional exponents, making the result a rational function rather than a polynomial.
For more such questions on polynomial
https://brainly.com/question/1496352
#SPJ8
If a food item with an original (AP) weight of 4 pounds at a cost of $1.10 per pound yields a servable weight of 2 pounds, what is the cost per servable pound for this food item? a. $0.50 b. $1.50 c. $2.20 d. $4.40
Answers
Given that the cost is $1.10 per pound, we can calculate the cost per servable pound by dividing the total cost ($1.10 * 4 pounds) by the servable weight (2 pounds). Therefore, the correct option is c. $2.20.
The original weight of the food item is 4 pounds, and the cost per pound is $1.10. Therefore, the total cost of the food item is 4 pounds * $1.10 = $4.40.
The servable weight of the food item is 2 pounds. To find the cost per servable pound, we divide the total cost ($4.40) by the servable weight (2 pounds):
Cost per servable pound = Total cost / Servable weight = $4.40 / 2 pounds = $2.20.
Hence, the cost per servable pound for this food item is $2.20. Therefore, the correct option is c. $2.20.
To learn more about cost click here, brainly.com/question/14566816
#SPJ11
SOMEONE HELP ME ASAPPPP PLEASE IM SO SLow

Answers
Answer:
b
Step-by-step explanation:
Answer:
The answer for question would be 3
a survey asks a random sample of 1500 adults in ohio if they support an increase in the state sales tax from 5% to 6%, with the additional revenue going to education. let ^ p denote the proportion in the sample who say they support the increase. suppose that 8% of all adults in ohio support the increase. the standard deviation of the sampling distribution is
Answers
The standard deviation of the sampling distribution is 0.00702.
In this case, we know that 8% of all adults in Ohio support the increase. We can use this information, along with the sample size of 1500, to calculate the standard deviation of the sampling distribution. The formula for the standard deviation of the sampling distribution is:
standard deviation = √ [(population proportion x (1 - population proportion)) / sample size]
Plugging in the numbers, we get:
standard deviation = √ [(0.08 x 0.92) / 1500]
standard deviation = √0.00004928
standard deviation = 0.00702
To know more about standard deviation here
https://brainly.com/question/16555520
#SPJ4
a rectangular parking lot is 67.5 ft wide and 148 ft long. what is the area of the parking lot in square meters?
Answers
The area of the rectangular parking lot is 929.03 square meters.
Use the formula for the area of a rectangle to calculate the area of the rectangular parking lot, which is given as:
Area = length × width
We know that the parking lot is 67.5 ft wide and 148 ft long, the area can be calculated as follows:
Area = 67.5 ft × 148 f
t= 9990 sq. ft
However, the question asks for the area in square meters, so we need to convert square feet to square meters. 1 square foot is equal to 0.092903 square meters, so we can use this conversion factor to convert square feet to square meters.
Area in square meters = Area in square feet × 0.092903
= 9990 sq. ft × 0.092903
= 929.03 sq meters
Therefore, the area is 929.03 square meters.
Learn more about rectangular https://brainly.com/question/32444543
#SPJ11
4 - 3
0
4
-2
2
6
If A=
31 and B=
5
2.
0
find A-B.
-4
- 2
2
0
4
-4
5 01
9
3
4 3
00N
1
- 6
1
0
-6 11
12 0
20
2 0-11
-1 4 3
-6-1
4
-80
-1
Answers
Answer:
4
Step-by-step explanation:
the company has been a long term solution to the point that 666666
10
be
=1
90 cm
b
Save answer
=1
el
54 cm
el
=1
19
20
1
What is the length of the missing leg? 1cessary, round to the nearest tenth.
centimeters
o
G
6
22 23
4
24
25
26
Answers
The length of the missing leg is approximately 72 centimeters.
To find the length of the missing leg, we can use the Pythagorean theorem.
According to the given information, we have a right triangle with two known sides:
One leg: 90 cm
Hypotenuse: 54 cm
Let's denote the missing leg as "x" cm.
The Pythagorean theorem states that the square of the hypotenuse is equal to the sum of the squares of the other two sides.
Therefore, we can set up the following equation:
\(90^2 + x^2 = 54^2\)
Simplifying the equation, we have:
\(8100 + x^2 = 2916\)
Subtracting 2916 from both sides:
\(x^2 = 8100 - 2916\)
\(x^2 = 5184\)
Taking the square root of both sides:
x = √5184
x ≈ 72 cm (rounded to the nearest tenth)
For similar question on length.
https://brainly.com/question/31511655
#SPJ8
NEED HELP ASAP PLEASE THANKS!

Answers
The value of g(4) in the function is -5 which is option b
What is the value of g(4)To find the value of g(4) in the composite function, we need to evaluate the function when x = 4
In the first equation, since x is greater than 4 already, we can't use ot.
Let's proceed to the second equation;
g(x) = -2x + 3, x ≤ 4
Let's put the value x = 4 into the equation;
g(4) = -2(4) + 3
g(4) = -8 + 3
g(4) = -5
Learn more on composite function here;
https://brainly.com/question/10687170
#SPJ1
The blood platelet count of a group of women have bell-shaped distribution with a mean of 245.5 and a standard deviation of 68.2 (all units are 1000 cells/ L) Using the empirical rule, fill in the blanks below (Round to the nearest hundredth):
a. Approximately 95% of healthy women in this group
b. Approximately 99.7% of healthy women in this have blood platelet counts between
group have blood platelet counts between
and(1000 cells/ ML). and (1000 cells/ ML).

Answers
The blood platelet count is an illustration of normal distribution Approximately 95% of the data lies within 2 standard deviations of the mean. There are approximately 99.7% of women with platelet count between 65.2 and 431.8
The given parameters are:
μ = 248.5
\(\sigma = 61.1\\\)
(a) The percentage within 2 standard deviation of mean or between 126.3 and 370.7
Start by calculating the z-score, when x = 126.3 and x = 370.7
\(Z = \frac{(x-u)}{\sigma}\)
So, we have:
x = -2
Also,
x = 2
The empirical rule states that:
Approximately 95% of the data lies within 2 standard deviations of the mean.
Hence, there are approximately 95% of women with platelet count within 2 standard deviations of the mean.
(b) The percentage with platelet count between 65.2 and 431.8
Start by calculating the z-score, when x = 65.2 and x = 431.8
So, we have:
Z =-3
Also:
Z = 3
The empirical rule states that:
Approximately 99.7% of the data lies within 3 standard deviations of the mean.
Hence, there are approximately 99.7% of women with platelet count between 65.2 and 431.8
Learn more about normal distributions at:
brainly.com/question/4260416
#SPJ1
At what ordered pair is "Parking Lot B" located at?
Question 1 options:
(-4, 1)
(-3, 4)
(7, -3)
(4, -3)
Answers
x² - kx+4-0
If the equation has
equal roots, then
b²-4ac=0
where a=1, b = -k and c=4
Answers
Answer:
k = ± 4
Step-by-step explanation:
Given
x² - kx + 4 = 0 ← in standard form
with a = 1, b = - k, c = 4
The equation has equal roots, thus the discriminant
b² - 4ac = 0, that is
(- k)² - (4 × 1 × 4) = 0
k² - 16 = 0 ( add 16 to both sides )
k² = 16 ( take the square root of both sides )
k = ± \(\sqrt{16}\) = ± 4
You must show all your work to earn credit for this problem. Triangle ABC below is an isosceles triangle. What is the measure of the vertex angle?

Answers
Answer:
angle ABC = 36 degrees
Step-by-step explanation:
6x = (4x+24)
2x = 24
x = 12
angle ABC = 180 -6x - (4x+24)
angle ABC = 180 - 6(12) - 4(12) - 24 = 36 degrees
If f(x)=(1)/(3)x-5,g(x)=-4x^(2)-5x+9, and h(x)=(1)/(x-8)+3, find g(-2). Type your exact answer, simplified if necessary, in the empty text box.
Answers
To find g(-2), we'll substitute -2 for x in the equation g(x) = -4x² - 5x + 9. So,g(-2) = -4(-2)² - 5(-2) + 9g(-2). The value of g(-2) is -6.
To find g(-2), substitute -2 for x in the equation
g(x) = -4x² - 5x + 9 to get
g(-2) = -6 + 9g(-2)
We are given three functions as follows:
f(x) = (1/3)x - 5, g(x)
= -4x² - 5x + 9, and
h(x) = 1/(x - 8) + 3.
We are asked to find g(-2), which is the value of g(x) when x = -2.
Substituting -2 for x in the equation g(x) = -4x² - 5x + 9, we get
g(-2) = -4(-2)² - 5(-2) + 9.
This simplifies to g(-2) = -16 + 10 + 9 = -6.
Hence, g(-2) = -6.
The value of g(-2) is -6.
To know more about substitute visit:
brainly.com/question/29383142
#SPJ11
lauren has a 9 ft. long peice of wire. if she cuts it into 2/3 ft. long. how many peices will she have? How much wire will she have left?
Answers
Answer:
Lauren will have 13 pieces of wire, each 2/3 ft. long, and 1/3 ft. of wire left over.
What is the fundamental theorem of algebra state and prove?
Answers
The Fundamental Theorem of Algebra states that every non-constant single-variable polynomial with complex coefficients has at least one complex root. This theorem is important as it provides a way to prove the existence of solutions to polynomial equations and provides an analytical tool to find the exact location of the solutions.
This theorem is also known as the algebraic version of the Intermediate Value Theorem as it states that if a polynomial is continuous on a closed interval, then it must take on all values between its maximum and minimum.
The theorem can be easily proven by considering a single-variable polynomial of degree n and transforming it into a polynomial of degree n−1 with the same roots. By repeating this process, the polynomial can be reduced to a constant and hence, it must have at least one root.
know more about Algebra here
https://brainly.com/question/24875240##
#SPJ11
Show how Polly can share 12 sweets in the ratio of 3:1
Answers
Answer:
3 and 9
Step-by-step explanation:
let,the ratio be 3x and 1x
3x+1x=12
4x=12
x=12/4
x=3
3x=3*3=9
x=3
Hence,the ratio are 3 and 9
Is the point (11,5) on the parabola? Show or explain your work.

Answers
Answer:
The point (11, 5) is NOT on the parabola.
Step-by-step explanation:
Let \((x_0,y_0)\) be any point on the parabola
Let \((a, b)\) be the focus
Let \(y=c\) be the the directrix
Distance between \((x_0,y_0)\) and the focus: \(\sqrt{(x_0-a)^2+(y_0-b)^2}\)
Distance between \((x_0,y_0)\) and the directrix is \(|y_0-c|\)
Given:
focus (6, 4)directrix y = 0Therefore:
Distance between \((x_0,y_0)\) and the focus: \(\sqrt{(x_0-6)^2+(y_0-4)^2}\)
Distance between \((x_0,y_0)\) and the directrix is \(|y_0-0|\)
Equate the two expressions and solve for \(y_0\):
\(\sqrt{(x_0-6)^2+(y_0-4)^2}=|y_0-0|\)
\(\implies (x_0-6)^2+(y_0-4)^}=(y_0-0)^2\)
\(\implies {x_0}^2-12x_0+36+{y_0}^2-8y_0+16={y_0}^2\)
\(\implies {x_0}^2-12x_0-8y_0+52=0\)
\(\implies 8y_0={x_0}^2-12x_0+52\)
\(\implies y_0=\dfrac18{x_0}^2-\dfrac{12}{8}x_0+\dfrac{52}{8}\)
\(\implies y_0=\dfrac18{x_0}^2-\dfrac32x_0+\dfrac{13}{2}\)
Now rewrite with (x, y):
\(\implies y=\dfrac18x^2-\dfrac32x+\dfrac{13}{2}\)
Therefore, this is the equation of the parabola
To determine if the point (11, 5) is on the parabola, input x = 11 into the equation:
\(\implies y=\dfrac18(11)^2-\dfrac32(11)+\dfrac{13}{2}\)
\(\implies y=\dfrac{41}{8}\)
So the point (11, 5) is NOT on the parabola.
does any one know the Inequality Notation for this [9,∞)
Answers
Answer:
x>=9
Step-by-step explanation:
so, we can plot this on a number line
the point at 9 is a solid dot, since brackets (not parenthasis) indicates that we including the number. the shaded part of the line extends all the way to positive infinity. the shaded part means that it is a solution, while the un-shaded parts aren't solutions to [9,infinity) it is x>=9 and not x>9 because 9 is included.
x>=9 is the answer
why do you think a compass needle always points north? (write your initial ideas. it is ok if you are unsure about them.)
Answers
The compass needle always points north because it is magnetic north pole.
Earth has magnetic field due to magnetic elements present in Earth's core in molten form. They are assumed to be in the form of giant bar to ease the calculations concerning magnetic field and polarity. The assumptions are geographic south pole harbours magnetic north pole and vice versa for the opposite pole.
So, we know that like poles attract each other and unlike poles repel each other. The north end of magnetic needle will point towards the magnetic south pole which is geographic north pole. Thus, we see compass needle directed to North pole.
Learn more about magnets -
https://brainly.com/question/2320811
#SPJ4