tan theta equals 8 / 15 find sine theta + cos theta / cos theta (1 - cos theta)
Answers
I guess you have to find
\(\dfrac{\sin\theta+\cos\theta}{\cos\theta(1-\cos\theta)}\)
given that \(\tan\theta=\frac8{15}\).
We can immediately solve for \(\sec\theta\):
\(\sec^2\theta=1+\tan^2\theta\implies\sec\theta=\pm\dfrac{17}{15}\)
(without knowing anything else about \(\theta\), we cannot determine the sign)
Then we get \(\cos\theta\) for free:
\(\cos\theta=\dfrac1{\sec\theta}=\pm\dfrac{15}{17}\)
and we can now solve for \(\sin\theta\):
\(\sin^2\theta+\cos^2\theta=1\implies \sin\theta=\pm\dfrac8{17}\)
Notice that we have 2*2 = 4 possible choices of sign for either sin or cos.
• If both are positive, then
\(\dfrac{\sin\theta+\cos\theta}{\cos\theta(1-\cos\theta)}=\dfrac{391}{90}\)
• If both are negative, then
\(\dfrac{\sin\theta+\cos\theta}{\cos\theta(1-\cos\theta)}=\dfrac{391}{480}\)
• If sin is positive and cos is negative, then
\(\dfrac{\sin\theta+\cos\theta}{\cos\theta(1-\cos\theta)}=\dfrac{119}{480}\)
• If cos is positive and sin is negative, then
\(\dfrac{\sin\theta+\cos\theta}{\cos\theta(1-\cos\theta)}=\dfrac{119}{30}\)
Related Questions
slope: -2, y-intercept: -3
Write an equation of a line in slope intercept form with the given slip and y intercept
Answers
Answer:
Step-by-step explanation:
The slope intercept form of a line is Y=MX + B where M is the slope and B is the y-intercept.
We know both of these so the equation turns into Y=1/2X-3
93,83,65,59,88,76,86,93,48,73,54,79
What is the percentage of these test scores that are less than 88?
Answers
Find the slope of the line passing through the points (-7,9) and (7,9)
Answers
Answer:
slope is zero
Step-by-step explanation:
Since the y coordinate of both points is 9
there is no rise between the points
therefor the slope is zero
it's a horizontal line
A random number generator is used to select an integer from 1 to 100 (inclusively). What is the probability of selecting the integer 730?
Answers
If a random number generator is used to select an integer from 1 to 100, then the probability of selecting the integer 730 is zero.
Here a random number generator is used to select an integer from 1 to 100.
Therefore the range of the outcome = 1 to 100
Here we have to find the probability of selecting the integer 730
The probability = Number of favorable outcomes / Total number of outcomes.
Here a random number generator is used to select an integer from 1 to 100, but the given number is 730 which is out of range. Therefore the probability is zero
Hence, if a random number generator is used to select an integer from 1 to 100, then the probability of selecting the integer 730 is zero.
Learn more about probability here
brainly.com/question/11234923
#SPJ1
help I don't understand

Answers
With the help of proportions in the given similar triangles we know that the value of x is 3.5 units.
What is triangle similarity?Euclidean geometry states that two objects are comparable if they have the same shape or the same shape as each other's mirror image.
One can be created from the other more precisely by evenly scaling, possibly with the inclusion of further translation, rotation, and reflection.
These three theorems—Angle-Angle (AA), Side-Angle-Side (SAS), and Side-Side-Side (SSS)—are reliable techniques for figuring out how similar triangles are to one another.
So, in the given situation:
TR and WY are as follows:
TR/WU
24/2
2/1
Similarly,
TS/WV
2/1
7/x
7/3.5
Therefore, with the help of proportions in the given similar triangles we know that the value of x is 3.5 units.
Know more about triangle similarity here:
https://brainly.com/question/14285697
#SPJ1
Calculus 2 master needed; stuck on evaluating the integral please show steps \(\int {sec(x/2)tan^5(x/2)} \, dx\) I am thinking that we want to split up the tan^5, making \(\int {sec(x/2)tan^2 tan^3(x/2)} \, dx\) and then \(\int {sec(x/2)*sec^2x-1* tan^3(x/2)} \, dx\) but I am not sure this is correct. Can anyone help? The thing I am unsure of is that tan^3 is still odd, so would we do the same thing again and factor so we will be left with just tanx?
Answers
Answer:
\(\displaystyle \int \sec\left(\frac{x}{2}\right)\tan^5\left(\frac{x}{2}\right)dx=\frac{2\sec^5\left(\dfrac{x}{2}\right)}{5}-\frac{4\sec^3\left(\dfrac{x}{2}\right)}{3}+2\sec\left(\frac{x}{2}\right)+C\)
Step-by-step explanation:
We want to find the integral:
\(\displaystyle \int \sec\left(\frac{x}{2}\right)\tan^5\left({\frac{x}{2}\right)dx\)
First, perform the substitution y = x / 2. This yields:
\(\displaystyle dy = \frac{1}{2} \, dx \Rightarrow dx = 2\, dy\)
Hence, the integral becomes;
\(\displaystyle =2\int \sec y\tan^5 y \, dy\)
Next, as you had done, let's expand the tangent term but to the fourth:
\(\displaystyle =2\int \sec y\tan^4 y\tan y\, dy\)
Recall that:
\(\tan^2(y)=\sec^2(y)-1\)
Hence:
\(\displaystyle =2\int \sec y(\sec^2 y-1)^2\tan y\, dy\)
We can use substitution once more. This time, let u = sec(y). Hence:
\(\displaystyle du = \sec y \tan y \, dy\)
Rewrite:
\(\displaystyle =2\int \left((\sec^2y-1)^2\right)\left(\sec y \tan y\right)dy\)
Therefore:
\(\displaystyle = 2\int (u^2 - 1)^2\, du\)
Expand:
\(\displaystyle =2\int u^4-2u^2+1\, du\)
Reverse Power Rule:
\(\displaystyle = 2\left(\frac{u^5}{5} - \frac{2u^3}{3} + u\right) + C\)
Simplify:
\(\displaystyle = \frac{2u^5}{5} - \frac{4u^3}{3} + 2u + C\)
Back-substitute:
\(\displaystyle = \frac{2\sec^5 y }{5}-\frac{4\sec^3 y}{3}+2\sec y+C\)
Back-substitute:
\(\displaystyle =\frac{2\sec^5\left(\dfrac{x}{2}\right)}{5}-\frac{4\sec^3\left(\dfrac{x}{2}\right)}{3}+2\sec \left(\frac{x}{2}\right)+C\)
Therefore:
\(\displaystyle \int \sec\left(\frac{x}{2}\right)\tan^5\left(\frac{x}{2}\right)dx=\frac{2\sec^5\left(\dfrac{x}{2}\right)}{5}-\frac{4\sec^3\left(\dfrac{x}{2}\right)}{3}+2\sec\left(\frac{x}{2}\right)+C\)
Answer:
= 2 (sec (x/2) - 2sec³(x/2) + sec⁵(x/2) ) + C
3 5
Step-by-step explanation:
∫sec(x/2) tan⁵(x/2) dx
apply u substitute u = x/2
= ∫sec(u) tan⁵(u) * 2du
= 2 * ∫sec(u) tan⁴(u) tan(u) du
= 2 * ∫sec(u) (tan²(u))² tan(u) du
= 2 * ∫sec(u) (-1 + sec²(u))² tan(u) du
apply u substitute v = sec(u)
= 2 * ∫(-1 + v²)² dv
expand
= 2 * ∫1 - 2v² + v⁴ dv
sum
= 2 (v - 2v³ + v⁵ )
3 5
substitute it back
= 2 (sec (x/2) - 2sec³(x/2) + sec⁵(x/2) )
3 5
add constant to the solution.
= 2 (sec (x/2) - 2sec³(x/2) + sec⁵(x/2) ) + C
3 5
An electrician cuts a 50 ft piece of wire into 3 pieces. The first piece is 4 ft longer than the second piece and the third piece is 2 feet shorter than the second piece. How long are the pieces?
Answers
Answer: 1st is 20 2nd is 16 and 3rd is 14
Step-by-step explanation:
find the value of expression with a=1/3, b=9, c=5, d=10; the equation is d^2 /2c - b + 3a)
Answers
Equation:
\(d^2\colon2c-b+3a\)Replace d with 10, b with 9 , c with 5, and a with 1/3.
\(\frac{10^2}{2\cdot5}-9+3\cdot\frac{1}{3}\)Solve:
\(\frac{100}{10}-9+1\)\(10-9+1\)\(2\)210 people are asked how many siblings they have?
# of Siblings Frequency Relative Frequency Cumulative Frequency
43
43
41
0
1
2
3
4
43
Submit Question
0.2048
0.2048
0.2048
0.1905
43
86
127
210
a. Complete the table (Use 4 decimal places when applicable)
b. What percent of the people have exactly one sibling?
Hint: Frequency Tables

Answers
The frequency table is completed and the percent of the people who have exactly one sibling is 20.48%.
What is Relative Frequency and Cumulative Frequency?Relative frequency is found by dividing the frequency with the total number of values.
Cumulative frequency is calculated by adding the previous frequencies together.
(a) From the table,
Total number of people = 210
So, Total frequency = 210
Frequency of number of siblings as 4 = 210 - (43 + 43 + 41 + 43) = 40
Relative frequency = Frequency / Total frequency
Relative frequency of number of siblings as 2 = 0.1952
Cumulative frequency of number of siblings as 3 = 127 + 43 = 170
(b) Percent of people having exactly 1 sibling = (43 / 210) × 100
= 20.48%
Hence the percent of people having exactly 1 sibling is 20.48%.
Solve more problems on Relative and Cumulative Frequency here :
https://brainly.com/question/24066370
#SPJ9
1. Given the following information about trig ratios, determine the value of sin 0 and cot 0. Make sure you answer
is given in simplified radical form.
sec and sin 0 <0
7
2

Answers
The solution is, sinФ = √45/7 & cotФ =2/√45.
What is trigonometry?Trigonometry is a branch of mathematics that studies relationships between side lengths and angles of triangles.
here, we have,
secФ = 7/2
so, cosФ = 2/7
cos^2Ф = 4/49
i.e. sin^2Ф = 1- cos^2Ф
= 1 - 4/49
= 45/49
so, sinФ = √45/7
now, we know,
cotФ = cosФ/sinФ
= 2/√45
Hence, The solution is, sinФ = √45/7 & cotФ =2/√45.
To learn more trigonometry click:
brainly.com/question/26719838
#SPJ1
NO LINKS!! URGENT HELP PLEASE!!!
11. Write the equation for the graph

Answers
This is the same as writing y = sqrt(4(x+5)) - 1
===============================================
Explanation:
The given graph appears to be a square root function.
The marked points on the curve are:
(-4,1)(-1,3)(4,5)Reflect those points over the line y = x. This will have us swap the x and y coordinates.
(-4,1) becomes (1,-4)(-1,3) becomes (3,-1)(4,5) becomes (5,4)Recall the process of reflecting over y = x means we're looking at the inverse. The inverse of a square root function is a quadratic.
----------
Let's find the quadratic curve that passes through (1,-4), (3,-1) and (5,4).
Plug the coordinates of each point into the template y = ax^2+bx+c.
For instance, plug in x = 1 and y = -4 to get...
y = ax^2+bx+c
-4 = a*1^2+b*1+c
-4 = a+b+c
Do the same for (3,-1) and you should get the equation -1 = 9a+3b+c
Repeat for (5,4) and you should get 4 = 25a+5b+c
We have this system of equations
-4 = a+b+c-1 = 9a+3b+c4 = 25a+5b+cUse substitution, elimination, or a matrix to solve that system. I'll skip steps, but you should get (a,b,c) = (1/4, 1/2, -19/4) as the solution to that system.
In other words
a = 1/4, b = 1/2, c = -19/4
We go from y = ax^2+bx+c to y = (1/4)x^2+(1/2)x-19/4
----------
Next we complete the square
y = (1/4)x^2+(1/2)x-19/4
y = (1/4)( x^2+2x )-19/4
y = (1/4)( x^2+2x+0 )-19/4
y = (1/4)( x^2+2x+1-1 )-19/4
y = (1/4)( (x^2+2x+1)-1 )-19/4
y = (1/4)( (x+1)^2-1 )-19/4
y = (1/4)(x+1)^2- 1/4 - 19/4
y = (1/4)(x+1)^2 + (-1-19)/4
y = (1/4)(x+1)^2 - 20/4
y = (1/4)(x+1)^2 - 5
The equation is in vertex form with (-1,-5) as the vertex. It's the lowest point on this parabola. Placing it into vertex form allows us to find the inverse fairly quickly.
----------
The last batch of steps is to find the inverse.
Swap x and y. Then solve for y.
y = (1/4)(x+1)^2 - 5
x = (1/4)(y+1)^2 - 5
x+5 = (1/4)(y+1)^2
(1/4)(y+1)^2 = x+5
(y+1)^2 = 4(x+5)
y+1 = sqrt(4(x+5))
y = sqrt(4(x+5)) - 1
I'll let the student check each point to confirm they are on the curve y = sqrt(4(x+5)) - 1.
You can also use a tool like GeoGebra to verify the answer.
the bottom angle is also 45 could some one answer this for me

Answers
The length of the side opposite to the reference angle is 7.77 units.
What are trigonometric ratios in terms of a right-angle triangle?We know a right-angled triangle has three sides they are -: Hypotenuse,
Opposite and Adjacent.
We can remember SOH CAH TOA which is,
sin = opposite/hypotenuse, cos = adjecen/hypotenuse and
tan = opposite/adjacent.
If we take the reference angle 45°, The side 'x' is opposite and the side having a length of 11 units is the hypotenuse.
We know, sin = opposite/hypotenuse.
Therefore,
sin45° = x/11.
1/√2 = x/11.
x = 11/√2.
x = 7.77 units.
learn more about trig ratios here :
https://brainly.com/question/14977354
#SPJ1
a number added to the difference between triple the number and thirteen
Answers
Answer:
4x-13
Step-by-step explanation:
Let the number is x.
We know that triple of a number is 3 times that number i.e. 3x
The difference between triple the number and thirteen 3x-13
Now, we need to add a number to the difference between triple the number and thirteen. So, the expression can be written as :
x+3x-13 = 4x-13
Hence, the expression is (4x-13).
Answer: 4x-13
Step-by-step explanation:
Round 93,411 to nearest tens
Answers
Step-by-step explanation:
Round 93,411 to nearest tens
93,411
93,410
sorry if wrong
3 6 9 12 15 18 21 24 27 30 is odd or even numbers?
Answers
Answer: Half of them are even and half of them are odd.
Step-by-step explanation:
The even numbers are 6, 12, 18, 24, and 30. An even number is defined as a number that is divisible by 2, meaning it has no remainder when divided by 2. For example, 6 divided by 2 equals 3 with no remainder, so 6 is even.
The odd numbers are 3, 9, 15, 21, and 27. An odd number is defined as a number that is not divisible by 2, meaning it has a remainder of 1 when divided by 2. For example, 9 divided by 2 equals 4 with a remainder of 1, so 9 is odd.
Therefore, out of the given numbers, half of them are even and half of them are odd.
________________________________________________________
PLEASE HELP QUICKLY AS POSSIBLE THANK YOU :)

Answers
Answer:
$40.52
Step-by-step explanation:
You add the amount of all the t-shirts that Mike bought and subtract it from 100.
15. Write the slope-intercept form of the equation of the line.

Answers
Answer:
D. y=1/2x+2
Step-by-step explanation:
you find your rise over run formula so could up how many squares the. over how many and I'd it is a positive slop it's a positive rise over run then you find the base which is where you start which is the second part of the equation which is 2
For the following two numbers, find two factors of the first number such that their product is the first number and their sum is the second number.
65,18
Answers
Product of 5 and 13 is 65 and sum of 5 and 13 is 18
Can I get help with this, I’m so confused

Answers
The linear and exponential equations for the amount of money in the banks indicates;
TCF Bank Equation
y = 100·x + 1000
Well Fargo Bank Equation
y = (1.06)ˣ
The number of years it will take for both accounts to have the same amount of money is about 17 years
What is an exponential equation?An exponential equation is an equation of the form; y = a·bˣ, where the input variable, x is an exponent.
The equation for calculating the amount in each bank, based on the details are;
TCF Bank;
Amount invested = $1,000
Amount added each year = 100·x
The TCF Bank Equation is; y = 100·x + 1000Well Fargo Bank Equation
The percentage of the amount earned as interest each year = 6% = 0.06
The exponential equation for compound interest amount is; y = 1000·(1 + 0.06)ˣ
Therefore; y = 1000·(1.06)ˣ
The Well Fargo Bank Equation is; y = 1000·(1.06)ˣWhen the amount in the two accounts have the same amount of money, we get;
1000·(1.06)ˣ = 100·x + 1000
Therefore; (1.06)ˣ = (100·x + 1000)/1000 = 0.1·x + 1
(1.06)ˣ = 0.1·x + 1
Solving the above equation using the substitution method and graphing indicates that we get;
x = 0, and x ≈ 17.125732
Therefore, it takes about 17 years for the two accounts to have the same amount of money
Learn more on exponential equations here: https://brainly.com/question/28595962
#SPJ1

if Susan finished her lunch at 1:00 and it took her 45 minutes to eat. what time did the class start
Answers
Answer:
12:15
Step-by-step explanation:
i dont really have an explanation
but i am in 6th grade not sure how
to explain it lol
Suppose that a safety deposit box at your bank costs $45/month to rent. How much would
it cost for you to rent the box for 15 years?
Answers
It would cost \($8,100\) to rent the safety deposit box for 15 years at a rate of \($45\) per month.
The total cost of renting a safety deposit box for 15 years, we need to first determine the total number of months in 15 years.
Since there are 12 months in a year, the total number of months in 15 years is:
15 years x 12 months/year = 180 months
So, if the safety deposit box costs \($45\) per month to rent, then the total cost of renting the box for 15 years would be:
180 months x \($45\)/month = \($8,100\)
We must first ascertain the entire number of months in 15 years in order to compute the total cost of renting a safety deposit box for 15 years.
Since there are 12 months in a year, there are a total of 180 months in 15 years: 15 years multiplied by 12 months per year.
In this case, if the monthly rental fee for the safety deposit box is \($45\) and the rental period is 15 years, the total cost would be calculated
180 months x \($45\)/month = \($8,100\)
For similar questions on safety deposit box
https://brainly.com/question/11505007
#SPJ11
need some help on this problem

Answers
The equation is 3y / 3 = 2y / y. And the value of 'y' will be 2.
The polygonal shape of a triangle has a number of sides and three independent variables. Angles in the triangle add up to 180°.
The ratio of the matching sides will remain constant if two triangles are comparable to one another.
The equation is given as,
3y / 3 = 2y / y
Simplify the equation, then we have
3y / 3 = 2y / y
3y / 3 = 2
y = 2
More about the triangle link is given below.
https://brainly.com/question/25813512
#SPJ1
Find the y-intercept, The equation of the x of symmetry and the x-coordinate of the vertex for 12.

Answers
Answer:
0, x=0, (0,0)
Step-by-step explanation:
f(x) =4x²
y-intercept is at (0,0) because there is no term with no variable
axis of symetry is x=0, because is the y-axis
x-coordinate of the vertex is 0 because the vertex is at (0,0)
Answer:
axis of symmetry-x=0
y-int-(0,0)
vertex-(0,0)
Step-by-step explanation:
Police response time to an emergency call is the difference between the time the call is first received by the dispatcher and the time a patrol car radios that it has arrived at the scene. Over a long period of time, it has been determined that the police response time has a normal distribution with a mean of 9.6 minutes and a standard deviation of 2.3 minutes. For a randomly received emergency call, find the following probabilities. (For each answer, enter a number. Round your answers to four decimal places.)
a. between 5 and 10 min
b. less than 5 min
c. more than 10 min
Answers
Answer:
a) 0.5447
b) 0.0228
c) 0.4325
Step-by-step explanation:
Normal Probability Distribution:
Problems of normal distributions can be solved using the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the z-score of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
Normal distribution with a mean of 9.6 minutes and a standard deviation of 2.3 minutes.
This means that \(\mu = 9.6, \sigma = 2.3\)
a. between 5 and 10 min
This is the p-value of Z when X = 10 subtracted by the p-value of Z when X = 5. So
X = 10
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{10 - 9.6}{2.3}\)
\(Z = 0.17\)
\(Z = 0.17\) has a p-value of 0.5675
X = 5
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{5 - 9.6}{2.3}\)
\(Z = -2\)
\(Z = -2\) has a p-value of 0.0228
0.5675 - 0.0228 = 0.5447 probability that a randomly received emergency call is between 5 and 10 minutes.
b. less than 5 min
p-value of Z when X = 5, which from item a), is 0.0228, so 0.0228 probability that a randomly received emergency call is of less than 5 minutes.
c. more than 10 min
1 subtracted by the p-value of Z when X = 10, which, from item a), is of 0.5675.
1 - 0.5675 = 0.4325
0.4325 probability that a randomly received emergency call is of more than 10 minutes.
HELP PLEASE
During a Soccer game, Erin scored 1 out of 2 goals attempted. Lauren scored 6 out of 8 goals attempted. Write the fraction the represents each and compare. Who was the most successful?
Be sure to show your work and explain your answer to get full credit.
Answers
Answer:
Erin scored 4/8 and Lauren scored 6/8. Lauren scored more.
Step-by-step explanation:
6 is a bigger number than 4
graph h(x)=(x-1)^2-9
Answers
The graph of h(x) = (x-1)^2 - 9 is a U-shaped parabola that opens upwards, with the vertex at (1, -9), and it extends indefinitely in both directions.
The function h(x) = (x-1)^2 - 9 represents a quadratic equation. Let's analyze the different components of the equation to understand the behavior of the graph.
The term (x-1)^2 represents a quadratic term. It indicates that the graph will have a parabolic shape. The coefficient in front of the quadratic term (1) implies that the parabola opens upwards.
The constant term -9 shifts the graph downward by 9 units. This means the vertex of the parabola will be at the point (1, -9).
Based on this information, we can draw the following conclusions:
The graph will be a U-shaped curve with the vertex at (1, -9).
The vertex represents the minimum point of the parabola since it opens upward.
The parabola will be symmetric with respect to the vertical line x = 1 since the coefficient of the quadratic term is positive.
The graph will extend indefinitely in both directions.
To accurately plot the graph, you can choose several x-values, substitute them into the equation to find the corresponding y-values, and then plot the points on the graph. Alternatively, you can use graphing software or calculators that can plot the graph of the equation for you.
Remember to label the axes and indicate the vertex at (1, -9) to provide a complete representation of the graph of h(x) = (x-1)^2 - 9.
for such more question on parabola
https://brainly.com/question/9201543
#SPJ8
t hits the square dartboard shown below at a random point. Find the probability that the dart lands in the shaded circular region. Each side of the dartbozn, and the radius of the shaded region Is 2 in.the value 3.14 for T. Round your answer to the nearest hundredth.

Answers
Given:
Required:
To find the probability that the dart land will be in the shaded region.
Explanation:
Area of the circle is given by the formula:
\(A=\pi r^2\)Where r = radius
Thus the area of the circular region
\(\begin{gathered} =(3.14)\times(2)^2 \\ =3.14\times4 \\ =12.56\text{ square in.} \end{gathered}\)The area of the square is given by the formula:
\(=(side)^2\)Thus the area of the given square
\(\begin{gathered} =(6)^2 \\ =36\text{ square in.} \end{gathered}\)The probability of an event is given by the formula:
\(P=\frac{number\text{ of possible outcomes}}{Total\text{ number of outcomes}}\)The probability that the dart land will be in the shaded region
\(=\frac{Area\text{ of the shaded region}}{Area\text{ of the square}}\)Thus probability
\(\begin{gathered} P=\frac{12.56}{36} \\ P=0.3488 \\ P=0.349 \end{gathered}\)Final answer:
Thus the probability that the dart land will be in the shaded region is 0.349.

At a high school, the probability that a student is a senior is 0.25. The
probability that a student plays a sport is 0.20. The probability that a student
is a senior and plays a sport is 0.08.
What is the probability that a randomly selected student plays a sport, given
that the student is a senior?
• A. 0.32
• B. 0.25
• c. 0.08
• D. 0.17
Answers
Answer:
the answer is c. 0.08
Step-by-step explanation:

Assume that each circle shown below represents one unit. Express the shaded amount as a single fraction and as a mixed number.
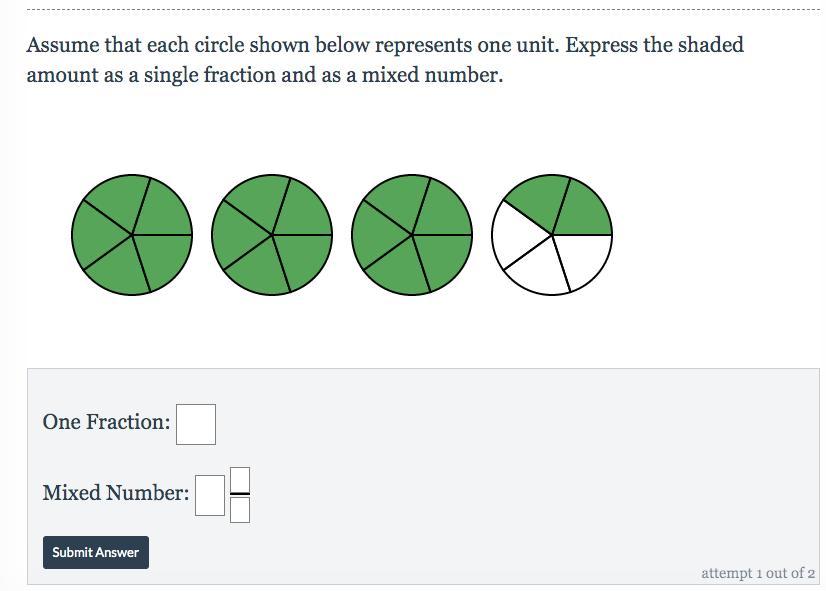
Answers
Answer:
one fraction 17/5
mixed number: 3 2/5
Step-by-step explanation:
Write the equation of the line in fully simplified slope-intercept form.

Answers
Answer:
y = 6x - 5
Step-by-step explanation:
Two points on the line are (0, -5) (this is the y-intercept) and (2, 7).
Starting at (0, -5), we move to the right to (2, 7). In doing this we see that x increases by 2 (this is the 'run') and y increases by 12 (this is the 'rise').
The slope of this line is thus m = rise/run = 12/2, or m = 6.
As we have already found, the y-intecept is (0, -5).
The slope-intercept form is y = mx + b. With m = 6 and b = -5, we have the equation
y = 6x - 5. This is the "simplified slope-intercept form."
Answer: y=6x-5
Step-by-step explanation:
first, find the slope using the formula rise/run
count the squares on the y axis from point to point
and you get 6
count the squares on x axis and you get 1
so the slope is 6/1 or 6
the formula for slope intercept form is y=mx+b where b is the y intercept and m is the slope
to find the y intercept look at the graph to see where the line touches the y axis, and you will see it is at -5
therefore y=6x-5