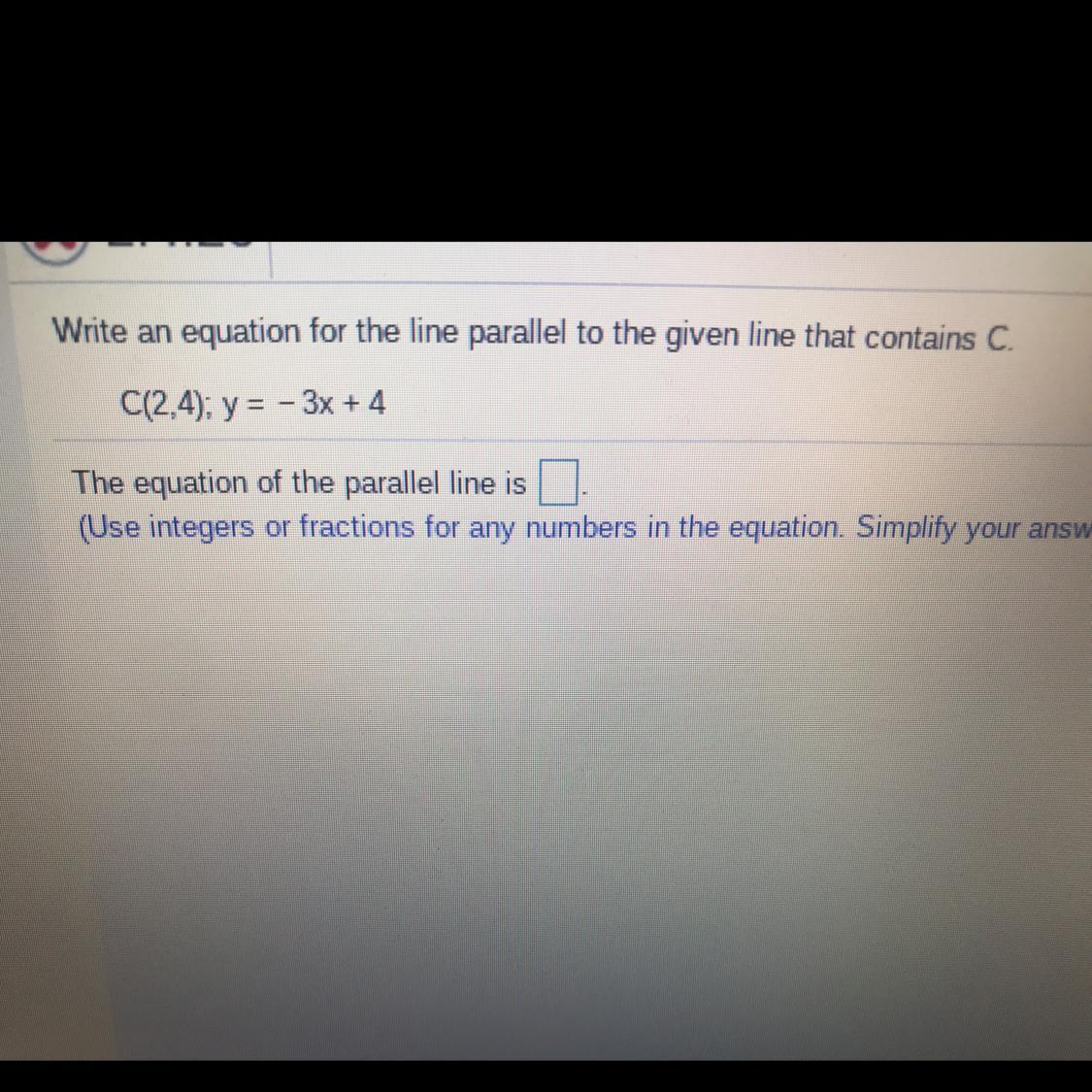
that contains C.
C(2,4); y = - 3x + 4
The equation of the parallel line is
(Use integers or fractions for any numbers in the equation. Simplify your answer.)

71

that's all there is to it

Answer: 71

Step-by-step explanation: 6.39 /0.09

71

Answer:

m∡x = 95°

m∡y = 147°

m∡z = 128°

Step-by-step explanation:

m∡z = 180-52 = 128

measure of third angle in left triangle = 180-(45+50) = 85°

m∡x = 180-85 = 95°

measure of top angle in right triangle = 180-(95+52) = 33°

m∡y = 180-33 = 147°

Based on the information given, there are a total of 118 solutions.

This is a problem of solving a Diophantine equation subject to some conditions. Let's introduce a new variable y4 = 20 - (x1 + x2 + x3 + x4). Then the problem can be restated as finding the number of solutions to:

x1 + x2 + x3 + y4 = 20

Subject to the following conditions:

0 ≤ x1 < 3

0 ≤ x2 < 4

0 ≤ x3 < 5

0 ≤ y4 < 6

We can solve this problem using the technique of generating functions. The generating function for each variable is:

(1 + x + x^2) for x1

(1 + x + x^2 + x^3) for x2

(1 + x + x^2 + x^3 + x^4) for x3

(1 + x + x^2 + x^3 + x^4 + x^5) for y4

The generating function for the equation is the product of the generating functions for each variable:

(1 + x + x^2)^3 (1 + x + x^2 + x^3 + x^4 + x^5)

We need to find the coefficient of x^20 in this generating function. We can use a computer algebra system or a spreadsheet program to expand the product and extract the coefficient. The result is: 1118

Learn more about solutions in https://brainly.com/question/30665317

#SPJ1

Answer: This problem involves finding the number of non-negative integer solutions to the equation x₁ + x₂ + x3 + x₁ = 20 subject to the given constraints. We can use the stars and bars method to solve this problem.

Suppose we have 20 stars representing the sum x₁ + x₂ + x3 + x₁. To separate these stars into four groups corresponding to x₁, x₂, x₃, and x₄, we need to place three bars. For example, if we have 20 stars and 3 bars arranged as follows:

**|**||

then the corresponding values of x₁, x₂, x₃, and x₄ are 2, 4, 6, and 8, respectively. Notice that the position of the bars determines the values of x₁, x₂, x₃, and x₄.

In general, the number of ways to place k identical objects (stars) into n distinct groups (corresponding to x₁, x₂, ..., xₙ-₁) using n-1 separators (bars) is given by the binomial coefficient (k+n-1) choose (n-1), which is denoted by C(k+n-1, n-1).

Thus, the number of non-negative integer solutions to the equation x₁ + x₂ + x3 + x₁ = 20 subject to the given constraints is:

C(20+4-1, 4-1) = C(23, 3) = 1771

However, this count includes solutions that violate the upper bounds on x₁, x₂, x₃, and x₄. To eliminate these solutions, we need to use the principle of inclusion-exclusion.

Let Aᵢ be the set of non-negative integer solutions to the equation x₁ + x₂ + x3 + x₁ = 20 subject to the given constraints, where xᵢ ≥ mᵢ for some integer mᵢ. Then, we want to find the cardinality of the set:

A = A₀ ∩ A₁ ∩ A₂ ∩ A₃

where A₀ is the set of all non-negative integer solutions to the equation x₁ + x₂ + x3 + x₁ = 20, and Aᵢ is the set of solutions that violate the upper bound on xᵢ.

To find the cardinality of A₀, we use the formula above and obtain:

C(20+4-1, 4-1) = 1771

To find the cardinality of Aᵢ, we subtract the number of solutions that violate the upper bound on xᵢ from the total count. For example, to find the cardinality of A₁, we subtract the number of solutions where x₂ ≥ 4 from the total count. To count the number of solutions where x₂ ≥ 4, we fix x₂ = 4 and then count the number of solutions to the equation x₁ + 4 + x₃ + x₄ = 20 subject to the constraints 0 ≤ x₁ < 3, 0 ≤ x₃ < 5, and 0 ≤ x₄ < 6. This count is given by:

C(20-4+3-1, 3-1) = C(18, 2) = 153

Similarly, we can find the cardinalities of A₂ and A₃ by fixing x₃ = 5 and x₄ = 6, respectively. Using the principle of inclusion-exclusion, we obtain:

|A| = |A₀| - |A

Step-by-step explanation:

Answer:

The value is  \(\sigma _x = 1.1832\)

Step-by-step explanation:

From the question we are told that

   The  population mean is \(\mu = 25\)

    The population standard deviation is  \(\sigma = 7\)

    The sample size is  n =  35

Generally the standard deviation of the sampling distribution is mathematically represented as

       \(\sigma _x = \frac{\sigma}{\sqrt{n} }\)

=>    \(\sigma _x = \frac{7}{\sqrt{35} }\)

=>    \(\sigma _x = 1.1832\)

Answer:

it has one root x=2/5

Step-by-step explanation:

Answer:

7.45

Step-by-step explanation:

The mass of the cubic cuboid is 1.479 kilograms.

Dimensionally speaking, density (ρ), in kilograms per cubic meter, is mass (m), in kilograms, per unit volume (V), in cubic meters. By the assumption of uniform density within the copper cuboid, the mass of the solid is equal to:

m = ρ · V

Where V is the volume of cuboid, in cubic meters.

And the volume of cuboid is:

V = w · h · l

Where:

Please notice that a kilogram equals 1000 grams and a meter equals 100 centimeters.

First, calculate the volume of the cuboid:

V = (0.05 m) · (0.03 m) · (0.10 m)

V = 1.5 × 10⁻⁴ m³

Second, determine the mass of the cuboid:

m = (9.86 g / cm³) · (1 kg / 1000 g) · (1000000 cm³ / m³) · (1.5 × 10⁻⁴ m³)

m = 1.479 kg

To learn more on density: https://brainly.com/question/15164682

#SPJ1

Answer:

3/65 = 0.046 = 4.6%

Step-by-step explanation:

Notice that the total number of marbles is:

6 + 14 + 8 + 12 = 40

Then , the probability of getting a black marble first is 6/40 = 3/20

Now, since the experiment is without replacing, he is left with a total of 39 marbles in the bag, and the probability of getting a clear one is now 12/39

The total probability is the product of these two:

(3/20) x (12/39) = 3/65 = 0.046 = 4.6%

Answer:

1. Option D. 15x²

2. Option C. 3

Step-by-step explanation:

1. Determination of the area of one section.

Length (L) of one section = 25x/5 = 5x

Width (W) of one section = 3x

Area (A) of one section =?

The area of one section can be obtained as follow:

Area (A) = Length (L) × Width (W)

A = L × W

A = 5x * 3x

A = 15x²

Thus, the area of one section is 15x²

2. Determination of the expressions that are equivalent to (p²)³.

We'll begin by simplifying (p²)³. This can be obtained as follow:

(p²)³ = p²*³

(p²)³ = p⁶

Next we shall compare each expression given in the question above to see which will be the same as p⁶.

p × p × p × p × p × p = p¹⁺¹⁺¹⁺¹⁺¹⁺¹

p × p × p × p × p × p = p⁶

p² × p² × p² = p²⁺²⁺²

p² × p² × p² = p⁶

p² × p³ = p²⁺³

p² × p³ = p⁵

Thus,

p² × p³ ≠ p⁶

p⁵ ≠ p⁶

p⁶ = p⁶

SUMMARY

p × p × p × p × p × p = p⁶ = (p²)³

p² × p² × p² = p⁶ = (p²)³

p⁶ = (p²)³

Therefore, 3 expressions are equivalent to (p²)³. Option C gives the correct answer to the question.

Answer:

x2+2x−15

Step-by-step explanation:

Answer:

x^2 + 2x - 15

Step-by-step explanation:

X × X + 5x - 3x - 3 × 5 =

x^2 + 5x - 3x - 3 × 5 =

X^2 + 2x - 15

also x^2 means x to the 2nd power

binomial distribution

When the production process is in control, the mean number of blue chocolate chips per bag is 6.0. The manager is interested in analyzing the probability that any particular bag being inspected has fewer than 5.0 blue chocolate chips. a) binomial distribution.

Answer: F(x) approaches - infinity

Step-by-step explanation:

Answer:

24:
6 of 4
or

8 of 3

Step-by-step explanation:

6x4=24
8x3=24

Based on the information, both ∧ (conjunction) and ∨ (disjunction) satisfy the commutative property.

It should be noted that to prove the commutativity of the logical connectives ∧ (conjunction) and ∨ (disjunction), we need to show that they satisfy the commutative property

From the truth table, both P ∧ Q and Q ∧ P have the same truth values for all combinations of truth values of P and Q. Therefore, we can conclude that P ∧ Q ≡ Q ∧ P, and ∧ (conjunction) is commutative.

In order to prove P ∨ Q ≡ Q ∨ P, we construct a truth table for both expressions. Both P ∨ Q and Q ∨ P have the same truth values for all combinations of truth values of P and Q. Therefore, we can conclude that P ∨ Q ≡ Q ∨ P, and ∨ (disjunction) is commutative.

Hence, both ∧ (conjunction) and ∨ (disjunction) satisfy the commutative property.

Learn more about commutative property. on

https://brainly.com/question/778086

#SPJ1

Answer:

Step-by-step explanation:

Looks good

Answer:

I think it is correct and also check to your teacher also

Answer:

All Sides are equal to their corresponding ones [SK = BG and so on...]

All angles are equal to thei corresponding angles [angle(T) = angle(I) and so on..]

(C.P.C.T)

Step-by-step explanation:

From the given figure, ignoring the fact that she rotated it, we can prove that the two triangles are congruent by RHS congruence criterion.

After proving so, we can use C.P.C.T to find the relations.

Hope it helps. Thank you :)

Answer:

B and D

Step-by-step explanation: I got it right

Answer:

4 feet

Step-by-step explanation:

Let the side length of the square be x feet.

x^2 - 5 = 59

x^2 = 64

x = ±4 (reject - 4 since length > 0)

Topic: Areas of polygons

If you would like to venture further, you can check out my Instagram page (learntionary) where I post notes and mathematics tips. Thanks!

a) To find the number of students getting more than 45 marks, we count the students whose marks are greater than 45 in the given list.

In the given list, the students with marks greater than 45 are: 50, 49, 47, 48, 49, 48, 47, 49, 48, 50, 47, 49, 48, 46, 47, 48, 47.

Counting these numbers, we find that there are 17 students who obtained more than 45 marks.

b) To find the number of students getting less than 45 marks, we count the students whose marks are less than 45 in the given list.

In the given list, the students with marks less than 45 are: 44, 44, 42, 41, 41, 42, 41, 44, 44, 42, 45, 45, 45, 44, 45.

Counting these numbers, we find that there are 15 students who obtained less than 45 marks.

c) To find the maximum number of students getting the same marks, we look for the mark that appears most frequently in the given list.

In the given list, the marks obtained by the students are: 44, 50, 44, 49, 42, 47, 45, 42, 44, 48, 49, 48, 47, 49, 47, 41, 45, 48, 41, 48, 41, 42, 47, 49, 49, 48, 50, 47, 49, 48, 46, 44, 45, 45, 46, 44, 42, 47, 48, 45.

Counting the frequency of each mark, we find that the marks 47 and 48 appear most frequently, with a count of 6 each. Therefore, the maximum number of students getting the same marks is 6.

d) To find the average marks obtained by the students in the class, we sum up all the marks and divide by the total number of students.

Total marks = 44 + 50 + 44 + 49 + 42 + 47 + 45 + 42 + 44 + 48 + 49 + 48 + 47 + 49 + 47 + 41 + 45 + 48 + 41 + 48 + 41 + 42 + 47 + 49 + 49 + 48 + 50 + 47 + 49 + 48 + 46 + 44 + 45 + 45 + 46 + 44 + 42 + 47 + 48 + 45

           = 1912

Total number of students = 40

Average marks = Total marks / Total number of students

                 = 1912 / 40

                 = 47.8

Therefore, the average marks obtained by the students in the class is 47.8.

e) To find the number of students getting more than the average marks, we count the students whose marks are greater than 47.8.

In the given list, the students with marks greater than 47.8 are: 50, 50, 49, 48, 49, 48, 49, 48, 50, 49, 49, 48, 48, 50, 49, 49, 48, 48, 47, 49, 49, 48, 50, 47,

49, 49, 48, 50, 47, 49, 49, 48, 48, 49, 48, 47, 48, 49, 49, 48, 50, 49.

Counting these numbers, we find that there are 40 students who obtained more than the average marks.

Part (a)

Answer: Constant of proportionality = 5/8

Reason:

The general template equation is y = kx where k is the constant of proportionality. It is the slope of the line.

The direct proportion line must pass through the origin. In other words, the y intercept must be zero.

=====================================

Part (b)

Answer: Not Proportional

Reason:

The y intercept isn't zero.

Plug x = 0 into the equation to find y = 1 is the y intercept. This graph does not pass through the origin.

Answer:

It is correct please I do not want yo sound rude can you give me brainliest answer.

Answer:

correct steps

Step-by-step explanation:

if asked to find angles in terms of the ratios, then don't forget to shift sin / cos / tan across the equal sign and change it to arc sin / cos / tan.

Answer:

FALSSOO

Step-by-step explanation:

lol srry I pretty sure its false I did teh math

Hope its right and it helps! c:

Answer:

x^2+12x=-11

Step-by-step explanation:

Answer:

(-1, 12) and (-11, 132)

Step-by-step explanation:

Answer:

0.9036

Step-by-step explanation:

Calculation to determine the probability that the proportion of Labradors

P(Proportion greater than 4%)

= P(z> 0.04 -0.05 /√0.05 * 0.95/806

= P(z > -1.30)

=0.9036

Thereforethe probability that the proportion of Labradors is =0.9036

Given,

\(h(x) = {6x}^{2} + {4x}^{4} + 7x\)

\(g( x) = {6x}^{2} - 4x + {3x}^{4} \)

Therefore,

\(h(x) + g(x)\)

\(( {6x}^{2} + {4x}^{4} + 7x) + ({6x}^{2} - 4x + {3x}^{4})\)

\( {6x}^{2} + {4x}^{4} + 7x + {6x}^{2} - 4x + {3x}^{4}\)

\((4 {x}^{4} + 3 {x}^{4} ) + ( {6x}^{2} + {6x}^{2} ) + (7x - 4x)\)

\( {7x}^{4} + {12x}^{2} + 3x\)

Answer:

Minimum=60

First Quartile(Q1)=79

Median=86

Third Quartile (Q3)=89

Interquartile range (IQR)=10

Answer: A

because slope are

Including an ACT score of 25, we are in the 78th percentile, placing us in front of 75 percent of examinees. You performed better than 93% of your classmates with a score of 30.

Percentage is commonly indicated by the percent sign, "%." The % amount has no boundaries. Percentages are essentially decimals when the denominator is 100. Use the percent sign (%) to indicate that a number is a percentage by placing it adjacent to it. For example, you score a 75% if you properly answer 75 out of 100 questions on an exam (75/100). Split the money by the total and multiply the outcome by 100 to calculate percentages. The formula for calculating the percentage is (value/total) x 100%.

With an ACT score of 25, one will be in the 78th percentile, placing you above of 75% of examinees. You performed better than 93% of your classmates with a score of 30. Besides a score between 25 and 30, which is still pretty good, you may enroll to numerous institutions.

To know more about percentage visit:

brainly.com/question/28269290

#SPJ1