The drama club has 72 members. 1/4 of the drama club members are in 5th grade and the rest of the members are in 6th grade. How many drama club members are in 6th grade?
answer choices
72
54
18
3
Answers
Answer:
54
Step-by-step explanation:
1/4 of 72 is 18. 72-18=54.
Related Questions
Refer to the diagram for Exercises 1-3.
125°
xº
zº
y°
what is the value of y z and x

Answers
Answer:
x=180-125
=55
z=55
y=180-(55+55)
y=70
Ghzhdbnhjhb. Hfuffryfygdufgh
An elephant charged 13.3 meters in 15seconds. At this rate, how far would theelephant move if it could charge for 1minute? (15 seconds = minute)
Answers
Answer:
53.2 m/min
Step-by-step explanation:
I'm not sure if (15 seconds = minute) is part of the question.
Because 15 seconds is not minute. 60 seconds is in a minute
if (15 seconds = minute) then the answer's just 13.3 m/min?? That makes no sense
so assuming 60seconds = min
13.3/15s * 60s/min would be 13.3*4 = 53.2
Other way to think about this is to say to yourself if elephant moved 13.3 meter in 15 seconds how many meter would they go in 1 minute(which would be 4x longer).
Answer:
53.2 meters
Step-by-step Explanation:
If an elephant charged 13.3 meters in 15 seconds, to calculate the distance charged by the elephant in one minute we do the following:
15 seconds by 13.3 meters and 1 minute (60 seconds) by x
Note: Why x and why set up this way? We need to figure out how far it traveled in 1 min aka 60 seconds, so if we set up what we know (16 by 13.3) we can cross multiply to figure out the ratio and get our answer :D
also written as:
\(\frac{15}{13.5} = \frac{60 [1 min]}{x}\)
(time on top and distance on bottom!)
Cross multiply:
15 * x = 13.3 * 60
15x = 798
Divide both sides by 15
5x/15 = 798/15
x = 53.2 meters
So the elephant will charge 53.2 meters for 1 minute!
Hope this helps, have a nice day! :D
(I said I couldn't do it and left it in the comments, but now it is letting me!)
From the given figure,write the corresponding angle of puv

Answers
Answer: RVN
Step-by-step explanation:
Corresponding angles are angles which occupy the same relative position at each intersection where a straight line crosses two others. If the two lines are parallel, the corresponding angles are equal.
By that - the bottom angle RVN can be an example of corresponding angles.
Please give brainliest!
Answer:
∠ RVN
Step-by-step explanation:
∠ PUV and ∠ RVN are corresponding angles.
Each is in the same position (lower left hand corner ) in its group of 4 angles.
Segment FG begins at point F(-2, 4) and ends at point G(-2, -3). The segment is translated 3 units left and 2 units up, then reflected across the y-axis to form segment F'G'.How many units long is segment F'G'?
Answers
Answer:
\(F'G' = 7\)
Step-by-step explanation:
Given
\(F = (-2,4)\)
\(G = (-2,-3)\)
Required
Distance of F'G'
The transformation that give rise to F'G' from FG are:
TranslationReflectionThe above transformations are referred to as rigid transformation, and as such the side lengths remain unchanged.
i.e.
\(F'G' = FG\)
Calculating FG, we have:
\(FG = \sqrt{(x_1 - x_2)^2 + (y_1 - y_2)^2}\)
Where:
\(F = (-2,4)\) --- \((x_1,y_1)\)
\(G = (-2,-3)\) --- \((x_2,y_2)\)
\(FG = \sqrt{(-2 - -2)^2 + (4 - -3)^2}\)
\(FG = \sqrt{(0)^2 + (7)^2}\)
\(FG = \sqrt{0 + 49}\)
\(FG = \sqrt{49}\)
Take positive square root
\(FG = 7\)
Recall that:
\(F'G' = FG\)
\(F'G' = 7\)
when an auditor uses monetary-unit sampling to examine the total value of invoices, each invoice group of answer choices has an equal probability of being selected. can be represented by no more than one monetary unit. has an unknown probability of being selected. has a probability proportional to its monetary value of being selected.
Answers
When an auditor uses monetary-unit sampling to examine the total value of invoices, each invoice has a probability proportional to its monetary value of being selected.
Monetary unit sampling (MUS) refers to a statistical sampling method utilized to determine if the account balances or monetary amounts in a population contain any misstatements in an account of transaction. Auditors use monetary unit sampling, which is also known as probability-proportional-to-size sampling or dollar-unit sampling, to establish the accuracy of financial accounts. With monetary unit sampling, each dollar in a transaction is a separate sampling unit. When an auditor uses monetary-unit sampling to examine the total value of invoices, each invoice has a probability proportional to its monetary value of being selected.
Learn more about Monetary unit sampling:
https://brainly.com/question/29440137
#SPJ4
Which expressions are equivalent choose ALL that apply.
No links please help!!
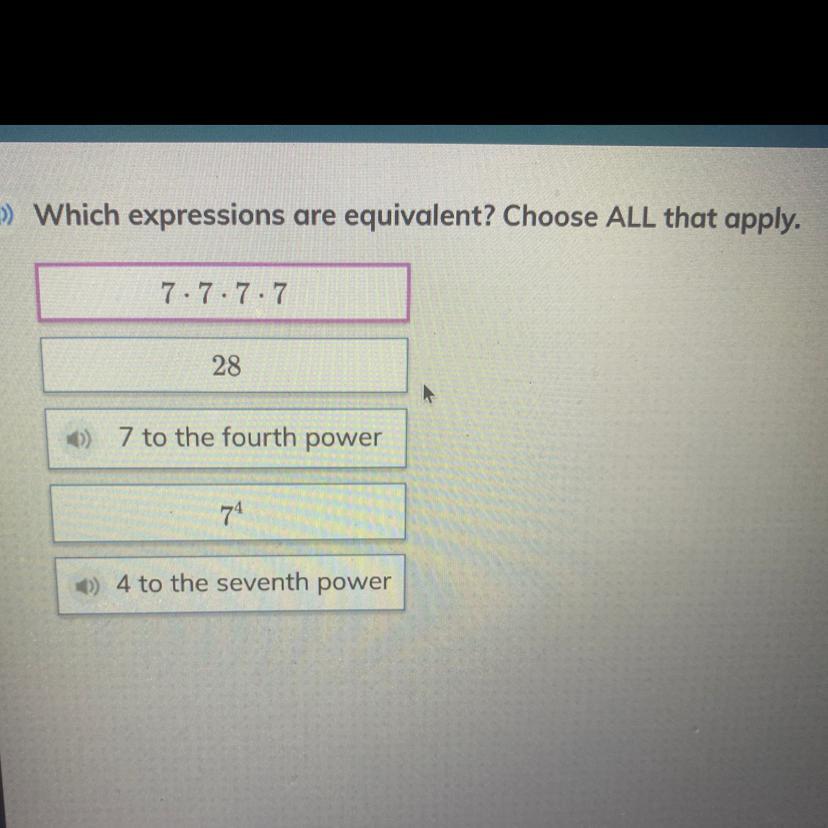
Answers
The First one, third one, and 4th one.
Value in scientific notation 48, 461, 873
Answers
Answer:...
Step-by-step explanation:
Answer:
See below
Step-by-step explanation:
4.8461873 x 10^ 7
The point P(-6, -6) is reflected over the x-axis. What are the coordinates of the resulting
point, P?

Answers
Answer:
It would be (6,-6)
Step-by-step explanation:
Hope this helps.
plete parts
a. Arrange the scores in order from least to greatest in the third trimester.

Answers
The scores from least to greatest is -8. -6, 4, 7
The absolute value of the scores from least to greatest is 4, 6, 7, 8
What is the order of the numbers?A negative number is a number that is less than zero. Negative numbers are denoted with a minus sign in front of the number. The further away from zero a negative number is, the smaller the number. Examples of negative numbers are -2, -9.
A positive number is a number that is greater than zero. The further away from zero a positive number is, the greater the number. Examples of negative numbers are 2, 9.
The scores from least to greatest is: -8. -6, 4, 7
The absolute value of a number is when the negative signs from the numbers are removed.
The absolute value of the scores from least to greatest is: 4, 6, 7, 8
To learn more about negative numbers, please check: https://brainly.com/question/258076
#SPJ1
Someone answer the whole thing fast plz

Answers
Answer:
y = 2x + 54
Step-by-step explanation:
slope of equation:
(y2-y1)/(x2-x1)
= (72-58)/(9-2)
= 14/7
= 2
y = 2x + b
58 = 2(2) + b
58 = 4 + b
b = 54
Write the slope-intercept form of the equation of the line described.

Answers
Answer:
Please check the explanation.
Step-by-step explanation:
6)
Given the line
\(y=-\frac{1}{3}x+3\)
We know that the slope-intercept form of the line equation is
\(y=mx+b\)
where m is the line and b is the y-intercept
Thus, the slope of line = -1/3
We know that parallel lines have the same slope.
Hence, the slope of the parallel line is also m=-1/3
So, substituting m = -1/3 and (3, 1) in the slope-intercept form to find the y-intercept
\(y=mx+b\)
\(1=\frac{-1}{3}\left(3\right)+b\)
Switch sides
\(\frac{-1}{3}\left(3\right)+b=1\)
\(-1+b=1\)
\(b=2\)
Thus, the equation line in slope-intercept form
\(y=mx+b\)
\(y=-\frac{1}{3}x+2\)
8)
Given the line
\(y=\frac{1}{4}x+1\)
We know that the slope-intercept form of the line equation is
\(y=mx+b\)
where m is the line and b is the y-intercept
so the slope of line = 1/4
As we know that the slope of the perpendicular line is basically the negative reciprocal of the slope of the line, so
The slope of the perpendicular line will be: -4
Thus, subtituting m = -4 and (-1, 4) in the point-slope form
\(y-y_1=m\left(x-x_1\right)\)
\(y-4=-4\left(x-\left(-1\right)\right)\)
writing the point-slope form in the slope-intercept form
\(y-4=-4\left(x+1\right)\)
add 4 to both sides
\(y-4+4=-4\left(x+1\right)+4\)
\(y=-4x\)
for my homework i need to factorise 14x^2-x-3
Answers
Answer:
the factorisation of 14x^2 - x - 3 is (7x + 3)(2x - 1).
Step-by-step explanation:
To factorise the quadratic expression 14x^2 - x - 3, we can use the following steps:
Look for two numbers that multiply to 14 and add to -1. The numbers we need are -7 and 2.Write the expression as a product of two binomials: (14x^2 - 7x) + (-x - 3).Now, we use the difference of squares factorization to separate the middle term: (7x + 3)(2x - 1).Check our answer by multiplying the two binomials together and comparing the result to the original expression.So, the factorisation of 14x^2 - x - 3 is (7x + 3)(2x - 1).
Answer:
Step-by-step explanation:
14×14-×-3
196-×-3=196-3
=193
The first two steps in determining the solution set of the system of equations, y = x2 – 6x + 12 and y = 2x – 4, algebraically are shown in the table.
Which represents the solution(s) of this system of equations?
(4, 4)
(–4, –12)
(4, 4) and (–4, 12)
(–4, 4) and (4, 12)

Answers
Answer:
(4,4)
Step-by-step explanation:
The solution set of the system of equations can be found by setting the two equations equal to each other and solving for x.
x^2 - 6x + 12 = 2x - 4
x^2 - 8x + 16 = 0
(x - 4)^2 = 0
x = 4
Since both equations in the system are equal to y, we can substitute x = 4 into either equation to find the corresponding value of y.
y = 2x - 4 = 2(4) - 4 = 4
Therefore, the solution of this system of equations is (4, 4).
Therefore, the correct answer is (4, 4).
pls help me lol i cant do this. .........

Answers
Consider the exponential function with equation A = P(1+r)t
a. Name the independent and dependent variables.
b. What is the growth factor?
Answers
The given exponential function equation is A = P(1+r)t, where P represents the principal amount, A represents the final amount, r represents the annual interest rate, and t represents the time in years.a.
The independent variable is t (time in years) while the dependent variable is A (final amount).b. The growth factor can be obtained by dividing A by P. Therefore, Growth factor = A/PLet us assume P=100, r=20%, and t=1. Then we can find A as follows:A = P(1+r)tA = 100(1+0.2)1A = 100(1.2)A = 120Therefore, the growth factor is:A/P = 120/100 = 1.2If we assume P=150, r=5%, and t=2, thenA = P(1+r)t = 150(1+0.05)2= 150(1.1025)= 165.375The growth factor is:A/P = 165.375/150 = 1.1025
Learn more about principal amount
https://brainly.com/question/30163719
#SPJ11
Jodi wants to visit her extended family in barbados next summer, and the flight costs $450. how much does she need to save each month if she wants to buy the flight in exactly 9 months?
Answers
If Jodi wants to visit her extended family in Barbados next summer, and the flight costs $450, then she needs to save 50$ each month if she wants to buy the flight in exactly 9 months.
If the flight costs $450, then the amount of money Jodi needs to save each month can be determined by using division.
Division is one of the four basic operations of arithmetic and involves the separation into equal parts.
By dividing the cost of the flight by the number of months, the money Jodi needs to save each month can be calculated.
Considering x to be money Jodi needs to save each month,
x = flight cost / number of months
x = 450 / 9
x = 50
Hence, the money Jodi needs to save each month if she wants to buy the flight in 9 months is calculated to 50$.
To learn more about division, click here:
https://brainly.com/question/28119824
#SPJ4
1 459/500 as a decimal
Answers
Answer:
1.918
Step-by-step explanation:
1 then do 459/500 x 2 to make 918/1000 then add and convert
Write the ratio of corresponding sides for the similar triangles and reduce the ratio to lowest terms.
a.
10
d.
4 5
b. 4 5
I
s 100
10
4
8
--
8 10
415
이
00
I
C. 10 85
815
I
10
I
2/5
I
211
552
415
Mark this and return
Next
Submit
Answers
The ratio of corresponding sides for the given similar triangles is 2/5.
In the given options, the ratio of corresponding sides is provided for each set of similar triangles. Let's analyze each option to determine the correct ratio:
a. 10
This option only provides a single number and does not specify the ratio of corresponding sides. Therefore, it is not the correct answer.
b. 4/5
This option provides the ratio 4/5 for the corresponding sides of the similar triangles. However, the ratio can be simplified further.
To simplify the ratio, we divide both the numerator and denominator by their greatest common divisor (GCD). In this case, the GCD of 4 and 5 is 1.
Dividing 4 and 5 by 1, we get:
4 ÷ 1 = 4
5 ÷ 1 = 5
Therefore, the simplified ratio is 4/5.
c. 10/85
This option provides the ratio 10/85 for the corresponding sides of the similar triangles. However, this ratio cannot be simplified further, as 10 and 85 do not have a common factor other than 1.
Therefore, the correct ratio of corresponding sides for the given similar triangles is 2/5, as determined in option b.
For more such questions on triangles, click on:
https://brainly.com/question/17335144
#SPJ8
Show that the characteristic equation for the complement output of a JK flip-flop is: Q(t+1) = JQ+KQ =
Answers
The complement output of a JK flip-flop is given by the Boolean expression JQ + KQ is the same as the characteristic equation for the regular output Q(t+1).
The characteristic equation for the complement output of a JK flip-flop can use the following steps:
Start with the excitation table for a JK flip-flop:
J K Q(t) Q(t+1)
0 0 0 0
0 0 1 1
0 1 0 0
0 1 1 0
1 0 0 1
1 0 1 1
1 1 0 1
1 1 1 0
The expression for the complement output Q'(t+1) in terms of J, K, Q(t), and Q'(t):
Q'(t+1) = not(Q(t+1))
= not(JQ(t) + K'Q'(t)) // since Q(t+1) = JQ(t) + K'Q'(t)
= not(JQ(t)) × not(K'Q'(t)) // De Morgan's Law
= (not(J) + Q(t)) × KQ'(t) // since not(JQ)
= not(J) + not(Q)
Simplify the expression using Boolean algebra:
Q'(t+1) = (not(J) + Q(t)) × KQ'(t)
= not(J)KQ'(t) + Q(t)KQ'(t) // Distributive Law
= J'K'Q'(t) + JKQ'(t) // De Morgan's Law
= (J'K' + JK)Q'(t)
The characteristic equation for the complement output of a JK flip-flop is:
Q'(t+1) = J'K'Q'(t) + JKQ'(t)
= JQ + KQ
For similar questions on flip-flop
https://brainly.com/question/30640426
#SPJ11
if a regular polygon has exterior angles that measure 40 each how many sides tdoes the polygon have
Answers
If you have exterior angles that are 40° each, then you can see how many times it goes into 360° to find your number of sides.
360° / 40° = 9 sides
Hope this helps!
What is the interest rate if an $85 loan for 9 months earned $4.78?
6,0
5.7
8.3
7.5
Answers
Answer:
Monthly loan payment is $400.76 for 60 payments at 7.5%. ... monthly on the current outstanding balance of your loan at 1/12 of the annual rate. 0% ... Interest rate; Number of payments, and; Amount of money you need to borrow (the principal). ... For example, if the approximate term of the loan is 4 years or 48 months
Step-by-step explanation: Monthly loan payment is $400.76 for 60 payments at 7.5%. ... monthly on the current outstanding balance of your loan at 1/12 of the annual rate. 0% ... Interest rate; Number of payments, and; Amount of money you need to borrow (the principal). ... For example, if the approximate term of the loan is 4 years or 48 months, you ...
Answer:
7.5
Step-by-step explanation:
Which of the following best describes the blue line in the image below

Answers
Answer:
C the line through point C that is perpendicular to the segment AB
Prime factor decmposition to find the highest common factor of 32 and 48
Answers
Answer:
The biggest common factor number is the GCF number. So the greatest common factor 32 and 48 is 16.
Step-by-step explanation:
Answer:
16
Step-by-step explanation
32 =2^5
48 =2^4×3
Prime factor is 2 ^4 =16
True or false: If PQ QR, with PQ = 3x-7, QR = 2x + 2, and RS = x + 9, then PS = 48.
Answers
The measure of PS is 58 not 48 showing that the statement PS = 48 is false
Line segmentsA line is a shortest distance between two points. If the measure of PQ is equivalent to QR where;
PQ = 3x - 7
QR = 2x + 2
PQ = QR
3x - 7 = 2x + 2
Collect the like term
3x - 2x = 2 + 7
x = 9
Determine RS
RS = x + 9
RS = 9 + 9
RS = 18
PS = PR + RS
PS = PQ + QR + RS
PS = 3x - 7 + 2x + 2 + 18
PS = 3(9) - 7 +. 2(9) + 20
PS = 27 - 7 + 38
PS = 20 + 38
PS = 58
Hence the measure of PS is 58 not 48 showing that the statement PS = 48 is false
Learn more on line segment here: https://brainly.com/question/17374569
#SPJ1
Jennifer bought 12 pens. Out of 12 pens, 7 are blue and the rest are red.
What is the ratio of the number of red pens to the total number of pens?

Answers
Answer:
The answer is 5:12
Step-by-step explanation:
It is 5:12 because We already know that 7 pens are blue. To find the number of red pens we must subtract 12 - 7 and get 5 as the number of red pens. Now knowing the no. of red pens and total number we can say the ratio of red pens to total no. is 5:12
due
in 30 pls help!! will rate good (:
One question in total
Let \( f(x)=x^{2 / 3}-x \), with domain \( [0,8] \). Find the absolute maximum and minimum of \( f(x) \).
Let \( f(x)=x^{2 / 3}-x \), with domain \( [0,8] \). Find the linear approximation for \( f(x
Answers
The given function is f(x)=x^{2/3}-x and the domain of the function is [0, 8].
Absolute Maximum and Minimum of the function f(x):
First, we will find the critical points of the function f(x) by finding its first derivative.
f(x) = x^(2/3) - x
Differentiating w.r.t x, we get: f'(x) = (2/3)x^(-1/3) - 1
Equate this to zero to find the critical points: (2/3)x^(-1/3) - 1 = 0(2/3)x^(-1/3) = 1x^(-1/3) = 3/2x = (3/2)^(-3) = 2
The critical point is x = 2.
Since the domain is given to be [0, 8], we need to check the values of the function at x = 0, x = 2, and x = 8.f(0) = 0^(2/3) - 0 = 0f(2) = 2^(2/3) - 2f(8) = 8^(2/3) - 8= 2.8284
Therefore, the absolute minimum of the function f(x) is 0, which occurs at x = 0, and the absolute maximum of the function f(x) is 2.8284, which occurs at x = 8.
The function f(x) is f(x)=x^{2/3}-x The domain of f(x) is [0,8] The critical point is x = 2 The absolute minimum of the function f(x) is 0, which occurs at x = 0
The absolute maximum of the function f(x) is 2.8284, which occurs at x = 8.
The absolute minimum of the function f(x) is 0, which occurs at x = 0, and the absolute maximum of the function f(x) is 2.8284, which occurs at x = 8.
The linear approximation of the function f(x) is given by the tangent of the function f(x) at the point a.
Let's assume the point a to be 2. The function f(x) is given by f(x) = x^(2/3) - x
The derivative of the function f(x) is f'(x) = (2/3)x^(-1/3) - 1
The derivative of the function f(x) at x = 2 is given by f'(2) = (2/3)2^(-1/3) - 1 = -0.0796
The equation of the tangent to the function f(x) at x = 2 is given by: y = f(a) + f'(a) * (x - a) Substituting x = 2 and a = 2 in the above equation, we get: y = f(2) + f'(2) * (x - 2)y = 2^(2/3) - 2 - 0.0796 * (x - 2)
To know more about tangent of the function visit :
https://brainly.com/question/30162652
#SPJ11
what is the closet time to midnight?
A. 11:55AM
B. 12:06AM
C. 11:50AM
D. 12:03AM
Answers
Answer:
11:55 is closest time time to mid night
Option D is correct, 12:03AM is the closet time to midnight.
Midnight is typically defined as the beginning of a new day, precisely at 12:00 AM.
In a 12-hour clock format, AM (ante meridiem) is used to represent the time before noon (from midnight to 11:59 AM), while PM (post meridiem) is used to represent the time after noon (from 12:00 PM to 11:59 PM).
12.06am is 6 minutes past midnight.
11.50am is 10 minutes from midday, or, if you prefer, 11 hours and 55 minutes past midnight.
12.03am is 3 minutes past midnight.
Hence, the closet time to midnight is 12:03 AM.
To learn more on Closet time click:
https://brainly.com/question/31732120
#SPJ2
Problem 7. A car drives North for 1 km. Then it turns and drives 2 km at 30
∘
South of West. Finally the driver turns again and drives 0.5 km Northwest. (a) What is the total distance driven? (b) What are the magnitude and direction of the car's overall displacement? Problem 8. A circular track has a radius of 500 m. What is the displacement of a bicyclist when they travel around the track from the north side to the south side? When they make one complete circle around the track? Explain your answers.
Answers
In Problem 7, the total distance driven by the car is approximately 3.17 km. The car's overall displacement has a magnitude of approximately 1.84 km and is directed at an angle of approximately 45 degrees northwest.
In Problem 8, when a bicyclist travels around a circular track from the north side to the south side, their displacement is equal to the diameter of the track, which is 1000 m. When they make one complete circle around the track, their displacement is zero since they return to their starting point.
Problem 7:
To calculate the total distance driven, we sum up the distances traveled in each direction: 1 km + 2 km + 0.5 km = 3.5 km. So, the car has driven a total distance of approximately 3.17 km.
To find the car's overall displacement, we need to consider both the magnitude and direction. The first leg of the journey going north does not contribute to the displacement in the east-west direction. The second leg, driving 2 km at 30 degrees south of west, contributes to both the east and south directions. Using trigonometry, we can calculate the eastward component as 2 km * cos(30°) ≈ 1.73 km and the southward component as 2 km * sin(30°) ≈ 1 km. The third leg, driving 0.5 km northwest, contributes to both the east and north directions. Using trigonometry again, we can calculate the eastward component as 0.5 km * cos(45°) ≈ 0.35 km and the northward component as 0.5 km * sin(45°) ≈ 0.35 km. Adding up the eastward components (1.73 km + 0.35 km) and the northward components (1 km + 0.35 km), we find that the car's overall displacement has a magnitude of approximately 1.84 km and is directed at an angle of approximately 45 degrees northwest.
Problem 8:
When the bicyclist travels from the north side to the south side of the circular track, their displacement is equal to the diameter of the track. Since the track has a radius of 500 m, the diameter is 2 * 500 m = 1000 m. Therefore, the displacement when traveling from the north side to the south side is 1000 m.
When the bicyclist makes one complete circle around the track, they return to their starting point. Displacement is a vector that represents the change in position, and in this case, the change is zero since the starting and ending points coincide. Thus, the displacement when making one complete circle around the track is zero.
Learn more about angle here:
https://brainly.com/question/30147425
#SPJ11
What is the length of the altitude?

Answers
Answer: A
Step-by-step explanation:
when you add the 2 and 6 then multiply the answer by 2 then subtract you get A as ur answer
The length of a bacterial cell is about 4 x 10−6 m, and the length of an amoeba cell is about 2.5 x 10−4 m. How many times smaller is the bacterial cell than the amoeba cell? Write the final answer in scientific notation with the correct number of significant digits.
6.25 x 102
2 x 102
6 x 101
16 x 101
Answers
the bacterial cell is 6.25*10 times smaller than the amoeba cell.
How many times smaller is the bacterial cell than the amoeba cell?
To find how many times smaller is the bacterial cell than the amoeba cell, we need to take the quotient between the amoeba cell's length and the bacterial cell's length
We know that:
bacterial cell's length = 4*10^(-6) m
amoebas cell's length. = 2.5*10^(-4) m
The quotient gives:
Q = ( 2.5*10^(-4) m)/(4*10^(-6) m) = (2.5/4)*(10^(-4)*10^6) = 0.625*10^(-4 + 6)
Q = 0.625*10^2
Now we want this in scientific notation, so we need to have a digit in the left fo the decimal point, so we can rewrite this as:
Q = 0.625*10^2 = 6.25*10^1 = 6.25*10
Then the bacterial cell is 6.25*10 times smaller than the amoeba cell.
If you want to learn more about scientific notation:
https://brainly.com/question/5756316
#SPJ1