The Massachusetts state lottery game, Cash WinFall, used to have a way that anyone with enough money and time could stand a good chance of getting rich, and it is reported that an MIT computer scientist did just that. In this game, a player picks 6 numbers from the range from 1 to 46. If he matches all 6, then he could win as much as $2 million, but the odds of that payout don't justify a bet, so let us ignore the possibility of winning this jackpot. Nevertheless, there were times when matching just 5 of the 6 numbers in a $2 lottery ticket would pay $100,000. Suppose in this scenario that you were able to bet $600,000.
(a) What is the expected amount that you would win?
(b) Derive a bound on the probability that you would lose $300,000 or more in this scenario, that is, that you would have 3 or fewer of the 5 of the 6 winning tickets.
Answers
The expected amount that you would win is approximately $0.334891, and the probability of losing $300,000 or more is approximately 0.00030986749 (or 0.030986749%).
To calculate the expected amount that you would win in this scenario, we need to consider the probabilities of various outcomes and their corresponding winnings.
(a) Expected Amount of Winnings:
Let's calculate the expected amount by considering the probabilities and winnings for different outcomes:
- Probability of matching exactly 5 out of 6 numbers:
The probability of matching 5 numbers correctly is given by the combination formula:
P(5) = C(6, 5) * C(40, 1) / C(46, 6) = 0.00000096739
The corresponding winnings are $100,000.
- Probability of matching exactly 4 out of 6 numbers:
The probability of matching 4 numbers correctly is given by the combination formula:
P(4) = C(6, 4) * C(40, 2) / C(46, 6) = 0.0000186101
The corresponding winnings are $5,000.
- Probability of matching exactly 3 out of 6 numbers:
The probability of matching 3 numbers correctly is given by the combination formula:
P(3) = C(6, 3) * C(40, 3) / C(46, 6) = 0.000290201
The corresponding winnings are $500.
Now, let's calculate the expected amount:
Expected Amount = (P(5) * $100,000) + (P(4) * $5,000) + (P(3) * $500)
Expected Amount = (0.00000096739 * $100,000) + (0.0000186101 * $5,000) + (0.000290201 * $500)
Expected Amount = $0.096739 + $0.093051 + $0.145101
Expected Amount = $0.334891
Therefore, the expected amount that you would win is approximately $0.334891.
(b) Probability of Losing $300,000 or More:
To derive a bound on the probability of losing $300,000 or more, we need to calculate the cumulative probability of having 3 or fewer of the 5 winning tickets.
Cumulative Probability = P(3) + P(4) + P(5)
Cumulative Probability = 0.000290201 + 0.0000186101 + 0.00000096739
Cumulative Probability = 0.00030986749
Therefore, the bound on the probability of losing $300,000 or more is approximately 0.00030986749, which is equivalent to 0.030986749%.
To know more about probability, refer here:
https://brainly.com/question/30034780
#SPJ4
Related Questions
If a+ 1/a = 5 then find the value of; i) a² + 1/a² ii) a³ + 1/a³
Answers
Answer:
23
110
Step-by-step explanation:
We have a + 1/a = 5
(a + 1/a)² = a² + (1/a²) + 2.a.1/a = a² + (1/a²) + 2
But (a + 1/a)² = 5² = 25
So a² + (1/a²) = 25 - 2 = 23
(a + 1/a)³ = a³ + 3a²(1/a) + 3a(1/a)² + (1/a)2
= a³ + 1/a³ + 3a + 3/a
= a³ + 1/a³ + 3(a + 1/a)
= a³ + 1/a³ + 3(5)
= a³ + 1/a³ + 15
But (a + 1/a)³ = 5³ = 125
So
a³ + 1/a³ + 15 = 125
a³ + 1/a³ = 110
Answer:
23 and 110
Step-by-step explanation:
using the expansion
(a + b)² = a² + 2ab + b² , then
a + \(\frac{1}{a}\) = 5 ( square both sides )
(a + \(\frac{1}{a}\) )² = 5²
a² + 2(a × \(\frac{1}{a}\) ) + \(\frac{1}{a^2}\) = 25
a² + 2(1) + \(\frac{1}{a^2}\) = 25
a² + 2 + \(\frac{1}{a^2}\) = 25 ( subtract 2 from both sides )
a² + \(\frac{1}{a^2}\) = 23
---------------------------------------------------------------------
using the expansion
(a + b)³ = a³ + b³ + 3ab(a + b) , then
a + \(\frac{1}{a}\) = 5 ( cube both sides )
(a + \(\frac{1}{a}\) )³ = 5³
a³ + \(\frac{1}{a^3}\) + 3(a × \(\frac{1}{a}\) )(a + \(\frac{1}{a}\) ) = 125
a³ + \(\frac{1}{a^3}\) + 3(1)(5) = 125
a³ + \(\frac{1}{a^3}\) + (3 × 5) = 125
a³ + \(\frac{1}{a^3}\) + 15 = 125 ( subtract 15 from both sides )
a³ + \(\frac{1}{a^3}\) = 110
PLEASE HELP FOR BRAINLIEST!!! Find the lengths of the dashed sides of the diagram below. Make sure your answers are fully simplified.

Answers
Answer:
BC = 4, CD = 6
Step-by-step explanation:
Simply apply the distance formula.
(4 - 0) + (4 - 4) = 4
|(6 - 4, 0 - 4)| = 2 + 4 = 6
We take the absolute value when one term is negative because distance always ignores negatives.
at one point along a straight road the direction toward mount krasha makes an angle of 33 degrees with the direction of the road. at another point 16 km farther along the road, the angle is 35 degrees. find the perpendicuar distance x of mount krasha from the road
Answers
The perpendicular distance x of Mount Krasha from the road is approximately 297.33 km.
What is trigonometry?One of the most significant areas of mathematics, trigonometry has a wide range of applications.
We can solve this problem using trigonometry. Let's draw a diagram to help us visualize the situation:
Let's let the point where the direction toward Mount Krasha makes an angle of 33 degrees with the road be point A, and let the point 16 km farther along the road where the angle is 35 degrees be point B. Let's also let the perpendicular distance from Mount Krasha to the road be x.
From the diagram, we can see that:
- The distance from point A to point B along the road is 16 km.
- The angle between the road and the perpendicular line from Mount Krasha to the road is (90 - 33) = 57 degrees at point A, and (90 - 35) = 55 degrees at point B.
Using trigonometry, we can set up two equations:
```
tan(57) = x / d (where d is the distance from the starting point to point A)
tan(55) = x / (d + 16) (where d + 16 is the distance from the starting point to point B)
```
We want to solve for x, so we can rearrange each equation to isolate x:
```
x = d * tan(57)
x = (d + 16) * tan(55)
```
Now we can set these two equations equal to each other and solve for d:
```
d * tan(57) = (d + 16) * tan(55)
d * 1.5403 = (d + 16) * 1.4281
1.5403d = 1.4281d + 22.8496
0.1122d = 22.8496
d = 203.76 km
```
Therefore, the distance from the starting point to point A is 203.76 km. We can now substitute this value into either equation for x to solve for x:
```
x = d * tan(57)
x = 203.76 km * tan(57°)
x ≈ 297.33 km
```
Therefore, the perpendicular distance x of Mount Krasha from the road is approximately 297.33 km.
Learn more about trigonometry on:
https://brainly.com/question/13729598
#SPJ4

If bolt thread length is normally distributed, what is theprobability that the thread length of a randomly selected boltis
a) Within 1.5 SDs of its mean value
b)Farther than 2.5 SDs from its mean value
c)Between 1 and 2 SDs from its mean value
Answers
Probability that the thread length of a randomly selected bolt is within 1.5 SDs of its mean value is 0.8664, is farther than 2.5 SDs from its mean value is 0.0124 and between 1 and 2 SDs from its mean value is 0.2728.
Probability that the thread length of a randomly selected bolt is within 1.5 SDs of its mean value P (μ - 1.5σ < X < μ + 1.5σ)= P(Z < 1.5) - P(Z < -1.5)Here, Z is the standard normal variable P(Z < 1.5) = 0.9332 (from standard normal table)P(Z < -1.5) = 0.0668 (from standard normal table) So, P (μ - 1.5σ < X < μ + 1.5σ) = 0.9332 - 0.0668= 0.8664
Thus, probability that the thread length of a randomly selected bolt is within 1.5 SDs of its mean value is 0.8664. Probability that the thread length of a randomly selected bolt is farther than 2.5 SDs from its mean value P (X < μ - 2.5σ) + P (X > μ + 2.5σ) = P (Z < -2.5) + P (Z > 2.5)P (Z < -2.5) = 0.0062 (from standard normal table)P (Z > 2.5) = 0.0062 (from standard normal table)
So, P (X < μ - 2.5σ) + P (X > μ + 2.5σ) = 0.0062 + 0.0062 = 0.0124 Probability that the thread length of a randomly selected bolt is between 1 and 2 SDs from its mean value P (μ - 2σ < X < μ - 1σ) = P (Z < -1) - P (Z < -2) + P (Z < 1) - P (Z < 2)P (Z < -1) = 0.1587 (from standard normal table)
P (Z < -2) = 0.0228 (from standard normal table)P (Z < 1) = 0.8413 (from standard normal table)P (Z < 2) = 0.9772 (from standard normal table) So, P (μ - 2σ < X < μ - 1σ) = 0.1587 - 0.0228 + 0.9772 - 0.8413= 0.2728
To know more about normal distribution, refer here:
https://brainly.com/question/29509087#
#SPJ11
hELP
Which equation below would have a y-intercept at (0,7) and open downward? Question 1 options: y = 7x2+16x+26 f(x) =−x2−7x−11 f(x)=−3x2+16x+7 y =7x2+7x−7
Answers
Select the correct answer. A figure shows a pyramid of height 3 h and a rectangular prism of height h. This pyramid has the same base as the prism, and its height is three times the height of the prism. What is the ratio of the volume of the pyramid to the volume of the prism? A. volume of pyramid volume of prism = 1 B. volume of pyramid volume of prism = 1 9 C. volume of pyramid volume of prism = 3 D. volume of pyramid volume of prism = 2 3 Reset Next
Answers
Answer:
Step-by-step explanation:
The ratio of the volume of the pyramid to the volume of the prism is 1.so, the option A. is correct.
Let the base of the base of a prism is b and the height of the prism is h.
so, the volume of the prism-
V= \(b^{2}\) h
also, given that base of the given pyramid is also b and the height of the pyramid is three times the height of the prism.
h'=3h
so, the volume of the square pyramid-
V'=\(\frac{1}{3}\)\(b^{2}\)h'
V'=\(\frac{1}{3}\)\(b^{2}\)(3h)
V'=\(b^{2}\)h
so, now-
\(\frac{V'}{V}\)=\(\frac{b^{2}h }{b^{2}h }\)
\(\frac{V'}{V}\)= 1
so. the ratio of the volume of the pyramid to the volume of the prism is 1.
Use the distributive property to write an equivalent expression. 6(3y+6)
Answers
Answer:
18y + 36
Step-by-step explanation:
6 x 3y = 18y
6 x 6 = 36
18y = 36
18y/18 = 36/18
y = 2 this is the final answer
what is the length x of a side of the small inner square?
Answers
The length x of a side of the small inner square can be determined using the properties of similar triangles.
To find the length x, we can set up a proportion between the small inner square and the larger outer square.
Let's denote the side length of the small inner square as s and the side length of the larger outer square as S.
Since the small inner square is completely contained within the larger outer square, the ratio of their side lengths will be the same as the ratio of their corresponding sides.
Therefore, we can set up the following proportion:
s / S = x / (x + 10)
Here, the x + 10 represents the side length of the larger outer square, as it is 10 units longer than the side length of the small inner square.
To solve for x, we can cross-multiply the proportion:
s * (x + 10) = x * S
Expanding the equation:
sx + 10s = xS
Rearranging the equation to isolate x:
sx - xS = -10s
Factoring out the common term x:
x(s - S) = -10s
Dividing both sides by (s - S):
x = -10s / (s - S)
Now, we have an expression for x in terms of s and S.
It's important to note that the given information is insufficient to find the exact value of x without additional measurements or equations. The value of x will depend on the specific dimensions of the small inner square and the larger outer square.
to know more about small inner square here:
brainly.com/question/30517179
#SPJ11
3(6-14)
Evaluate
0 -15
o 6
0 -1
06
Answers
Answer:
It's -15 hope this helped!!
If you can Awnser ur a god and my best friend

Answers
IS the square root of 16 rational or irrational and why?
Answers
Answer: It is rational because the square root of 16 is 4 which rational.
Would appreciate brainly <3
Answer:
Rational
Step-by-step explanation:
Because square root of 16 is a whole number, 4.
Find the Least Squares Solution to the following system of linear equations: [1 point] Find the Least Squares Solution to the following system of linear equations: [ x-y-z =-2; -x =4; -2 x+3 y+4 z =-1; -x+2 y+2 z =1 ]
Answers
The least squares solution to the given system of linear equations is x* = [3/14; 1/7; 1/14].
In order to find the least squares solution to the given system of linear equations, we can use the following steps:
Step 1: Write the system of equations in matrix form. In other words, we write the coefficients of the variables as a matrix, and the variables themselves as another matrix.
The matrix form of the given system of equations is given by:
Ax = b, where
A = [1 -1 -1; -1 0 0; -2 3 4; -1 2 2],
x = [x; y; z], and
b = [-2; 4; -1; 1].
Step 2: Compute the transpose of matrix A, denoted by \(A^T\) .
\(A^T\) is given by:
\(A^T\) = [1 -1 -2 -1; -1 0 3 2; -1 0 4 2].
Step 3: Compute the product of \(A^T\) and A, denoted by \(A^T\) A.
\(A^T\) A is given by:
\(A^T\) A = [7 -8 -8; -8 14 18; -8 18 21].
Step 4: Compute the inverse of \(A^T\) A, denoted by (\(A^T\) A)⁻¹.
(\(A^T\) A)⁻¹ is given by: ( \(A^T\) A)⁻¹ = [9/28 1/7 5/28; 1/7 4/7 -2/7; 5/28 -2/7 9/28].
Step 5: Compute the product of \(A^T\) and b, denoted by \(A^T\) b.
\(A^T\) b is given by: \(A^T\) b = [-1; 6; 5].
Step 6: Compute the least squares solution, denoted by x*. x* is given by: x* = (\(A^T\) A⁻¹ \(A^T\)
b. Substituting the values from steps 4 and 5 into this equation, we get: x* = [3/14; 1/7; 1/14].
Therefore, the least squares solution to the given system of linear equations is x* = [3/14; 1/7; 1/14].
Know more about the least squares solution
https://brainly.com/question/23305357
#SPJ11
What is the difference quotient for the function f (x) = negative startfraction 1 over 5 x minus 12 endfraction?
Answers
The difference quotient of f(x) is \(\frac{-\frac{1}{h}+ 5}{5x+ h -12}\) .
According to the given question.
We have a function
f(x) = -1/(5x -12)
As we know that, the difference quotient is a measure of the average rate of change of the function over and interval.
The difference quotient formula of the function y = f(x) is
[f(x + h) - f(x)]/h
Where,
f(x + h) is obtained by replacing x by x + h in f(x)
f(x) is a actual function.
Therefore, the difference quotient formual for the given function f(x)
= [f(x + h) - f(x)]/h
= \(\frac{\frac{-1}{5(x+h)-12} -\frac{-1}{5x-12} }{h}\)
= \(\frac{\frac{-1}{5x + 5h -12}+\frac{1}{5x-12} }{h}\)
= \(\frac{\frac{-1+5h}{5x + 5h-12} }{h}\)
= \(\frac{-1+5h}{(5x +h-12)(h)}\)
= \(\frac{-1+5h}{5xh + h^{2} -12h}\)
= \(\frac{h(-\frac{1}{h}+5) }{h(5x+h-12)}\)
= \(\frac{-\frac{1}{h}+ 5}{5x+ h -12}\)
Hence, the difference quotient of f(x) is \(\frac{-\frac{1}{h}+ 5}{5x+ h -12}\) .
Find out more information about difference quotient here:
https://brainly.com/question/18270597
#SPJ4
suppose you lift a laptop that weighs 4.1 pounds off the floor onto a shelf that is 2 feet high. how much work have you done?
Answers
The required work done in lifting the laptop to a height of 2 feet will be 263.79 foot-poundal.
Total weight of the laptop is 4.1 pounds
Acceleration due to gravity = 32.17 ft/\(s^{2}\)
Now the height to which the laptop has been lifted = 2 feet
Since, there is no mentioned velocity of the laptop, therefore the kinetic energy will be zero
Therefore, the total work in lifting the laptop will be equal to the change in potential energy.
Potential energy is the energy that comes by virtue of its position with reference to other
Therefore, potential energy = mgh
Where, m = mass of the laptop = 4.1 pounds
g = acceleration due to gravity = 32.17 ft/\(s^{2}\)
h = height to which the laptop is lifted = 2 feet
Therefore potential energy = (4.1)(32.17)(2) = 263.79 foot-poundal
263.79 foot-poundal is the required work done.
To know more about the potential energy, visit here:
https://brainly.com/question/8664733
#SPJ4
am i the only one that's confused? i don't get this and neither does my older sister! please tell me if u get it! :0

Answers
Answer:
I believe the answer is C. I'd have to work this out on paper to be certain, but from what i worked out mentally, i got C. lol
Step-by-step explanation:
Answer:
C
Step-by-step explanation:
It explains all ways he can learn for each topic
Gt= Graph and text
Gv= Graph and vid
(same pattern with the rest)
and at the end
T/V= both text and video
find the value of trigonometric ratio
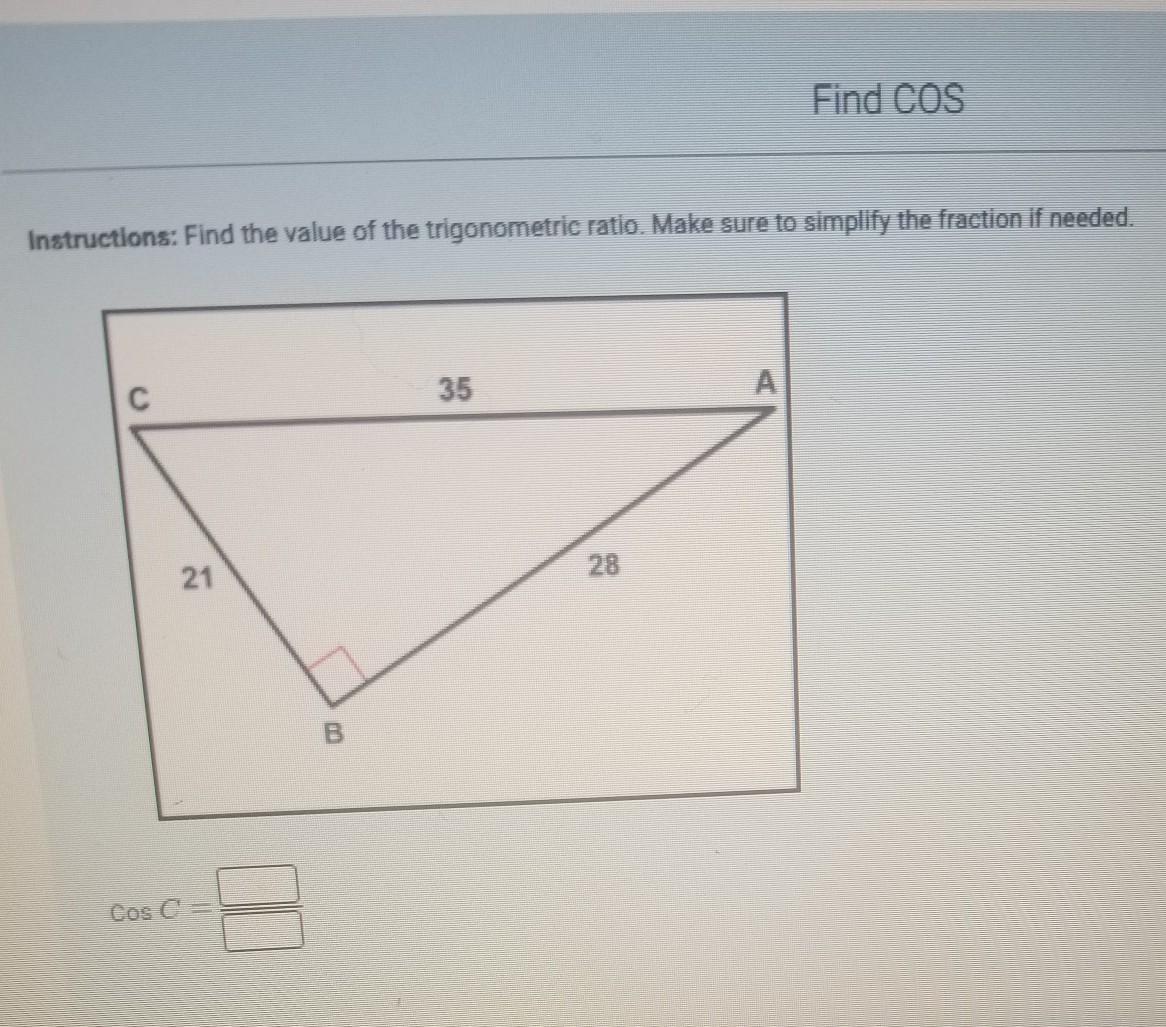
Answers
Answer:
3/5
Step-by-step explanation:
Cos C = adjacent/hypotenuse
or, Cos C = 21/35 = 3/4
Answered by GAUTHMATH
Let A = {−5, −4, −3, −2, −1, 0, 1, 2, 3} and define a relation R on A as follows: For all (m, n) is in A, m R n ⇔ 5|(m2 − n2). It is a fact that R is an equivalence relation on A. Use set-roster notation to list the distinct equivalence classes of R. (Enter your answer as a comma-separated list of sets.)
Answers
The distinct equivalence classes of R are: [{-5, 0, 5}, {-4, 1, 2, 3}, {-1, 1, 4}]
How to calculate the distinct equivalence classes of RTo find the distinct equivalence classes of R, we will determine the elements in A that are related to each other based on the relation defined (5|(m² - n²)).
We can do this by examining each element of A and seeing which elements satisfy this relation.
For -5:
{(-5, -5), (-5, 0), (-5, 5)}
For -4:
{(-4, -4), (-4, 1), (-4, 2), (-4, 3)}
For -3:
{(-3, -3), (-3, 2), (-3, 3)}
For -2:
{(-2, -2), (-2, 3), (-2, 1)}
For -1:
{(-1, -1), (-1, 4), (-1, 1)}
For 0:
{(0, 0), (0, 5)}
For 1:
{(1, 1), (1, 4), (1, -1)}
For 2:
{(2, 2), (2, 3), (2, -3)}
For 3:
{(3, 3), (3, 2), (3, -2)}
Now we can group the related elements together to form the distinct equivalence classes:
[{-5, 0, 5}, {-4, 1, 2, 3}, {-3, 2, 3}, {-2, 1, 3}, {-1, 1, 4}, {0, 5}, {1, -1, 4}, {2, -3, 3}, {3, -2, 2}]
We can see that some of the classes are overlapping or identical, so we can remove the duplicates and combine the overlapping classes:
[{-5, 0, 5}, {-4, 1, 2, 3}, {-1, 1, 4}]
Learn more about equivalence class at
https://brainly.com/question/14307463
#SPJ11
If AB is parallel to CD and the slope of CD is -8, what is the slope of AB?
О
A.
1
8
OB. 8
O C. -8
O D. 1/3
Answers
Answer:
If AB is parallel to CD and the slope of CD is -8, what is the slope of AB?
✘ О A. \(\frac{1}{8}\)
✘ O B. 8
✔ O C. -8
✘ O D. 1/3
Have a Nice Day . if Try Do This \(\frac{x}{y}\) Look For This symbol \(\sqrt{x}\)
i Have Look For Right Answer or i Guest it .
Kenny is walking at a constant speed of 3.5 miles. How far can she walk in 6 hours? Write an equation to find the total distance after the....
Answers
Answer:
The equation formed: 3.5 x 6 = x
Kenny's speed: 3.5m/h
Distance covered by her in 6 hours: 3.5x6 =
18.0
Please help! I will mark as brainliest IF answer is right. <3

Answers
Answer:7x - 2
Step-by-step explanation:

The base of a solid is a circular disk with radius of 6. Find the volume of the solid if parallel cross sections perpendicular to the x-axis are squares. Set-up but do not evaluate the integral.
Answers
The integral to find the volume V is then:
V = ∫[0 to L] 144 dx
What is volume of the solid?
The volume of a solid is a measure of how much area of space an object occupies. It is measured by the number of unit cubes needed to fill the body. It is decided by counting the unit cubes in the solid.
To find the volume of a solid, we need to consider parallel cross-sections perpendicular to the x-axis. Since these cross-sections are square, each square cross-section will have the same area throughout the body.
Consider a representative square cross-section at a given x-coordinate. The length of each side of this square will be equal to the diameter of the corresponding circular disc at this x-coordinate. Since the radius of a circular disk is given as 6, the diameter will be twice the radius, which is 12.
Therefore, the area of a square cross-section at any coordinate is x (12)^2 = 144 square units.
To find the volume of a body, we must integrate the areas of all these square cross-sections over the range of values of x that the body occupies. Since no specific range is mentioned in the information provided, I will assume that the body extends from x = 0 to x = L, where L is the length of the body along the x-axis.
The integral to find the volume V is then:
V = ∫[0 to L] 144 dx
Note: dx represents an infinitesimal change in x.
Note that this integral is just a setup and the actual evaluation of the integral is not required in the question.
To learn more about volume of solid from the given link
https://brainly.com/question/29246646
#SPJ4
The last question!!!!!!!!!!!!!!

Answers
Answer:
800
Step-by-step explanation:
if you look at the graph and go where t=2 you see that it will have 800 bacteria at two hours
Answer:
800
Step-by-step explanation:
What is the difference between repressed and recovered memories?
Answers
Recovered memory in CSA refers to the new development of abusive memories. Repressed memory is when the patient believes the CSA occurred, even though they have no specific memory of the event.
Recovered memory:
The term "recovered memory" implies that at some point the memory must become inaccessible to conscious awareness (as opposed to "continuous memory"). Although the term is not ideal, it is clear that people often fail to report important events, such as known hospitalizations (Loftus, 1993). In 1995, the debate over reclaiming memory was near its most violent climax. Hundreds of people have recovered memories of child sexual abuse (CSA), and sometimes in therapy it is thought that repressed or dissociative memories need to be recovered for a person to 'heal'.
Repressed Memory:
Repressed memory is a purported psychiatric phenomenon involving the inability to recall autobiographical information, usually traumatic or stressful. The concept has its origins in psychoanalytic theory, where repression is understood as a defense mechanism that keeps painful experiences and unacceptable impulses out of awareness. Repressed memory is a controversial concept, especially in a legal context, where it is used to unjustly and inaccurately accuse individuals, causing significant harm. Meanwhile, a working group from the American Psychological Association has stated that while "most people who were sexually abused in childhood remember some or all of what happened to them, it is possible to remember long-forgotten abuses.
Learn more about Even:
https://brainly.com/question/2289438
#SPJ4
Look at this diagram:
a) What fraction is shaded?
b) What percentage is shaded?
Diagram ⬇️

Answers
Answer:
fraction 3/9
percentage is 90%
There are 60 seconds in a minute and 60 minutes in an hour. How many seconds are in one hour?
Answers
Answer:
3,600 seconds
Step-by-step explanation:
multipy how many hours by 3,600
(so 1 times 3,600)
Answer:
3,600 seconds
Step-by-step explanation:
\(\frac{60 seconds}{1 minute} (\frac{60 minutes}{1 hour} )=\frac{3,600 seconds}{1 hour}\)
Simplify the following expression:
(66)4

Answers
Step-by-step explanation:
Given
\(( {6}^{6})^{4} \\ = {6}^{6 \times 4} \\ = {6}^{24} \\ = 4.7383813e18\)
HELP PLZ PLZ PLZZZZ I WILL MARK BRAINLYLIESF!!!!!!

Answers
Answer:
150.8
Step-by-step explanation:
The formula for soving cylinder is V=Bh or V=πr2hmso if you plug that in you should get this answer like I did.
What's the solution: 4 (6x - 4) = 8 (3x - 2)
Answers
Answer: Simplifying
4(6x + -4) = 8(3x + -2)
Reorder the terms:
4(-4 + 6x) = 8(3x + -2)
(-4 * 4 + 6x * 4) = 8(3x + -2)
(-16 + 24x) = 8(3x + -2)
Reorder the terms:
-16 + 24x = 8(-2 + 3x)
-16 + 24x = (-2 * 8 + 3x * 8)
-16 + 24x = (-16 + 24x)
Add '16' to each side of the equation.
-16 + 16 + 24x = -16 + 16 + 24x
Combine like terms: -16 + 16 = 0
0 + 24x = -16 + 16 + 24x
24x = -16 + 16 + 24x
Combine like terms: -16 + 16 = 0
24x = 0 + 24x
24x = 24x
Add '-24x' to each side of the equation.
24x + -24x = 24x + -24x
Combine like terms: 24x + -24x = 0
0 = 24x + -24x
Combine like terms: 24x + -24x = 0
0 = 0
Solving
0 = 0
Step-by-step explanation: oof lol bye
x = 2/3 = 0.667
thats your answer
if a single die is rolled, what is the probability of a 2 or odd?
Answers
Solution:
The dice have 6 faces, and it is possible to get a 2 only once, so the possibility of getting a 2 is 1/6.There are three possible outcomes of getting an odd number: 1, 3, or 5.
Therefore, the probability of getting an odd number is 3/6, which reduces to 1/2.
The possibility of getting a 2 or an odd number is the probability of getting a 2 plus the probability of getting an odd number minus the probability of getting a 2 and an odd number (since 2 is even).
P(2 or odd) = P(2) + P(odd) - P(2 and odd)
Since 2 is not an odd number, getting a 2 and an odd number simultaneously is not feasible.
Therefore, the equation is:P(2 or odd) = 1/6 + 1/2 - 0= 4/6= 2/3Answer: Therefore, the probability of getting a 2 or odd is 2/3.
To know more about probability visit :
https://brainly.com/question/31828911
#SPJ11
Emir's living room is 5 yards wide and 8 yards long. He wants to install blue
carpet that costs $4.00 per square yard. How much will it cost to buy enough
carpet for the living room?
Answers
Answer:
$160.00
Step-by-step explanation: