The mileage (in 1,000s of miles) that car owners get with a certain kind of radial tire is a random variable having an exponential distribution with a mean of 50.
a. What is the probability that a tire will last at most 40,000 miles? (Round intermediate calculations to at least 4 decimal places and final answer to 4 decimal places.)
b. What is the probability that a tire will last at least 65,000 miles? (Round intermediate calculations to at least 4 decimal places and final answer to 4 decimal places.)
c. What is the probability that a tire will last between 70,000 and 80,000 miles? (Round intermediate calculations to at least 4 decimal places and final answer to 4 decimal places.)
Answers
a) The probability that a tire will last at most 40,000 miles is approximately 0.3297
b) The probability that a tire will last at least 65,000 miles is approximately 0.0656
c)The probability that a tire will last between 70,000 and 80,000 miles is approximately 0.0505...
a. We are given that the mileage that car owners get with a certain kind of radial tire is a random variable having an exponential distribution with a mean of 50. Therefore, the rate parameter λ can be found as follows:
λ = 1/mean = 1/50 = 0.02
Now, we need to find the probability that a tire will last at most 40,000 miles. This can be calculated using the cumulative distribution function (CDF) of the exponential distribution:
P(X ≤ 40) = 1 - e^(-λx) = 1 - e^(-0.02*40) ≈ 0.3297
Therefore, the probability that a tire will last at most 40,000 miles is approximately 0.3297.
b. To find the probability that a tire will last at least 65,000 miles, we can use the same exponential distribution with rate parameter λ = 0.02:
P(X ≥ 65) = e^(-λx) = e^(-0.02*65) ≈ 0.0656
Therefore, the probability that a tire will last at least 65,000 miles is approximately 0.0656.
c. Finally, we need to find the probability that a tire will last between 70,000 and 80,000 miles. This can be calculated using the CDF of the exponential distribution as follows:
P(70 ≤ X ≤ 80) = P(X ≤ 80) - P(X ≤ 70)
= (1 - e^(-λ80)) - (1 - e^(-λ70))
= e^(-0.0270) - e^(-0.0280) ≈ 0.0505
Therefore, the probability that a tire will last between 70,000 and 80,000 miles is approximately 0.0505.
Click the below link, to learn more about probability:
https://brainly.com/question/30034780
#SPJ11
Related Questions
the fourth term in the geometric sequence in part (a) appears as the $n$th term in the arithmetic sequence. find the value of $n$.
Answers
The value of n is 30.33. The thirty-fourth term can be found by solving for the common difference (d) and the number of terms (n) in the arithmetic sequence and then using the formula for the nth term of an arithmetic sequence.
Let's assume that the common ratio of the geometric sequence is r. Then, the terms of the geometric sequence are given by:
\(a_2 = a_1 * ra_{10} = a_1 * r^8a_{34} = a_1 * r^{32}\)
Since these terms also appear in the arithmetic sequence, we can write:
\(a_2 = a_1 + da_{10} = a_1 + 9da_{34} = a_1 + 33d\)
Substituting the expressions for a_2 and a_{10} from the geometric sequence into the arithmetic sequence, we have:
\(a_1 * r = a_1 + da_1 * r^8 = a_1 + 9d\)
Solving for d, we get:
\(d = a_1 * (r - 1)d = a_1 * (r^8 - 1) / 9\)
Next, we use the expression for a_{34} from the arithmetic sequence and substitute the expression for d to obtain:
\(a_{34} = a_1 + 33d = a_1 + 33a_1 * (r^8 - 1) / 9\)
Since the first term of the arithmetic sequence is 1, we have:
\(a_{34} = 1 + 33 * 1 * (r^8 - 1) / 9\)
The value of n can be found by using the formula for the nth term of an arithmetic sequence:
\(a_n = a_1 + (n-1)d\)
Setting n = 34 and substituting the expression for d, we have:
\(a_{34} = 1 + (33) * (a_1 * (r^8 - 1) / 9)\)
So the thirty-fourth term of the arithmetic sequence is:
\(a_{34} = 1 + 33 * (1 * (r^8 - 1) / 9) = 34 - 33/9.\)
Learn more about geometric sequence here:
https://brainly.com/question/1509142
#SPJ4
Complete Question:
An arithmetic sequence with first term 1 and common difference not equal to 0 has second, tenth, and thirty-fourth terms that form a geometric sequence.
What is the thirty-fourth term of the arithmetic sequence?
The fourth term in the geometric sequence above appears as the nth term in the arithmetic sequence. Find the value of n.
Can the triangles be proven similar and how
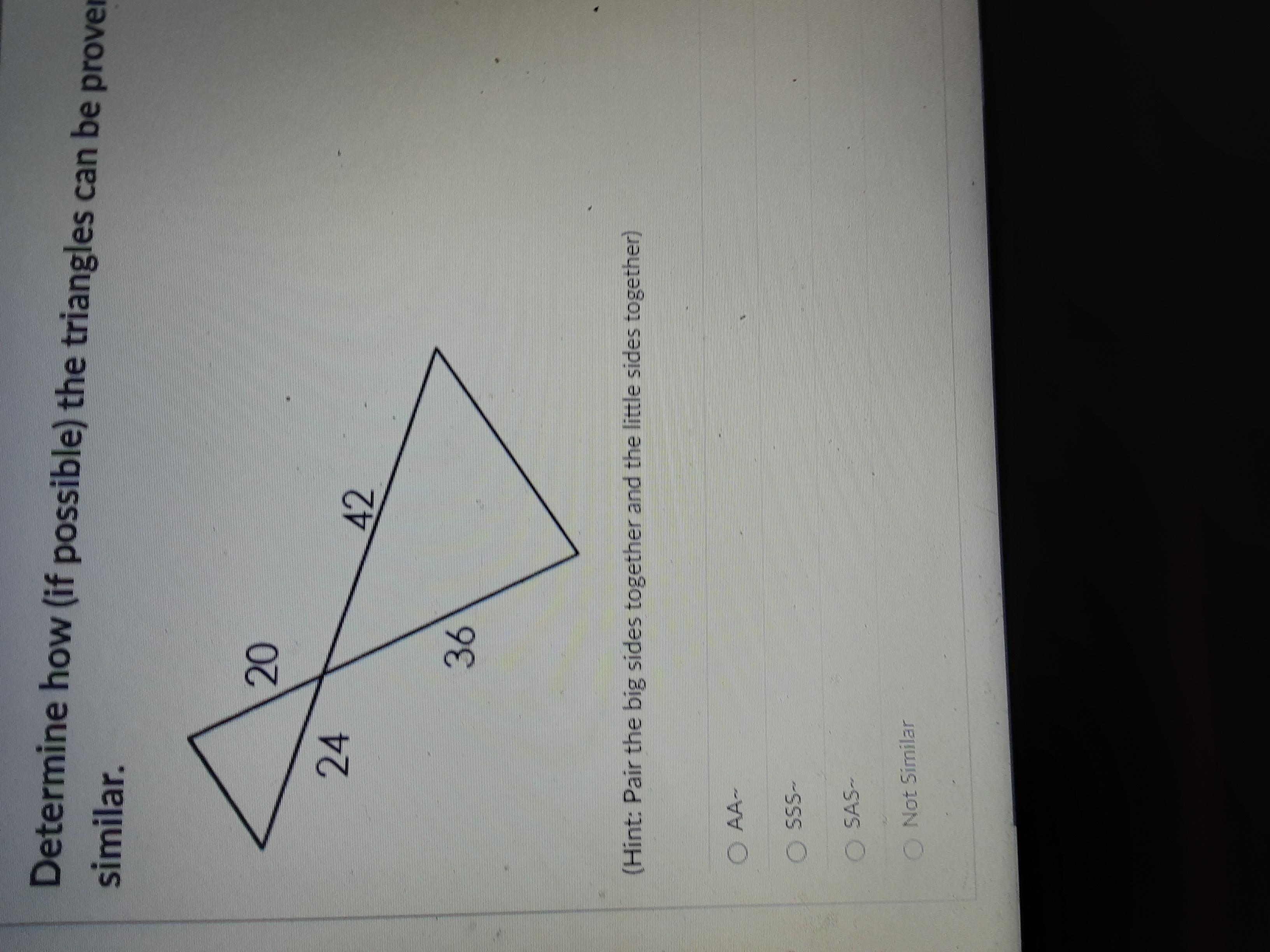
Answers
Answer:
yes
Step-by-step explanation:
please help me answer this question asap

Answers
Answer:
It's quite easy
Step-by-step explanation:
people less than 30 years = frequency of people 0 to 15 + 15 to 30 = 8+15 =23
Therefore there are 23 people less than 30 years old.
pls mark me as brainliest pls.
The measure of <E , the angle of elevation from point A to point B, is (3x+1)". The measure of <D.
the angle of depression from point B to pointA, is 2(x+8). Find the measure of each angle.
m<E = 22⁰, m<D = 30°
m<E = 15⁰, m<D=15°
m<E = 46°, m<D = 46°
m<E = 52⁰, m<D= 50°
Answers
Answer:
last answer choice
Step-by-step explanation:
Use the sum of cubes identity to write this polynomial expression in factored form: 8x^3 + 27
Answers
(2x + 3) (x^2 - 6x + 9)
What are the four conditions necessary for X to have a Binomial Distribution? Mark all that apply.
a. There are n set trials.
b. The trials must be independent.
c. Continue sampling until you get a success.
d. There can only be two outcomes, a success and a failure
e. You must have at least 10 successes and 10 failures
f. The population must be at least 10x larger than the sample. T
g. he probability of success, p, is constant from trial to trial
Answers
Options a, b, d, and g are the correct conditions for a Binomial Distribution.
The four conditions necessary for X to have a Binomial Distribution are:
a. There are n set trials: In a binomial distribution, the number of trials, denoted as "n," must be predetermined and fixed. Each trial is independent and represents a discrete event.
b. The trials must be independent: The outcomes of each trial must be independent of each other. This means that the outcome of one trial does not influence or affect the outcome of any other trial. The independence assumption ensures that the probability of success remains constant across all trials.
d. There can only be two outcomes, a success and a failure: In a binomial distribution, each trial can have only two possible outcomes. These outcomes are typically labeled as "success" and "failure," although they can represent any two mutually exclusive events. The probability of success is denoted as "p," and the probability of failure is denoted as "q," where q = 1 - p.
g. The probability of success, p, is constant from trial to trial: In a binomial distribution, the probability of success (p) remains constant throughout all trials. This means that the likelihood of the desired outcome occurring remains the same for each trial. The constant probability ensures consistency in the distribution.
The remaining options, c, e, and f, are not conditions necessary for a binomial distribution. Option c, "Continue sampling until you get a success," suggests a different type of distribution where the number of trials is not predetermined. Options e and f, "You must have at least 10 successes and 10 failures" and "The population must be at least 10x larger than the sample," are not specific conditions for a binomial distribution. The number of successes or failures and the size of the population relative to the sample size are not inherent requirements for a binomial distribution.
Learn more about Binomial Distribution at: brainly.com/question/29137961
#SPJ11
Can you write a number using 20 digits after the decimal point. Explain your thinking.
Answers

Given the function defined in the table below, find the average rate of change, in
simplest form, of the function over the interval 5 ≤ x ≤9.
7
8
9
6
x
4
5
f(x)
4
8
16
32
64
medically explained PAINL X
128
Deltal/ath
Answers
The average rate of change, in simplest form, is -2/5.
What is average rate ?
Divide the change in y-values by the change in x-values to determine the average rate of change. Identifying changes in quantifiable parameters like average speed or average velocity calls for the knowledge of the average rate of change.
The rate of change of the function is its gradient or slope.
The formula for calculating the gradient of a function is expressed as:
\(m=\frac{d y}{d x}=\frac{y_2-y_1}{x_2-x_1}$$\)
Using the coordinate points from the table (0,41) and (15,35)
Substitute the coordinate into the expression:
\($$\begin{aligned}& m=\frac{35-41}{15-0} \\& m=\frac{-6}{15} \\& m=\frac{-2}{5}\end{aligned}$$\)
To learn more about average rate visit:https://brainly.com/question/3605446
#SPJ1
Consider a situation that you might need to use your understanding of probability to make an informed decision. What sorts of information would you collect
Answers
To make an informed decision using probability, collect relevant background information, data/observations, assumptions/constraints, sprobabilistic model, expert opinions, and external sources.
Probabilistic models: Depending on the complexity of the problem, I might develop or utilize probabilistic models. These models help to quantify uncertainties and estimate the likelihood of various outcomes. This could involve using statistical techniques, simulation methods, or probabilistic algorithms.
Expert sprobabilistic model: If available, I would seek expert opinions or consult with individuals who have domain-specific knowledge or expertise. Their insights can provide valuable perspectives and help refine the probabilistic assessment.
External sources: I would consider relevant external sources such as published studies, reports, or industry benchmarks. These sources can provide additional information or benchmarks against which the probabilities and outcomes can be evaluated.
To know more about sprobabilistic model,
https://brainly.com/question/31444919
#SPJ11
Find perimeter and area

Answers
Answer:
well if it's 8 on bottom that means it has to be 8 on top and the right side add these together and you have perimeter
If Patrick scored 33 marks out of a possible 55 marks, what % did he get?
Answers
Answer:
60%
Step-by-step explanation:
33/55 = 0.6 which is 60%
Triangle ABC is translated 6 units to the right and 1 units up.
What are the coordinates of C’?
C’(4, –2)
C'(4, –0)
C'(4, 2)
C'(1, 2)
Answers
The coordinate of the point C from the given triangle ABC after translation is C' = (4, -2)
Translation of coordinatesA triangle is a 2D figure with 3 sides and angles. Given the coordinate C points of the triangle;
C = (-2, -3)
If these coordinate point is translated 6 units to the right and 1 units up, the resulting coordinate will be at:
C' = (-2 + 6, -3 + 1)
C' = (4, -2)
Hence the coordinate of the point C from the given triangle ABC after translation is C' = (4, -2)
Learn more on translation here; https://brainly.com/question/12861087
#SPJ1

Answer: the answer is A C'(4, -2)
Step-by-step explanation:
Which of the expressions below would help you to calculate the percent
increase from Value 1 to Value 2?
Answers
Answer:
D. (Value 2 - Value 1) ÷ Value 1Step-by-step explanation:
Percent increase is the percent of the increased value over the initial value.
In our case the increase is represented by the difference of values and the initial value is Value 1.
So the matching choice is D.
=====================================
Answer choices in the comment:
A. Value 2 ÷ Value 1B. Value 1 ÷ Value 2C. (Value 2 - Value 1) ÷ Value 2D. (Value 2 - Value 1) ÷ Value 1The mapping diagram represents a relation where x represents the independent variable and y represents the
dependent variable.
x-values
-4
-2
0
3
y-values
-5
-4
-3
-2
-1
Is the relation a function? Explain.
O No, because for each input there is not exactly one output
O No, because for each output there is not exactly one input
O Yes, because for each input there is exactly one output
O Yes, because for each output there is exactly one input
Answers
Answer: Option C is correct, Yes, because for each input there is exactly one outputWhat is a function?A relation is a function if it has only One y-value for each x-value.To determine whether the relation is a function or not, we need to check whether each input in the domain (the x values) is paired with exactly one output in the range (the y values).The input value of -3 is paired with the output value of 0The input value of -1 is paired with the output value of 2The input value of 1 is paired with the output value of 0The input value of 3 is paired with the output value of 2The input value of 5 is paired with the output value of 5Since each input in the domain is paired with exactly one output in the range, the relation is a function.Therefore, the correct answer is: Yes, because for each input there is exactly one output.
Step-by-step explanation:
Find the area of the trapezoid a21 b22.50 c11.25 d42

Answers
(1 ÷ 2 3 ⁄ 4 ) + (1 ÷ 3 1 ⁄ 2 ) = _____.
Answers
Answer:
50/77
Step-by-step explanation:
(1÷2 3/4)+(1÷3 1/2)
2 3/4 is same as 11/44
1/2 is same as 7/2
so to divide fraction you have to flip the second number and multiply
so 1 times 4/11=4/11
and 1 times 2/7=2/7
4/11 +2/7=28/77+22/77=50/77
hey can someone pls help me asap?!

Answers
Hope this helps
Angle A and angle B are supplementary. Angle A is vertical to a 75 degree angle. What is the measure of angle B ?
Answers
The measure of angle B is 75 degrees.
What are supplementary angles?
Supplementary angles are those angles that sum up to 180 degrees. For example, angle 130° and angle 50° are supplementary angles because sum of 130° and 50°
Vertical angles are congruent, so Angle B = 75 degrees.
Supplementary angles add up to 180 degrees so Angle A is 105 degrees.
Two angles are supplementary if the sum of their measures is 180 degrees.
If angle A is vertical to a 75 degree angle, then the measure of angle A must be 105 degrees.
Learn more about supplementary angles at:https://brainly.com/question/12919120
#SPJ1
The sum of the first 10 terms of a linear sequence is 5 and the sumof the next 10 terms is 215. Find the sequence.
Answers
Answer:
The first term of the sequence is -8.95 with a common difference of 2.1.
The sequence is:
-8.95, -6.85, -4.75, -2.65...
Step-by-step explanation:
We are given that the sum of the first ten terms of a linear sequence is 5, and the sum of the next ten terms is 215.
And we want to find the sequence.
Recall that the sum of an A.P. is given by:
S = k / 2 ( a + xk)
Where a is the first term, k is the number of terms, and x_k is the last term.
We can represent our term values with the direct formula:
Xn = a + d (n-1)
Where the first term, x₁ = a.
The sum of the first ten terms is 5. Hence, S = 5, k = 10, a = x₁ and x_k = x₁₀:
(5) = 10/2 (a+xk)
5 = 5(a+(a+9d))
2a + 9d = 1
The sum of the next ten terms (that is, from x₁₁ to x₂₀) is 215. Hence, S = 215 when k = 10, a = x₁₁, and x_k = x₂₀:
(215) = (10)/2 (a + xk)
215 = 5((a+d(11-1))+(a+d(20-1)))
2a + 29d = 43
This yields a system of equations:
\(\left \{ {{2a+9d=1} \atop {2a+29d=43}} \right.\)
Solve:
(2a + 9d) + (-2a - 29d) = (1) + (-43)
-20d = -42
d = 2.1
Consequently:
a = 1/2(1-9d)
= 1/2(1-9(2.1))
= -8.95
In conclusion, the first term of the sequence is -8.95 with a common difference of 2.1.
The sequence is:
-8.95, -6.85, -4.75, -2.65...
Please help
No links

Answers
Answer:
same thing as adding 3/5 +3/5
total number of parts in a rectangle is 5
the product is 6/5 or 1 1/5
Using only the values given in the table for the function, f(x), what is the interval of x-values over which the function is increasing?
Answers
The interval of x-values over which the function is increasing is (-2, 1).
We have,
To determine the interval of x-values over which the function is increasing, we need to look for where the function values are increasing as the input values increase.
In other words,
We need to find where the slope of the function is positive.
Using the table,
We can see that the function values are decreasing over the intervals
(-6, -5), (-5, -4), (-4, -3), (-3, -2), (-2, -1), (0, 1).
Therefore, the function is not increasing over any of these intervals.
However,
Between x = -2 and x = 1, the function values are increasing.
Therefore,
The interval of x-values over which the function is increasing is (-2, 1).
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ1

What scale factor takes hexagon J to hexagon K?
Answers
Answer:
Step-by-step explanation:
boody boody
What are the types of moment of inertia units?
Answers
The units of moment of inertia depend on the shape and dimensions of the object, and can be expressed in kg m², g cm², lb ft², or oz in².
The units of the moment of inertia depend on the shape and dimensions of the object. Some common units for a moment of inertia are:
kg m² (kilogram-meter squared)
g cm² (gram-centimeter squared)
lb ft² (pound-foot squared)
oz in² (ounce-inch squared)
These units represent the distribution of mass around an axis of rotation and are used in calculations involving rotational motion and torque.
The moment of inertia is a physical property of an object that determines its resistance to rotational motion. It depends on the mass distribution of the object and its orientation with respect to the axis of rotation. The moment of inertia is typically represented by the symbol I and is measured in units of kg m², g cm², lb ft², or oz in². The moment of inertia can be calculated using the object's mass, dimensions, and shape, and is used in calculations involving rotational motion, such as torque and angular acceleration. Different shapes and distributions of mass have different moments of inertia, which can affect the object's motion and stability.
Learn more about inertia:
https://brainly.com/question/3268780
#SPJ4
Rewrite, using the distributive
property.
16b-8b = ([?]-8)b = [?]b
![Rewrite, using the distributiveproperty.16b-8b = ([?]-8)b = [?]b](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/9Tmzv11947B9UtnbF6DBvWJp63YQcon7.png)
Answers
Answer:
8b
Step-by-step explanation:
You can factor the b-term out since b-term exists for all terms in the expression. By factoring out, you are basically dividing the factored term off and put it outside of the bracket, thus:
\(\displaystyle{16b-8b=\left(16-8\right)b}\)
Then evaluate and simplify:
\(\displaystyle{\left(16-8\right)b=8\cdot b}\\\\\displaystyle{=8b}\)
m/3 = 4
solve m.
Please show your work
Answers
1/3m=4 /x3
The answer is m=12
Is this true or false? Ill give brainliest

Answers
Answer:
It is true
Step-by-step explanation:
Clayton is at most 2 meters above sea level. Using C for Clayton, which inequality correctly represents this statement?
Answers
Answer:
C ≤ 2
Step-by-step explanation:
Given that :
Clayton is at most 2 meters above sea level ;
Let Clayton = C
Clayton's distance can be represented by the inequality :
2 meters above sea level is positive, +2
At most 2 meters above sea level means, Clayton could be anywhere between + 2 metwrs but not more than 2 meters
Hence, Clayton's distance can be represented by the inequality :
C ≤ 2
Consider the standard parametrization of the LDS model, with a new latent transition that depends on an observed sequence of inputs y1:T in the form:
zt+1= Azt + Byt + wt
where matrix B is an additional model parameter and yt is the observed input vector at time t. How do
the Kalman filtering and smoothing updates change for this variation?
Answers
The Kalman filtering and smoothing updates for the variation of the LDS model with an observed input sequence (yt) include the introduction of the matrix B, which relates the observed inputs to the latent transition.
For the standard parametrization of the Linear Dynamical System (LDS) model, the Kalman filtering and smoothing updates involve estimating the hidden states and their uncertainties given the observed inputs. In the variation you mentioned, where there is a new latent transition that depends on the observed sequence of inputs (yt), the Kalman filtering and smoothing updates need to be modified to account for this additional dependency.
In the Kalman filtering step, which is the prediction-update process, the estimates of the hidden states (zt) and their uncertainties are updated sequentially as new observations become available. In the standard LDS model, the filtering equations involve the state transition matrix (A) and the measurement matrix (C), which relate the current state to the previous state and the observation. In the modified model, we introduce an additional matrix (B) that relates the observed input vector (yt) to the latent transition.
The Kalman filtering equations for this variation would be as follows:
Prediction step:
zt+1|t = Azt|t + Byt
Pt+1|t = A Pt|t AT + Q
Update step:
Kt+1 = Pt+1|t BT (BPt+1|t BT + R)^-1
zt+1|t+1 = zt+1|t + Kt+1(yt+1 - Bzt+1|t)
Pt+1|t+1 = (I - Kt+1B)Pt+1|t
Here, B is the matrix that relates the observed input vector (yt) to the latent transition, and R is the observation noise covariance matrix. The rest of the variables (A, Q) have the same interpretation as in the standard LDS model.
Similarly, for the Kalman smoothing step, which involves estimating the hidden states based on all the available observations, the equations need to be modified accordingly to incorporate the new latent transition. The modified Kalman smoothing equations would involve the same matrices (A, B, C) and additional computations to update the estimates and uncertainties.
In summary, the Kalman filtering and smoothing updates for the variation of the LDS model with an observed input sequence (yt) include the introduction of the matrix B, which relates the observed inputs to the latent transition. The filtering equations are adjusted to incorporate this new dependency, and the smoothing equations would involve similar modifications to estimate the hidden states based on all available observations.
Learn more about variation here
https://brainly.com/question/13998680
#SPJ11
How many distinct triangles can be formed for which m∠E = 64°, g = 9, and e = 10?
triangle(s)
How many distinct triangles can be formed for which m∠J = 129°, k = 8, and j = 3?
triangle(s)
Answers
Answer:
10Step-by-step explanation:
1.For two side measures and one angle measure to form one distinct triangle, the given angle must be between the given sides, or opposite the longest side.
When the given measures are ...
E = 64°g = 9e = 10The given angle is opposite the longest side, so one distinct triangle can be formed.
2.No triangle can be formed if the given angle is opposite the shorter given side, and either of ...
the angle is right or obtusethe ratio of shorter to longer sides is less than the sine of the given angle.__
Additional comment
As we can see from the above, the only ambiguous cases arise when the given angle is opposite the shorter given side. When that happens, the ratio of the shorter to longer given side relative to the sine of the given angle determines the nature of the solution:
two distinct triangles if the ratio is greater than the sineone right triangle if the ratio is equal to the sine.Answer:
1
0
Step-by-step explanation:

Find the surface area of a tetrahedron whose vertices are at the points a( 1, 2, -1 ) , b( 2, 0, 1 ) , c( -1, 1, 2 ) and d( 3, 2, 4 ).
Answers
The surface area of the tetrahedron with vertices A(1, 2, -1), B(2, 0, 1), C(-1, 1, 2), and D(3, 2, 4) is approximately 7.71 square units.
To find the surface area of a tetrahedron, we can use the formula:
Surface area = 1/2 * base * height
First, we need to find the base of the tetrahedron. We can do this by finding the lengths of the sides AB, AC, and BC.
Using the distance formula, we find that the lengths of these sides are:
AB ≈ 2.82 units
AC ≈ 4.36 units
BC ≈ 3.74 units
Next, we need to find the height of the tetrahedron. We can do this by finding the distance from point D to the plane formed by points A, B, and C.
Using the formula for the distance between a point and a plane, we find that the distance is approximately 2.45 units.
Finally, we can calculate the surface area using the formula mentioned earlier:
Surface area ≈ 1/2 * (2.82 + 4.36 + 3.74) * 2.45 ≈ 7.71 square units.
Therefore, the surface area of the tetrahedron is approximately 7.71 square units.
Know more about tetrahedron here:
https://brainly.com/question/11946461
#SPJ11