The regular price of a baseball hat is $16.85. If Ernesto buys the baseball hat on sale for 20% off the regular price, how much change will
receive after paying with $20?
Answers
Answer:
$6.52
Step-by-step explanation:
The price of the hat would be $13.48 after the discount.
So the amount of change he would receive would be 20-13.48 which is $6.52
Related Questions
Addison was given a gift card for a coffee shop. Each morning, Addison uses the card to buy one cup of coffee. Each cup of coffee costs $3 and after buying 4 cups of coffee, the card had a $3 remaining balance. Write an equation for, A, in terms of ,x, representing the amount money remaining on the card after buying x cups of coffee.
Answers
Answer:
Step-by-step explanation:
after buying x cups of coffee, we will have x/4 times remaining on the card, and every time remaining $3, so
A=3(x/4)
solve the inequality h/3 >8
which means h divided by 3 less than 8
PLS HELP
THANKS
Answers
Answer:
h>24
Step-by-step explanation:
multiply both sides by 3 to get ride of the denominator:
h>24
Whats the 100 digits of pie?\
im only asking because brainly keeps telling me to ask a question
Answers
Answer:
3.14159265358979323846264338327950288419716939937510
58209749445923078164062862089986280348253421170679
Step-by-step explanation:
Answer:
3.1415926535 8979323846 2643383279 5028841971 6939937510 5820974944 5923078164 0628620899 8628034825 3421170679
Step-by-step explanation:
i don't know how to solve it
A company is manufacturing an open-top rectangular box. They have 30 cm by 16 cm sheets of material. The bins are made by cutting squares the same size from each corner of a sheet, bending up the sides, and sealing the corners. Create an equation relating the volume V of the box to the length of the corner cut out x. Identify the dimensions of the box that will have the maximum volume.
Answers
Answer:
a. (30 - 2x)(16 - 2x)x. b. length 21.2 cm , width = 7.2 cm and height x = 4.4 cm
Step-by-step explanation:
a. Let x be the side of the squares to be cut from each corner. Since we have two corners on each side, the length of the resulting box from the 30 cm by 16 cm sheet is L = 30 - 2x. Its breadth is B = 16 - 2x. The height of the resulting box is x. So its volume V = LBx = (30 - 2x)(16 - 2x)x.
b. To find the maximum value of V, we differentiate V with respect to x and equate it to zero.
So, dV/dx = 0
d(30 - 2x)(16 - 2x)x/dx = 0
-2(16 - 2x)x + (-2)(30 - 2x)x + (30 - 2x)(16 - 2x) = 0
32x + 4x² - 60x - 4x² + 480 - 60x - 32x + 4x² = 0
4x²- 120x + 450 = 0
x²- 30x + 112.5 = 0
Using the quadratic formula, we find x. So, with a = 1, b = - 15 and c = 112.5,
\(x = \frac{-(-30)+/-\sqrt{(-30)^{2} - 4 X 1 X 112.5} }{2 X 1} \\= \frac{30+/-\sqrt{900 - 450} }{2}\\ = \frac{30+/-\sqrt{450} }{2}\\ = \frac{30+/-21.21 }{2}\\\\ = \frac{30+21.21 }{2} or \frac{30-21.21 }{2}\\ = \frac{51.21 }{2} or \frac{8.79}{2}\\ = 25.605 or 4.395\)
x ≅ 25.61 or 4.4
V = (30 - 2x)(16 - 2x)x
Substituting the values of x into L and B, we have )x
L = (30 - 2x) = (30 - 2(25.61)) = 30 - 51.22 = -21.22
B = (16 - 2x) = (16 - 2(25.61)) = 16 - 51.22 = -35.22
Since L and B cannot be negative, we use the other value for x = 4.4, So
L = (30 - 2x) = (30 - 2(4.4)) = 30 - 8.8 = 21.2
B = (16 - 2x) = (16 - 2(4.4)) = 16 - 8.8 = 7.2
So V = LBx = 21.2 × 7.2 × 4.4 = 671.62 cm³
The dimensions that maximize the volume of the box are 23.34 by 9.34 by 3.33
The dimensions of the material is:
\(\mathbf{Length = 30}\)
\(\mathbf{Width = 16}\)
Assume the cut-out is x.
So, the dimensions of the box are:
\(\mathbf{Length = 30 - 2x}\)
\(\mathbf{Width = 16 -2x}\)
\(\mathbf{Height =x}\)
The volume is calculated as:
\(\mathbf{V = Length \times Width \times Height}\)
So, we have:
\(\mathbf{V = (30 - 2x) \times (16 - 2x) \times x}\)
Expand
\(\mathbf{V = 480x - 92x^2 +4x^3}\\\)
Differentiate
\(\mathbf{V' = 480 - 184x +12x^2}\)
Set to 0
\(\mathbf{480 - 184x +12x^2 = 0}\)
Using a calculator, we have:
\(\mathbf{x = 12, x =3.33 }\)
12 is greater than the dimensions of the box.
So, we have:
\(\mathbf{x =3.33 }\)
Substitute 3.33 for x in
\(\mathbf{Length = 30 - 2x}\)
\(\mathbf{Width = 16 -2x}\)
\(\mathbf{Height =x}\)
So, we have:
\(\mathbf{Length = 23.34}\)
\(\mathbf{Width = 9.34}\)
\(\mathbf{Height = 3.33}\)
Hence, the dimensions that maximize the volume of the box are 23.34 by 9.34 by 3.33
Read more about volumes at:
https://brainly.com/question/11599887
Surveying 200 likely voters from each group of libertarians, independents, democrats, and republicans is an example of?
Answers
Surveying 200 likely voters from each group of libertarians, independents, Democrats, and Republicans is an example of stratified sampling.
The survey conducted, which involves sampling 200 likely voters from each group of libertarians, independents, Democrats, and Republicans, exemplifies stratified sampling. Stratified sampling is a sampling technique where the population is divided into distinct subgroups or strata based on certain characteristics, and then samples are selected from each subgroup in proportion to their representation in the population.
In this case, the four political groups serve as the strata, and by selecting an equal number of likely voters from each group, the survey aims to ensure that each group is adequately represented in the sample. Stratified sampling allows for more accurate analysis and conclusions by considering the characteristics of each subgroup separately, enhancing the overall validity and reliability of the survey results.
To know more about stratified,
https://brainly.com/question/30876035#
#SPJ11
Curves to parametric equations Finc parametric ecuations for the following curves. Include an interval for the parameter values. Answers are not unlcue. 42. The line segment starting at P(-1, -3) and ending at Q(6, – 16) 53–56. Circular motion Find parametric equations that describe the circular path of the following objects. For Exercises 53-55, assume (x, y) denotes the position of the object relative to the origin at the center of the circle. Use the units of time specified in the problem. There are many ways to describe any circle 54. The tip of the 15-inch second hand of a clock completes one revolution in 60 seconds.
Answers
For the line segment starting at P(-1, -3) and ending at Q(6, -16), we can use the parameter t to describe the movement from P to Q. The x and y coordinates can be expressed as:
x = -1 + 7t
y = -3 - 13t
where t varies from 0 to 1 (to cover the entire line segment).
53. For an object moving in a circular path, we can use the parameter t to describe the angle of rotation around the circle. The radius of the circle (denoted by r) can be found by the distance from the origin to the object's position.
54. For the tip of the 15-inch second hand of a clock completing one revolution in 60 seconds, we can assume the circle has a radius of 15 inches. Since the hand completes one revolution in 60 seconds, it travels a full circle in 60 seconds or 2π radians.
Therefore, the parametric equations for the circular motion are:
x = 15cos(t)
y = 15sin(t)
where t varies from 0 to 2π (to cover one complete revolution).
To learn more about “coordinates” refer to the https://brainly.com/question/11337174
#SPJ11
The small number is a 3 please help

Answers
What is the solution to this system of equations?
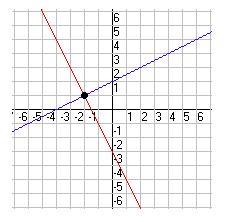
Answers
Answer:
(-2,1)
Step-by-step explanation:
the blank is a aprt of the streen
A submarine dove from the surface at a rate of 50 meters per minute. It is now at a depth of 450 meters. Write a numeric equation using negatives to show the number of minutes it took to get to the current depth.
Answers
Explanation
Step1
let
\(\begin{gathered} \text{rate}=-50\frac{mt}{\text{sec}} \\ \text{rate}=\frac{dis\tan ce}{\text{time}} \end{gathered}\)\(\begin{gathered} y_1=initial\text{ position=0} \\ y_2=\text{ final positivion=-50} \\ \text{time}=\text{ 1 minute} \end{gathered}\)Step 2
equation
\(\begin{gathered} time=\text{ }\frac{\text{distance}}{-50}\text{ Equation(1)} \\ \\ \text{replacing} \\ \text{time}=\frac{-450\text{ mt}}{-50\text{ }\frac{mt}{\min}} \\ \text{time}=9\text{ minutes} \end{gathered}\)I hope this helps you

compute four-week and five-week moving averages for the time series. do not round intermediate calculations. if required, round your answers to two decimal places.
Answers
To compute the four-week and five-week moving averages for a time series, you would first add up the values for each consecutive four or five weeks, depending on which moving average you are calculating. Then, you would divide the total by four or five, respectively, to find the average for that time period. You would then repeat this process for each subsequent set of four or five weeks, depending on which moving average you are calculating.
For example, to calculate the four-week moving average for a time series with values of 10, 12, 14, 16, 18, 20, 22, and 24, you would add up the values for each consecutive set of four weeks:
Weeks 1-4: 10 + 12 + 14 + 16 = 52
Weeks 2-5: 12 + 14 + 16 + 18 = 60
Weeks 3-6: 14 + 16 + 18 + 20 = 68
Weeks 4-7: 16 + 18 + 20 + 22 = 76
Weeks 5-8: 18 + 20 + 22 + 24 = 84
Then, you would divide each total by four to find the four-week moving average for that time period:
Weeks 1-4: 52 / 4 = 13
Weeks 2-5: 60 / 4 = 15
Weeks 3-6: 68 / 4 = 17
Weeks 4-7: 76 / 4 = 19
Weeks 5-8: 84 / 4 = 21
To calculate the five-week moving average, you would follow the same process but with consecutive sets of five weeks instead of four.
Note that intermediate calculations should not be rounded, but the final answers should be rounded to two decimal places, as specified in the question.
Learn more about average here:
https://brainly.com/question/24057012
#SPJ11
compare new france and the 13 colonies in the terms of population, government and economy
Answers
Answer:
5 or
Step-by-step explanation:
Amber invests $7,000 in bonds and stocks.
• The bonds, b, yield 4% interest annually
• The stocks, s, yield 5% interest annually.
• She earns $320 in income from her investments in a year.
Which of these statements are TRUE about Amber's investments? Select all that apply.
Answers
The true statements are 0.04b + 0.05s = 320, b + s = 7000, and Amber invests $3,000 in bonds and $4,000 in stocks. Then the correct options are A, C, and F.
What is simple interest?Simple interest is the concept that is used in many companies such as banking, finance, automobile, and so on.
The formula for interest is written as,
I = (PRT)/100
Where P is the principal, R is the rate of interest, and T is the time.
Amber invests $7,000 in bonds and stocks.
The bonds, b, yield 4% interest annually.The stocks, s, yield 5% interest annually.She earns $320 in income from her investments in a year.Then the equation is given as,
b × 0.04 + s × 0.05 = $320
0.04b + 0.05s = 320 ...1
b + s = 7000 ...2
From equations 1 and 2, then we have
0.04(7000 - s) + 0.05s = 320
0.01s = 40
s = 4000
Then the value of 'b' is given as,
4000 + b = 7000
b = 3000
The true statements are 0.04b + 0.05s = 320, b + s = 7000, and Amber invests $3,000 in bonds and $4,000 in stocks. Then the correct options are A, C, and F.
More about the simple interest link is given below.
https://brainly.com/question/2793278
#SPJ1
The complete question is attached below.

Question 7 of 10
Evaluate the expression and enter your answer below.
V64
v4

Answers
Answer:
4
Step-by-step explanation:
the answer is 4
I hope this is the answer
Answer:
4
Step-by-step explanation:
\( \\ \sf \frac{ \sqrt{64} }{ \sqrt{4} } \)
\( \\ \sf = \frac{ \sqrt{8 \times 8} }{ \sqrt{2 \times 2} } \)
\( \\ \sf = \frac{8}{2} \)
\( \\ \sf = 4\)
every morning, laura practices shooting basketball free throws. she doesn't shoot a fixed amount, instead she keeps shooting free throws until she makes 12. suppose laura is an 82% free throw shooter. let x be how many free throws laura will miss tomorrow morning. then x has a negative binomial distribution. what is a trial? [ select ] what is a success? [ select ] how many successes are required? r
Answers
The probability of Laura missing 3 free throws before making 12 is approximately 0.0037 or 0.37%.
The basketball free throw.
A success would be considered when Laura makes a free throw.
A failure would be when Laura misses a free throw.
The negative binomial distribution is a probability distribution that calculates the probability of obtaining a certain number of failures before a specified number of successes occur.
The specified number of successes is 12, and the number of failures is represented by the variable x.
To calculate the probability of x failures, we need to know the probability of a single failure.
Since Laura is an 82% free throw shooter, the probability of her missing a free throw is 18%.
We can use the formula for negative binomial distribution to determine how many failures are required before 12 successes are achieved.
The formula is:
\(P(X = x) = (r+x-1)C(x) \times p^r \times (1-p)^x\)
Where:
P(X = x) is the probability of having x failures before achieving 12 successes
r is the number of successes required (in this case, 12)
p is the probability of a single success (in this case, 0.82)
\((r+x-1)C(x)\)is the combination of r+x-1 choose x
For example, if we want to find the probability of Laura missing 3 free throws before making 12, we will plug in x = 3, r = 12, p = 0.82 into the formula:
\(P(X = 3) = (12+3-1)C(3) \times 0.82^1^2\times (1-0.82)^3\)
\(P(X = 3) = 14C3 \times 0.082^1^2 \times 0.18^3\)
\(P(X = 3) = 364 \times 0.000018 \times 0.005832\)
P(X = 3) = 0.000037
Overall, the trial in this scenario is each individual attempt at a free throw, the success is making a free throw, and 12 successes are required before the shooting session is considered complete.
The negative binomial distribution is used to calculate the probability of obtaining a certain number of failures before achieving the specified number of successes.
For similar questions on probability
https://brainly.com/question/24756209
#SPJ11
'x' follows a negative binomial distribution with 'r' being 12 successes.
In this scenario, a trial refers to each individual attempt at shooting a free throw. A success is when Laura successfully makes a free throw. To achieve her goal of making 12 free throws, she needs to have 11 successes (since she will miss on the final attempt). Therefore, r = 11.
In this context, a "trial" refers to each individual attempt Laura makes to shoot a basketball free throw. A "success" is when Laura makes a free throw (hits the basket), while a "failure" (not mentioned in the question) would be when Laura misses a free throw. Since Laura keeps shooting free throws until she makes 12, the number of successes required, denoted as 'r', is 12.
Therefore, 'x' follows a negative binomial distribution with 'r' being 12 successes.
Learn more about binomial distribution here:
https://brainly.com/question/31197941
#SPJ11
This question makes an important point: the maximum point of a function may not always be found by solving f'(x) = 0 Remember: functions can have their minimum or maximum at an endpoint of their domain, at a point of non-differentiability (think of the absolute value function, which has its minimum point at zero) or may not even have a maximum or minimum. This means that the most thorough way of solving optimization problems involves sketching the objective function. (For questions that appear on tests, however, the optimum will usually occur at a relative max. or relative min. that can be found by solving f'(x) = 0.) Two parts of this question are multiple choice: you only get one submission attempt for those two parts. A peach orchard owner wants to maximize the amount of peaches produced by his orchard. He has found that the per-tree yield is equal to 900 whenever he plants 30 or fewer trees per acre, and that when more than 30 trees are planted per acre, the per-tree yield decreases by 40 peaches per tree for every extra tree planted. For example, if there were 25 trees planted per acre, each tree would produce 900 peaches. If there were 35 trees planted per acre, each tree would produce 900 - 40 (35 - 30) peaches, which is roughly equal to 700 peaches. Find the function that describes the per-tree yield, Y, in terms of x. Y = if is no more than 30 trees per acre Y= Find the total yield per acre, T, that results from planting x trees per acre. T = if is no more than 30 trees per acre T = | c. Differentiate T with respect to x dT/dx = dT/dx = Does this derivative ever equal zero? Yes No d. Sketch the graph of T as x varies and hence find the value of x that maximizes the yield and the maximum value of the yield. Optimal value of : trees per acre Maximum yield : peaches per acre Is T differentiable when Times equals 30? Yes No
Answers
The maximum point of a function found by solving f'(x) = 0
1.Y(x) = 900, if x ≤ 30
2.900 - 40(x - 30), if x > 30
3.T(x) = x × Y(x)
4.dT/dx = 900, if x < 30
5.2100x - 40x² if x > 30
6.The derivative dT/dx equals zero at x = 52.5, but it is not valid in the given context.
7.The maximum yield occurs at x = 30 trees per acre, with a maximum yield of 27,000 peaches per acre.
8.T(x) is differentiable at x = 30.
To find the function that describes the per-tree yield, Y, in terms of x, use the given information:
If there are 30 or fewer trees planted per acre (x ≤ 30), the per-tree yield is 900 peaches per tree.
If there are more than 30 trees planted per acre (x > 30), the per-tree yield decreases by 40 peaches for every extra tree planted beyond 30.
The function Y(x) as follows:
Y(x) = 900, if x ≤ 30
Y(x) = 900 - 40(x - 30), if x > 30
find the total yield per acre, T, that results from planting x trees per acre. The total yield is simply the per-tree yield (Y) multiplied by the number of trees per acre (x):
T(x) = x × Y(x)
differentiate T with respect to x (dT/dx) to find where the maximum yield occurs.
dT/dx = d/dx (x × Y(x))
dT/dx = d/dx (x × (900 - 40(x - 30)))
To find if this derivative ever equals zero, it to zero and solve for x:
0 = 900 - 40(x - 30)
40(x - 30) = 900
x - 30 = 22.5
x = 52.5
So, the derivative dT/dx equals zero at x = 52.5. However, we need to check whether this value is valid for our function. Recall that if x > 30, the per-tree yield decreases by 40 peaches for every extra tree planted beyond 30. Therefore, planting 52.5 trees per acre would result in a negative per-tree yield, which is not physically meaningful in this context. Thus, the maximum yield occurs at the critical point within the valid domain, which is x = 30.
Now, let's find the maximum value of the yield at x = 30:
T(30) = 30 ×Y(30) = 30 × 900 = 27,000 peaches per acre
The optimal value of x that maximizes the yield is 30 trees per acre, and the maximum yield is 27,000 peaches per acre.
Finally, let's determine if T(x) is differentiable when x equals 30. Since T(x) is a piecewise function, we need to check if the left and right-hand derivatives at x = 30 are the same.
Left-hand derivative (x < 30):
dT/dx = d/dx (x × 900) = 900
Right-hand derivative (x > 30):
dT/dx = d/dx (x × (900 - 40(x - 30))) = d/dx (x × (900 - 40x + 1200)) = d/dx (2100x - 40x²)
Now, evaluate the right-hand derivative at x = 30:
dT/dx = 2100(30) - 40(30)² = 63,000 - 36,000 = 27,000
Since the left-hand derivative and the right-hand derivative at x = 30 are equal (both are 27,000), T(x) is differentiable at x = 30.
To know more about function here
https://brainly.com/question/31062578
#SPJ4
find the slope (-4,2) (8,-1)
Answers
Answer: Slope = -3/4 using the slope formula
Step-by-step explanation:
Answer:
-1/4
Step-by-step explanation:
\(slope=\frac{-1-2}{8-(-4)} =\frac{-3}{8+4} =\frac{-3}{12} =-\frac{1}{4}\)
I hope this help you
which paths shows the probability of picking exactly one red counter
Answers
this s hit makes no f ucking sense
The bearing of Measham from Tamworth is 052°
Calculate the bearing of Tamworth from Measham.
Answers
Answer:
232°
Step-by-step explanation:
1. 180-52=128
2. 360-128=232
first you subtract 52 from 180 bc you have to get the angle not included for calculating the whole bearing bc the starting point is the north line. so everything before the north line is not valid bc when you're calculating an angle like this you go from lef to to right, clockwise. in every calculation of bearings you go clockwise. next you just subtract the product you got from 360 and that's it.
hope it didn't sound too complicated.
test the hypothesis that the mean weight of the two sheets is equal (μ1−μ2)against the alternative that it is not (and assume equal variances). find the t-stat to 3 decimal places.
Answers
To test the hypothesis that the mean weight of two sheets is equal (μ1 - μ2) against the alternative that it is not, and assuming equal variances, we can use a two-sample t-test. The t-statistic can be calculated using the following formula:
t = (x1 - x2) / (s_p * sqrt(1/n1 + 1/n2))
where:
x1 and x2 are the sample means of the two sheets,
s_p is the pooled standard deviation,
n1 and n2 are the sample sizes.
The pooled standard deviation (s_p) can be calculated using the following formula:
s_p = sqrt(((n1 - 1) * s1^2 + (n2 - 1) * s2^2) / (n1 + n2 - 2))
where:
s1 and s2 are the sample standard deviations.
To calculate the t-statistic, we need the sample means, sample standard deviations, and sample sizes.
Once you provide the specific values for these variables, I can assist you in calculating the t-statistic to 3 decimal places.
To learn more about equal variances: -brainly.com/question/30777199
#SPJ11
To test the hypothesis that the mean weight of the two sheets is equal (μ1 - μ2) against the alternative that it is not, we can use a paired t-test assuming equal variances. The paired t-test is used when we have paired data or measurements on the same subjects or objects.
The t-statistic for a paired t-test is calculated as follows:
t = (X1 - X2) / (s / √n)
where X1 and X2 are the sample means of the two samples, s is the pooled standard deviation, and n is the number of pairs.
Please provide the sample means, standard deviation, and sample size for each sheet so that we can calculate the t-statistic to 3 decimal places.
To learn more about equal variances: -brainly.com/question/30777199
#SPJ11
help meeeeeeeeee pleasee

Answers
Answer: 2.5, 5.3
Step-by-step explanation:
\(-16t^2 +126t=217\\ \\ 16t^2 -126t+217=0\\\\t=\frac{-(-126) \pm \sqrt{(-126)^2 -4(16)(217)}}{2(16)}\\\\t \approx 2.5, 5.3\)
The density function of the continuous random variable x, the total number of hours, in units of 100 hours, that a family runs a vacuum cleaner over a period of one year is given below: f(x) = { x, 0 < x < 1 8 − x, 1 ≤ x < 2 0, otherwise Find the average number of hours per year that families run their vacuum cleaners?
Answers
Answer:
1000 hours
Step-by-step explanation:
∫xf(x)dx = ∫x*xdx (1,0) + ∫x*(8-x)dx (2,1)
∫xf(x)dx = ∫x²dx (1,0) + ∫8x - x²dx (2,1)
∫xf(x)dx = ∫x²dx (1,0) + ∫8xdx (2,1) - ∫x²dx (2,1)
∫xf(x)dx = x³/3(1,0) + 8x²/2 (2,1) - x³/3(2,1)
∫xf(x)dx = 1/3 + 8(4/2 - 1/2) - (8/3 - 1/3)
∫xf(x)dx = 1/3 + 8(2-0.5) - (7/3)
∫xf(x)dx = 1/3 + 8(1.5) - 7/3
∫xf(x)dx = 1/3 + 12 - 7/3
∫xf(x)dx = - 6/3 + 12
∫xf(x)dx = - 2 + 12
∫xf(x)dx = 10
The average number of hours the family runs their vacuum cleaner in units of 100 hours
100 * 10 hours = 1000 hours
Explain how you use a net to find the surface area of a prism.
Answers
Answer:
Nets of rectangular prisms are made up of rectangles and squares. Finding the areas of each of the rectangles and squares of the net of a rectangular prism and adding up those areas gives the surface area or total surface area of the prism.
Step-by-step explanation:
Answer:
By drawing a net, you can see each face of the prism. You can find the areas of each face and then add them together to find the total surface area.
Step-by-step explanation:
This is edges sample resopnse
10
वाल
8
What is the
solution to
this system
of equations?
6
4-
2+
([?]
-2
8
10
2
![108What is thesolution tothis systemof equations?64-2+([?]-28102](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/60OZQ4xcsUpcdlpnlfInCPLrlC0iM28M.png)
Answers
Answer:
(7, 4)
Step-by-step explanation:
The solution to the system of the 2 equations represented on the graph by the two lines is the c
x and y coordinates of the point where the two lines intersect.
They intersect at when x = 7, y = 4.
Therefore, the solution to the system of equations would be (7, 4).
what is the difference between mutiplying and adding polynomials
Answers
Answer:
When you add polynomials, you combine only like terms together. When you multiply polynomials, you multiply all pairs of terms together.
Step-by-step explanation:
.
Answer:
adding, subtracting and multiplying polynomials are, basically, the same as adding, subtracting and multiplying numbers. They only difference is that we have a pesky variable to worry about
In the figure below, N lies between M and P.
Find the location of N so that the ratio of MN to NP is 7 to 2.
M
- 27
Location of N
N
?
X
→→
Ś
P
-9
Answers
The position of N on the line segment is at the -14 mark
What are coordinates?A coordinate system in geometry is a system that employs one or more integers, or coordinates, to define the position of points or other geometric components on a manifold such as Euclidean space.
How to determine the location of N?The complete question is added as an attachment
From the attachment, we have
The ratio is given as:
MN : NP = 7 : 2
Also, we have
Location of M = -27
Location of P = -9
The location of N is then calculated as
N = MN/(MN + NP) * (M - P)
Substitute the known values
N = 7/(7 + 2) * (-27 + 9)
Evaluate the sum
N = 7/9 * -18
Evaluate the product
N = -14
Hence, the location of point N is -14
Read more about line segment ratio at
https://brainly.com/question/17374569
#SPJ1

13. Writing to Explain Explain how you would convert a
measurement given in ounces into pints.
Answers
Answer:
you divide the number of ounces by the number of pints
Step-by-step explanation:
Expand and combine like terms.
(6y^2+ 5y^4)^2
Answers
Answer:
36y⁴ + 60y⁶ + 25y⁸
Step-by-step explanation:
(6y² + 5y⁴)² = (6y² + 5y⁴)(6y² + 5y⁴)
we can use the FOIL method to get:
36y⁴ + 30y⁶ + 30y⁶ + 25y⁸
we can simplify the two middle terms and get:
36y⁴ + 60y⁶ + 25y⁸
Answer:
\(\left(6y^2+5y^4\right)^2\)
\((6y^2+5y^4)^2=36y^4+60y^6+25y^8\)
\(=\left(25y^8+60y^6+36y^4\right)\)
\(=\left(36y^4+60y^6+25y^8\right)\)
\(=36y^4+60y^6+25y^8\)
---------------------------
hope it helps...
have a great day!!
Question 8 in the regression demand equation e(log〖(sales) | price))=11. 015 -2. 442 log(price)〗, at a price of $1, what is the expected value of sales? the log here, is the natural log. 0 / 1 point
Answers
The expected value of sales at a price of $1 is 11.015 for the given regression demand equation.
In the regression demand equation e(log(sales | price)) = 11.015 - 2.442 log(price), you want to find the expected value of sales at a price of $1. To find the expected value of sales, you need to substitute the given price of $1 into the equation and solve for the sales. Substituting the price of $1 into the equation, you get: e(log(sales | 1)) = 11.015 - 2.442 log(1). Since log(1) = 0, the equation simplifies to: e(log(sales | 1)) = 11.015 - 2.442 * 0, e(log(sales | 1)) = 11.015
Therefore, the expected value of sales at a price of $1 is 11.015.
To know more about regression equation: https://brainly.com/question/25987747
#SPJ11
probability sampling procedures are question 13 options: a) instances where every unit in the population has a non-zero chance of being selected b) every possible answer is correct c) typically used when population parameters are known to exist d) certain to eliminate random sample error
Answers
Probability sampling procedures are instances where every unit in the population has a non-zero chance of being selected. (Option a)
Probability sampling is a type of sampling method used in statistical analysis, where each member of the population has a known and equal probability of being selected. This means that every possible unit in the population has a non-zero chance of being selected for the sample.
Probability sampling methods include simple random sampling, systematic sampling, stratified sampling, and cluster sampling. These methods are typically used when population parameters are unknown or when the researcher wants to make generalizations about the population based on the sample data.
Learn more about Probability Sampling:
https://brainly.com/question/28016369
#SPJ4
What type of angle iS ZCEB?
•
obtuse
O right
O straight
acute
Answers
Obtuse angle is the name of the angle m<CEB
Different types of anglesThe point where two lines meet is known as an angle. rom the given diagram, the sum of angles on a straight line is 180 degrees.
<CEB + 88 = 180 degrees
<CEB = 180 - 88
<CEB = 92 degrees
Since an obtuse angle is an angle greater than 90 but less than 180 degrees, hence the measure of angle <CEB is obtuse angle
Learn more on obtuse angle here: https://brainly.com/question/22828009
#SPJ1
