The sum of 13 and twice a number and is it linear
Answers
Answer:
Step-by-step explanation:
The number is already defined as ' h '. "Twice" means times by 2. So, 13 and twice 'h' are being added together. Here 13 and h are to be added, and the answer is doubled.
Related Questions
need help asap. pls somebody help.

Answers
Answer:
D
Step-by-step explanation:
why the panic ? you only need to compare the tiles with the actual terms in the equations and add them up.
x²
-x²
-x -x
x x x x (clearly that means 4x)
-1 -1 -1
1 1
so, we see it is D.
Solve by completing the square: 4x^2 - 8x - 8 = 0
a) -2 + sqt. 2, -2 - sqt.2
b) 1 + sqt. 3, 1 - sqt. 3
c) 1 + sqt. 2, 1 - sqt. 2
d) -2 + sqt. 3, -2 - sqt.3
Answers
Answer: the correct answer is B 1+\/~3,1-\/~3
edge 2021-2022
Step-by-step explanation:
Find the midpoint of each line segment.

Answers
Answer:
(1.5, 0.5)
Step-by-step explanation:
(5, 3) and (-2, -2)
5 - 2 / 2 = 1.5
-2 + 3 / 2 = 0.5
If Melinda wanted to build a patio in her backyard that was 14 feet long, 6 feet wide
and 4 inches deep using pervious concrete mix that is 4 parts aggregate to 4.5 parts
loose cement, with some water added then how many cubic feet of aggregate would
she need to build the walkway?
Round the final answer to the hundredths place. If you answer doesn't have a
hundredths place include zeros so that it does
Answers
The volume of aggregate that she needs to build the patio is 13.18 ft^3
How many cubic feet of aggregate would she need to build the walkway?
We know that the backyard is 14ft long, 6 feet wide and 4 inches deep.
Rememeber that:
1ft = 12 in
Then:
4 in = (4/12) ft = (1/3) ft
Then the volume of the backyard is:
V = (14ft)*(6ft)*(1/3 ft) = 28ft^3
Now we know that we have a mix of 4 parts aggregate to 4.5 parts loose cement that need to fill these 28 cubic feet.
Note that:
4 + 4.5 = 8.5
How many times we have 8.5 in 28?
28/8.5 = 3.294
Now for each of these we have 4 parts of aggregate, then the volume of aggregate that we need is:
4*3.294 ft^3 = 13.18 ft^3
The volume of aggregate that she needs to build the patio is 13.18 ft^3
If you want to learn more about volumes:
https://brainly.com/question/1972490
#SPJ1
please help me solve this problem

Answers
The features related to vectors are listed below:
Case A: u' = (- 4√41 / 41, - 5√41/ 41)
Case B: Tip coordinantes of vector v: (x, y) = (10, 10)
Case C: r = 11.402
How to determine vectors
In this question we must determine three features related to vectors: (a) unit vector, (b) the definition of a vector based on the coordinates of its base and its tip, (c) The magnitude of a resulting vector.
Case A - Unit vector
Unit vectors are vectors with magnitude 1, for the case of vector u we have the following definition:
u' = u / ||u||
Where:
u' - Unit vector.u - Vectoru - ||u|| - Magnitude of the vector.Please notice that the magnitude of the vector is determined by Pythagorean theorem:
r = √[(Δx)² + (Δy)²]
Where:
r - MagnitudeΔx - Change in the x-direction.Δy - Change in the y-direction.Thus, the unit vector of u is:
u' = (- 4, - 5) / √[(- 4)² + (- 5)²]
u' = (- 4, - 5) / √41
u' = (- 4 / √41, - 5 / √41)
u' = (- 4√41 / 41, - 5√41/ 41)
Case B - Definition of a vector
This can be found easily by vector subtraction:
v = Tip coordinates - Base coordinates
(3, 4) = (x, y) - (7, 6)
(x, y) = (3, 4) + (7, 6)
(x, y) = (10, 10)
Case C - Magnitude of a vector
The magnitude is found by means of Pyhtagorean theorem:
r = √[(- 4 - 3)² + (- 5 - 4)²]
r = √[(- 7)² + (- 9)²]
r = 11.402
To learn more on unit vectors: https://brainly.com/question/28028700
#SPJ1
2. Write Equation of a Circle given graph or two points Erin Hoffmann •
Answers
The equation of the circle graphed is (x + 4)² + (y + 5)² = 16
Determining the equation of the circle graphedFrom the question, we have the following parameters that can be used in our computation:
The circle (see attachment for complete question)
Where, we have
Center = (a, b) = (-4, -5)
Radius, r = 4 units
The equation of the circle graphed is represented as
(x - a)² + (y - b)² = r²
So, we have
(x + 4)² + (y + 5)² = 4²
Evaluate
(x + 4)² + (y + 5)² = 16
Hence, the equation is (x + 4)² + (y + 5)² = 16
Read more about circles at
brainly.com/question/24810873
#SPJ1

Find a vector function that represents the curve of intersection of the two surfaces: The cone z=sqrt(x^2 + y^2) and the plane z =1 + y.
Answers
Thus, a vector function that represents the curve of intersection of the cone and the plane is: \(r(t) = tcos(t), 1 +\sqrt{ (1 - t^2)}, 1 -\sqrt{ (1 - t^2)}, tsin(t)\)
The vector function that represents the curve of intersection of a cone and a plane can be found by equating the equation of the cone and the equation of the plane, and then solving for x and y in terms of z.
Given the cone \(z = \sqrt{(x^2 + y^2)}\) and the plane \(z = 1 + y\) , we have:
\(\sqrt{(x^2 + y^2) }= 1 + y\)
Squaring both sides, we get:
\(x^2 + y^2 = (1 + y)^2 = 1 + 2y + y^2\)
Solving for y, we get:
\(x^2 + y^2 - 2y = 1\)
\(y^2 - 2y + x^2 - 1 = 0\)
Using the quadratic formula, we get:
\(y = 1 + \sqrt{(1 - x^2)} , 1 + \sqrt{(1 - x^2)}\)
Thus, a vector function that represents the curve of intersection of the cone and the plane is:
\(r(t) = tcos(t), 1 +\sqrt{ (1 - t^2)}, 1 -\sqrt{ (1 - t^2)}, tsin(t)\)
where t varies between -1 and 1.
The resulting curve is a circle with center (0, 1, 0) and radius 1, which lies in the xy-plane and is perpendicular to the z-axis.
Learn more about curve :
https://brainly.com/question/28793630
#SPJ4
Help please! thank you xoxo!
2 math problems


Answers
3. x = 45
4. 73°
Step-by-step explanation:
3. The sum of the interior angles of a pentagon is 540° so
90° + 112° + 2x + (2x + 10) + 148° = 540°
Simplifying this, we get
4x + 360 = 540 => 4x = 180 or x = 45
4. The sum of the interior angles of a pentagon is 540° which means that the unknown interior angle is 107°. This angle is a supplementary angle to \(\angle{A}\) so \(\angle{A}\) is
180° - 107° = 73°.
Find the perimeter of the quadrilateral below.
Give your answer in centimetres (cm) to 1 d.p.
W
9 cm
Z
12 cm
X
8 cm
Answers
For given measurements, the perimeter of the quadrilateral is 30cm.
What is the perimeter of the quadrilateral ?The length of a quadrilateral's border, or what remains after joining all four of its sides to form a single line segment, is referred to as the quadrilateral's perimeter. As a result, a quadrilateral's perimeter is measured in the same linear units as its sides, such as meters, inches, centimeters, etc.
This may be stated using a straightforward formula. For instance, the formula for a quadrilateral ABCD's perimeter may be written as,
AB + BC + CD + DA = The perimeter
perimeter of the quadrilateral = sum of all sides.
perimeter of the quadrilateral = 1 + 9 + 12 + 8
= 30 cm.
To learn more about perimeter of the quadrilateral from given link
https://brainly.com/question/12546443
#SPJ9
Identify the key features of these functions:
f(x) = 3(x + 5)2 − 10
g(x) = 200(0.95)x
h(x) = -0.25(x + 3)(x − 1)
The vertex of f(x) is at
. The function g(x) is decreasing at a rate of
%. The zeros of h(x) are at
and
.
Answers
Answer:
The vertex of f(x) is at (-5,-10).
The function g(x) is decreasing at a rate of 5%.
The zeros of h(x) are at x = -3 and x = 1.
Step-by-step explanation:
Vertex of a quadratic function:
Suppose we have a quadratic function in the following format:
\(f(x) = ax^{2} + bx + c\)
It's vertex is the point \((x_{v}, f(x_{v})\)
In which
\(x_{v} = -\frac{b}{2a}\)
If a<0, the vertex is a maximum point, that is, the maximum value happens at \(x_{v}\), and it's value is \(f(x_{v})\)
Decaying exponential function:
A decaying exponential function has the following format:
\(A(x) = A(0)(1-r)^{x}\)
In which A(0) is the initial quantity and r is the decay rate.
Zeros of a function:
Given a polynomial f(x), this polynomial has roots \(x_{1}, x_{2}, x_{n}\) such that it can be written as: \(a(x - x_{1})*(x - x_{2})*...*(x-x_n)\), in which a is the leading coefficient.
Vertex of f:
The function f is given by:
\(3(x+5)^2 - 10\)
Expanding the calculations to place at the correct format:
\(3(x+5)^2 - 10 = 3(x^2 + 10x + 25) - 10 = 3x^2 + 30x + 75 - 10 = 3x^2 + 30x + 65\)
Which means that
\(a = 3, b = 30, c = 65\)
The x-value of the vertex is:
\(x_{v} = -\frac{30}{2*3} = -5\)
The y-value of the vertex is:
\(f(-5) = 3(-5+5)^2 - 10 = -10\)
The vertex of f(x) is at (-5,-10).
Decreasing rate of g(x).
We have that:
1 - r = 0.95
So
r = 1 - 0.95 = 0.05
Which means that the decreasing rate is of 5%.
The zeros of h(x) are
The zeros are
x + 3 = 0 -> x = -3
x - 1 = 0 -> x = 1
So
The zeros of h(x) are at x = -3 and x = 1.
Is -1.27 a rational number
Answers
Answer:
Yes.
Step-by-step explanation:
Answer:
si
Step-by-step explanation:
Find the value of x in the diagram. ΔPQR ~ ΔSTU

Answers

Reuben made a shirt using 7/8yards of red fabric and 1/4yards of yellow fabric. How many more yards of red fabric did Reuben use?
Answers
Answer and Step-by-step explanation:
To find out how many more yards of red fabric Reuben used, we need to subtract the amount of yellow fabric from the amount of red fabric. Since the two fractions have different denominators, we need to find a common denominator before subtracting them. The least common multiple of 8 and 4 is 8, so we can rewrite both fractions with a denominator of 8:
7/8 - 1/4 = 7/8 - (1/4) * (2/2) = 7/8 - 2/8 = (7 - 2)/8 = 5/8
So, Reuben used 5/8 yards more red fabric than yellow fabric.
99 6th graders took a test the median score was 72 how many students scored greater than 72
Answers
For the given median, there are 50 students who scored greater than 72.
What is median?
The median is a measure of central tendency in a set of numerical data. It is the value that separates the higher half of the data from the lower half when the data is arranged in order from least to greatest or greatest to least.
We are given that 99 6th graders took a test and the median score was 72. We want to find out how many students scored greater than 72.
Let the scores be denoted by S1, S2, ..., S99. We can sort these scores in order from least to greatest:
S1 ≤ S2 ≤ ... ≤ S99
Since the median score is 72, exactly half of the scores are above 72 and half are below 72. Thus, the 50th score in the sorted list is 72.
Since there are 99 scores in total, we can approximate the number of students who scored above 72 by dividing the total number of students by 2:
\($\frac{99}{2} = 49.5$$\)
This means that approximately 49.5 students scored above 72.
Since 0.5 rounds up to 1, we round 49.5 up to 50. Therefore, approximately 50 students scored greater than 72.
To learn more about median visit:
https://brainly.com/question/26177250
#SPJ1
Solve and will mark brainliest
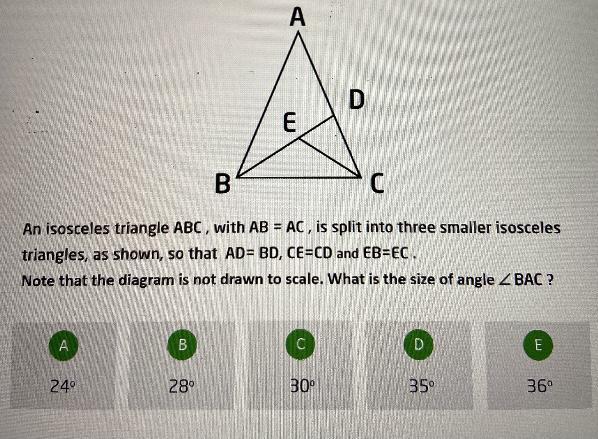
Answers
Answer:
24 degrees
Step-by-step explanation:
24 degrees because
angle a is 24 degrees
and since its the middles and main angle
24 degrees is the answer
At the opening of a new movie 45% of the people in the theater were children.There were 187 adults were in the theater.What was the total number of people at the movie?
Answers
The number of people at the movie is 340
How to determine the number of people at the movieLet's represent the total number people at the movie as "x".
We know that the number of adults in the movie is 187 and the percentage of children is 45%
So we can write:
187 = (1 - 45%) * x
This gives
187 = 0.55x
To solve for x, we can divide both sides by 0.55:
x = 187/0,55
Evaluate
x = 340
Hence, the number of people is 340
Read more about percentage at
https://brainly.com/question/24877689
#SPJ1
Necesito ayuda Urgentemente

Answers
Answer:
x = 100° || y = 20°
Step-by-step explanation:
Two pairs of parallel lines visible.
Create equations 1 and 2:
x - 2y = 60°
x + y = 120°
Make the x subject:
x - 2y = 60°
x = 60° + 2y ........equation 1
x + y = 120°
x = 120° - y ........equation 2
Solve them simultaneously:
120° - y = 60° + 2y
2y + y = 120°- 60°
3y = 60°
y = 20°
If y is 20° then the x is:
x = 120° - y
x = 120° - 20°
x = 100°
Which equations represent circles that have a diameter of 12 units and a center that lies on the y-axis? Select two options. x2 + (y – 3)2 = 36 x2 + (y – 5)2 = 6 (x – 4)² + y² = 36 (x + 6)² + y² = 144 x2 + (y + 8)2 = 36
Answers
Answer:
x^2 + (y – 3)^2 = 36
Step-by-step explanation:
The standard equation of a circle with center at (h, k) and radius r is
(x - h)^2 + (y - k)^2 = r^2.
If the center lies on the y-axis, then h = 0: (x - 0)^2 + (y - k)^2 = r^2
If the circle diameter is 12, then the circle radius is 6, and so r^2 = 36
So, among the given equations, your
x^2 + (y – 3)^2 = 36 is correct (but only if you use " ^ " for exponentiation).
❗❗100 POINTS FOR CORRECT ANSWER AND EXPLANATION❗❗~math question~
thanks)

Answers
Answer:
k = 110
Step-by-step explanation:
You want the integer multiple of pi that is the area of 300° of a donut with inner radius 8 cm and outer radius of 14 cm.
Area of a circleThe area of a circle is given by the formula ...
A = πr²
Then the area of the outer circle is ...
A = π(14 cm)² = 196π cm²
The area of the inner circle is ...
A = π(8 cm)² = 64π cm²
Donut areaThe area of the whole donut is the difference of these areas:
whole donut area = (196 -64)π cm² = 132π cm²
Shaded areaThe shaded area of the figure represents 300° of the 360° full donut. The area is proportional to the central angle, so the shaded area is ...
shaded area = (300°/360°)×whole donut area
shaded area = 5/6 × 132π cm² = 110π cm²
Comparing this to the expression kπ cm², we see that ...
k = 110
ANSWERS NEEDED ASAP 50 POINTS
1. what is the y-intercept when the coordinate is 9,1 and the slope is 4 8
2. What is the y intercept when the coordinate is -3,-3 and the slope is -1
Answers
1. The y-intercept when the coordinate is 9,1 and the slope is 4 8= -35
2. The y intercept when the coordinate is -3,-3 and the slope is -1= -6
What is the purpose of coordinate geometry?The term "coordinate geometry" refers to the study of geometry using coordinate points (or analytic geometry). Coordinate geometry allows for a variety of operations, like measuring the distance between two points, segmenting lines into m:n pieces, determining a line's midpoint, calculating a triangle's area in the Cartesian plane, and more.
1.To find the y-intercept, we can use the slope-intercept form of a linear equation: y = mx + b, where m is the slope and b is the y-intercept.
Given the point (9,1) and slope 4 8, we can plug in these values to get:
1 = (4 8)9 + b
Simplifying this equation, we get:
1 = 36 + b
b = -35
Therefore, the y-intercept is -35.
2. Again, using the slope-intercept form of a linear equation, we have:
y = mx + b
Given the point (-3,-3) and slope -1, we can plug in these values to get:
-3 = (-1)(-3) + b
Simplifying this equation, we get:
-3 = 3 + b
b = -6
Therefore, the y-intercept is -6.
To know more about coordinate geometry visit:
https://brainly.com/question/29634141
#SPJ1
3 6 9 12 15 18 21 24 27 30 is odd or even numbers?
Answers
Answer: Half of them are even and half of them are odd.
Step-by-step explanation:
The even numbers are 6, 12, 18, 24, and 30. An even number is defined as a number that is divisible by 2, meaning it has no remainder when divided by 2. For example, 6 divided by 2 equals 3 with no remainder, so 6 is even.
The odd numbers are 3, 9, 15, 21, and 27. An odd number is defined as a number that is not divisible by 2, meaning it has a remainder of 1 when divided by 2. For example, 9 divided by 2 equals 4 with a remainder of 1, so 9 is odd.
Therefore, out of the given numbers, half of them are even and half of them are odd.
________________________________________________________
3
Type the correct answer in the box. If necessary, use / for the fraction bar.
Both pyramids in the figure have the same base area as the pyramid to the volume of the prism, expressed as a fraction in simplest form is
Answers
Answer:
I got 1/3
Step-by-step explanation:
hope it helps
may somone give me the answers with question 3 4 and 5. one BRAINLIEST IF SOMONE CAN ANSWER AND FIVE STAR

Answers
Answer:
Well, we need a definition or length/width of the figure of it first, let me see what I can find, I'll leave it in my replies.
Step-by-step explanation:
so basically the writing says to draw the lines indicated by arrows and include the markings on the last one because they indicate that all the sides are equal in length
;P hope this helps!

9. Find m DF
m
140°
DF:
=
E
44°
20. Find
mZPQR.
131*
m/POR=
Need done asap please show work

Answers
According to the angles between intersecting secant and tangent, the value of
angle DF is 52 degrees
angle PQR = 49 degrees
How to solve for angle DFThe value of angle DF is solved using the angles of intersecting secant out side the circle
The theorem give the formula in the form
44 = 1/2 (140 - DF)
88 = 140 - DF
DF = 140 - 88
DF = 52 degrees
Using intersection of tangents, for the second figure
exterior angle = 1/2 (major arc - minor arc)
major arc = 360 - 131 = 229
PQR = 1/2 (229 - 131)
PQR = 49 degrees
Learn more about intersecting secant theorem at
https://brainly.com/question/30242636
#SPJ1
h(1)=0. h(n)=h(n-1)-9 explicit formula for h(n)=
Answers
Answer:
0-9(n-1)
Step-by-step explanation:
We can tell the first term of the sequence is 0 and the common difference is -9. So the explicit formula would be h(n)=0-9(n-1)
The explicit formula for h(n) comes to be h(n)=9-9n.
What is an arithmetic progression?An arithmetic progression is a list of numbers where the difference between consecutive terms is always constant.
\(h(n)=h(n-1)-9\)
\(h(n)-h(n-1)=-9\)
So the common difference of the arithmetic progression will be -9.
\(h(1)=0\) means the first term of the AP is 0.
We know that \(n^{th}\) term of an AP is given by:
\(h(n)=a+(n-1)d\)
Where a is the first term and d is a common difference.
Fo the given series \(a=0\) and \(d=-9\)
So, \(h(n)=0+(n-1)(-9)\)
\(h(n)=9-9n\)
So, the explicit formula for h(n) is \(h(n)=9-9n\)
Hence, the explicit formula for h(n) comes to be \(h(n)=9-9n\).
To get more about arithmetic progressions visit:
https://brainly.com/question/6561461
What is the constant up a proportionally in a equation y=x/g
Answers
Answer:
Step-by-step explanation:
\(y=(\frac{1}{g} )x\)
Constant up a proportionally is \(\frac{1}{g}\).
How do you isolate the variable in an inequality?
Answers
For one to isolate the variable by using equation rules. Inverse inequality sign if multiplying/dividing by negative. Steps to isolate variable in inequality: Simplify, move constants with inverse operations. Divide coefficient to isolate variable. Reverse direction if using negative number.
What is the variable?Inequalities require you to apply the same principles as those used in equations when isolating a variable. For example, if there is an inequality 3x - 5 < 7. one can isolate the variable, by first:
Add 5 to both sides to be: 3x < 12.
Then divide both sides by 3: x < 4.
So, the variable x is one that is isolated, and the solution to the inequality is one where x < 4.
Learn more about inequality from
https://brainly.com/question/24372553
#SPJ1
Two ropes are attached to a wagon, one horizontal to the west with a tension force of 30N, and the other east and at an angle of 30 degrees northward and a tension force of 40 N. Find the components of the net force on the cart. Show all work.
Answers
The net force on the cart is 62N
and the vertical component =36.64 N
the horizontal component= 50N
What is revolution of vector?
The process of splitting a vector into its components is called resolution of the vector. We resolve a vector into two components which are. component along the x-axis called horizontal component. component along the y-axis called vertical component
30N tension on the cart is westward which mean the vertical component will be 0 and the horizontal component will be 30N.
40N tension isN 30° E which means
the vertical component= 40 sin60=36.64N
Horizontal component= 40 cos 60= 20N
sum of vertical = 36.64N +0= 36.64N
sum of horizontal= 30N+20N= 50N
60° was used for tension 40N because the angle of vector must always lie on the horizontal axis
therefore the net force F is obtained by using Pythagoras theorem
F= √(50^2+36.64^2)
= 62N to the nearest whole number.
learn more about resolution of vectors from
https://brainly.com/question/17369412
#SPJ1
For a géometric sequence, if a1=9 and a5
=2304, what is the value of r?
Answers
The value of common ration r is 4.
Given that,
a₁ = 9
a₅ = 2304
We know that,
A geometric sequence is a sequence in which each term (except from the first term) is multiplied by a fixed amount to obtain the following term.
The following term in the geometric sequence must be obtained by multiplying it by a set term (referred to as the common ratio), and the previous term in the sequence can be obtained by dividing it by the same common ratio.
Since we know that nth term of GP is,
\(a_{n}\) = a₁ \(r^{n-1}\)
Therefore,
a₅ = a₁ \(r^{4}\) = 2304
⇒ 9 x \(r^{4}\) = 2304
⇒ \(r^{4}\) = 256
⇒ r = 4
Hence, r = 4
To learn more about GP visit:
https://brainly.com/question/29323941
#SPJ1
Analyze the graph of the function f(x) to complete the statement. On a coordinate plane, a curved line, labeled f of x, with a minimum value of (0, negative 3) and a maximum value of (negative 2.4, 17), crosses the x-axis at (negative 3, 0), (negative 1.1, 0), and (0.9, 0), and crosses the y-axis at (0, negative 3). f(x)<0 over and what other interval?
Answers
The interval where function f(x) is negative and greater than 0 is (-∞, -3) U (-1.1, 0)
We have,
The function f(x) crosses the x-axis at (-3, 0), (-1.1, 0), and (0.9, 0), it means that f(x) is negative for x values less than -3, between -1.1 and 0.9, and greater than 0.
Therefore, we can say that:
f(x) < 0 for x < -3 and -1.1 < x < 0.9
And,
The function f(x) has a minimum value of (0, -3) and a maximum value of (-2.4, 17).
This means that f(x) is positive for x values greater than -2.4. Therefore, we can say that:
f(x) > 0 for x > -2.4
Now,
Combining these inequalities, we can say that f(x) is negative over the intervals (-∞, -3) and (-1.1, 0.9), and positive over the interval (-2.4, ∞).
So,
The interval where f(x) is negative and greater than 0 is:
(-∞, -3) U (-1.1, 0)
Thus,
The interval where f(x) is negative and greater than 0 is (-∞, -3) U (-1.1, 0)
Learn more about functions here:
https://brainly.com/question/28533782
#SPJ1