The sum of the firstt five terms of a geometric series is 33 and the sum of the first ten terms of the geometric series is -1023. a) Find the common ratio and the first term of the series. b) Find the general term of the series. Simplify your answer.
Answers
a. The common ratio is 2.
Given that the sum of the first five terms of the geometric series is 33, we use the formula for the sum of terms of a geometric series, S
Sₙ = a(rⁿ - 1)/(r - 1) where a = first term and r = common ratio
Since n = 5, the first 5 terms, and S₅ = 33
Sₙ = a(rⁿ - 1)/(r - 1)
33 = a(r⁵ - 1)/(r - 1) (1)
Also, when n = 10, the sum of the first 10 terms is S₁₀ = -1023
So, -1023 = a(r¹⁰ - 1)/(r - 1) (2)
Dividing (2) by (1), we have
-1023/33 = a(r¹⁰ - 1)/(r - 1) ÷ a(r⁵ - 1)/(r - 1)
-31 = (r¹⁰ - 1)/(r⁵ - 1)
-31(r⁵ - 1) = r¹⁰ - 1
-31r⁵ + 31 = r¹⁰ - 1
r¹⁰ - 1 + 31r⁵ - 31 = 0
r¹⁰ + 31r⁵ - 32 = 0
Let r⁵ = y
(r⁵)² + 31r⁵ - 32 = 0
y² + 31y - 32 = 0
Factorizing, we have
y² + 32y - y - 32 = 0
y(y + 32) - (y + 32) = 0
(y - 1)(y - 32) = 0
y - 1 = 0 or y - 32 = 0
y = 1 or y = 32
r⁵ = 1 or r⁵ = 32
r = ⁵√1 or r = ⁵√32
r = 1 or r = 2
Since for a geometric series, r ≠ 1, r = 2.
So, the common ratio is 2.
ii. The first term of the series.
The first term of the series is 33/31
Using (1)
33 = a(r⁵ - 1)/(r - 1) (1) where r = 2,
33 = a(2⁵ - 1)/(2 - 1) (1)
33 = a(32 - 1)/1
33 = 31a
a = 33/31
So, the first term of the series is 33/31
b. Find the general term of the series. Simplify your answer.
The general term of the geometric series is (33/62) × 2ⁿ
The general term of a geometric series is Uₙ = arⁿ⁻¹
With a = 33/31 and r = 2,
Uₙ = arⁿ⁻¹
Uₙ = (33/31) × 2ⁿ⁻¹
Uₙ = (33/31) × 2ⁿ/2
Uₙ = (33/62) × 2ⁿ
So, the general term of the geometric series is (33/62) × 2ⁿ
Learn more about geometric series here:
https://brainly.com/question/17445865
Related Questions
If △NMO ∼ △XYZ, and the area of △NMO is 147 square feet, find the area of △XYZ?

Answers
The area of the triangle △XYZ is 362.34 square feet
Similar trianglesThe ratio of the sides of the triangles is equal to a constant.
Area of triangle △NMO = 0.5bh
21168 = 0.5(14)b
7b =21168
b = 3024in
Using the similar triangle theorem;
14/3024 = 22/XZ
XZ = 33 in = 33/12 =396ft
YZ = 22/12 = 1.83ft
Area of △XYZ = 0.5 *396 * 1.83
Area of △XYZ = 362.34 square feet
Hence the area of the triangle △XYZ is 362.34 square feet
Learn more on similar triangle here: https://brainly.com/question/2644832
A company owns two manufacturing plants with daily production levels of 8x + 17 widgets and 5x - 7 widgets, where x represents a minimum quantity. How many more items does the first plant produce daily than the second plant?Immersive Reader
Answers
Answer:
3x + 24
Step-by-step explanation:
Given that;
Company owns two manufacting plants with the following daily production levels :
PLANT A:
8x + 17 widgets
PLANT B:
5x - 7 widgets
Where x = minimum quantity
How many more items does the first plant produce daily than the second plant?
Subtract the Plant B's production level from plant A
8x + 17 - (5x - 7)
8x + 17 - 5x + 7
Collecting like terms
8x - 5x + 17 + 7
3x + 24
Hence, the number of items the first plant produce daily than the second plant is :
3x + 24
The quotient of a number divided by 4 is -12. What is the number? _____
Answers
Answer:
-48
Step-by-step explanation:
I'm Bigbrain
Find a recurrence relation for the number of n digit quaternary (0, 1, 2, 3) sequences with at least one 1 and the first 1 occurring before the first 0 (possibly no 0s).
Answers
The recurrence relation for the number of n-digit quaternary sequences with at least one 1 and the first 1 occurring before the first 0 is:
\(A_n = 2 * A_{n-1}\)
Let's denote the number of n-digit quaternary sequences satisfying the given conditions as \(A_n\). We can establish a recurrence relation for A_n based on the position of the first digit in the sequence.
Consider the possible cases:
1. Case 1: The first digit is 1.
In this case, the remaining n-1 digits can be any valid (n-1)-digit quaternary sequence that satisfies the given conditions.
Since the first 1 has already been placed, the remaining sequence can have any combination of digits 1, 2, and 3. Hence, the number of such sequences is \(A_{n-1}\).
2. Case 2: The first digit is not 1.
If the first digit is not 1, it must be 2 or 3 (as per the given conditions). In this case, the remaining n-1 digits can be any valid (n-1)-digit quaternary sequence satisfying the given conditions, without any restriction on the placement of 0s and 1s.
Hence, the number of such sequences is \(A_{n-1}\).
Therefore, the recurrence relation for the number of n-digit quaternary sequences with at least one 1 and the first 1 occurring before the first 0 is:
\(A_n = 2 * A_{n-1}\)
This recurrence relation states that the number of n-digit sequences satisfying the given conditions is twice the number of (n-1)-digit sequences satisfying the same conditions.
We also have the base case A₁ = 1, as there is only one valid 1-digit quaternary sequence that satisfies the given conditions, which is "1".
Using this recurrence relation, you can compute the number of n-digit quaternary sequences with the specified conditions for any given value of n.
To know more about recurrence relation refer here:
https://brainly.com/question/31055199#
#SPJ11
While reading about a research study, which of the following would tell you that an association claim is being
made?
a. The presence of a scatterplot or bar graph
b. The measurement of two variables
c. The use of a correlation coefficient
d. The interrogation of internal validity
Answers
The answer to this question is c.The use of a correlation coefficient.
The use of a correlation coefficient would tell you that an association claim is being made in a research study. A correlation coefficient is a statistical measure that shows the strength and direction of a relationship between two variables. It ranges from -1 to 1, with 0 indicating no relationship and -1 or 1 indicating a perfect negative or positive relationship, respectively.
A scatterplot or bar graph may be used to visually display the relationship between two variables, but they do not necessarily indicate that an association claim is being made. The measurement of two variables is necessary for any type of research study, but it does not inherently imply that an association claim is being made.
Interrogation of internal validity is a process used to ensure that the study's results accurately reflect the relationship between the variables being studied. It is important for any research study but does not specifically indicate that an association claim is being made.
Therefore,the answer to this question is c.The use of a correlation coefficient.
Know more about correlation coefficient here,
https://brainly.com/question/29704223
#SPJ11
scores on an exam follow an approximately normal distribution with a mean of 76.4 and a standard deviation of 6.1 points. what is the minimum score you would need to be in the top 7%?
Answers
To find the minimum score needed to be in the top 7%, we need to find the score that corresponds to the 93rd percentile, since the top 7% is the complement of the bottom 93%.
Using a standard normal distribution table or a calculator, we can find the z-score that corresponds to the 93rd percentile, which is approximately 1.44. This means that a score of 1.44 standard deviations above the mean corresponds to the 93rd percentile.
To find the actual score, we can use the formula:
z = (x - μ) / σ
where z is the z-score, x is the actual score, μ is the mean, and σ is the standard deviation. Solving for x, we get:
x = z * σ + μ
= 1.44 * 6.1 + 76.4
= 85.084
So, the minimum score needed to be in the top 7% is approximately 85.084 points.
refer to exercise 7.11. suppose that in the forest fertilization problem the population standard deviation of basal areas is not known and must be estimated from the sample. if a random sample of n = 9 basal areas is to be measured, find two statistics g1 and g2 such that p (g1 ≤ ( y - u ) ≤ g2 ) = 90
Answers
Confidence interval = (y ± t∗s/√n)g1 = y - t*s/√ng2 = y + t*s/√n Substituting the values, g1 = 26.22 - 1.860*(0.11)/√9 = 25.84g2 = 26.22 + 1.860*(0.11)/√9 = 26.59Therefore, the statistics g1 and g2 that will satisfy the required inequality are 25.84 and 26.59 respectively.
The formula for finding the confidence interval is as follows: n − 1, where t is the value of the t-distribution corresponding to the specified confidence level and the sample size minus one.
As per the given exercise 7.11, suppose that in the forest fertilization problem the population standard deviation of basal areas is not known and must be estimated from the sample.
If a random sample of n = 9 basal areas is to be measured, find two statistics g1 and g2 such that p(g1 ≤ (y - u) ≤ g2) = 90
To find the statistics g1 and g2 that will satisfy the required inequality
the following formula can be used: Confidence interval = \((y ± t∗s/√n)\)
From the formula, we can see that the confidence interval depends on the values of y, s, t and n.
The value of y is the sample mean
the value of s is the sample standard deviation
And the value of n is the sample size.
The value of t depends on the confidence level desired and the degrees of freedom for the t-distribution. In this case, the confidence level is 90%, which means that we want to find the value of t that will give us a total area of 0.90 under the t-distribution curve with 8 degrees of freedom .Using the t-table, the value of t can be found to be 1.860, where the value for 90% and 8 degrees of freedom is 1.860.t = 1.860Now, we need to calculate the value of s, which is the sample standard deviation.
Since we do not have any information about the population standard deviation, we will use the sample standard deviation as an estimate of the population standard deviations = σ/√nσ = s*√nσ = 0.11*√9σ = 0.33Substituting the values in the confidence interval formula
for such more questions on statistics
https://brainly.com/question/30915447
#SPJ11
Find the equation of the linear function represented by the table below in slope-
intercept form.
X
у
-3
10
1
1
-10
5
30
9
-50

Answers
Answer:
y= -5x-5
Step-by-step explanation:
because m=y2-y1/x2-x1 and then you plug in one of the point to find the y intercept or b and the answer you should get is -5 for be so it ends up being y= -5x-5
During January, the normal high temperature in Duluth, Minnesota, is 16 degrees * F , and the normal low temperature is - 2 degrees * F . Find the difference between the temperatures
Answers
Answer:
18 deg F
Step-by-step explanation:
Positive difference =
16 deg F - (-2 deg F) =
(16 - (-2)) deg F =
(16 + 2) deg F =
18 deg F
what are the minimum and maximum numbers of elements in a heap of height h?
Answers
In a heap, the height is defined as the number of edges on the longest path from the root to a leaf node. The height of a heap with n elements is at most log₂(n+1).
To find the minimum and maximum numbers of elements in a heap of height h, we can use the formula:
The minimum number of elements in a heap of height h is 2^h (a complete binary tree of height h with the minimum number of nodes).
The maximum number of elements in a heap of height h is 2^(h+1) - 1 (a complete binary tree of height h with the maximum number of nodes).
Therefore, the minimum and maximum numbers of elements in a heap of height h are:
Minimum: 2^h
Maximum: 2^(h+1) - 1
Note that not all values of h are valid heap heights. A heap must be a complete binary tree, so its height can only take on values that satisfy the formula: \(h < = log₂(n+1),\)where n is the number of elements in the heap.
Learn more about height here:
https://brainly.com/question/10726356
#SPJ11
HELP WILL GIVE BRAINLIEST
help me find the equations for the piece wise graph
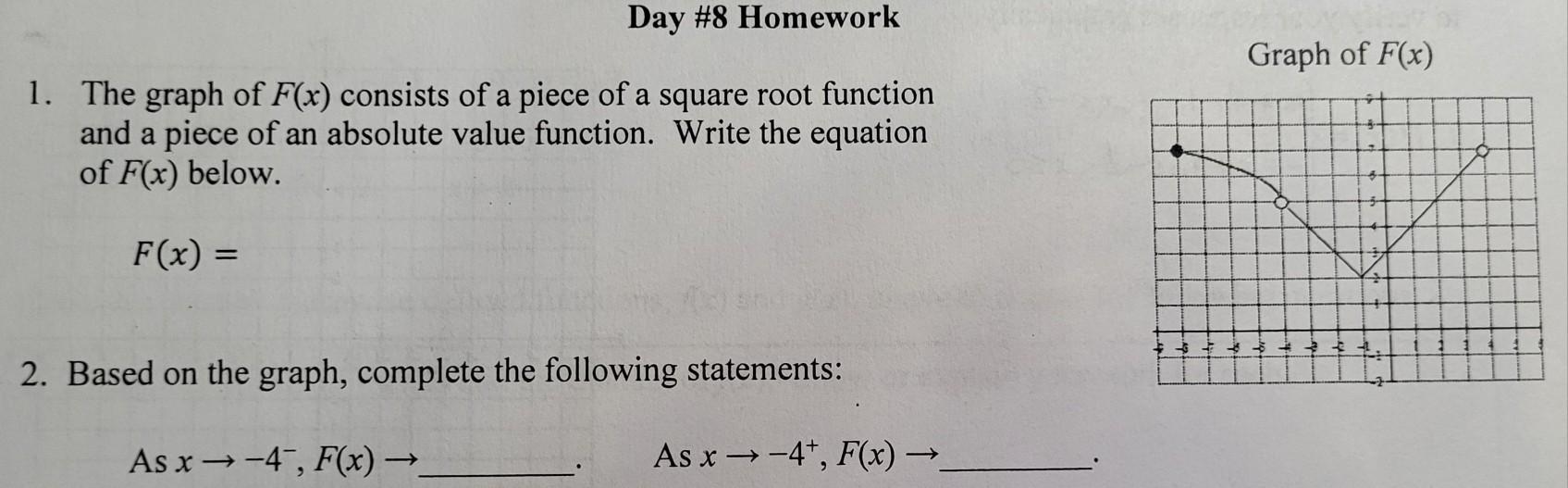
Answers
The equations for the Piece Wise Graph is:
F(x) = -1/8x² - 2x -1 -8 ≤ x < -4
(x + 1) + 2 -4 < x < 4
What is a Piece Wise Graph?A piecewise function is one that has several curve parts in its graph. It signifies that it has several meanings based on the input value. A piecewise function, in other words, performs differently depending on the input.
To derive the above equation,
Set a square root function y = ax² + bx + C
7 = 64a = 8b +c a = -1/8
5 = 16a - 4b + c b = -2
-b/2a = -8 c = -1
So y = -1/8 x² - 2x -1
Absolute Value Function:
Right: y = x + 3
so y = (x+1) + 2
F(x) = -1/8x² - 2x -1 -8 ≤ x < -4
(x + 1) + 2 -4 < x < 4
Thus the piece-wise functions are computed in turn.
Learn more about piece-wise functions:
https://brainly.com/question/16984860
#SPJ1
Help me with this stuff

Answers
Answer:
(-6,3]
Step-by-step explanation:
for domain just looks for the x axis, dont forget open and closed bracket by look types of dot
What is the length of segment TR?
Answers
Answer:
8 units
Step-by-step explanation:
PLEASE HELP!!
Find the area of the sector.

Answers
Answer:
B.
\( \frac{275\pi}{6} {m}^{2} \)
Step-by-step explanation:
\(area \: of \: sector \: is \\ \frac{the \: angle}{360} \times \pi {r}^{2} \\ hence \: we \: have \\ \frac{165}{360} \times {(10m)}^{2} = \frac{275\pi}{6} {m}^{2} \)
what do you think proof is
Answers
Answer:
proof is where you have something to explain/show what you saw to people when they don't believe you.
Almost sooo close guys just 5 more left sorry if it’s taking long

Answers
Answer:
As a decimal its 6.34, If you round up then its 6.35
As a fraction its 400/63
As a mixed number its 6 22/63
Look at the picture to answer.
Find the volume of the composite figure. Round the answer to the nearest tenth.
Volume = ____cm3

Answers
Answer:
around 433.1 cm
find the first shape's volume.
L x W x H method.
\(4 * 10 * 8 = 320cm\)
Length is eight, height is 4, and width is 10.
Now, the 2nd shape.
The formula of finding a sphere with a radius of 3 is V = 4/3\(pi^3\)
In that case, the answer should be an estimated 113.1
Add the two cm's together.
\(320 + 113.1 = 433.1\)
Please note that this is rounded to tenths place and estimated.
Please mark brainliest. Thanks! :)
First of all we will divide the figure in two shapes. One is hemisphere and other is a cuboid.
Volume of hemisphere:
\( \boxed{ \tt \:v = \frac{2}{3} \pi {r}^{3} }\)
Volume of cuboid:
\( \boxed{ \tt \: v = length \times breadth \times height}\)
\(\red{ \rule{35pt}{2pt}} \orange{ \rule{35pt}{2pt}} \color{yellow}{ \rule{35pt} {2pt}} \green{ \rule{35pt} {2pt}} \blue{ \rule{35pt} {2pt}} \purple{ \rule{35pt} {2pt}}\)
Volume of the hemisphere ⤵️r = 3 pi = 22/7\( \sf \dashrightarrow \: v = \frac{2}{3} \times \frac{22}{7} \times {3}^{3} \)
\( \sf \dashrightarrow \: v = \frac{2}{ \cancel3} \times \frac{22}{7} \times \cancel{27}\)
\( \sf \dashrightarrow \: v = 2 \times \frac{22}{7} \times 9\)
\( \sf \dashrightarrow \: v = \frac{396}{7} \)
\( \sf \dashrightarrow \: v = 56.6 \: {cm}^{3} \)
Volume of the cuboid ⤵️Length = 8cmBreadth = 10cmHeight = 4cm\( \bf \multimap \: v = 8 \times 10 \times 4\)
\( \bf \multimap \: v = 80 \times 4\)
\( \bf \multimap \: v = 320 \: {cm}^{3} \)
Now, Total volume ↯\( \rm \leadsto \: total \: volume = 56.6 + 320 \: {cm}^{3} \)
\( \rm \leadsto \: total \: volume = 376.6\: {cm}^{3} \)
If we round to the nearest tenth the total volume is ᭄
\( \rm \twoheadrightarrow volume = 380 \: {cm}^{3} \)
A square root function, that is stretched by a factor of 3, and shifted 4 units left and 6 units up.
Answers
Answer:
The square root function will turn to;
y = 3 √(x + 4) + 6
Step-by-step explanation:
Let the square root function be ;
y = √x
Now, let’s stretch this by a factor of 3
This means we are dilating by a factor of 3
Mathematically, this will be
y = 3 * √x = 3 √x
Shifting this 4 units up means that we are extending the x-axis value by 4 units
That will be ;
y = 3 √x + 4)
Now shifting this 6 units up, we finally will have;
y = 3 √(x + 4) + 6
Mrs. Arsenault need 14 cups of food for 7 days for her dog Apache. how much food will she need to feed him for 28 days (i dont get it)
Answers
Answer:
56 cups
Step-by-step explanation:
If Mrs. Arsenault's dog needs 14 cups per 7 days, then we simply need to find how many "groups of 7 days" are in 28, and then multiply by 14, since for each of these 7 days, 14 cups are needed.
Dividing 28 by 7 results in 4, which we then multiply by 14 to get 56 cups which will be needed for 28 days.
I attached a diagram that further illustrates this point.

A car travels 286 km in three hours with a constant speed. How far can It travel in four hours with the same speed?
Answers
Answer:
381.(3) [km.]
Step-by-step explanation:
if according to the condition distance=286 [km] per 3 hours, then it is possible to make up the equation:
\(\frac{286}{3}=\frac{d}{4};\)
and to calculate required distance 'd':
\(d=\frac{286*4}{3}=381.(3)[km].\)
Help please thank you!

Answers
Answer:
g(f(x)) = x
Yes, the functions are inverses.
Step-by-step explanation:
Hi there!
We're given:
\(f(x) = 8x-7\)
\(g(x)=\displaystyle\frac{x+7}{8}\)
To solve for g(f(x)), plug f(x) into g(x) as x:
\(g(f(x))=\displaystyle\frac{(8x-7)+7}{8}\\\\g(f(x))=\displaystyle\frac{8x-7+7}{8}\\\\g(f(x))=\displaystyle\frac{8x}{8}\\\\g(f(x))=x\)
Because g(f(x)) = x, the two functions are inverses.
I hope this helps!
help please, which pic is it a, b, c or d?

Answers
Answer:
top right graph
Step-by-step explanation:
Having a zero at 3 means that the y coordinate is 0 when the x-coordinate is 3.
A y-intercept of 9 means it includes the point (0, 9).
Answer: top right graph
By multiplying 5/3^4 by _________, we get 5^4
Answers
The missing Value, x, that when multiplied by 5/3^4 gives the result of 5^4 is 13125.
The missing value that, when multiplied by 5/3^4, gives the result of 5^4, we can set up the equation:
(5/3^4) * x = 5^4
To solve for x, we can simplify both sides of the equation. First, let's simplify the right side:
5^4 = 5 * 5 * 5 * 5 = 625
Now, let's simplify the left side:
5/3^4 = 5/(3 * 3 * 3 * 3) = 5/81
Now we have:
(5/81) * x = 625
To solve for x, we can multiply both sides of the equation by the reciprocal of 5/81, which is 81/5:
(81/5) * (5/81) * x = (81/5) * 625
On the left side, the fraction (81/5) * (5/81) simplifies to 1, leaving us with:
1 * x = (81/5) * 625
Simplifying the right side:
(81/5) * 625 = 13125
Therefore, the missing value, x, that when multiplied by 5/3^4 gives the result of 5^4 is 13125.
For more questions on Value.
https://brainly.com/question/843074
#SPJ8
Can someone please help me ASAP?? It’s due today!! I will give brainliest If It’s correct.

Answers
The correct option that indicates how Christa sliced the rectangular pyramid is the second option.
Christa sliced the pyramid perpendicular to its base through two edges.
What is a rectangular pyramid?A rectangular pyramid is a pyramid with a rectangular base and four triangular faces.
The height of the cross section indicates that the location where Christa sliced the shape is lower than the apex of the pyramid.
The trapezoid shape of the cross section of the pyramid indicates that the top and base of the cross section are parallel, indicating that Christa sliced the pyramid parallel to a side of the base of the pyramid, such that it intersects two of the edges of the pyramid
The correct option is therefore the second option;
Christa sliced the pyramid perpendicular to its base through two edges
Learn more on pyramids here: https://brainly.com/question/16098828
#SPJ1
find the area plz and thank u

Answers
Answer:
A = 173.82
Step-by-step explanation:
\(A = 2 (1 + \sqrt{2}) a^{2}\)
a = 6
substitute 6 for a
A = 2 (1 + \(\sqrt{2}\)) \(6^{2}\)
solve:
A = 2 (1 + \(\sqrt{2}\)) 36
A = 173.82
Answer:
Around 173.8 km (rounded to the nearest tenth of a km
Step-by-step explanation:
The area of an octagon can be found using the expression:
A=2(1+sqrt of 2)a^2
plug your side of 6 in to create the following equation:
A=2(1+sqrt of 2)(6^2)
Solve for A:
A= (2 + 2*sqrt of 2)(36) I multiplied 6 by 6 to get 36 and used distributive property on the other section.
A=72+72sqrt of 2
plug that in a calculator and you get around 173.8 km
Please Help!!! Geometry!

Answers
The correct missing statement and the reason is NP = NO + OP by Segment Addition Property
Given: MONP
Prove: MN = OP
The Segment Addition Property states that,
When three locations A, B, and C are on the same line and point B is located between points A and C, the length of the line segment AC is equal to the sum of the lengths of the three line segments AB and BC.
In mathematical notation, this property can be represented as:
AB + BC = AC
Statements Reasons
1. MO = NP 1. Given
2. MO = MN+ NO 2. Segment Addition Property
3. NP = NO + OP 3. Segment Addition Property
4. MN+NO = NO+ OP 4. Substitution Property
5. MN = OP 5. Subtraction Property of Equality
Hence the correct option is 2nd.
Learn more about Segment Addition Property click;
https://brainly.com/question/28772342
#SPJ1
djtdjdfdgdkdfhgg- math again

Answers
Answer:
All your answers are right. A and d are functions but b and c are not.
Step-by-step explanation:
The number less than zero are called
Answers
Answer:
Step-by-step explanation: Negatives because a number lower than 0 go like this -1 -2 -3 -4 -5 -6 -7 -8 -9 -10 see? so the answer is Negatives.
Solve the inequality. 5x-6≥9
Answers
Answer:
x ≥ 3
Step-by-step explanation:
5x-6≥9
put +6 on both sides to get rid of the -6
5x-6 +6 ≥ 9 +6
5x ≥ 15
x ≥ 3
Answer: x≥3
First, add 6 to both sides.
5x − 6 + 6 ≥ 9 + 6
5x ≥ 15
Then, Divide both sides by 5.
\(\frac{5x}{5} \geq \frac{15}{5}\)
Solve for x
A.3/4
B.52/7
C.17
D. 52/3

Answers
Answer:
at the picture or the a b c d ?