Two times W squared minus 2W plus 3 when w equals five
Answers
Answer:
Step-by-step explanation:
W2 - 2w + 3
Putting value when w = 5
(5)2 - 2(5) + 3
25 - 10 + 3
18
Related Questions
Jason is pulling a box across the room. He is pulling with a force of 10 newtons and his arm is making a 74 angle with the horizontal, what is the vertical component of the force he is pulling with?
Answers
Using vector concepts, it is found that the vertical component of the force is of 9.613 newtons.
-------------------------------------
A vector (x,y) has horizontal component x and vertical component y.The magnitude is \(M = \sqrt{x^2 + y^2}\)The components can be represented as function of the magnitude M and the angle between them \(\alpha\), that is, \((M\cos{\alpha}, M\sin{\alpha}\)-------------------------------------
The force is of 10 newtons, that is, M = 10.Angle of 74 degrees, that is, \(\alpha = 74\).The vertical component is y, thus:\(y = M\sin{\alpha} = 10\sin{74} = 9.613\)
The vertical component of the force is of 9.613 newtons.
A similar problem is given at https://brainly.com/question/24606756
The vertical component of the force Jason is pulling with is 9.613 N.
The vertical component of a force is given as :
\( F_{y} = Fsin\theta \)
Force, F = 10 N
θ = 74°
Therefore,
\( F_{y} = 10 \times sin74 \)
\( F_{y} = 10 \times 0.9612616 \)
\( F_{y} = 9.612 N \)
Therefore, the vertical component of the force is 9.613 N
Learn more : https://brainly.com/question/1454477?referrer=searchResults
Which choices are equivalent to the quotient below check all that apply. square root of 16 over square root of 8

Answers
To solve the quotient below;
\(\frac{\sqrt[]{16}}{\sqrt[]{4}}\)We simply both the numerator and the denominator as follows;
\(undefined\)PLEASEE help I’m really confused

Answers
Answer:
\(\frac{13}{\sqrt5}\)
Step-by-step explanation:
To get the shortest distance from a point to a line, we need to find a perpendicular line that intersects the line and also intersects the line. We know the slope of the line will be 1/2, but to find the y-intercept, we can just plug it into the point because we already know it will be on the line.
-3 = 1/2(-2)+c
-3 = -1+c
-2 = c
Now, we know the slope and the y-intercept, and since it will intersect the other line, we can set it equal to the other lines formula:
1/2x-2 = -2x+6
5/2x=8
x=16/5
Now, plugging this value of x into any of the equations, we can find the y-coordinate:
16/5(1/2)-2 = -2/5
We have the point (16/5, -2/5)
Now, we need to find the distance between (-2, -3) and (16/5, -2/5)
Plugging into distance formula:
\(\sqrt{(-2 - \frac{16}{5})^2+(-3-(-\frac{2}{5}))^2}\\= \sqrt{(-5.2)^2+(-2.6)^2}\\= \sqrt{33.8}\)
= \(=\frac{13}{\sqrt5}\)

Which number is closest to 1
A. 0.987
b. 0.111
c.0.561
d. 0.786
Answers
Answer:
Letter A
Step-by-step explanation:
Just think: which number would have the greatest value if it was rounded to the nearest hundredth
Answer: A
Step-by-step explanation:
I think the answer is correct becuase, If we taken all the numbers and put them on a number line they would all be under one. But when we look at the number line we would see that .987 is the closest because it is almost a whole number. And even if we did it without the number line what ever number we see that has the greatest digit and its in the negatives thats the closest to one. (I hope what I said makes sense!)
Hope This Helps!
Barkery’s Bakery sold 20 dog cakes during Week 1 of operation, 27 dog cakes during Week 2, and 34 dog cakes
during Week 3.
a. If this pattern continues, represent the first five
terms of the sequence using a table of values.
Answers
Answer:
Week 1 - 20
Week 2 - 27
Week 3- 34
Week 4 - 41
Week 5 - 48
Etc, etc,...
Step-by-step explanation:
The values increase by 7 each week.
1,-4,-9,-14,-19 what’s the 5th term
Answers
Answer:
-24
Step-by-step explanation:
goes by -5
Answer:
-24
Step-by-step explanation:
We are subtracting 5 each time
1-5 = -4
-4-5 = -9
-9-5=-14
-14-5=-19
-19-5 =-24
The fifth term is -24
Gonzalo bought ice cream for $2.29 and the sales tax was 5.5% What was the total
cost of the ice cream
Answers
Answer:
$2.41
Step-by-step explanation:
2.29+(2.29*(5.5/100))= 2.41
Plot the point (3,3)
Answers
Step-by-step explanation:
Plot the point (3,3):
this means where x = 3 and y = 3
Answer:

On a local sports team, 20% of 50 players are left handed. how many left handed players are om the team
Answers
Answer:
10
Step-by-step explanation:
In the derivation of the formula for the volume of a cone, the volume of the cone is calculated to be StartFraction pi Over 4 EndFraction times the volume of the pyramid that it fits inside. A cone is inside of a pyramid with a square base. The cone has a height of h and a radius r. The pyramid has a base edge length of 2 r. Which statement best describes where the StartFraction pi Over 4 EndFraction comes from in the formula derivation? It is the ratio of the area of the square to the area of the circle from a cross section. It is the ratio of the area of the circle to the area of the square from a cross section. It is the difference of the area of the square and the area of the circle from a cross section. It is the sum of the area of the square and the area of the circle from a cross section.
Answers
In the derivation of the formula for the volume of a cone, the volume of the cone is calculated to be D. the the sum of the area of the square and the area of the circle from a cross section.
How to illustrate the information?It should be noted that a volume simply means the amount of three dimensional space that's enclosed by a closed surface.
It is typically meaured on cubic units.
In this case, in the derivation of the formula for the volume of a cone, the volume of the cone is calculated to be the the sum of the area of the square and the area of the circle from a cross section.
In conclusion, the correct option is D.
Learn more about cone on:
brainly.com/question/28184308
#SPJ1
Answer:
B. It is the ratio of the area of the circle to the area of the square from a cross section.
Step-by-step explanation:
Completing the square to find the zeros 3. a^2+2a-3=0
Answers
Let's solve the equation by completing the square:
\(\begin{gathered} a^2+2a-3=0 \\ a^2+2a=3 \\ a^2+2a+(\frac{2}{2})^2=3+(\frac{2}{2})^2 \\ a^2+2a+1^2=3+1^2 \\ (a+1)^2=4 \\ \sqrt[]{(a+1)^2}=\sqrt[]{4} \\ \lvert a+1\rvert=2 \\ a+1=\pm2 \\ \text{then} \\ a+1=2 \\ a=2-1 \\ a=1 \\ or \\ a+1=-2 \\ a=-2-1 \\ a=-3 \end{gathered}\)therefore the solutions are a=1 and a=-3
PLEASE HELPP ASAPPP pleaseeeee

Answers
answer: 28561^2in the area involves multiplying by the 4 sides of the shaded square and the shaded is the colored square.
The perimter of a rectangle is 34 units. Its width is 6.5 units. Write an equation to determine the length (l) if the rectangle
Answers
Answer:
Step-by-step explanation:
P=2(w)+2(l)
34=2(6.5)+2(l)
34=13+2(l)
21=2(l)
10.5=l
X-3 + x + 3 gl call claim
Answers
2x
Explanation
Answer:
2
Step-by-step explanation:
Cómo resolver este problema matemático
El problema matemático es
−
3
+
+
3
x-3+x+3
x−3+x+3
Simplifica
1
Suma los números
−
3
+
+
3
+
2
Combina los términos semejantes
+
2
use the shell method to find the volume generated by revolving the shaded regions bounded by the curves and lines in exerciss 7-12about the y-axis
Answers
The answer is 1) V = \(2\pi\int\limits(2)+ {x} \, dx\); 2) V = \(2\pi \int\limits(1 - 2x) - 2x dx\); 3) V =\(2\pi \int\limits {\sqrt{2} } \, dx\) ; 4) V = \(2\pi\int\limits {\sqrt{(-2/2)(2-2)} \ dx\) .
1) The volume of the shell is then given by the product of the area of its curved surface and its height. The height is equal to 2 - (-2) = 4, and the radius is equal to the minimum of the distances from x = 2 to the two curves, which is x = 2 - () = 2 + . The volume of the solid is then given by the definite integral:
V = \(2\pi\int\limits(2)+ {x} \, dx\) = \(2\pi [(/3) + 2x]\) evaluated from 0 to 1 = (4/3)π.
2) The height of the region is equal to - (2x) = -2x, and the radius is equal to the minimum of the distances from x = 1 to the two curves, which is x = 1 - (2x) = 1 - 2x. The volume of the solid is then given by:
V = \(2\pi \int\limits(1 - 2x) - 2x dx\)=\(2\pi [/5 - 2/3 + /2]\) evaluated from 0 to 1 = (8π/15).
3) The height of the region is equal to (2-x) - = 2-x. The radius is equal to the minimum of the distances from x = 0 to the two curves, which is x = The volume of the solid is then given by:
V =\(2\pi \int\limits {\sqrt{2} } \, dx\) = \(2\pi [(x^4/4)]\) evaluated from 0 to √2 = (π/2).
4) The height of the region is equal to () - (2-) = 2 - 2. The radius is equal to the minimum of the distances from x = 0 to the two curves, which is x = √((2-)/2). The volume of the solid is then given by:
V = \(2\pi\int\limits {\sqrt{(-2/2)(2-2)} \ dx\) = \(4\pi [(2/3)\± (2\sqrt{2} /3)]\)
The complete Question is:
Use the shell method to find the volumes of the solids generated by revolving the regions bounded by the curves and lines in about the
1. y = x, y = -x/2, and x = 2
2. y = 2x, y = x/2, and x = 1
3. y = x/2, y = 2-x, and x = 0
4. y = 2-x/2, y = x/2, and x = 0
To know more about Shell Methods:
brainly.com/question/17074517
#SPJ4
Three coins are tossed. Let the event T = all Tails and the event k = at least one Tails. What is the probability that the
outcome is all tails is at least one coin shows a tails?
A. 1/8
B. 1/7
C.7/8
D.7/64
Answers
Answer:
1/8
Step-by-step explanation:
If three coins are tossed, you have a 50% chance that 1 coin will land on Tails. You will have a 25% chance that 2 coins will land on Tails. You will have a 12.5% chance that 3 coins will land on Tails.
Solve for the unknown variable. -7x + 5 = 61
A) -66/7
B) 8
C) -8
D) 66/7
Answers
Answer:
C, -8
Step-by-step explanation:
Subtract 5 from both sides then divide -7 to both sides to get X = -8
the equation 4(x+9)=76 models this situation which choice shows the sequence of operations neede to slove this problem using both arithmetic and algebra
Answers
Answer:
Step-by-step explanation:
Here you go mate
Use PEMDAS
Parenthesis,Exponent,Multiplication,Division,Addition,Subtraction
Step 1
4(x+9)=76 Equation/Question
Step 2
4(x+9)=76 Remove parenthesis by multiplying 4
4x+36=76
Step 3
4x+36=76 Subtract 36 from sides
4x=40
Step 4
4x=40 Divide sides by 4
answer
x=10
Hope this helps
How many times greater is the value of the 6 in 868,171 than the value of the 6 in 886,171
Answers
Which is the correct equation to use to find the *
60 PTS!
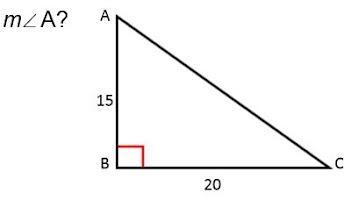

Answers
Answer:
B. m∠A = \(tan^{-1}(\frac{20}{15} )\)
Step-by-step explanation:
Angle A has opposite side = 20 and adjacent side = 15
Using sohcahtoa method,
\(\frac{opposite}{adjacent} = tan(A)\)
\(\frac{20}{15} = tan(A)\)
\(tan^{-1}(\frac{20}{15} ) = A\) .....this is the answer.
m∠A = 53.1° .....angle m∠A

find slope of the graph 9x-3y=15
Answers
\(y = mx + b\)
where m = slope and b = y-intercept.
What we have to do is to make the y-term as the subject of equation.
\(9x - 3y = 15 \\ 9x - 15 = 3y \\ \frac{9x - 15}{3} = y\)
Simplify\( \frac{9x}{3} - \frac{15}{3} = y \\ 3x - 5 = y \\ y = 3x - 5\)
From y = mx+b, the slope is 3.
Answer\( \large \boxed {slope = 3}\)
choose the correct top, front, and side views for this object and labeled each one.

Answers
Top, D
Front, A
Side, B
1) Looking at that figure, we can state that:
Top:
Note that this a 2 Dimensional representation, so the top view of this object is the shape D
Front
Looking to the front of that shape we can see that the shape that fits this 2D representation is A
Side
And finally, the side views of this object has to have a height of two squares, so the side view is given in B
And that is the answer
the managers of an airline analyzed a random sample of flights and found that 95% of the flights arrived on time.

Answers
From the information given,
probability that a flight arrives on time = 95% = 95/100 = 0.95
Probability that a flight will not arrive on time = 1 - 0.95 = 0.05
Given 480 scheduled flights,
number of flights that will not arrive on time = 0.05 x 480 = 24
The best estimate of the number of these flights that will not arrive on time = 24
Help me please…………………….

Answers
Answer:
8.7
Step-by-step explanation:
If you like my answer mark me brainliest
The equation 320=x+7.50(36) represents the cost of ordering potted plants at a second store.
What does the x represent in this situation?
Answers
Answer:
I would say it's the amount of potted plants.
Step-by-step explanation:
x is the independent variable and y is the dependent variable. Y counts on x, so total cost counts on the amount of potted plants.
Find the unit rate (constant of proportionality) of the distance traveled.
Number of hours
0.25 1.5 2.5 3
Distance traveled (km) 3 18 30 36
Answers
Answer:
12.
Step-by-step explanation:
if to re-write the given condition, then
\(\frac{3}{0.25} =\frac{18}{1.5} =\frac{30}{2.5} =\frac{36}{3} ;\)
it is clear, the required constant is 12 (12 per hour).
Hey can someone help me with this plzzzzz

Answers
Answer:
Step-by-step explanation:
41 + 17 = 58
consider a two-factor factorial design with three levels for facts a, three levels for factor b, and four replicates in each of the nine cells
a. how many degrees of freedom are there in determining the A variation and the factor B variation
b. how many degrees of freedom are there in dreaming the interaction variation
c. how many degrees of freedom are there in determining the random variation
d. how many degrees of freedom are there in determining the total variation
Answers
In calculating the factor A variation, there are two degrees of freedom. In determining the variation of factor B, there are two degrees of freedom.
What is a two-factorial design?A two-factor factorial design is an experiment that collects data for all potential values of the two factors of the study. The design is a balanced two-factor factorial design if equivalent sample sizes are used for every of the possible factor combinations.
Suppose we have two components, A and B, each of which has a high number of levels of interest. We will select a random level of component A and a random level of factor B, and n observations will be taken for each experimental combination.
From the data given:
a.
In calculating the factor A variation, there are two degrees of freedom.
In determining the variation of factor B, there are two degrees of freedom.
b.
Finding the degree of freedom using the interaction variation, there are four degrees of freedom.
c.
In finding the random variable, there are 9(4-1) = 27 degrees of freedom.
d.
In calculating the total variable, there are 9*4-1 =35 degrees of freedom.
Learn more about using a two-factor factorial design for determining the degree of freedom here:
https://brainly.com/question/14292694
#SPJ1
Find the area under the standard normal curve between z = -1.5 and z = 2.5.
Answers
Answer:
0.1359
step by step explanation
The area under the standard normal curve between z = -1.5 and z = 2.5 should be considered as the 0.9270.
Calculation of the area:Since
p value for z= -1.5 is 0.066807
p value for z= 2.5 is 0.99379
Now
area under the standard normal curve between z = -1.50 and z = 2.50. should be
= 0.99379-0.066807
= 0.926983
= 0.9270
hence, The area under the standard normal curve between z = -1.5 and z = 2.5 should be considered as the 0.9270.
Learn more about an area here: https://brainly.com/question/19461476
The first three terms of an arithmetic sequence are x, 2x + 4, 5x. Find the value of x.

Answers
The value of x for the given arithmetic sequence is x = 4.
What is an arithmetic sequence?A series of numbers called an arithmetic progression or arithmetic sequence has a constant difference between the terms. An arithmetic progression with a common difference of 2 is found, for instance, in the numbers 5, 7, 9, 11, 13, and 15.
Given,
First three terms of an arithmetic sequence are x, 2x + 4, 5x
The difference of 1 and 2 term equals the difference of 3 and 2 term.
⇒ 2x + 4 - x = 5x - 2x - 4
⇒ x + 4 = 3x - 4
⇒ 2x = 8
⇒ x = 4
To learn more about arithmetic sequence from given link
https://brainly.com/question/6561461
#SPJ9