vă rog am nevoie de ajutor la ex 6 dacă il faceți dau corana ex este de clasa a 6

Answers
Answer:
sry but i can't help you with it bcz i don't understand spanish can u write it in clear english
Sry
Related Questions
Please help me on this question!

Answers
Based on the information presented, one cup measures a total of 14 centimeters.
How to know the measure of one cup?To discover this, let's start by analyzing the information provided:
2 cups = 16 centimeters4 cups = 20 centimetersThis means that by adding 2 cups, the total height increases by 4 centimeters, and therefore if you add one cup this will increase by 1 centimeter.
Based on this idea, if two cups are 16 cm, let's calculate the height of one cup:
\(16 centimeters -2 centimeters = 14 centimeters\)
Based on the previous information one cup is 14 centimeters.
Learn more about height in https://brainly.com/question/29131380
#SPJ1
g (x) = 3x + 12 for x = 5
Answers
We will investigate how to evaluate a given function as per the indicated value of the independent variable.
A function is defined as a relationship between two variables ( x and y ). One of these variables mostly ( x ) is denoted as an independent quantity and ( y ) as the independent quantity.
A unique mathematical relationship is expressed between the two variables. Where, the independent variable ( x ) serves as an input to the function. The function is then evaluated on the basis of mathematical manipulation to give the output ( y ).
We have a function g ( x ) defined as follows:
\(g\text{ ( x ) = 3x + 12}\)We are to evaluate the above function for the indicated value of the input ( independent variable ) - x as follows:
\(x\text{ = 5}\)The process of function evaluation always start with the substitution of the indicated value of independent variable ( x ) into the function relation as follows:
\(g\text{ ( 5 ) = 3}\cdot(5)\text{ + 12}\)Then we go about applying the basic mathematical operation defined in the relationship expressed. Keeping in mind the order in which each mathematical operator is to be performed i.e PEMDAS rule!
We will go ahead and solve our parenthesis and evaluate the function g ( x ) at x = 5 as follows:
\(\begin{gathered} g\text{ ( 5 ) = 15 + 12} \\ g\text{ ( 5 ) = 27} \end{gathered}\)For the indicated value of the independent variable ( x = 5 ) the function g ( x ) outputs the the value of:
\(27\ldots\text{ Answer}\)
HELPP ME PLEASEEEE!!!!
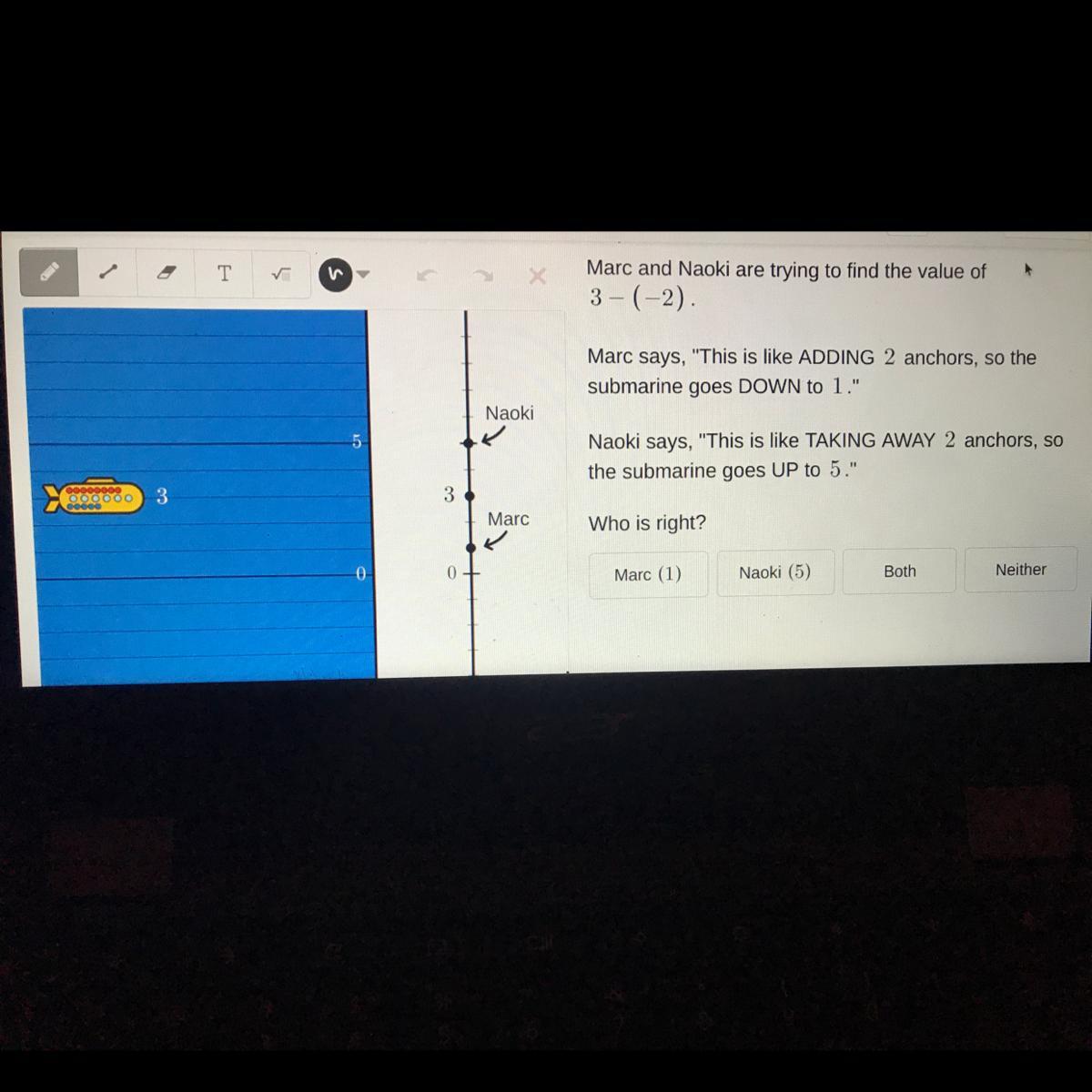
Answers
Answer 5
when the relationship between the independent variable and dependent variable changes depending on the level of another variable, that other variable is called a
Answers
When conducting studies to examine cause-and-effect relationships, researchers frequently modify or measure independent and dependent variables.
what is independent variable ?It is a variable that is independent of the other factors you are attempting to assess. Other elements (such as what they consume, how much they attend school, and how much television they watch) won't alter a person's age.
given
Its worth is unrelated to other study variables. The effect serves as the dependent variable. The independent variable's modifications determine its value.
When conducting studies to examine cause-and-effect relationships, researchers frequently modify or measure independent and dependent variables.
To know more about independent variable visit :-
https://brainly.com/question/29430246
#SPJ4
los ladrillos de una balanza está en equilibrio pesan todos lo mismo en un en un lado de la balanza hay 5 kg y 9 ladrillos en el otro hay 22 kilogramos y 4 ladrillos Cuánto pesa un ladrillo
Answers
The brick weighs 3.4 kilograms.
Define the term Quantities?Quantities are values that can be measured or counted. They are typically represented by numerical values and units of measurement.
Let's assume that the weight of each brick is the same. Let's also denote the weight of each brick by "w" (in kilograms).
On one side of the scale, we have 9 bricks and 5 kilograms. So the total weight on this side is:
Total weight = (9 x w) + 5
On the other side of the scale, we have 4 bricks and 22 kilograms. So the total weight on this side is:
Total weight = (4 x w) + 22
Since the bricks on the scale are in balance, the total weight on both sides must be equal. Therefore, we can set the two expressions for total weight equal to each other and solve for w:
(9 x w) + 5 = (4 x w) + 22
Expanding and simplifying the equation, we get:
5 x w = 17
Therefore, the weight of each brick is:
w = 17 / 5
w = 3.4 kilograms
So each brick weighs 3.4 kilograms.
To know more about equation, visit:
https://brainly.com/question/2228446
#SPJ1
In the standard (x,y) coordinate plane, what is the distance, in coordinate units, between (−3,−2) and (5,5)?
Answer choices:
A. √13
B. √15
C. √113
D. 5
E. 15
Answers
To find the distance between two points in the coordinate plane, we can use the distance formula: Distance = √[(x2 - x1)^2 + (y2 - y1)^2].So the correct answer is C. √113.
Let's apply this formula to the given points in the coordinate plane (-3, -2) and (5, 5):
Distance = √[(5 - (-3))^2 + (5 - (-2))^2]
= √[(8)^2 + (7)^2]
= √[64 + 49]
= √113
Therefore, the distance between two points in the coordinate plane (-3, -2) and (5, 5) is √113. Others options A. √13 B. √15 D. 5 E. 15
So the correct answer is C. √113.
To learn more about distance formula click here : brainly.com/question/25841655
#SPJ11
A probability is a numerical value that indicates the chance, or likelihood, of a specific event occurring.
Select one:
True
False
Answers
This statement is true
A probability is a numerical value that indicates the chance, or likelihood, of a specific event occurring. Probability is the measure of the likelihood of a random event happening, and it is expressed as a number between 0 and 1, with 0 implying that the event would never occur and 1 implying that it is certain to happen.
The probability of an event happening is referred to as its likelihood. Probability can be expressed as a fraction, percentage, or decimal, and it is a vital tool in statistics. It is frequently utilized in mathematics to estimate real-world situations that involve random variables such as coin flips, weather patterns, stock market trends, and even sporting events.
Learn more about probability
https://brainly.com/question/31828911
#SPJ11
the event containing the outcomes belonging to a or b or both is the __________ of a and b.
Answers
The event containing the outcomes belonging to a or b or both is the union of a and b.
Let us consider two sets a and b, and say events or the elements in set a are: (x, y, z), and the elements in set b are: (o, p, q)
The union of these two sets will give:
aUb=(x, y, z, o, p, q)
Now, a union of two sets (denoted by U), for our case a and b, is the set or event containing the elements belonging to a or b or both the elements in a and b altogether.
Thus, the even containing the outcomes belonging to a or b or both in a and b altogether is the union of a and b.
Learn more about union of a set here:
https://brainly.com/question/23337676
#SPJ4
Solve a system.
|x-3|+|x+3|=6
x^2>1
Answers
Answer:
[-3,3]
Step-by-step explanation:
if f(x) = 2x + 7, what is the value of f(-10) and f(5)?
Answers
if f(x)= 2x+7
f(x)= two points
-10 and +5
the solution is : A,,,,f(-10)=2(-10)+7
= -20+7
= -13
and
B,,,,, f(5)= 2(5)+7
= 10+7
= 17
Give the sum in simplest form: 2x/w + y/w
Answers
Answer:
\(\frac{2x+y}{w}\)
3(x - 5) + 6(x+1)=9
Answers
Answer:
x=2
Step-by-step explanation:
plz mark as brainliest

help me with this please..!

Answers
Answer:
\(y \geq -4\)
Step-by-step explanation:
This is because the lowest the y-coordinate goes to is -4.
Write a formula for a quadratic function with the indicated characteristics. Has horizontal intercepts at t = 3 and t = 4 and graph containing the point (1, -3)
Answers
Answer:
\(\text{ y = -}\frac{1}{2}(t^2-7t+12)\)Explanation:
Here, we want to write the equation of the quadratic function
We have the general form as:
\(\text{ y = ax}^2\text{ + bx + c}\)from the question, we have the horizontal intercepts at t= 3 and t =4
that means (t-3) is a factor and also (t-4) is another root of the equation
The product of these two is as follows:
\(\text{ a(t-3)(t-4) = a(t}^2-7t\text{ + 12)}\)Finally, we need to find the value of a
We can do this by making a substitution
We substitute 1 for t and -3 on the other side of the equation
Mathematically, we have this as:
\(\begin{gathered} \text{ a(1-7+12) = -3} \\ 6a\text{ = -3} \\ a\text{ = }\frac{-3}{6}\text{ = -}\frac{1}{2} \end{gathered}\)Thus, we have the equation as:
\(\text{ y = -}\frac{1}{2}(t^2-7t+12)\)
2
3
6 7 8 9 10
Question
Set up and solve a system of equations to solve the problem.
A jar contains n nickels and d dimes. There are 20 coins in the jar, and the total value of
the coins is $1.30. How many nickels and how many dimes are in the jar?
The jar contains
nickels and
dimes.
Answers
9514 1404 393
Answer:
14 nickels6 dimesStep-by-step explanation:
The system of equations can be written ...
n + d = 20 . . . . . . . . coins in the jar
5n +10d = 130 . . . . . value in cents
_____
Using the first equation, we can write an expression for n:
n = 20 -d
We can substitute this into the second equation:
5(20 -d) +10d = 130
100 +5d = 130 . . . . . . simplify
5d = 30 . . . . . . . . . . . . subtract 100
d = 6 . . . . . . . . . . . . divide by 5
n = 20-d = 14
The jar contains 14 nickels and 6 dimes.
What fraction is missing from the
following equation?
1 -
1-
-
=
+
8
moo
(A)
(В)
+ Amloom-00100
(D.
oloo

Answers
First, we make the denominators of 1/4 and 3/8 the same as the LCM (= 8)
-> 1/4 = 1 x 2 / 4 x 2 = 2/8
-> 1 - ... = 2/8 + 3/8 = 5/8
The missing fraction = 1 - 5/8 = 3/8.
3 years ago, you received a gitt of 10000 and you want to spend it in 3 years. How much will it be worth? Assume the interest rate is 4%.
$12,986.16
$12,653.19
$12,536.23
Answers
If you received a gift of $10,000 3 years ago and you want to spend it in 3 years with interest rate is 4%, it will be worth $12,653.19. Option b is correct.
To calculate the future value of a present sum after a specified period, we can use the formula for compound interest:
Future Value = Present Value * (1 + Interest Rate)ᴺ
In this case, the present value is $10,000, the interest rate is 4% or 0.04, and the number of periods is 6 years because you received the gift 3 years ago and want to spend it in 3 years.
Using the formula:
Future Value = \(\$10,000 * (1 + 0.04)^6\)
Future Value = \(\$10,000 * (1.04)^6\)
Future Value = $10,000 * 1.1265319
Future Value ≈ $12,653.19
Therefore, the amount will be approximately $12,653.19. Option b is correct.
Learn more about interest rate https://brainly.com/question/28236069
#SPJ11
I Need help asap please

Answers
Answer:
15. 7.71
16. 102.83
17. 1.4
18. 19
19. a. week one: 23
week two: 47
b. About two times greater
Step-by-step explanation:
15 & 16 ; to find the perimeter of a semicircle it is r(π + 2)
17 & 18; to find radius from circumference it is \(r=\frac{C}{2\pi }\)
19 a: to find diameter from circumference it is C ÷ π
help me I need to make sure I got the correct answer.

Answers
Answer:
I dont know pls ask you parents...
Step-by-step explanation:
I dont know
Question 9 (5 points)
(05.05 LC)
A bus company charges a fixed amount plus a fee based on the distance traveled. The cost chart for the company is shown:
Cost Chart
Distance traveled (miles) (x) Cost (dollars) (y)
0
4
1
9
2
14
What is the fixed amount charged? (5 points)
$4
Oь
$5
$10
512
Answers
Answer:
The Answer is $4 or option (A)
Step-by-step explanation:
I took the test :)
Simplify. (Use the form a + bi.)
3 − 6i
−7i
Answers
Answer:
3 - 13i
Step-by-step explanation:
3 - 6i - 7i
3 - 13i
A set of cubes, each with sides 1 cm long, is stacked 5 cubes high, 8 cubes deep and 7 cubes long.
How many cubes are in the set?
Answers
Answer:
280 Cubes
Explanation:
This problem has to do with volume. To find the number of cubes in the set, you multiply the length, width, and height of all the cubes. This would look like 7*8*5. This equals 280 cubes.
45% of the population of a town are men and 35% are women. If the number
of children is 30,000, find the number of men.
Answers
Answer:
67,500 men
Step-by-step explanation:
Percent of children = 20% (.2)
30,000 x 5 = 150,000 (Total pop.)
150,000-30,000 = 120,000 (Pop. minus children)
.35 (Percent of women) x 150,000 =52,500
120,000-52,500 = 67,500
Answer:
67,500
Step-by-step explanation:
20 percent of the population is children. 45 percent is men. If children population is 30000, you multiply by 2 to get 40 percent. That means that if men were 40 percent, men population would be 60000. When you add another 5 percent to get 45 percent, it makes 67500 men.
Dock diving is a great form of athletic competition for dogs of all shapes and sizes. Sheba, the American Pit Bull Terrier, runs and jumps off the dock with an initial speed of 8.72 m/s at an angle of 28
Answers
Sheba's initial horizontal velocity (Vx) is approximately 7.81 m/s, and her initial vertical velocity (Vy) is approximately 4.09 m/s.
To analyze Sheba's motion during dock diving, we can break down her initial velocity into horizontal and vertical components.
Given an initial speed of 8.72 m/s and an angle of 28 degrees, we can calculate the horizontal and vertical velocities using trigonometry.
The horizontal velocity (Vx) can be found using the cosine function:
Vx = V * cos(angle)
Vx = 8.72 m/s * cos(28 degrees)
Vx ≈ 7.81 m/s
The vertical velocity (Vy) can be determined using the sine function:
Vy = V * sin(angle)
Vy = 8.72 m/s * sin(28 degrees)
Vy ≈ 4.09 m/s
So, Sheba's initial horizontal velocity (Vx) is approximately 7.81 m/s, and her initial vertical velocity (Vy) is approximately 4.09 m/s.
For such more questions on velocity
https://brainly.com/question/29523095
#SPJ8
How can i show that p^(q-1) + q^(p-1) = 1 (mod pq)?
Answers
Step-by-step explanation:
you can just put in some values to check.
I actually used p =2 and q=3
the It will be
2^3-1 + 3^2-1 = 1 (mod 2×3)
2^2 +3^1 = 1 (mod 6)
4+3= 1 (mod6)
7= 1 (mod6)
which is true.
therefore p^(q-1) + q^( p-1) = 1 ( mod pq) is true
To show that p^(q-1) + q^(p-1) = 1 (mod pq), we can use Fermat's Little Theorem, which states that if p is a prime number and a is an integer not divisible by p, then a^(p-1) = 1 (mod p). Using this theorem, we can first show that p^(q-1) = 1 (mod q), since q is a prime number and p is not divisible by q. Similarly, we can show that q^(p-1) = 1 (mod p), since p is a prime number and q is not divisible by p.
Therefore, we can write:
p^(q-1) + q^(p-1) = 1 (mod q)
p^(q-1) + q^(p-1) = 1 (mod p)
By the Chinese Remainder Theorem, we can combine these two equations to obtain:
p^(q-1) + q^(p-1) = 1 (mod pq)
Thus, we have shown that p^(q-1) + q^(p-1) = 1 (mod pq).
We'll use Fermat's Little Theorem to show that p^(q-1) + q^(p-1) = 1 (mod pq).
Fermat's Little Theorem states that if p is a prime number and a is an integer not divisible by p, then:
a^(p-1) ≡ 1 (mod p)
Step 1: Apply Fermat's Little Theorem for p and q:
Since p and q are prime numbers, we have:
p^(q-1) ≡ 1 (mod q) and q^(p-1) ≡ 1 (mod p)
Step 2: Add the two congruences:
p^(q-1) + q^(p-1) ≡ 1 + 1 (mod lcm(p, q))
Step 3: Simplify the congruence:
Since p and q are prime, lcm(p, q) = pq, so we get:
p^(q-1) + q^(p-1) ≡ 2 (mod pq)
In your question, you've mentioned that the result should be 1 (mod pq), but based on Fermat's Little Theorem, the correct result is actually:
p^(q-1) + q^(p-1) ≡ 2 (mod pq)
To learn more about integer : brainly.com/question/15276410
#SPJ11
each employee of company z is an employee of either division x or division y, but not both. if each division has some part-time employees, is the ratio of the number of full-time employees to the number of part-time employees greater for division x than for company z?
Answers
Ratio in X > Ratio in Z> Ratio in Y
lets assume: -
Total employees in Z = 50, Total Full Time = 30, Total Part Time = 20 Ratio in Z = 30/20 = 3/2
So, No. of Full time employees in X > 15(more than half) lets assume 18,
Also No. of part time employees in Y > 10(more than half). lets assume 12
So the no. of part time employees in X =8 Hence ratio in X = 18/8 = 9/4 >2
Similarly ratio in Y = 12/12 = 1
So Ratio in X > Ratio in Z > Ratio in Y
But we don't know the distribution of Full time Vs Part time employees in Z, X& Y
So lets flip to the no of F.T in Z = 20 and P.T = 30 Now Ratio in Z = 20/30 = 2/3
So No. F.T in X > 10 (Assume 12) so F.T in Y = 8
and P.T in Y > 15 (Assume 18) so P.T in X = 12
Ratio in X = 12/12 = 1
Ratio in Y = 8/18 = 4/9
Ratio in X = 2/3
Ratio in X > Ratio in Z> Ratio in Y
To learn more about Ratio:
https://brainly.com/question/13419413
#SPJ4
Ayden filled up hi car with ga before embarking on a road trip acro the country. The car ue 2 gallon of ga for every hour driven, and after driving for 4 hour, there were 4 gallon of ga left in the tank. Write an equation for G,G in term of T,T, repreenting the number of gallon of ga remaining in Ayden ga tank after TT hour of driving
Answers
Equation for G, representing the number of gallons of gas left after ‘T’ hours will be: G = 12 - 2T
Let G be the total number of gallons of gas left
And t be the time in hours
Since, car uses 2 gallons of gas in 1 hours
Therefore, total gallons of gas used in 4 hours will be 2 x 4 = 8 gallons
Total gallons of gas left after 4 hours = 4 gallons
Therefore, total gallons of gas before embarking on a road trip = 8 + 4 = 12 gallons
In general from the unitary method, number of gallons of gas used in time ‘T’ will be 2T
Therefore, Total gallons of gas left = (Total gallons of gas embarked on a road trip) - ( Total gallons of gas used in the trip at time ‘T’ )
From the above conclusions, we can write
G = 12 - 2T
To know more about the equation on unitary method, visit here:
https://brainly.com/question/23423168
#SPJ4
according to the world health organization (who) child growth standards, the head circumference for boys at birth has a normal probability distribution with a mean of 34.5cm and a standard deviation of 1.3cm. what is the head circumference of a newborn boy who marks the start of the 75th percentile? enter a number without units.
Answers
The head circumference of a newborn boy who marks the start of the 75th percentile is approximately 35.38 cm.
To find the head circumference of a newborn boy who marks the start of the 75th percentile, we need to first find the z-score corresponding to the 75th percentile using the standard normal distribution.
The z-score formula is
z = (x - μ) / σ
where x is the observed value, μ is the mean, and σ is the standard deviation.
To find the z-score that corresponds to the 75th percentile, we need to look up the z-score associated with a cumulative area of 0.75 under the standard normal distribution curve. This value can be found using a table or calculator and is approximately 0.674.
Now we can use the formula for the z-score to solve for the head circumference of a newborn boy at the 75th percentile
z = (x - μ) / σ
0.674 = (x - 34.5) / 1.3
0.674 × 1.3 = x - 34.5
0.8762 + 34.5 = x
x = 35.3762
x ≈ 35.38 cm
Learn more about z score here
brainly.com/question/15016913
#SPJ4
Find the average value fave of the function f on the given interval. f(x) = 7 sin(4x), [−, ]
Answers
The average value fave using the formula fave = (1 / (b - a)) ∫[a,b] 7 sin(4x) dx. The definite integral of f(x) over the interval [a, b] is:
∫[a,b] 7 sin(4x) dx = -7/4 [cos(4x)] [from a to b]
To find the average value fave of the function f(x) = 7 sin(4x) on the given interval, we need to calculate the definite integral of the function over the interval and then divide it by the length of the interval.
The given interval is specified as [−, ], where the lower and upper limits are missing. To proceed with the calculation, we need the specific values for the lower and upper limits of the interval. Please provide the missing values so that we can compute the average value of the function.
Once we have the interval limits, we can calculate the definite integral of f(x) = 7 sin(4x) over that interval. The integral of sin(4x) with respect to x is evaluated as -cos(4x) / 4. Therefore, the definite integral of f(x) over the interval [a, b] is:
∫[a,b] 7 sin(4x) dx = -7/4 [cos(4x)] [from a to b]
Next, we need to find the length of the interval, which is given by b - a.
Finally, we can compute the average value fave using the formula:
fave = (1 / (b - a)) ∫[a,b] 7 sin(4x) dx
By plugging in the specific values for a, b, and evaluating the definite integral, we can calculate the average value fave of the function f(x) over the given interval.
Please provide the missing values for the interval, and I'll be able to assist you in finding the average value fave in a more specific manner.
Learn more about definite integral here
https://brainly.com/question/31271414
#SPJ11
Kendall has to identical toy lizard
Answers
We know that
• The probability of landing on its belly is 3/5.
,• The probability of landing on its back is 2/5.
Given that the events are independents, then we have to multiply their probabilities of landing on their backs.
\(P=\frac{2}{5}\cdot\frac{2}{5}=\frac{4}{25}\)Hence, the answer is A.