What are outliers? Describe the effects of outliers on the mean, median, and mode.
a. An outlier in a data set is a value that is much higher than almost all other values. An outlier can change the median of a data set, but does not affect the mean or mode.
b. An outlier in a data set is a value that is much higher or much lower than almost all other values. An outlier does not change the mean, median, or mode of a data set.
c. An outlier in a data set is a value that is much higher than almost all other values. An outlier can change the mode of a data set, but does not affect the mean or median.
d. An outlier in a data set is a value that is much higher or much lower than almost all other values. An outlier can change the mean of a data set, but does not affect the median or mode.

Solution

From given equation

Slope = -2

and y-intercept is (0,0)

Therefore,

if we put x= 0

then y = -2*0 = 0

if we put x = 1

then y = -2*1 = -2

for graph plotting, see the attachment.

To learn more about the coordinate plane, refer to https://brainly.com/question/25042607

#SPJ1

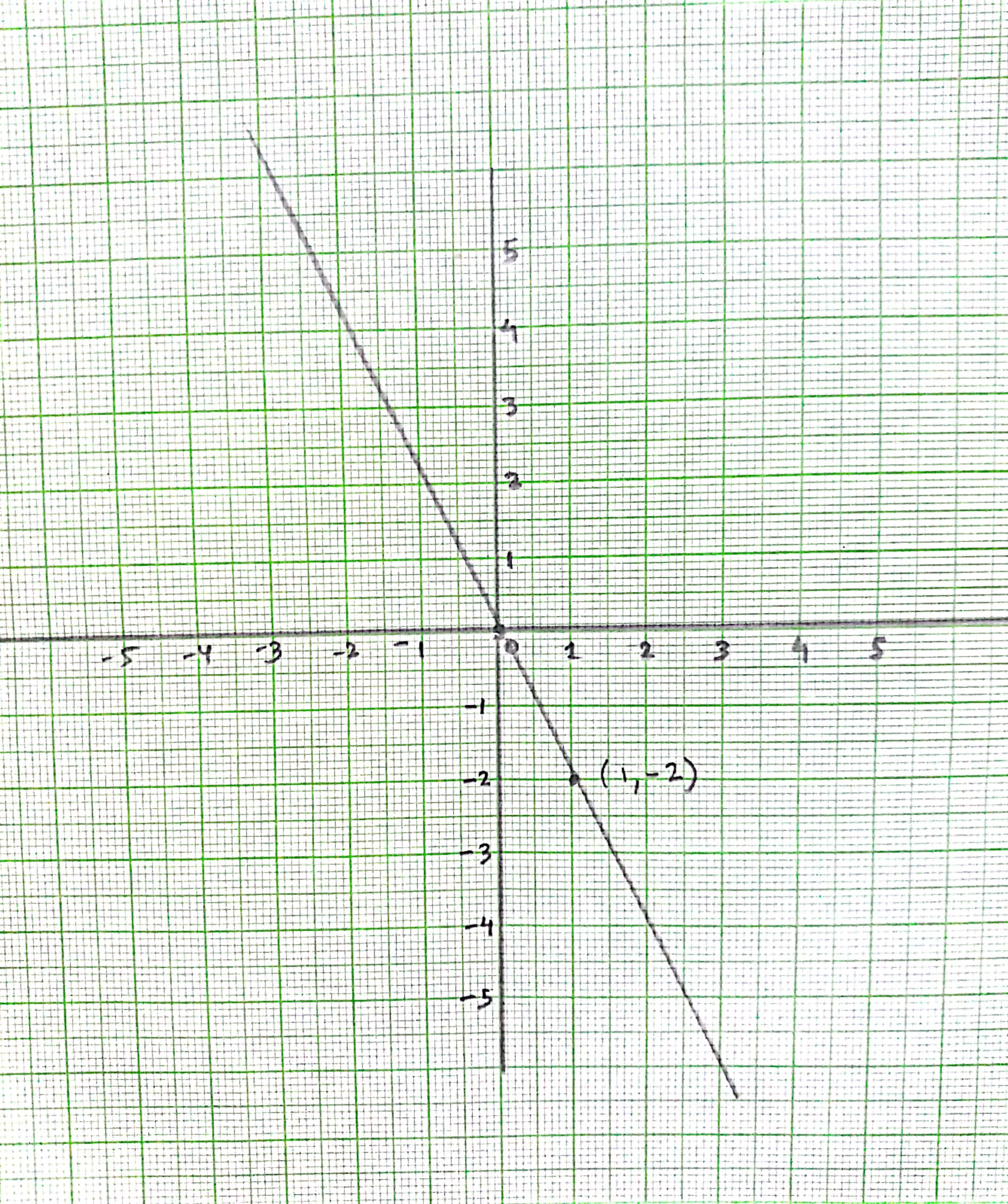
Solution

From given equation

Slope = -2

and y-intercept is (0,0)

Therefore,

if we put x= 0

then y = -2*0 = 0

if we put x = 1

then y = -2*1 = -2

for graph plotting, see the attachment.

To learn more about the coordinate plane, refer to brainly.com/question/25042607

#SPJ1

Answer:

Greetings!!!

Converse:- MELTS IN YOUR HAND NOT IN YOUR MOUTH!

Inverse:- DOESN'T MELT IN YOUR HAND BUT MELTS IN YOUR HAND!

Contrapositive:- MELTS IN YOUR HAND NOT IN YOUR MOUTH!

Hope it helps!!!

To prove the given statements, we will use the triangle inequality property, which states that for any real numbers a, b, and c:

|a + b| ≤ |a| + |b|
|a - b| ≤ |a| + |b|

(a) To prove sm+1 - sm ≤ 2·2^(-m)·|s₂ - s₁| for all m ∈ ℕ, we will use the given inequality |sn+2 - sn+1| ≤ |sn+1 - sn|.

Let's consider m ∈ ℕ. Using the given inequality, we can write:

|sm+1 - sm| ≤ |sm - sm-1|
≤ |sm-1 - sm-2|
≤ ...
≤ |s₂ - s₁|

Since we have |s₂ - s₁| on the right side, we can substitute it into the inequality:

|sm+1 - sm| ≤ |s₂ - s₁|

Now, we need to prove that |s₂ - s₁| ≤ 2·2^(-m)·|s₂ - s₁|. Let's multiply both sides of the inequality by 2^m:

2^m·|s₂ - s₁| ≤ 2·2^(-m)·|s₂ - s₁|

Since 2^m·2^(-m) = 2^(m-m) = 2^0 = 1, the inequality becomes:

|s₂ - s₁| ≤ 2·2^(-m)·|s₂ - s₁|

Therefore, we have shown that sm+1 - sm ≤ 2·2^(-m)·|s₂ - s₁| for all m ∈ ℕ.

(b) To prove |sn - sm| ≤ 4·2^m·|s₂ - s₁| for all n > m > 1, we can use the same approach as in part (a).

Let's consider n > m > 1. Using the given inequality, we can write:

|sn - sm| ≤ |sn - sn-1|
≤ |sn-1 - sn-2|
≤ ...
≤ |sm+1 - sm|

Using the inequality from part (a), we can substitute it into the inequality:

|sn - sm| ≤ |sm+1 - sm|
≤ 2·2^(-m)·|s₂ - s₁|

Now, we need to prove that 2·2^(-m)·|s₂ - s₁| ≤ 4·2^m·|s₂ - s₁|. Let's simplify this inequality:

2·2^(-m)·|s₂ - s₁| ≤ 4·2^m·|s₂ - s₁|

Since 2^(-m)·2^m = 2^(-m+m) = 2^0 = 1, the inequality becomes:

|s₂ - s₁| ≤ 4·2^m·|s₂ - s₁|

Therefore, we have shown that |sn - sm| ≤ 4·2^m·|s₂ - s₁| for all n > m > 1.

Learn more about inequalities in Mathematics: https://brainly.com/question/30238989

#SPJ11

False. The statement "To determine the probability of getting no more than 3 events of interest in binomial distribution; you will find the area under the normal curve for X= 2.5 and below" is False. What is the binomial distribution?Binomial distribution is a kind of probability distribution that is used in statistical inference. Binomial distribution refers to the likelihood of obtaining one of two possible outcomes as a result of an experiment.

The Binomial distribution's requirements include a fixed sample size (n) and independent trials. Additionally, the probabilities of success (p) and failure (q) must remain constant throughout the entire process.How to determine the probability of getting no more than 3 events of interest in binomial distribution?The Binomial Distribution is used to determine the probability of obtaining a specific number of successful outcomes. The following formula is used to calculate the binomial distribution probability:$$P(X=k) = \dbinom{n}{k}p^kq^{n-k}$$where:1. n: The total number of observations or trials.2. k: The number of successful outcomes.3. p: The probability of a successful outcome.4. q: The probability of an unsuccessful outcome.

Thus, we will find the probability by calculating P(X ≤ 3), where X is the number of successful outcomes. We can't use the normal distribution to calculate the probability in a binomial distribution because the binomial distribution is discrete in nature, and the normal distribution is continuous.  Therefore, the statement "To determine the probability of getting no more than 3 events of interest in binomial distribution; you will find the area under the normal curve for X= 2.5 and below".

To know more about probability  visit:-

https://brainly.com/question/31828911

#SPJ11

To find the line of best fit we follow this steps:

1. Calculate the mean of the x -values and the mean of the y -values

2. The following formula gives the slope of the line of best fit:

In our case, such computation will yield as a result:

The period of the function based on the information will be D. 2π.

The period of the function is the interval between repetitions of any function. A trigonometric function's period is the length of one whole cycle.

One cycle of the sine curve's length is referred to as its period. The period of the function is the minimum value of the dependent variable x after which the function will repeat itself.

f(x + k) = f(x).

-2sin(k + x) + 2 = 2sinx + 1

sin(k + x) = sin(2π + x)

k + x = 2π + x

k = 2π

The period is 2π.

Learn more about period on:

https://brainly.com/question/26486072

#SPJ1

The table provides various values, including all minimums and maximums, of a cosine function f (x) on the interval [0, 2π].

Angle 0 pi over 6 pi over 2 5 pi over 6 3 times pi over 2 2π

f (x) 1 0 –1 0 3 1

What is the period of the function?

A) 2 times pi over 3

B) pi over 2

C) π

D) 2π

Answer:

-45

Step-by-step explanation:

inside the parentheses: 2*-8= -16

                                        -16+1=-15

3 (-15)= -45

Answer:

-45

Step-by-step explanation:

hello

Answer:

A could finish the whole work in 60 days

Step-by-step explanation:

Both A and B can finish the work in 30 days.

That means both A and B can finish 1/30 part of the work in 1 day.

So in 20 days, both A and B can finish 20/30 = 2/3 part of the work.

Both A and B worked together for 20 days and completed 2/3 part of the work. Now 1/3 part of the work is remaining.

But after 20 days B left the work. Now A finished the remaining 1/3 part of the work alone.

Given A has completed the remaining 1/3 part of the work in 20 days. We are asked to find in how many days A could finish the whole work.

Let A could finish the whole work in x days.

That means A could finish 1/x part of the work in 1 day.

So in 20 days, A could finish 20(1/x) = 20/x part of the work.

But it's given that A finished the remaining 1/3 part of the work in 20 days.

Thus we are able to equate 20/x and 1/3.

\(\longrightarrow\dfrac{20}{x}=\dfrac{1}{3}\)

\(\Longrightarrow\underline{\underline{x=60}}\)

Hence A could finish the whole work in 60 days.

Answer:

\(10^7\)

Step-by-step explanation:

There are \(7\) zeroes in the number \(10,000,000\). Therefore, \(10,000,000\) can be written as \(\fbox{$10^7$}\).

*Any number written as \(10^n\) will be equal to \(1\) followed by \(n\) zeroes.

Answer:10^7

Step-by-step explanation:

Step-by-step explanation:

When dividing two exponents, you can use a formula of:

\(\frac{x^a}{x^b} = x^{a - b}\)

So your question would return:

\(\frac{3^2}{3^6} = 3^{2-6} = 3^{-4}\)

When an exponent is negative, it is equal to 1 divided by the positive number, so for this question, it would be:

\(\frac{1}{3^4}\)

Or in its expanded form:

\(\frac{1}{81}\)

Hope this helps!

Answer:

It's the 2nd one 3y2-43y over 12y2

Step-by-step explanation:

First u do a common denominator u multiply on the first side by 6y on the second side by 4 then u solve it till u get 6y2-86y over 24y2 then simplify by dividing by 2

The probability that a teenager plays varsity sports, given that they play a team sport, is approximately 0.4884 or 48.84%.

To find the probability that a teenager plays varsity sports given that they play a team sport, we can use conditional probability.

Let's denote:

A: Playing varsity sports

B: Playing a team sport

We are given:

P(B) = 43% = 0.43 (Probability of playing a team sport)

P(A) = 21% = 0.21 (Probability of playing varsity sports)

We need to find PA(|B), which represents the probability of playing varsity sports given that the teenager plays a team sport.

The conditional probability formula is:

P(A|B) = P(A ∩ B) / P(B)

P(A ∩ B) represents the probability of both A and B occurring simultaneously.

In this case, the probability of a teenager playing varsity sports and a team sport simultaneously is not given directly. However, we can make an assumption that all teenagers who play varsity sports also play a team sport. Under this assumption, we can say that P(A ∩ B) = P(A) = 0.21.

Now we can calculate P(A|B):

P(A|B) = P(A ∩ B) / P(B)

       = 0.21 / 0.43

      ≈ 0.4884

Therefore, the probability that a teenager plays varsity sports, given that they play a team sport, is approximately 0.4884 or 48.84%.

To learn more about probability click here:

brainly.com/question/22946813

#SPJ11

Answer:

A e f

Step-by-step explanation:

Hope this was helpful!!!

The equation of the secant line is y = 2x + 2.  The equation of the tangent line when x = -1 is y = -x - 1, in slope-intercept form.

(a) The equation of the secant line through the points where x has the given values can be found by calculating the slope between the two points and using the point-slope form of a line.

Let's calculate the slope first:

Slope (m) = (f(x2) - f(x1)) / (x2 - x1)

For x = -1 and x = 2:

f(-1) = \((-1)^2 + (-1)\) = 1 - 1 = 0

f(2) = \((2)^2 + 2\)= 4 + 2 = 6

Slope (m) = (f(2) - f(-1)) / (2 - (-1)) = (6 - 0) / (2 + 1) = 6 / 3 = 2

Now, we can use the point-slope form with either of the two given points (-1, 0) or (2, 6). Let's use the first point:

\(y - y_1 = m(x - x_1)\)

y - 0 = 2(x - (-1))

y = 2x + 2

Therefore, the equation of the secant line is y = 2x + 2.

(b) To find the equation of the tangent line when x has the first value, we need to calculate the derivative of the function and evaluate it at that point.

f(x) = \(x^2 + x\)

Taking the derivative of f(x) with respect to x:

f'(x) = 2x + 1

For x = -1:

f'(-1) = 2(-1) + 1 = -2 + 1 = -1

Using the point-slope form with the point (-1, f(-1)) = (-1, 0):

\(y - y_1 = m(x - x_1)\)

y - 0 = -1(x - (-1))

y = -x - 1

Therefore, the equation of the tangent line when x = -1 is y = -x - 1, in slope-intercept form.

Learn more about slope here:

https://brainly.com/question/30088055

#SPJ11

The complete question is:

For the given function, find (a) the equation of the secant line through the points where x has the given values and (b) the equation of the tangent line when x has the first value. y=f(x)=x^2+x; x=-1, x=2 (a) The equation of the secant line is y=______ (b) The equation of the tangent line is y=_____ type your answer in slope intercept form

stay home stay safe

hope you understand

The doctor used inductive reasoning because the doctor used various observations to come up with a final conclusion.

Answer:

x=35

Step-by-step explanation:

These two angles are supplementary (or a linear pair) meaning that they add up to 180°.

We can use this information to set up an equation:

3x+75=180

Subtract 75 from both sides

3x=105

Divide both sides by 3

x=35

Answer:

the answer is e i think.

Step-by-step explanation:

Answer:

Step-by-step explanation:

6c + 18-4c + 16

Solving like terms

2c + 34

Answer: 2c + 2

6(c + 3) - 4(c + 4)

= 6c + 18 - (4c + 16)

= 6c + 18 - 4c - 16

= 2c + 2

Step-by-step explanation:

Part A: To find the total surface area of the rectangular prism, we need to calculate the areas of all six faces and then add them together.

Given dimensions:

Length = 7 feet

Width = 3 feet

Height = 4.8 feet

Surface Area of each face:

Front and back faces: Length * Height

= 7 feet * 4.8 feet

= 33.6 square feet

Top and bottom faces: Width * Length

= 3 feet * 7 feet

= 21 square feet

Side faces: Width * Height

= 3 feet * 4.8 feet

= 14.4 square feet

Total Surface Area:

2 * (Front and back faces) + 2 * (Top and bottom faces) + 2 * (Side faces)

= 2 * 33.6 square feet + 2 * 21 square feet + 2 * 14.4 square feet

= 67.2 square feet + 42 square feet + 28.8 square feet

= 137 square feet

Therefore, the total surface area of the bench is 137 square feet.

Part B: To calculate the cost of covering the bench with ceramic tiles, we need to multiply the total surface area by the cost per square foot.

Cost per square foot = $0.89

Total Surface Area = 137 square feet

Total cost to cover the bench:

= Cost per square foot * Total Surface Area

= $0.89 * 137 square feet

= $121.93

Therefore, it will cost $121.93 to cover the bench with ceramic tiles.

According to the California Cigarette & Tobacco Products Tax Law, the average pack of cigarettes purchase in California costs $8.40.

Option A.

The price of a cigarette in California has been on the rise for many years, owing to the state's aggressive anti-tobacco initiatives.

The state of California, like many other US states, has implemented measures aimed at discouraging smoking and the use of tobacco products, including the introduction of high taxes on cigarettes.

The tax imposed on tobacco products is intended to help cover the expenses of treating tobacco-related diseases, which cost the state millions of dollars every year.

The average cost of a pack of cigarettes in California has been steadily increasing over the years.

This can be attributed to a variety of factors, including higher tobacco taxes and anti-smoking legislation, as well as increased public awareness about the dangers of smoking and the impact it can have on one's health and wellbeing.

In conclusion, the average pack of cigarettes purchase in California is $8.40, as mandated by the state's Cigarette & Tobacco Products Tax Law.

This law, along with other anti-smoking initiatives, has been effective in reducing the prevalence of smoking and tobacco use in California over the years.

Option A.

For more such questions on costs

https://brainly.com/question/25109150

#SPJ8

Step-by-step explanation:

The given number is 7,594,000,000.

To convert it into scientific notation,

First move the decimal point places to the left to find a number that is greater than or equal to 1 and less than 10. Then multiply by 10 , using an exponent on 10 that equals the number of places you moved the decimal. The exponent is negative if the original no was <1 and the exponent is positive, if the original number was  > 1.

In 7,594,000,000, we move the decimal point places to the left before 5. It means we have moved 9 digits left.

Hence, \(7,594,000,000=7.59\times 10^9\) is the scientific notation.

The given expression "the square of a number is 3 less than four times the number" is represented as x² = 3 - 4x and the values of number could be: x1= 0.645 , x2= -4.645

In mathematics, when we refer "square of a number" we mean that the exponent of that number is 2. So we express it mathematically as:

x²

When we express "3 less than" of a number we mean that the number 3 is subtracting that number. So we express it mathematically as: 3 -

x² = 3 -

When we express "four times the number" we mean that the number is exactly 4 times greater than a number or that it contains it 4 times. So we express it mathematically as: 4x

x² = 3 - 4x

Organizing the values, we have a quadratic equation: x² + 4x – 3 = 0

Where:

Solving the quadratic question we get the values for the (x) number

x= {- b ± √ [b² - (4* a*c)]} / (2 *a)

x= {- 4 ± √ [4² - (4* 1*-3)]} / (2 * 1)

x= {- 4 ± √ (16 + 12)} / 2

x= {- 4± √ 28} / 2

x= (- 4 ± 5.29 ) / 2

x1= (- 4 + 5.29 ) / 2

x1= (1,29) / 2

x1= 0.645

x2= (- 4 - 5.29 ) / 2

x2= (9.29) / 2

x2= -4.645

We can check the values, replacing them into the equation:

(x1)² = 3 - 4(x1)

(0.645)² = 3 - 4*(0.645)

0.42 = 0.42

(x2)² = 3 - 4(x2)

(-4.645)² = 3 - 4*(-4.645)

21.58 = 21.58

It is an algebraic equation where the polynomial formed has three terms, one variable has an exponent 2, another in which the variable has exponent 1 and the last term without any variable. Its standard form is:

ax²+ bx + c = 0

Learn more about quadratic equation at: brainly.com/question/25841119 and brainly.com/question/7784687

#SPJ4

Answer:

1) -2√2 2) 98

Step-by-step explanation:

the picture 1 and 2 are for question 1

the picture 3 and 4 are for question 2

We have to solve all of the parts of the given problem using our understanding of a sample and a population. It is given below.

A sample is taken when you want to take data from a specific set and then make observations based on that. A population is, on the other hand, the complete set that you want to make your observations from.

In the given problem -

A. To find how many books are published in one week by a famous publishing company - We are going to use a sample because we need to observe under s set category - one week.

B. To test a new drug produced by a biotech company - We are going to use a population because we need to monitor all individuals who take this drug.

C. To find the number of men and women working in an IT company with 600 people - We use a population here because we are going to use the data of all the people in the company.

D. To estimate the average salary of doctors in California - We use a sample here because the set category is California.

Learn more about Samples on

https://brainly.com/question/11045407

#SPJ4

Answer:

(-4 + sqrt11)/5

Step-by-step explanation:

-8 + sqrt(64-20)/10 = -4/5 + sqrt11/5

Each friend will receive approximately 0.10 gallons of popcorn.

To split the 5 eighths gallons of popcorn evenly between his 6 friends, we first need to convert the fraction to a decimal. Since one eighth is equal to 1/8, 5 eighths can be written as 5/8. To convert this fraction to a decimal, we divide the numerator (5) by the denominator (8). 5 divided by 8 equals 0.625. This means that the student has 0.625 gallons of popcorn.

To find out how much popcorn each friend will receive, we need to divide the total amount of popcorn (5 eighths gallons) by the number of friends (6).

First, let's convert 5 eighths to a decimal.

One eighth is equal to 1/8, so 5 eighths is 5/8.

To convert 5/8 to a decimal, divide the numerator (5) by the denominator (8):

5 ÷ 8 = 0.625.

Now, we can divide 0.625 by 6 to find the amount of popcorn each friend will receive.

0.625 ÷ 6 = 0.10416666667

Rounded to the nearest hundredth, each friend will receive approximately 0.10 gallons of popcorn.

Each friend will receive approximately 0.10 gallons of popcorn.

To know more about decimal visit:

brainly.com/question/30958821

#SPJ11

The equation to model the situation in slope intercept form is y = 2346x + 162418

2346 is the slope of the equation and it multiplies x, to determine the number of dentist that has increase in x years.

162418 is the y-intercept and its the initial number of dentist.

The slope intercept equation can be represented as follows:

where

Using the slope intercept formula the equation, 2y=4692x+324,836 can be modelled as follows:

divide both sides by 2

Therefore,

y = 2346x + 162418

x = number of years since 2001

y = number of active dentist

Therefore, 2346 is the slope of the equation and it multiply x, to determine the number of dentist that has increase in x years.

162418 is the y-intercept and its the initial number of dentist.

learn more on slope intercept here: https://brainly.com/question/16776778