What is 6(p+6)- (p-6)
Answers
Answer:
5p+42
Step-by-step explanation:
6(p+6)- (p-6)
Distribute
6p +36 - p +6
Combine like terms
6p -p + 36 +6
5p+42
Related Questions
3/4 + 1/3 as a fraction
Answers
Answer:
Step-by-step explanation:
Answer:1 1/12
Step-by-step explanation: 3/4+1/3=13/12=1 1/12
a bag contains 44 red and blue marbles. the ratio of red marbles to blue marble is 5:6. how many blue marbles are there?
Answers
Answer:
24 blue marbles
Step-by-step explanation:
We know
The ratio of red marble to blue marble is 5:6. Meaning for every 11 marbles; there are 5 red and 6 blue.
How many blue marbles are there?
6 x 4 = 24 blue marbles
So, there are 24 blue marbles.
AGE GROUP 25-----29 30-----34 35-----39 40-----44 45------49 50-----54 55------59
NUMBER OF PERSONS 3 7 21 28 23 6 1 Calculate: Mean, Median and Mode.
Answers
The mean of the data is 41.7, the median is 41.9 and the mode of the data is 42.9.
Here,
We have,
In mathematics, the three main methods for indicating the average value of a set of integers are mean, median, and mode. Adding the numbers together and dividing the result by the total number of numbers in the list yields the arithmetic mean. An average is most frequently used to refer to this. The middle value in a list that is arranged from smallest to greatest is called the median. The value that appears the most frequently on the list is the mode.
The mean is given as:
mean = summation of the frequency / total frequency
mean = 3708/89 = 41.66
The median of the given data is the central value.
In the given data median is the mean of the ages between 56 and 57.
Median = 45 + (89/2) - 59 / 23 * (5)
Median = 41.9
The mode is given for the data having the highest frquency.
The highest frequency is observed at 40-----44:
Mode = 40 + (28 - 21) / (56 - 21 - 23) (5)
Mode = 42.9
Hence, the mean of the data is 41.7, the median is 41.9 and the mode of the data is 42.9.
Learn more about median here:
brainly.com/question/28060453
#SPJ1
the expected number of typographical errors on a page of a certain magazine is .2. what is the probability that the next page you read contains (a) 0 and (b) 2 or more typographical errors? explain your reasoning!
Answers
(a) The probability that the next page you read contain zero is 0.8187.
(b) The probability that the next page you read contains or more typographical errors is 0.0175.
What is probability?
Simply put, probability is the likelihood that something will occur. When we don't know how an event will turn out, we can discuss the likelihood or likelihood of several outcomes
The probability that the next page you read contains zero. The expected number of typographical errors on a page of a certain magazine is
That is, y = 0.2
Let is a random variable representing the number of typos per page.
Since, we have a large number of letters per page, and the number of errors in one page will be small, we use Poisson distribution. The probability function of the Poisson distribution is defined as,
\(P(x = i) = e^-^y\frac{y^i}{i!},..... i = 0, 1, 2, ...\) ...(1)
Here, y is a parameter.
Find the probability that the next page you read contains zero.
That is, find P(y = 0)
plug y = 0 in equation (1), we get
P(x = 0) = 0.8187
The probability that the next page you read contain zero is 0.8187
Given the question that the probability that the next page you read contains more typographical errors. The expected number of typographical errors on a page of a certain magazine is 0.2
That is y = 0.2
Let is a random variable representing the number of typos per page.
Find the probability that the next page you read contains more typographical errors.
That is find P(x ≥ 2)
P(x ≥ 2) = 1 - P(x ≤ 1)
= 1 - (P(x = 0) + P(x = 1))
= 1 - 0.982477
= 0.0175
The probability that the next page you read contains or more typographical errors is 0.0175.
To know more about the probability, click on the link
https://brainly.com/question/24756209
#SPJ4
PLEASE HELP NEED THIS NOW DUE IN AN HOUR!!
Write and simplify an expression to represent the perimeter of the triangle shown. What is the perimeter of the triangle if y equals 3 feet?
(Please show work)

Answers
The perimeter of the triangle is 31 feet when the value if y is 3 feet.
Perimeter of the triangle:
Perimeter of the triangle is obtained by the total distance around the edges of a triangle.
The standard form for the perimeter of the triangle is
P = a + b + c.
where
a, b and c refers the edges of the triangle.
Given,
Here we have the triangle with the side values are (y + 5) feet, (3y + 5) feet, and (4y - 3) feet.
Then we have to find the perimeter of the triangle when the value of y is 3 feet.
We know formula of the perimeter of triangle, so first we have to calculate the side values of it, by apply the value of y as 3,
Apply the value of y as 3 feet then we get the value of the edges are
=> y + 5 => 3 + 5 = 8 feet
=> 3y + 5 => 3(3) + 5 = 14 feet
=> 4y - 3 => 4(3) - 3 = 9 feet.
Therefore, the edges of the triangle are 9ft, 14 ft and 9 ft.
Now, we have to apply the values on the formula of the perimeter of the triangle is, then we get
=> P = 8 + 14 + 9
Then the value of P = 31
Therefore, the value of the perimeter of the triangle is 31 feet.
To know more about Perimeter of the triangle here.
brainly.com/question/28660527
#SPJ1
Find a basis for the row space and the rank of the matrix. 5 -7 8 6 10 5 1-3 2 (a) a basis for the row space (b) the rank of the matrix
Answers
The answer to the questions are as follows:
(a) A basis for the row space is {(5, -7, 8), (6, 10, 5)}
(b) The rank of the matrix is 2
Step-by-Step Explanation:
To find a basis for the row space and the rank of the matrix, we need to perform row operations on the given matrix until it is in row echelon form. Here are the steps:
Use row operations to swap the first and third rows:
| 1 -3 2 |
| 6 10 5 |
| 5 -7 8 |
Use row operations to subtract 6 times the first row from the second row and 5 times the first row from the third row:
| 1 -3 2 |
| 0 28 -7 |
| 0 -8 2 |
Use row operations to divide the second row by 28 and subtract (-8/28) times the second row from the third row:
| 1 -3 2 |
| 0 1 -1/4 |
| 0 0 3/4 |
The matrix is now in row echelon form. The nonzero rows are the first two rows, which correspond to the first and second rows of the original matrix. Therefore, a basis for the row space is the set of these two rows:
{(5, -7, 8), (6, 10, 5)}
The rank of the matrix is the number of nonzero rows in the row echelon form, which is 2. So, the rank of the matrix is 2.
Learn more about the rank of the matrix :
https://brainly.com/question/29857274
#SPJ11
in macroland there is $12,000,000 in currency. the public holds 60% of the currency and banks hold the rest as reserves. if banks' desired reserve/deposit ratio is 25.0 percent, deposits in macroland equal and the money supply equals .
Answers
If banks' desired reserve/deposit ratio is 25.0 percent, deposits in macro land are $28800000 and the money supply is $36000000
The deposit ratio is the rate sum of money (stores) that commercial banks must not loan out or invest, the reserve ratio is as a rule fixed by the central banks to control inflation and the volume of cash in circulation inside a given country in a particular time.
Initial bank deposit= 60% of 12000000 = 7200000, The reserve/deposit will calculate as follows (25.0 /100%)=0.25 and If banks desired to keep 25.0 it deposit ratio will be : 1/0.25*7200000= 28800000
So the money supply will be the desired deposited :
=28800000+ 7200000 =36000000
To know more about money supply refer to the link https://brainly.com/question/28891105?referrer=searchResults.
#SPJ4
Lucy has $7 less than Kristine and $5 more than Nina together,the three have $35 how much does Lucy have?
Answers
Lucy has $7 less than Kristine and $5 more than Nina together, the three have $35. Lucy has $11.
Let's denote the amount of money that Kristine has as K, the amount of money that Lucy has as L, and the amount of money that Nina has as N.
According to the given information, we can form two equations:
Lucy has $7 less than Kristine: L = K - 7
Lucy has $5 more than Nina: L = N + 5
We also know that the three of them have a total of $35: K + L + N = 35
We can solve this system of equations to find the values of K, L, and N.
Substituting equation 1 into equation 3, we get:
K + (K - 7) + N = 35
2K - 7 + N = 35
Substituting equation 2 into the above equation, we get:
2K - 7 + (L - 5) = 35
2K + L - 12 = 35
Since Lucy has $7 less than Kristine (equation 1), we can substitute K - 7 for L in the above equation:
2K + (K - 7) - 12 = 35
3K - 19 = 35
Adding 19 to both sides:
3K = 54
Dividing both sides by 3:
K = 18
Now we can substitute the value of K into equation 1 to find L:
L = K - 7
L = 18 - 7
L = 11
Finally, we can find the value of N by substituting the values of K and L into equation 3:
K + L + N = 35
18 + 11 + N = 35
N = 35 - 18 - 11
N = 6
Therefore, Lucy has $11.
Learn more about Kristine from
https://brainly.com/question/27244350
#SPJ11
- is slightly variable - and follows a nearly normal distribution - with a mean of 25 square feet - and a standard deviation of 3 square feet. (a) what is the probability that - the area covered by a can of spray paint - is more than 27 square feet?
Answers
The probability that the area covered by a can of spray paint is 0.251
The variable of interest is:
X: are objects that can be painted with a single can of spray paint.
This variable has a normal distribution, with a mean of 25 feet square and a standard deviation of 3 feet square.
Symbolically:
P(X≥27)
To calculate the probability, use the standard normal distribution, so first standardize the value of X using:
Z= (X-μ)/σ
P(X≥27)= P(Z≥(27-25)/3)= P(Z≥0.67)
Now, because the Z-table contains cumulative probability information:
P(Zα≤Z₀)=1-α
you must perform the following conversion to calculate the requested probability:
1 - P(Z<0.67)= 1 - 0.749= 0.251
For more information on Probability, visit :
https://brainly.com/question/15222258
#SPJ4
1. (1 point) Let x be a real number. Show that a (1 + x)2n > 1+ 2nx for every positive integer n.
Answers
For a real number x, by using mathematical induction it is shown that a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n.
To prove the inequality a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n, we will use mathematical induction.
The inequality holds true for n = 1, and we will assume it is true for some positive integer k.
We will then show that it holds for k + 1, which will complete the proof.
For n = 1, the inequality becomes a\((1 + x)^2\) > 1 + 2x.
This can be expanded as a(1 + 2x + \(x^2\)) > 1 + 2x, which simplifies to a + 2ax + a\(x^2\) > 1 + 2x.
Now, let's assume the inequality holds true for some positive integer k, i.e., a\((1 + x)^{2k}\) > 1 + 2kx.
We need to prove that it holds for k + 1, i.e., a\((1 + x)^{2(k+1)}\) > 1 + 2(k+1)x.
Using the assumption, we have a\((1 + x)^{2k}\) > 1 + 2kx.
Multiplying both sides by \((1 + x)^2\), we get a\((1 + x)^{2k+2}\) > (1 + 2kx)\((1 + x)^2\).
Expanding the right side, we have a\((1 + x)^{2k+2}\) > 1 + 2kx + 2x + 2k\(x^2\) + 2\(x^2\).
Simplifying further, we get a\((1 + x)^{2k+2}\) > 1 + 2(k+1)x + 2k\(x^2\) + 2\(x^2\).
Since k and x are positive, 2k\(x^2\) and 2\(x^2\) are positive as well.
Therefore, we can write a\((1 + x)^{2k+2}\) > 1 + 2(k+1)x + 2k\(x^2\) + 2\(x^2\) > 1 + 2(k+1)x.
This proves that if the inequality holds for some positive integer k, it also holds for k + 1.
Since it holds for n = 1, it holds for all positive integers n by mathematical induction.
Therefore, we have shown that a\((1 + x)^{2n}\) > 1 + 2nx for every positive integer n.
Learn more about mathematical induction here:
https://brainly.com/question/29503103
#SPJ11
Find the percentage change from
40 to 60.
Answers
Answer:
50%
thats the answer
Solve.
h + 14 = -14
h =
Answers
Answer:
h= -28
Step-by-step explanation:
h+14=-14 isolate h by subtracting 14 on both sides
h= -28
Answer:
h=-28
Step-by-step explanation:
k byeeeeeeeeeeeeee:)
A new Youth Activity Center is being built in Hadleyville. The perimeter of the rectangular playing field is 442 yards. The length of the field is 4 yards less than quadruple the width. What are the dimensions of the playing field?
Answers
Answer:
45 yards and 176 yards
Step-by-step explanation:
Perimeter of a rectangular playing field = 2(Length + width)
P = 2(L+W)..................... Equation 1
From the question,
Let the width of the rectangular playing field = x yards,
The the Length = 4x-4 yards.
Given: P = 442 yards.
Substitute these values into equation 1
442 = 2(4x-4+x)
442/2 = 5x-4
221 = 5x-4
5x = 221+4
5x = 225
x = 225/5
x = 45 yards.
Width = 45 yards.
Length = (45×4)-4 yards = 176 yards
Hence the dimensions of the playing field are, 45 yards and 176 yards
Mark costs a shadow that is 4-feet long. At the same time, a nearby tree costs a shadow that is 20-feet long. If mark is 6 feet tall, how tall is the tree?
Answers
Answer:
30 feet
Step-by-step explanation:
For this problem we can use ratios 6:4 and x:20. We can also write these ratios as fractions 6/4 and x/20 or 3/2 and x/20. Set the two fractions equal to each other and solve for x:
3/2 = x/20
1.5 = x/20
30 = x
Select the type of cross section formed when a rectangular pyramid is cut by a plane containing its altitude and perpendicular to its base.
Answers
The rectangular pyramid is cut by a plane containing its altitude and perpendicular to its base and will be divided into two rectangular oblique pyramids.
What is Geometry?One of the first areas of mathematics is geometry, along with maths. It is preoccupied with spatial characteristics including the separation, form, size, and relative placement of objects. A geometer is a mathematician who specializes in shape.
A straight plane passes through the apex point of the given pyramid when it is intersected by a plane that is orthogonal to its foundation and contains its altitude. The perpendicular planes are parallel to the base at all times. Therefore, this kind of cross-section is a form of continuous passing through the triangle's vertex point.
The rectangular pyramid is cut by a plane containing its altitude and perpendicular to its base and will be divided into two rectangular oblique pyramids.
More about the geometry link is given below.
https://brainly.com/question/7558603
#SPJ1
The sides of an isosceles triangle are whole numbers, and its perimeter is 30 units. What is the probability that the triangle is equilateral?
Answers
The probability that the triangle is equilateral is `0`.
Let's assume that the two sides of an isosceles triangle are `a`. Then, the perimeter of the triangle is `2a + b = 30`, where `b` represents the base of the triangle.
Substituting the value of `b` in terms of `a` in the above equation, we get:`2a + 2sqrt(a^2 - (30 - 2a)^2/4) = 30`
Simplifying this equation, we get: 4a² - 120a + 675 = 4√(3)a²
Squaring both sides of the equation, we get: 16a⁴ - 960a³ + 40500a² - 243000a + 455625 = 0
The solutions of this equation are: a = 7, 15
The probability of the triangle being equilateral is 0.
An isosceles triangle can be equilateral only if its sides are of equal length. Since there is only one value of `a` such that `2a < 30` and `a` is a whole number, the probability of the triangle being equilateral is 0.
Hence, the probability that the triangle is equilateral is `0`. The given isosceles triangle can have sides of length `7-7-16` or `15-15-0`.
To learn about probability here:
https://brainly.com/question/251701
#SPJ11
gthe number of calls received by an office on monday morning between 8:00 am and 9:00 am has a mean of 8. calculate the probability of getting exactly 2 calls between eight and nine in the morning. round your answer to four decimal places.
Answers
The probability of receiving at least two calls between eight and nine in the morning is 0.8009, or 80.09%.
The chance that X represents the number of successes of a random variable in a Poisson distribution is provided by the following formula:
\(P(X=x)=\frac{e^{-u} *u^x}{(x)!}\)
where
The number of successes is x.
The Euler number is e = 2.71828.
μ is the mean in the specified time period
The mean number of calls that an office receives on a Monday between 8:00 AM and 9:00 AM is 8.
It follows that μ = 8
Determine the likelihood of receiving at least two calls between 8:00 and 9:00 in the morning.
You either receive no calls or at least one call. Decimal 1 represents the total of these events' probabilities. Mathematically, it follows that
\(P(X=0)+P(X\geq 1)=1\\\)
We desire \(P(X\geq 1 )\). So
\(P(X\geq 1)=1-P(X=0)\)
where
\(= 1-[P(x=0)+P(x=1)]\\=1-e^{-3} (1+3)\\=1-0.19915\\=0.8009\)
The probability of receiving at least two calls between eight and nine in the morning is 0.8009 or 80.09%.
To learn more about probability, refer:-
https://brainly.com/question/30034780
#SPJ4
Consider the following conjecture.
There are no prime numbers between 7,608 and 7,620
How could this statement be shown to be true or false? Would it still be a conjecture if no counterexample were found? Explain

Answers
There are no prime numbers between7,608 and 7,620
A prime number is a whole number greater than 1 whose only factors are 1 and itself
The numbers between 7608 and 7620 are:
7609,7610,7611,7612,7613,7614,7615,7616,7617,7618,7619
Here we have to check these numbers are prime number or not
Prime factorization
7609=7×1087
7610=2×5×761
7611=3×43×59
7612=2×2×11×173
7613=23×331
7614=2×3×3×3×3×47
7615=5×1523
7616=2×2×2×2×2×2×7×17
7617=3×2539
7618=2×13×293
7619=19×401
Hence, proved that there are no prime numbers between7608 and 7620
Please helpp ive been stuck in this exercise for an hourrrr(mathh)
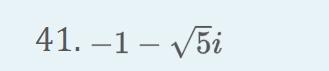

Answers
let's keep in mind that a conjugate is no more than the same "pair" but with a different sign between, so the conjugate of meow + quack is simply meow - quack, and so on, let's also recall that i² = -1.
\(\textit{difference of squares} \\\\ (a-b)(a+b) = a^2-b^2 \\\\[-0.35em] ~\dotfill\\\\ -1-\sqrt{5}i\hspace{5em}\stackrel{conjugate}{-1+\sqrt{5}i} \\\\\\ (-1-\sqrt{5}i)(-1+\sqrt{5}i)\implies (-1)^2~~ - ~~(\sqrt{5}i)^2\implies (-1)^2~~ - ~~(\sqrt{5})^2 i^2 \\\\\\ 1~~ - ~~(\sqrt{5^2})i^2\implies 1~~ - ~~(5)(-1)\implies 1-(-5)\implies 1+5\implies \text{\LARGE 6}\)
(x^2+3x+1)(x^2-2x+5)
Answers
Answer:
x^4 + x^3 + 13x + 5
Step-by-step explanation:
a pair of jeans is only $12 after a 60% discount what is the original price
Answers
Answer:
20
Step-by-step explanation:
I did this test is 20
60 percent of 20 is 12 dollars
The original price of a pair of jeans would be; 30 dollar.
How to find the percentage from the total value?Suppose the value of which a thing is expressed in percentage is "a'
Suppose the percent that considered thing is of "a" is b% Then since percent shows per 100 (since cent means 100), thus we will first divide the whole part in 100 parts and then we multiply it with b so that we collect b items per 100 items(that is exactly what b per cent means).
Given that a pair of jeans is only $12 after a 60% discount.
The original price in percentage terms = 100%
After the first discount, the price is : 100- 60% = 40%
The equation can be used to represent the price;
40% x original price = $12
0.40 x p = 12
where p = original price
Divide both sides of the equation by 0.40;
p= $30
Hence, The original price of a pair of jeans would be; 30 dollar.
Learn more about percent here:
https://brainly.com/question/11549320
#SPJ2
Simplify 6V 80.
0 6+16 51
0 6+415
0 6+1615
O 6+4v5i

Answers
Answer:
2nd option
Step-by-step explanation:
Using the rule of radicals
\(\sqrt{a}\) × \(\sqrt{b}\) ⇔ \(\sqrt{ab}\) and \(\sqrt{-1}\) = i
Simplifying the radical
\(\sqrt{-80}\)
= \(\sqrt{16(5)(-1)}\)
= \(\sqrt{16}\) × \(\sqrt{5}\) × \(\sqrt{-1}\)
= 4 × \(\sqrt{5}\) × i
= 4i\(\sqrt{5}\)
Then
6 + \(\sqrt{-80}\)
= 6 + 4i\(\sqrt{5}\)
Combine the like terms to create an equivalent expression.
-y+6y
Answers
Answer:
Step-by-step explanation:
6y - y = y(6 - 1) = y * 5
The answer is 5y.
A group of 75 math students were asked whether they
like algebra and whether they like geometry. A total of
45 students like algebra, 53 like geometry, and 6 do
not like either subject.
Algebra vs. Geometry
Likes Algebra
Does Not
Like Algebra
Total
Likes
Geometry
Mark this and return
a
3
53
Does Not
Like Geometry
b
6
e
Total
45
P
75
What are the correct values of a, b, c, d, and e?
a 16, b = 29, c = 22, d = 30, e = 24
a = 29, b = 16, c = 30, d = 22, e = 24
a 16, b = 29, c = 24, d = 22, e = 30
H
a = 29, b = 16, c = 24, d = 30, e = 22
Answers
The correct values for a, b, c, d, and e are a = 16, b = 29, c = 24, d = 22, and e = 30 for group of 75 students on asking whether they like Algebra or Geometry.
For the values of a, b, c, d, and e, we can use the information provided in the table. Let's break it down step-by-step:
We are given that a total of 75 math students were surveyed. Therefore, the total number of students should be equal to the sum of the students who like algebra, the students who like geometry, and the students who do not like either subject.
75 = 45 (Likes Algebra) + 53 (Likes Geometry) + 6 (Does Not Like Either)
Simplifying this equation, we have:
75 = 98 + 6
75 = 104
This equation is incorrect, so we can eliminate options c and d.
Now, let's look at the information given for the students who do not like geometry. We know that a + b = 6, where a represents the number of students who like algebra and do not like geometry, and b represents the number of students who do not like algebra and do not like geometry.
Using the correct values for a and b, we have:
16 + b = 6
b = 6 - 16
b = -10
Since we can't have a negative value for the number of students, option a is also incorrect.
The remaining option is option e, where a = 29, b = 16, c = 24, d = 22, and e = 30. Let's verify if these values satisfy all the given conditions.
Likes Algebra: a + c = 29 + 24 = 53 (Matches the given value)
Does Not Like Algebra: b + d = 16 + 22 = 38 (Matches the given value)
Likes Geometry: c + d = 24 + 22 = 46 (Matches the given value)
Does Not Like Geometry: b + e = 16 + 30 = 46 (Matches the given value)
All the values satisfy the given conditions, confirming that option e (a = 29, b = 16, c = 24, d = 22, and e = 30) is the correct answer.
For more such information on Algebra and Geometry:
https://brainly.com/question/24696219
#SPJ8
The five values for a data set are: minimum = 0 lower quartile = 2 median = 3. 5 upper quartile = 5 maximum = 10 Bruno created the box plot using the five values. What error did he make? The right whisker should go from 3. 5 to 10. The left whisker should go from 0 to 2. The box should go from 2 to 3. 5. The box should go from 3. 5 to 5
Answers
The five values for a data set are: minimum = 0 lower quartile = 2 median = 3. 5 upper quartile = 5 maximum = 10 Bruno created the box plot using the five values. Bruno made error. The left whisker should go from 0 to 2.
About quartileQuartiles is a type of quartile that divides data into four parts with approximately the same number. The first quartile or lower quartile (Q1) is the middle value between the smallest value and the median of the data group. The first quartile is a marker that the data in that quartile is 25% below the data group.
The second quartile (Q2) is the median data which marks 50% of the data (dividing the data in half). The third or upper quartile (Q3) is the middle value between the median and the highest value of the data set. The third quartile is a marker that the data in that quartile is 75% below the data group. Quartiles are a form of an ordered statistic because to determine quartiles, data needs to be sorted from smallest to largest value first.
Learn more about quartile at https://brainly.com/question/24329548.
#SPJ4
Mila invested $1,500 in an account in the year 2010, and the value has been growing exponentially at a constant rate. The value of the account reached $2, 100 in the year 2015. Determine the value of the account, to the nearest dollar in the year 2024.
Answers
Answer:
$3867.80
Step-by-step explanation:
f(x)=a(1+r)^{x}
f(x)=exponential growth function
a=initial amount
r=growth rate
{x}=number of time intervals
so let x=years
start amount is 1,500
1,500(1+r)^5=2100
(r+1)^5= 7 /5
((r+1)5) ^1 /5 =( 7 /5 ) ^1 /5
r=0.06961
which is rounded 7 percent growth rate
2024-2010=14 years
1,500(1+0.07)^{14}=
3867.801225
Now round cause it's money
$3867.80
AB=? Round your answer to the nearest hundredth

Answers
AB x sin 20 = 3
AB = 3 / sin 20
= 8.77 (2 dp)
Rahul orders cricket bats and balls. He orders 15 bats and 12 balls. What is the ratio of bats to balls?
Answers
Answer:
1,25:1
Step-by-step explanation:
We just have to divide the number of bats by the number of balls!
Guys can you please help. I dont understand. Thank you. :))))
Lines AB and CD intersect at E. If the measure of angle AEC=5x-20 and the measure of angle BED=x+50, find, in degrees, the measure of angle CEB.
Answers
Answer: 112.5
Step-by-step explanation: When line AB and CD intersect at point E, angle AEC equals BED so you set them equal to each other and find what x is. 5x -20 = x + 50, solving for x, which gives you 17.5. Finding x will tell you what AEC and BED by plugging it in which is 67.5. Angle BED and BEC are supplementary angles which adds up to 180 degrees. So to find angle CEB, subtract 67.5 from 180 and you get 112.5 degrees.
Evaluate the following expression: 8x - 6 - 7y + 3 use x=3 and y=6
Answers
Answer:
-27
Step-by-step explanation:
8x - 6 - 7y + 3
X = 3 Y = 6
8(3) - 6 - 7(6) + 3
8 x 3 = 24
7 x 6 = 42
24 - 6 - (42 + 3)
(24 - 6) - 45
18 - 45 = -27