what is a equation for 11 5 and b
Answers
Related Questions
Write the equation of the line that is parallel to y=5 and passes through point (4,2)
Answers
Answer:bro need more classification
Step-by-step explanation:
Please help for section d) 100 points, must show all working and step by step

Answers
Answer:
Step-by-step explanation:
(a) and (b) see diagram
(c) you can see from the graph, the purple line hits the parabola twice which is y=6 or k=6
(d) Solving simultaneously can mean to set equal
6x - x² = k >subtract k from both sides
6x - x² - k = 0 >put in standard form
- x² + 6x - k = 0 >divide both sides by a -1
x² - 6x + k = 0
(e) The new equation is the same as the original equation just flipped (see image)
(f) The discriminant is the part of the quadratic equation that is under the root. (not sure if they wanted the discriminant of new equation or orginal. I chose new)
discriminant formula = b² - 4ac
equation: x² - 6x + 6 = 0 a = 1 b=-6 c = 6
discriminant = b² - 4ac
discriminant= (-6)² - 4(1)(6)
discriminant = 36-24
discriminant = 12
Because the discriminant is positive, if you put it back in to the quadratic equation, you will get 2 real solutions.

A new suspension bridge is being built in Sunnydale. The last cable is being attached to the top of the tower. The cable is 400 feet long and has an a 39 degrees. Complete the sentence below to determine the height of the tower. Show your work on paper.
The tower, when rounded to the nearest foot, has a height of about……Ft
Answers
The height of the tower is about 250 feet.
We can use trigonometry to determine the height of the tower.
Let's label the height of the tower as "h". Then we can use the sine function:
sin(39) = h/400
Multiplying both sides by 400, we get:
h = 400 × sin(39)
h = 250.11.
Hence, the height of the tower is about 250 feet.
To learn more on trigonometry click:
https://brainly.com/question/25122835
#SPJ1
(1 point) a poll is taken in which 302 out of 525 randomly selected voters indicated their preference for a certain candidate. (a) find a 95% confidence interval for p.
Answers
Using the standard z table, a 95% confidence interval for p is (0.5327,0.6173).
In the given question,
A poll is taken in which 302 out of 525 randomly selected voters indicated their preference for a certain candidate.
We have to find a 95% confidence interval for p.
From the question, x=302, n=525
So estimation point,
P=x/n
P=302/525
P=0.575
Now the z value of 95% confidence interval is 1.960 using the standard z table.
Margin of Error (E)=z×√{P(1−P)}/n
Now putting the value
E=1.960×√{0.575(1−0.575)}/525
E=1.960×√(0.575×0.425)/525
E=1.960×√0.244/525
E=1.960×√0.000465
E=1.960×0.0216
E=0.0423
At 95% confidence interval for p is
P−E ≤ p ≤ P+E
Now putting the value
0.575−0.0423≤ p ≤0.575+0.0423
0.5327≤ p ≤0.6173
Hence, a 95% confidence interval for p is (0.5327,0.6173).
To learn more about confidence interval link is here
brainly.com/question/24131141
#SPJ4
i need help on my homework pls

Answers
Answer:
y=x2
I got it solved! tell me if it helped
NEED HELP ASAP FOR BRAINLYEST+
A statistics teacher decides to give his students a 5-question multiple-choice quiz with 4 possible answers for each question. If a student decides to randomly guess on each question, what is the probability the student will get 2 questions correct?
0.19
0.25
0.26
0.56
Answers
Answer:
0.26
Step-by-step explanation:
just took the quiz
the operation manager at a tire manufacturing company believes that the mean mileage of a tire is 37,014 miles, with a standard deviation of 4617 miles. what is the probability that the sample mean would differ from the population mean by less than 221 miles in a sample of 56 tires if the manager is correct? round your answer to four decimal places.
Answers
Probability or p-vale that the sample mean would differ from the population mean by less than 221 miles in a sample of 56 tires is equals to zero if the manager is correct.
We have data of an operation manager at a tire manufacturing company.
Mean mileage of a tire, \( \mu\)
= 37,014 miles
standard deviation, \( \sigma\)
= 4617 miles.
Sample size, n = 56
We have to determine the probability that the sample mean would differ from the population mean by less than 221 miles. Using Z-score formula in normal distribution, \(\small z= \frac{ \bar x-\mu }{\frac{\sigma }{\sqrt{n}}},\)
Plugging all known values in above formula, \(z = \frac{ 221 - 37,014} {\frac{4617}{ \sqrt{56}}}\)
= 59.634
\(P( \bar x < 221) = P ( \frac{ \bar x-\mu }{\frac{\sigma }{\sqrt{n}}} < \frac{ 221 - 37,014} {\frac{4617}{ \sqrt{56}}}) \\ \)
=> P ( z < 59.63) = P( \bar x < 221)
Using the Z-distribution table, probability value is equals to 0. Hence, required probability is zero.
For more information about P-value, refer:
https://brainly.com/question/4621112
#SPJ4
Is ASAM ADEL? If so, identify the similarity postulate or theorem that
applies

Answers
Step-by-step explanation:
Similar by SAS property.
5/15 = 9/27, corresponding angles = 31°.
Given the head of a singly linked list and an integer k, split the linked list into k consecutive linked list parts.
The length of each part should be as equal as possible: no two parts should have a size differing by more than one. This may lead to some parts being null.
The parts should be in the order of occurrence in the input list, and parts occurring earlier should always have a size greater than or equal to parts occurring later.
Return an array of the k parts.
Answers
To split a singly linked list into k consecutive parts with roughly equal sizes, you can use the following algorithm:
1. Calculate the length of the linked list by iterating through it.
2. Determine the size of each part by dividing the length by k, and the remainder by using the modulus operator (%).
3. Initialize an array of linked list nodes with a size of k to store the head of each part.
4. Iterate through the linked list, and for each part:
a. Assign the current node as the head of the current part in the array.
b. Determine the number of nodes for the current part by adding the base size, and if the current part index is less than the remainder, add 1.
c. Move the current node pointer to the last node of the current part by iterating through the determined number of nodes.
d. Set the next pointer of the last node of the current part to null, and move the current node pointer to the next node in the linked list.
5. Return the array of the k parts.
This algorithm ensures that the linked list is split into k consecutive parts with sizes as equal as possible, and parts occurring earlier have a size greater than or equal to parts occurring later.
To learn more about linked list : brainly.com/question/29360466
#SPJ11
Cam’s tent (shown below) is a triangular prism.
Find the surface are, including the floor of his tent
PLEASE HELP

Answers
Answer:
21.4 m²
Step-by-step explanation:
To find the surface area of this whole triangular prism, we have to look at the bases (the triangles), find their surface area, then look at the sides (the rectangles) and find theirs.
Let's start with the triangles. The area of any triangle is \(\frac{bh}{2}\). The base of this triangle is 2m (because there are 2 one meters) and the height is 1.7m.
\(\frac{2\cdot1.7}{2} = \frac{3.4}{2} = 1.7\)
So the area of one of these triangles is 1.7m. Multiplying this by two, because there are two triangles in this prism:
\(1.7\cdot2=3.4\)
Now let's find the area of the sides.
The side lengths are 2 and 3, so
\(2\cdot3=6\), and there are 3 sides (including the bottom/floor) so \(6\cdot3=18\).
Now we add.
\(18+3.4=21.4\) m².
Hope this helped!
Answer: 21.4 square meters^2
Step-by-step explanation:
Toboggan R Us has 49,000 toboggan caps. Red caps sell for $25, blue caps sell for $20, and green caps sell for $15. The number of red caps equals the total number of blue and green caps (combined). Suppose the store makes $1, 052, 000. How many caps of each color get sold?
Answers
If the store makes $1, 052, 000 then 24,500 red caps, 14,400 blue caps, and 10,100 green caps were sold.
Let's use the variables "r", "b", and "g" to represent the number of red, blue, and green caps sold, respectively.
From the problem statement, we know that:
r + b + g = 49,000 (total number of caps sold)
r = b + g (number of red caps equals the total number of blue and green caps)
25r + 20b + 15g = 1,052,000 (total revenue generated from selling caps)
We can use the first two equations to eliminate one of the variables. Since we have an equation that relates r to b and g, we can substitute that expression for r in the other two equations:
b + g + b + g = 49,000 (substituting r = b + g into the first equation)
25(b + g) + 20b + 15g = 1,052,000 (substituting r = b + g into the third equation)
Simplifying the first equation, we get:
2b + 2g = 49,000
b + g = 24,500
Substituting this expression for b + g in the second equation, we get:
25(b + g) + 20b + 15g = 1,052,000
25(24,500) + 20b + 15g = 1,052,000
612,500 + 20b + 15g = 1,052,000
20b + 15g = 439,500
We now have two equations with two unknowns. We can solve for one of the variables using one of the equations, and then substitute the solution back into the other equation to solve for the other variable.
Let's use the second equation to find the value of "g":
20b + 15g = 439,500
15g = 439,500 - 20b
g = (439,500 - 20b)/15
Since we know that b + g = 24,500, we can substitute this expression for "g" to get an equation in terms of "b" only:
b + (439,500 - 20b)/15 = 24,500
15b + 439,500 - 20b = 367,500
-5b = -72,000
b = 14,400
Substituting this value of "b" into b + g = 24,500, we get:
14,400 + g = 24,500
g = 24,500 - 14,400
g = 10,100
Finally, we can use the equation r = b + g to find the value of "r":
r = b + g
r = 14,400 + 10,100
r = 24,500
Therefore, the number of red, blue, and green caps sold are:
r = 24,500 red capsb = 14,400 blue capsg = 10,100 green capsTo learn more about variables, refer:-
https://brainly.com/question/29583350
#SPJ4
Salon he runs 1/5 of a mile in 1/20 of an hour he swims 2/3 of a mile and 1/9 of an hour he bikes for miles in a quarter of an hour at these rights how many minutes does it take being to run 2 miles swim 2 miles in bike eight
Answers
Answer: 30 mins + 30 mins + 20 Mins
= 80 Minutes
SIMPLIFY THE
EXPRESSION
3(x - 2) – 5x - 10
Answers
3(x−2)−5x−10
Distribute:
=(3)(x)+(3)(−2)+−5x+−10
=3x+−6+−5x+−10
Combine Like Terms:
=3x+−6+−5x+−10
=(3x+−5x)+(−6+−10)
=−2x+−16
Answer:
=−2x−16
Answer:
-2x - 16
General Formulas and Concepts:
Pre-Algebra
Distributive Property
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightAlgebra I
Terms/CoefficientsStep-by-step explanation:
Step 1: Define
3(x - 2) - 5x - 10
Step 2: Simplify
Distribute 3: 3x - 6 - 5x - 10Combine like terms (x): -2x - 6 - 10Combine like terms (Z): -2x - 16The dimensions of a triangle are represented by the functions shown. Which function represents the area of the triangle, ? h(x) = 12x2 + 6x h(x) = 12x2 – 6x h(x) = 24x2 – 12x h(x) = 12x – 6
Answers
Answer:
h(x) = 12x – 6
Step-by-step explanation:
To know which is the function that represents the area of a triangle, you take into account the degree of the given polynomials.
If the degree is 2, the graph of the function is a parabola.
If the degree is 1, the graph of the function is a line.
By comparing all functions you can conclude that only the last option (h(x)=12-x-6) is convenient to construct a triangle. The y-intersect of the function is at y=-6, the slope is positive and the x-intersect is x=1/2. This forms a triangle in the fourth quadrant of the coordinate system.

Answer:
B. g(x) = 12x2 – 6x
Step-by-step explanation:
correct on edge 2020
If 6 candy bars cost a total of $12, then $2 is the ___________ of the candy bars. *
Answers
5 → z5 be a random permutation function. what is pr[ f(1) = 1 | f(0) = 0 ]? express your answer as a reduced fraction without any spaces (eg, 1/10 and not 2/20 or 0.1), or as 0 or 1, if appropriate.
Answers
If f: Z₅→Z₅ be a random permutation function , then the Probability , Pr[f(2)=2| f(0)=0 and f(1)=1] is 1/60 .
Let f: Z₅→Z₅ be a random permutation function ;
There are n! permutations functions defined on set of n elements ;
So , the total number of permutations defined on Z₅ is 5! = 120 ;
Now , if f(2) = 2 , f(0) = 0 and f(1) = 1 , then the remaining elements of Z₅ are 3 and 4 .
So , the possible images of 3 and 4 are f(3) = 3 ⇒ f(4) = 4 ;
or f(3) = 4 ⇒ f(4) = 3 .
So , there are only 2 such permutations ,
⇒ Pr[f(2)=2| f(0)=0 and f(1)=1] = 2/120 = 1/60 .
Therefore , the probability Pr[f(2)=2| f(0)=0 and f(1)=1] is = 1/60 .
Learn more about Random Permutations here
https://brainly.com/question/15186748
#SPJ4
The given question is incomplete , the complete question is
Let f : Z₅→Z₅ be a random permutation function. what is Pr[ f(2)=2| f(0)=0 and f(1) = 1 ]? Express your answer as a reduced fraction without any spaces (e.g., 1/10 and not 2/20 or 0.1), or as 0 or 1, if appropriate.
How can you use transformations to graph this function? y=3⋅7 −x+2 Explain vour stess.
Answers
Given the function y=3⋅7−x+2, the general form of the function is y = a(x-h) + k, where "a" represents the vertical stretch or compression of the function, "h" represents the horizontal shift, and "k" represents the vertical shift of the graph.The given function can be transformed by applying vertical reflection and horizontal translation to the graph of the parent function.
Hence, we can use the transformations to graph the given function y=3⋅7−x+2.Solution:Comparing the given function with the general form of the function, y = a(x-h) + k, we can identify that:a = 3, h = 7, and k = 2We can now use these values to graph the given function and obtain its transformational form
.First, we will graph the parent function y = x by plotting the coordinates (-1,1), (0,0), and (1,1).Next, we will reflect the parent function vertically about the x-axis to obtain the transformational form y = -x.Now, we will stretch the graph of y = -x vertically by a factor of 3 to obtain the transformational form y = 3(-x).Finally, we will translate the graph of y = 3(-x) horizontally by 7 units to the right and vertically by 2 units upwards to obtain the final transformational form of the given function y=3⋅7−x+2.
Hence, the graph of the given function y=3⋅7−x+2 can be obtained by applying the vertical reflection, vertical stretch, horizontal translation, and vertical translation to the parent function y = x.
To know moreb about graph visit :
https://brainly.com/question/10712002
#SPJ11
PLEASE HELP ME ANSWER IF YOU KNOW!!!!!!!!!1111
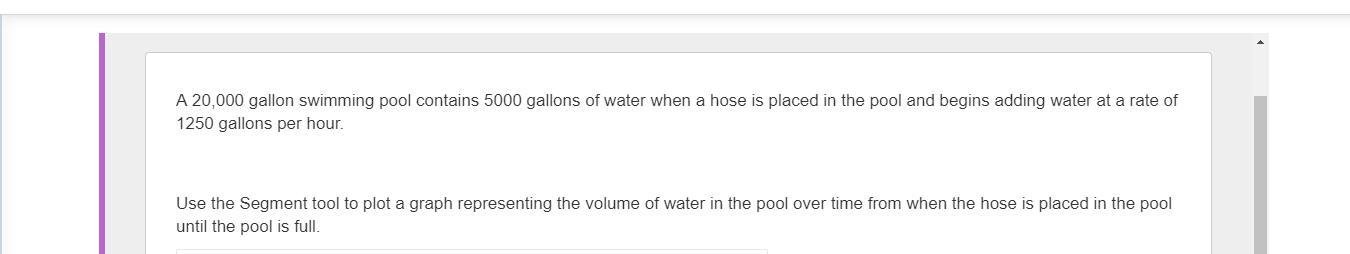

Answers
Answer:
20,000 divided by 4,250
Step-by-step explanation:
use cylindrical coordinates to evaluate the triple integral ∫∫∫ex2 y2−−−−−−√dv, where e is the solid bounded by the circular paraboloid z=1−9(x2 y2) and the xy -plane.
Answers
The triple integral ∫∫∫ex^2 y^2 dv in cylindrical coordinates evaluates to ∫ from 0 to 1, ∫ from 0 to 2π, and ∫ from 0 to (1-9r^2) e^r^2cos^2θsinr drdθdz.
In cylindrical coordinates, the given solid e is represented by the inequality 0 ≤ z ≤ 1-9r^2. Therefore, the limits of integration for z are 0 to 1-9r^2. The circular base of the solid is given by x^2 + y^2 ≤ 1/(9z), which can be rewritten as r^2 ≤ 1/(9z).
Thus, the limits of integration for r are 0 to √(1/(9z)). The angle θ ranges from 0 to 2π. Hence, the triple integral can be expressed as ∫ from 0 to 1, ∫ from 0 to 2π, and ∫ from 0 to √(1-9r^2) e^r^2cos^2θsinr drdθdz. Solving this integral yields the required answer.
For more questions like Integral click the link below:
https://brainly.com/question/18125359
#SPJ11
Find the product of 4V6 and 5V2 in simplest form.
Answers
Step-by-step explanation:
= 4√6 × 5√2
= (4 × 5)√(6 × 2)
= 20√12
= 20√(4.3)
= 2 × 20√3
= 40√3
WILL GIVE BRAINLIEST! Consider the graph at right and answer the following questions.

Answers
Answer:
a.)A and B are the intersection between the two curves. The x coordinate is the equation
\(f(x) = g(x)\)
Let's solve it
\(\frac12 (x-2)^3 +1 = 2x^2-6x-3\\x^3-6x^2+12x-8+2=4x^2-12x-6\\x^3-10x^2+24x=0\\x(x^2-10x+24)=0\\x(x-4)(x-6)=0\)
We can see that if we substitute x= 0 or x= 4 the equation is verified.
b. The equation has a third solution, x = 6, that is too far to the right to be visible in the graph.
c. the x coordinate of C is the solution of \(f(x)=0\) or \(\frac 12 (x-2)^3 +1=0\\(x-2)^3 = -2\\(x-2) = -\sqrt[3]2\)
At this point let's guesstimate the cubic root of 2 -let's say the cubic root of 2 is equal to 1plus an error e, and since we know how to calculate the cube of a binomial, let's build it.
\((1+e)^3 = 2 \rightarrow 1+3e+3e^2+e^3 = 2\) Now, assuming the error is small enough, it's square and its cube gets even smaller. (if the error is 0.1, it's square becomes 0.01 and the cube 0.01), let's ignore them. \(1+3e=2 \rightarrow 3e=1 e= \frac13\) So, going back to our equation, we can tell that the right hand side is [\(\mathbb{R}\)]-(1+\frac13)\) Not perfect, but good enough. Let's solve it now.
\(x-2= -1-\frac13 \rightarrow x=1-\frac13 = \frac23\)
Which seems close enough from the photo.
c. Domain of both function are the whole Real set, since they're polynomials. Range of f(x) is again the whole real set, since it's an odd degree polynomial. g(x) is a parabula and its range is for y above the vertex y-coord, which is \(y>g(\frac32) = 2(\frac94)-6(\frac32)-3 = \frac92 -3(\frac62) -\frac62 = \frac92-4\frac62= -\frac{24-9}2 = -\frac{15}2\)
Please help me I really don’t know

Answers
Answer:
acute-angled
Step-by-step explanation:
It doesn't have a right angle and one of its corners isn't for than 90 degrees
Pick all the correct statements from below. It is possible to have x
2
dx=0, ever when a pro 0 . ∥v
r
Av<0, then the matrix A rotates the vectore by moie than 90
∘
. The matrix B
2
AB is positive dehuite if A is pesitive thethite If the trace of a matik is zero, then it must be a singtalar matik. A rquare matrix and liss transpose both have the salle set of eheetwatiess A real shuare matix lias only real ehenvalues. Consider a matrix A∈R
6×8
whose rank is 4. Pick up the correct statements from the following. The dimension of the row space is 4. The dimension of the null space of A is 2 . The dimension of the left null-space is 4. Every element in the row space is mapped to a unique element in the column space by the linear transformation given by A. The dimension of the null space of A is 4.
Answers
The answer is, the correct statement are , 1. The matrix B² is positive definite if A is positive definite. ,2. A square matrix and its transpose have the same set of eigenvectors. , 3. A real square matrix has only real eigenvalues. , 4. The dimension of the row space of a matrix A with rank 4 is 4. , 5. The dimension of the null space of matrix A is 4.
the correct statements from the options provided:
1. The matrix B² is positive definite if A is positive definite.
2. A square matrix and its transpose have the same set of eigenvectors.
3. A real square matrix has only real eigenvalues.
4. The dimension of the row space of a matrix A with rank 4 is 4.
5. The dimension of the null space of matrix A is 4.
Please note that the statements regarding the values of x, dx, v, r, Av, and trace are incomplete or incorrect, so I have not included them in my answer.
To know more about Matrix visit:
https://brainly.in/question/13626298
#SPJ11
Find the rectangular coordinate for the polar coordinate (4,210^0)
Please I will give Brainly
Answers
The rectangular coordinate for the polar coordinate (4, 210°) is (-3.46, -2).
What is the rectangular coordinate?The rectangular coordinate for the polar coordinate (4, 210°) is calculated by applying the following formula as shown below;
x = r cos(θ)
y = r sin(θ)
where;
r is the distance from the origin of the coordinateθ is the angle or directionFrom the given polar coordinate (4, 210°);
r = 4
θ = 210⁰
The rectangular coordinate is calculated as follows;
x = 4 x cos(210°)
y = 4 x sin(210°)
x = -3.46
y = -2
Learn more about rectangular coordinate here: https://brainly.com/question/1402079
#SPJ1
If there were 500 students in Jamal’s class, approximately how many actual students scored higher than Jamal on the quiz if Jamal had a z-score of -1? a. 340 b. 420 c. 250 d. 490
Answers
it's 490 because when you get a zero or less it takes 10 points off your grade which leaves 490 students with good grades.
I need help with theses problems




Answers
Interchange (switch) the value of x and y in "y = x + 5" as x = y + 5, subtract 5 from both sides and re-write in the form "y = f(x)" as y = x - 5.
What is an inverse function?In Mathematics, an inverse function can be defined as a type of function that is obtained by reversing (undoing) the operation of a given function (f(x)).
The steps required to write the inverse of f(x) = x + 5 include the following:
Interchange (switch) the value of x and y in "y = x + 5" as x = y + 5.Subtract 5 from both sides, we have x - 5 = y.Re-write in the form "y = f(x)" as y = x - 5.Read more on inverse function here: https://brainly.com/question/10219387
#SPJ1
1. which of the following ordered pairs are equal ?
a. [7,6] and [2+5,3+3]
b. [1,6] and [6,1]
c. [-2,-3] and [-10/5,-6/2]
Answers
The equal ordered pairs are [7,6] and [2+5,3+3] and [-2,-3] and [-10/5,-6/2]. So, the correct answer is A) and C).
[7,6] and [2+5,3+3]
The ordered pair [7,6] represents a point in the coordinate plane that is 7 units to the right of the origin and 6 units above the origin.
The ordered pair [2+5,3+3] can be simplified to [7,6]. Therefore, the two ordered pairs are equal.
[1,6] and [6,1]
The ordered pair [1,6] represents a point in the coordinate plane that is 1 unit to the right of the origin and 6 units above the origin.
The ordered pair [6,1] represents a point in the coordinate plane that is 6 units to the right of the origin and 1 unit above the origin.
Therefore, the two ordered pairs are not equal.
[-2,-3] and [-10/5,-6/2]
The ordered pair [-2,-3] represents a point in the coordinate plane that is 2 units to the left of the origin and 3 units below the origin.
The ordered pair [-10/5,-6/2] can be simplified to [-2,-3]. Therefore, the two ordered pairs are equal.
Therefore, the ordered pairs are [7,6] and [2+5,3+3], [-2,-3] and [-10/5,-6/2] are equal pairs. So, the correct options are A) and C).
To know more about ordered pairs:
https://brainly.com/question/30805001
#SPJ1
30 POINTS AND BRAINLIEST HELP PLZ
Answers
Answer: What is the question?
Step-by-step explanation:
Suppose u and v are functions of x that are differentiable to d/dx (uv)= at x=0 and that u(0)=−5,u′ (0)=3,v(0)=2, and v′ (0)=−3. Find the values of the following derivatives at x=0 a. d/dx (uv) b. d/dx (u/v) c. d/dx (v/u) d. d/dx (6v−5u)
Answers
Values of the provided derivatives at x=0 are:
d/dx(uv) = 21, d/dx(u/v) = -9/4, d/dx(v/u) = 9/25, d/dx(6v-5u) = -33.
To obtain the values of the derivatives at x=0, we can use the rules of differentiation and the provided initial conditions.
a. To obtain d/dx (uv) at x=0, we can use the product rule:
d/dx (uv) = u'v + uv'
Substituting the initial conditions, we have:
d/dx (uv) = u'(0)v(0) + u(0)v'(0)
= 3 * 2 + (-5) * (-3)
= 6 + 15
= 21
Therefore, d/dx (uv) at x=0 is 21.
b. To obtain d/dx (u/v) at x=0, we can use the quotient rule:
d/dx (u/v) = (v * u' - u * v') / v^2
Substituting the initial conditions, we have:
d/dx (u/v) = (2 * 3 - (-5) * (-3)) / 2^2
= (6 - 15) / 4
= -9 / 4
Therefore, d/dx (u/v) at x=0 is -9/4.
c. To obtain d/dx (v/u) at x=0, we can use the quotient rule again:
d/dx (v/u) = (u * v' - v * u') / u^2
Substituting the initial conditions, we have:
d/dx (v/u) = (-5 * (-3) - 2 * 3) / (-5)^2
= (15 - 6) / 25
= 9 / 25
Therefore, d/dx (v/u) at x=0 is 9/25.
d. To obtain d/dx (6v - 5u) at x=0, we can use the sum and constant multiples rules:
d/dx (6v - 5u) = 6 * d/dx (v) - 5 * d/dx (u)
Substituting the initial conditions, we have:
d/dx (6v - 5u) = 6 * v'(0) - 5 * u'(0)
= 6 * (-3) - 5 * 3
= -18 - 15
= -33
Therefore, d/dx (6v - 5u) at x=0 is -33.
To know more about derivatives refer here:
https://brainly.com/question/32963989#
#SPJ11
Find the equation of a line through (-3,5) and perpendicular to (1,-3) (6,1)

Answers
Step-by-step explanation:
simply use the formula as given in the image
