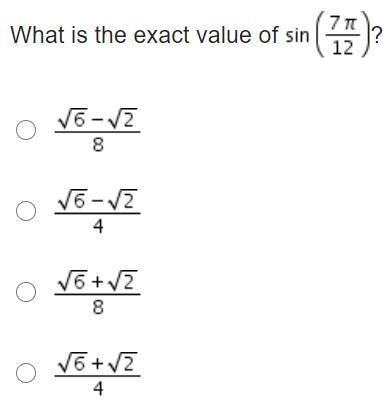Answers
Answer:
It is the 4th one
Step-by-step explanation:
The required value Sine (7pi/12) \(=\frac{\sqrt{6} +\sqrt{2} }{4}\). Option d is correct.
Value of Sine (7pi/12) to be evaluate.
What is simplification?It is to operate and interpret the function to make function simple or more understandable called simplification.
Here,
\(=sin(7\pi /12)\\=sin(105)\\=sin(60+45)\\= sin60cos45+cos45sin60\\=\sqrt{3} /2 *1/\sqrt{2} +1/\sqrt{2} *1/2\\\frac{\sqrt{6}+\sqrt{2} }{4}\)
Thus, the required value Sine (7pi/12) \(=\frac{\sqrt{6} +\sqrt{2} }{4}\).
Learn more about simplification here: https://brainly.com/question/12501526
#SPJ2
Related Questions
3/4 pages in 2 minutes how many pages in 1 minute
Answers
Answer:
3/8
Step-by-step explanation:
you can't split 3 in half to get a whole number, so you need to make it a different fraction with the same meaning to make it possible to divide by 2.
6/8 is the same as 3/4
divide by two to get 3/8 Don't divide the bottom number, just the top.
3/8 pages in one minute.
Hope this helps!
Michael budgets $600 of the $2,000 he brings home for
transportation expenses. What percent of the budget
does he allocate to transportation?
Answers
Answer:
600/2000= 30%
Step-by-step explanation:
The percentage of the budget Michael allocated to transportation is 30%
What is percentage?Percentage is defined as a given part or amount in every hundred. It is a fraction with 100 as the denominator and is represented by the symbol "%".
Given that, Michael budgets $600 of the $2,000 he brings home for
transportation expenses.
Here, percentage = 600/2000 ×100
= 600/20
= 30%
Therefore, the percentage of the budget Michael allocated to transportation is 30%
To learn more about the percentage visit:
brainly.com/question/24159063.
#SPJ2
Olivia and Jamie share
sweets in the ratio 3: 7. If
Olivia gets 27 how many
sweets does Jamie get?
Answers
Answer:
jamie gets 63
Step-by-step explanation:
27(olivias sweets)/3=9
9*7=63
=63
Answer:
63
Step-by-step explanation:
you have set them up so you can cross multiply.
(3 * x) = (7 * 27)
3x = (189)
to solve for x we need to isolate the variable, so divide both sides by 3:
x = 63
Use what you know about sine, cosine, and tangent to calculate the height of the buildings in the diagram below.

Answers
Answer:
x = 32 feet
Step-by-step explanation:
By applying tangent rule in ΔACD,
tan(40°) = \(\frac{\text{Opposite side}}{\text{Adjacent side}}\)
= \(\frac{AB+AC}{CD}\)
= \(\frac{x+BC}{87}\)
x + BC = 73 -----(1)
By applying tangent rule in ΔBCD,
tan(25°) = \(\frac{BC}{CD}\)
= \(\frac{BC}{87}\)
BC = 40.57
By substituting the value of BC in equation (1),
x + 40.57 = 73
x = 32.43
x ≈ 32 feet

Find the area of the composite figurep

Answers
Answer:
53.12 sq ft
Step-by-step explanation:
For triangle:
Base (b) = 8 ft
Height (h) = 7 ft
For semicircle:
Radius (r) = 8/2 = 4 ft
Area of the composite figure = Area of triangle + Area of semicircle.
\( = \frac{1}{2} bh + \frac{1}{2} \pi {r}^{2} \\ \\ = \frac{1}{2} (8)(7) + \frac{1}{2} (3.14)(4)^{2} \\ \\ = (4)(7) + (3.14)(8) \\ \\ = 28 + 25.12 \\ \\ = 53.12 \: {ft}^{2} \)
The following linear programming problem has been solved by The Management Scientist.
Use the output to answer the questions.
LINEAR PROGRAMMING PROBLEM
MAX 25X1+30X2+15X3 S.T.
1) 4X1+5X2+8X3<1200
2) 9X1+15X2+3X3<1500
OPTIMAL SOLUTION
Objective Function Value = 4700.000
Variable Value Reduced Cost
X1 140.000 0.000
X2 0.000 10.000
X3 80.000 0.000
Constraint Slack/Surplus Dual Price
1 0.000 1.000
2 0.000 2.333
OBJECTIVE COEFFICIENT RANGES
Variable Lower Limit Current Value Upper Limit
X1 19.286 25.000 45.000
X2 No Lower Limit 30.000 40.000
X3 8.333 15.000 50.000
RIGHT HAND SIDE RANGES
Constraint Lower Limit Current Value Upper Limit \
1 666.667 1200.000 4000.000
2 450.000 1500.000 2700.000
a. Give the complete optimal solution.
b. Which constraints are binding?
c. What is the dual price for the second constraint? What interpretation does this have?
d. Over what range can the objective function coefficient of x2 vary before a new solution point becomes optimal?
Answers
a. Optimal solution: X1 = 140, X2 = 0, X3 = 80, Objective Function Value = 4700.000.
b. The first constraint (4X1 + 5X2 + 8X3 < 1200) is binding.
c. The dual price for the second constraint is 2.333, indicating that for each unit increase in its right-hand side value, the objective function value increases by 2.333.
d. The objective function coefficient of X2 can vary between 30 and 40 without changing the optimal solution
a. The complete optimal solution is:
X1 = 140
X2 = 0
X3 = 80
Objective Function Value = 4700.000
b. The first constraint (4X1 + 5X2 + 8X3 < 1200) is binding because it has a slack/surplus value of 0.
c. The dual price for the second constraint (9X1 + 15X2 + 3X3 < 1500) is 2.333. This means that for each unit increase in the right-hand side value of the second constraint, the objective function value will increase by 2.333 units, assuming all other variables and constraints remain constant.
d. The objective function coefficient of X2 can vary between 30 and 40 before a new solution point becomes optimal. This means that as long as the coefficient remains within this range, the current solution will still be optimal. However, if the coefficient of X2 goes below 30 or above 40, a new optimal solution may be obtained.
Learn more about Objective Function here:
https://brainly.com/question/33272856
#SPJ11
PLZ HELP ME I WILL CALL YOU BRILLIANT Austin is comparing the prices of two car rental companies. Company A charges $24 per day and an additional $25 as service charges. Company B charges $22 per day and an additional $40 as service charges.
Part A: Write an equation to represent each company's total charges for renting a car for a certain number of days. For both equations (one for Company A and one for Company B), define the variable used.
Part B: Which company would charge less for renting a car for 4 days? Justify your answer.
Part C: How much money is saved by using the services of Company A instead of Company B to rent a car for 6 days?
Answers
Answer
Step-by-step explanation:
Its A
HI um yeaaaa so do u still need help and um this is taylor megan is at school and aaahhhhh umm yea so................... bye bye
5555555999933333888822228448845657483921234567
If the allele frequency of the dominant allele is 0.4, what value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1?
Answers
The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
According to the statement
we have given that the allele frequency of the dominant allele is 0.4, and we have to find that the value of p^2 in the equation p^2+ 2pq + q^2 = 1.
So, For this purpose, we know that the
The allele frequency represents the incidence of a gene variant in a population. Alleles are variant forms of a gene that are located at the same position, or genetic locus, on a chromosome.
And here
allele frequency is the 0.4 and represent the value of P.
So, The value of p is 0.4 and the
Then p^2 = (0.4)^2
so, the value becomes
p^2 = (0.4)^2
p^2 = 0.16.
So, The value is used for the term p^2 in the equation p^2+ 2pq + q^2 = 1 is 0.16.
Learn more about allele frequency here
https://brainly.com/question/6139888
#SPJ4
Write the equation that describes the line, in slope-intercept form.
The slope = 9,y-intercept = -1
Answers
Answer:
y = 9x -1
Step-by-step explanation:
The formula is y=mx+b
m = slope
b = y-intercept
All ya gotta do is plug in the slope and y-intercept then you have an equation!
Hope that helps and have a great day!
Bianca can run 5 miles in 41 minutes. Assuming she runs at a constant rate, write the linear equation that represents the situation.
Answers
Answer:
y=5/41x
Step-by-step explanation:
Alright, so we can get imagine two points. Imagine that the y axis is miles and the x axis is minutes. When bianca runs 0 miles, she would have run for 0 minutes, and we know that when Bianca runs 5 miles, she would have run for 41 minutes.
We can use slope formula using the change in y over change in x
\((y1 -y2)/(x1-x2)\\\)
So substituting values, we would get (5-0)/(41-0) or 5/41
slope is the letter m in the formula y=mx+b
so our equation would be \(y=\frac{5}{41}x+b\), b being the y intercept, which would be 0 as you cant run anything when no time has passed
Knowing all of this. the final equation is
\(y=\frac{5}{41}x\)
the average occupancy of vehicles that travel across the bridge is 1.6 people. what is the probability that you randomly select a vehicle with 2 occupants? (at) what probability distribution applies (binomial, geometric, or poisson)?
Answers
the probability of randomly selecting a vehicle with 2 occupants is approximately 0.245 or 24.5%.The probability distribution that applies in this scenario is the binomial distribution.
Let p be the probability of a vehicle having two occupants. Since the average occupancy is 1.6 people, we can calculate that the probability of a vehicle having one occupant is 1 - p - p = 0.4.
Using the binomial probability formula, the probability of selecting a vehicle with two occupants can be calculated as:
P(X = 2) = (100 choose 2) * p^2 * (1-p)^(100-2)
where (100 choose 2) is the number of ways to select 2 vehicles out of 100, p^2 is the probability of selecting a vehicle with two occupants twice, and (1-p)^(100-2) is the probability of selecting a vehicle with one occupant 98 times.
Assuming p = 0.16 (the average occupancy of 1.6 people divided by 2), we can calculate:
P(X = 2) = (100 choose 2) * 0.16^2 * 0.84^98 ≈ 0.245
Therefore, the probability of randomly selecting a vehicle with 2 occupants is approximately 0.245 or 24.5%.
To learn more about probability click here:brainly.com/question/32117953
#SPJ11
Question 1 You want to enclose a rectangular region with an area of 1200 square feet and a length of 40 feet, 50 feet, or 60 feet. Find the perimeter for each possible region.
Answers
The perimeter for each possible region is 140 feet, 148 feet, and 160 feet when the length of the rectangle is 40 feet, 50 feet or 60 feet, respectively.
Given that you want to enclose a rectangular region with an area of 1200 square feet and a length of 40 feet, 50 feet, or 60 feet.
We are to find the perimeter for each possible region.
Area of rectangle = length x breadth
Now, we are given the area of the rectangle as 1200 square feet and length as 40 feet, 50 feet or 60 feet.
Therefore, the breadth of the rectangle can be calculated as follows:
Breadth = Area/Length
We get the following breadth for each of the given lengths:
For length 40 feet,
breadth = 1200/40 = 30 feet
For length 50 feet, breadth = 1200/50 = 24 feet
For length 60 feet, breadth = 1200/60 = 20 feet
Now, we can use the formula to calculate the perimeter of each rectangle.
Perimeter of rectangle = 2 (length + breadth)
So, the perimeter for each possible region is given by:
Perimeter when length = 40 feet
Breadth = 30 feet
Perimeter = 2(40+30) = 2(70) = 140 feet
Perimeter when length = 50 feet
Breadth = 24 feet
Perimeter = 2(50+24) = 2(74) = 148 feet
Perimeter when length = 60 feet
Breadth = 20 feet
Perimeter = 2(60+20) = 2(80) = 160 feet
Therefore, the perimeter for each possible region is 140 feet, 148 feet, and 160 feet when the length of the rectangle is 40 feet, 50 feet or 60 feet, respectively.
To know more about perimeter visit:
https://brainly.com/question/7486523
#SPJ11
First try was incorrectJob #1 pays $52.50 for 7 hours of work. Job #2 pays $78 for 12 hoursof work.What does Job #1 pay in dollars per hour (to the nearest cent)?label required
Answers
To answer this question, we need to use the concept of unit rate. In this case, to find the payment per hour of Job number 1, we can proceed as follows:
\(\frac{52.50\text{dollars}}{7\text{hours}}=\frac{7.5d}{h}\)Therefore, Job number one pays $7.50 per hour (to the nearest cent).
(We divided the total amount of money by the total amount of time to find the number of dollars per hour.)
if p=(3,1) and Q=(-3,-7), find the equation of the circle that has segment PQ as the diameter (x-{?})^2+(y-{?})^2={?}
Answers
Answer:
x² + (y + 3)² = 25
Step-by-step explanation:
the centre (C) of the circle is at the midpoint of the diameter.
using the midpoint formula
midpoint = ( \(\frac{x_{1}+x_{2} }{2}\) , \(\frac{y_{1}+y_{2} }{2}\) )
with (x₁, y₁ ) = P (3, 1 ) and (x₂, y₂ ) = Q (- 3, - 7 )
C = ( \(\frac{3-3}{2}\) , \(\frac{1-7}{2}\) ) = ( \(\frac{0}{2}\) , \(\frac{-6}{2}\) ) = (0, - 3 )
the radius r is the distance from the centre to either P or Q
using the distance formula
r = \(\sqrt{(x_{2}-x_{1})^2+(y_{2}-y_{1})^2 }\)
with (x₁, y₁ ) = C (0, - 3 ) and (x₂, y₂ ) = P (3, 1 )
r = \(\sqrt{(3-0)^2+(1-(-3)^2}\)
= \(\sqrt{3^2+(1+3)^2}\)
= \(\sqrt{3^2+4^2}\)
= \(\sqrt{9+16}\)
= \(\sqrt{25}\)
= 5
the equation of a circle in standard form is
(x - h)² + (y - k)² = r²
where (h, k ) are the coordinates of the centre and r is the radius
here (h, k ) = (0, - 3 ) and r = 5 , then
(x - 0 )² + (y - (- 3) )² = 5² , that is
x² + (y + 3)² = 25
Determine the type of correlation represented in the scatter plot below.
A. a perfect positive correlation
B. a strong positive correlation
C. a weak positive correlation
D.no correlation
E. a weak negative correlation
F. a strong negative correlation
G. a perfect negative correlation

Answers
Answer:
f is correct
Step-by-step explanation:
A weak negative correlation. Correct option is E.
What is a correlation?A correlation between two variables tells the how the first variable will change, with change in second variable.
Given,
Value of y decreases as the value of x increases and vice versa.
Also, x and y are nearly linearly related.
But, there is still some deviation in the values and are not perfectly linear.
Hence, there is a weak negative correlation between x and y.
To learn more about correlation visit:
https://brainly.com/question/28898177
#SPJ1
Given A (-3,5) and B (6,7) find AB
Answers
Answer:2/9
Step-by-step explanation:
AB=\(\frac{7-5}{6+3}\)
=\(\frac{2}{9}\)
find x using
dialations

Answers
Answer:
x = 14.4
Step-by-step explanation:
Using dilations, the following equation can be written to show the relationship between the two similar triangles:
x/4 = (6.5 + 2.5)/2.5
x/4 = 9/2.5
Cross multiply
x*2.5 = 9*4
2.5x = 36
2.5x/2.5 = 36/2.5
x = 14.4
The library surveyed a total of 180 people about their favorite type of movie. If 40% of the people chose action movies, what part of the people chose action movies?
Answers
When adding and subtracting rationals, what should be the first thing you find? How do you find it?
Answers
Typically you should factor the denominators first, as it will make getting the least common multiple easier. For the first denominator, you can factor out x^2, the second denominator is a perfect square, (x^2+1) (x^2-1) and the last denominator is already simplified. It appears the denominators don't have a common factor though, so yes, you would have to multiply each of the denominators out.
this wont be graded but i need the help with it

Answers
Answer:
f(-1) = 0
f(0) = -1
f(1) = 0
Explanation:
Given the below function;
\(f(x)=x^2-1\)We're to find the corresponding values of f(x) given different values of x.
To determine the value of f(x) for each value of x, all we need to do is to substitute the said value of x into the function and solve for f(x).
When x = -1, let's go ahead and find f(-1);
\(f(-1)=(-1)^2-1=1-1=0\)When x = 0, let's solve for f(0);
\(f(0)=(0)^2-1=0-1=-1\)When x = 1, let's solve for f(1);
\(f(1)=(1)^2-1=1-1=0\)A storage locker measures 8 feet wide, 12 feet deep, and 9 feet high. The monthly rental price for the locker is $3.60 per cubic yard. How much does is cost to rent the locker each month? Complete the explanation to show your answer.
Answers
Answer:
The cost is $115.20
Step-by-step explanation:
The locker is 8ft by 12ft by 9ft.
The volume is:
V = 8 × 12 × 9
V = 864 cubic feet
But the price is given by cubic yards so we need to find that first. (we have cubic feet)
A cubic yard is
1yd × 1yd × 1yd
And a yard is 3 feet, so that is the same as:
3ft × 3ft × 3ft
= 27cubic feet
Divide:
864/27
= 32
The locker is 32 cubic yards.
The price is $3.60 per cubic yard. Multiply.
32 × 3.60
= 115.2
The cost of the locker will be $115.20.
Evaluate the expression for b = 3 and c = 5.
b2c − 11 =
Answers
Answer:
34Step-by-step explanation:
b = 3 and c = 5Substitute and evaluate:
b²c - 11 =3²*5 - 11 = 9*5 - 11 =45 - 11 =34Answer:
\(b = 3 \ \: \: : c = 5 \\ \\ b {}^{2} c - 11 = \\ \\ \\ {3}^{2} \times 5 - 11 = \\ \\ 9 \times 5 - 11 = \\ \\ 45 - 11 = \\ \\ = 34\)
Hi, I need help with this question. Here is the question: For which data set is the median greater than the mean?
(1) { 4,7,10,13,16} (3) {8,9,10,11,12}
(2) {8,9,10,18,19} (4) {1,2,10,11, 12}
Please give me the answer(the method used for this problem) and an explanation.
Answers
Answer:
Set 2
Step-by-step explanation:
First find the median of each set (the median being the number in the middle):
(1) { 4,7,10,13,16} = 10
(2) {8,9,10,18,19} = 10
(3) {8,9,10,11,12} = 10
(4) {1,2,10,11, 12} = 10
Then find the mean of each set (the mean being average of all the numbers, to find the average add up all the numbers and divide by the amount of numbers):
(1) { 4,7,10,13,16} = 10
(2) {8,9,10,18,19} = 12.8
(3) {8,9,10,11,12} = 10
(4) {1,2,10,11, 12} = 7.2
the answer being 12.8 since 12.8 is greater than 10
(4) {1,2,10,11, 12}
Answer:
Solution given:
1:
{ 4,7,10,13,16}
n=5
median={n+1)/2th term=6/2=3th term=10
mean=sum/n={ 4+7+10+13+16}/{5}=10
(2)
{8,9,10,18,19}
n=5
median={n+1)/2th term=6/2=3th term=10
mean=sum/n={8+9+10+18+19}/{5}=12.8
3) {8,9,10,11,12}
n=5
median={n+1)/2th term=6/2=3th term=10
mean=sum/n={8+9+10+11+12}/{5}=10
(4) {1,2,10,11, 12}
n=5
median={n+1)/2th term=6/2=3th term=10
mean=sum/n={1+2+10+11+13}/{5}=7.4
In above
1: mean=median
2:mean>median
3:mean=median
4:mean<median
so median is greater than mean is in 4th one.
(4) {1,2,10,11, 12}
In a 5% sale, the price of a phone is reduced by £19.4 Find the original price of the phone
Answers
Answer:
The original price of the phone is £388.
Step-by-step explanation:
Since 5% of the price of the phone is reduced, you can simply multiply the reduced number which is £19.4 by 20. Your final answer should be £388 when you put it on a calculator or do it on a piece of paper.
What happened to Christianity in Britain after the collapse of the Roman empire?
NEED DONE ASAP
Answers
Answer: After the collapse of Roman Empire, much of southern and eastern Britain was affected by the Anglo-Saxon migrations and a transition to Anglo-Saxon paganism as the primary religion. ... There remained an awareness among Anglo-Saxon Christian writers like Bede that a Romano-British Christianity had existed.
Step-by-step explanation: I hope this helps you
A rectangular plot measures 12 m by 5 m. A path of constant width runs along one side and one end. If the total area of the plot and the path is 120 m², find the width of the path.
Answers
Answer:
the width of the path is 24m²
Part E
The baseball team will break even when the amount of money it has received equals the amount of money it spent. In other words, the team
breaks even when the profit is equal to zero. How many cars does the baseball team have to wash to break even? What point represents this
value? What does the x-value of this point mean in terms of the problem?

Answers
Answer:
Step-by-step explanation:
Let y represent the amount of money earned, and x the number of cars they wash. Then
y = 5x
Why? Each car wash costs $5. So no car washes means no earnings; 1 car wash earns $5; 2 washes earn $10; 3 washes earn $15, and so on, so that x car washes generate $5x.
2. No matter how many cars they end up washing, they will have spent $75 to set up the operation, so you subtract 75 from the equation is part (1).
3. Taking into account the setup cost of $75, the profit they make is given by
y = 5x - 75
Suppose you've calculated a Pearson correlation a coefficient and had a final value of +10. How do you interpret this result? a O impossible value of r O significant correlation O positive correlation O negative correlation
Answers
If a Pearson correlation coefficient has a final value of +10, interpretation of this result is that it is an impossible value of r. Therefore, the correct option is A.
It is given that the Pearson correlation coefficient you calculated has a value of +10. This is actually an impossible value of r. Pearson correlation coefficient (r) ranges between -1 and +1. A value of +1 indicates a perfect positive correlation, while a value of -1 indicates a perfect negative correlation. A value of 0 suggests no correlation between the variables.
A value of +10 would fall outside of this range, making it an impossible value of r. Therefore, this result cannot be interpreted in terms of positive or negative correlation, nor can it be considered a significant correlation. It is important to ensure that the range of possible values is considered when interpreting statistical results. Hence, the correct answer is option A.
Learn more about Pearson correlation:
https://brainly.com/question/4117612
#SPJ11
Which expressions are equivalent to 3(2x - 4)? Mark all that apply.
Group of answer choices
6x - 1
6x - 12
3x - 12
2(3x - 6)
2(3x - 4)
6(x - 2)
Answers
Answer:
6x - 12 , 2(3x - 6) and 6(x - 2)
Step-by-step explanation:
You must times 3 by each of the numbers and variables within the brackets
For example: 3x 2x = 6x and 3 x 4 = 12
What is mean reversion? How is mean reverting level x1 is calculated for time series? How is it interpreted?
Answers
Mean reversion is the tendency of prices or variables to return to their average level. The mean-reverting level, x1, is calculated using statistical methods and indicates potential future decreases or increases.
Mean reversion refers to the tendency of asset prices or economic variables to move back to their average or mean level over time. The mean-reverting level, x1, for a time series can be calculated using statistical methods like moving averages or exponential smoothing. These techniques estimate the average value or trend of the data.
The interpretation of x1 depends on the context. If the current value is above x1, it suggests a potential future decrease, reverting back to x1. Conversely, if the current value is below x1, it indicates a potential future increase, also reverting back to x1. The deviation from x1 provides insights into the strength or speed of the mean reversion process.
Therefore, Mean reversion is the tendency of prices or variables to return to their average level. The mean-reverting level, x1, is calculated using statistical methods and indicates potential future decreases or increases.
To learn more about statistical click here
brainly.com/question/33214409
#SPJ11