What is the scale factor of the enlargement of shape A to shape B?

Answer:

The equation that has a vertex in (0, -5) is the first one.

Step-by-step explanation:

A standard form second degree equation is given by the following expression:

\(y(x) = a*x^2 + b*x + c\)

For which the vertex coordinates can be calculated by:

\(x_{vertex} = -\frac{b}{2*a}\)

While "y" for the vertex can be found by applying this coordinate on the expression. Using this knowledge in each equation gives us:

1. \(y(x) = x^2 - 5\)

\(a = 1\\b = 0\\c = -5\)

Therefore the vertex coordinate is:

\(x_{vertex} = -\frac{b}{2*a} = -\frac{0}{2*1} = 0\)

\(y_{vertex} = 0^2 - 5 = -5\)

This parabola has a vertex in (0,-5).

2. \(y(x) = x^2 + 5\)

\(a = 1\\b = 0\\c = 5\)

Therefore the vertex coordinate is:

\(x_{vertex} = -\frac{b}{2*a} = -\frac{0}{2*1} = 0\)

\(y_{vertex} = 0^2 + 5 = 5\)

This parabola has a vertex in (0,5).

3. \(y(x) =(x+ 5)^2 = x^2 + 10*x + 25\)

\(a = 1\\b = 10\\c = 25\)

Therefore the vertex coordinate is:

\(x_{vertex} = -\frac{b}{2*a} = -\frac{10}{2*1} = -5\)

\(y_{vertex} = (-5)^2 + 10*(-5) + 25 = 0\)

This parabola has a vertex in (-5,0).

4. \(y(x) =(x- 5)^2 = x^2 - 10*x + 25\)

\(a = 1\\b =- 10\\c = 25\)

Therefore the vertex coordinate is:

\(x_{vertex} = -\frac{b}{2*a} = -\frac{-10}{2*1} = 5\)

\(y_{vertex} = (5)^2 - 10*(5) + 25 = 0\)

This parabola has a vertex in (5,0).

Answer:

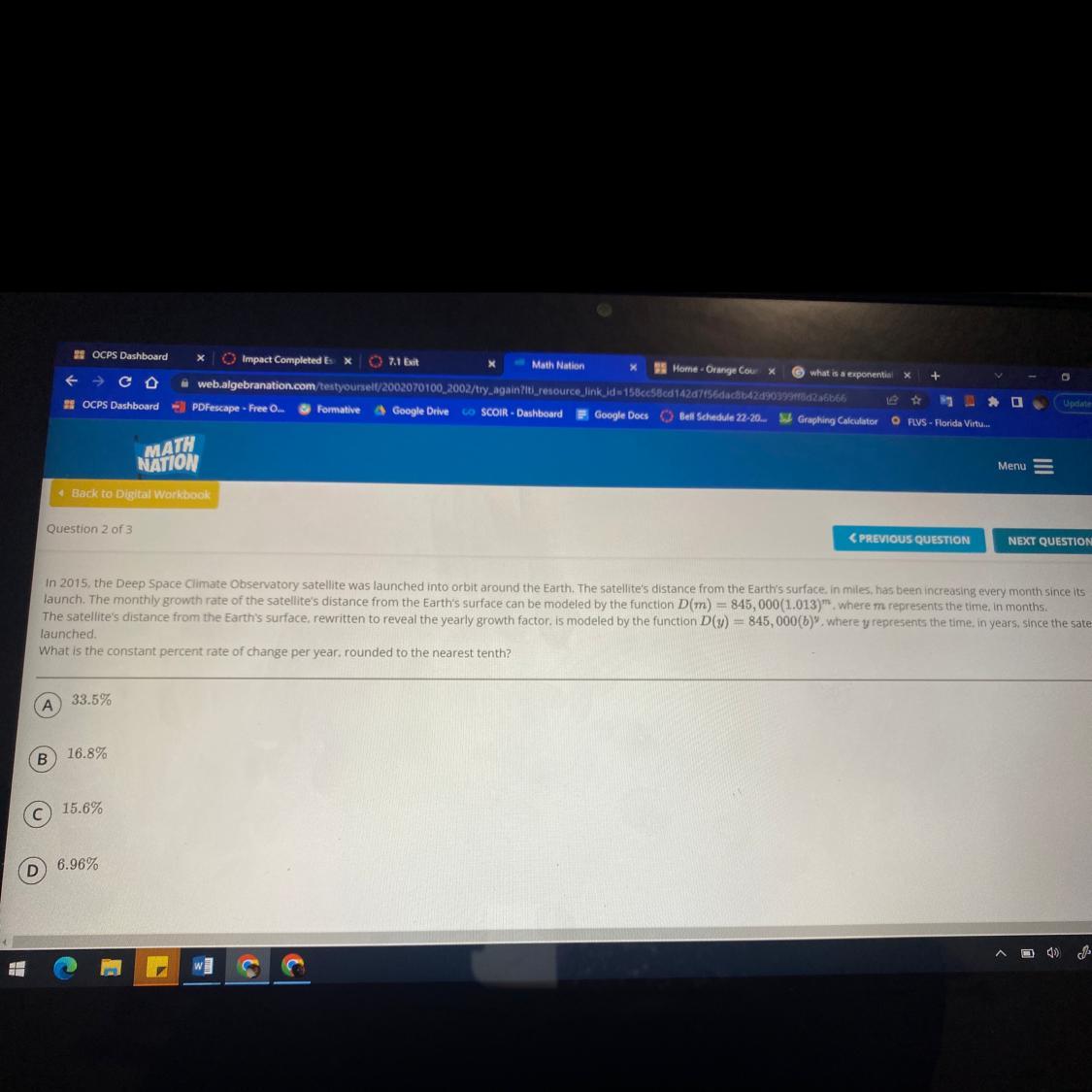
the first forth and fifth answers are correct.

Step-by-step explanation:

8 times 1.25 = 10

8 time 1.70 = 13.60

8 times 1.75 = 14

Step-by-step explanation:

let the number be x

and according to question,

six + 2 times a number = 2x + 6

26 + 6 times a number = 6x + 26

→ 2x + 6 = 6x + 26

→ -6x + 2x = 26 - 6

→ -4x = 20

→ x = 20/4 = 5

→ x = 5

therefore, the number is 5.

hope this answer helps you dear...take care and may u have a great day ahead!

Answer:

9) (68 + 160)/2 = 114°

10) 80/2 = 40°

11) 105 x 2 = 210°

12) 80 x 2 = 160°

13) (360 - 180 - 108)/2 = 36°

14) (360 - 69 - 95)/2 = 98°

The constant percent rate of change per year 33.5 %. So the correct option is A.

Growth factor is a mathematical concept that represents the amount by which a quantity increases or decreases over a period of time. It is usually expressed as a decimal or a percentage. A growth factor greater than 1 indicates an increase in the quantity, while a growth factor less than 1 indicates a decrease. A growth factor of 1 indicates no change in the quantity.

For example, if the population of a city is growing by 2% per year, the growth factor would be 1.02, since the population is increasing by 2% (0.02) every year. If the population was decreasing by 3% per year, the growth factor would be 0.97, since the population is decreasing by 3% (0.03) every year.

To find the constant percent rate of change per year, we need to rewrite the function D(m) in terms of years, and then find the annual growth factor.

First, we can divide m by 12 to get the time in years:

m/12 = y

Substituting this into the function D(m) gives:

D(m) = 845,000(\(1.013^{m}\)) = 845,000(\(1.013^{12y}\))

Now we have D(y) in terms of the annual growth factor b:

D(y) = 845,000(\(b^{y}\)), where b = 1.013¹²

To find the constant percent rate of change per year, we need to find the value of b-1 as a percent:

(b-1)*100

= (1.013¹² - 1)*100

= 33.5% (rounded to the nearest tenth)

Therefore, the answer is (A) 33.5%.

To know more about function visit:

https://brainly.com/question/16142287

#SPJ1

The probability of all 4 computers being good was 45.62%, the probability of 3 being good and 1 being defective was 4.58%, and the probability of 2 being good and 2 being defective was 2.29%.

1. The probability that all 4 are good is 90/100 x 89/99 x 88/98 x 87/97 = 0.4562, or 45.62%.

2. The probability that 3 are good and 1 is defective is 90/100 x 89/99 x 88/98 x 10/97 = 0.0458, or 4.58%.

3. The probability that 2 are good and 2 are defective is 90/100 x 89/99 x 10/98 x 10/97 = 0.0229, or 2.29%.

For the first problem, we can use the formula P(A) = n(A)/n(T), which is the probability of an event A occurring. In this case, P(A) = n(A)/n(T) = 4/100 = 0.04. This gives us the result of 0.4562, or 45.62%.

For the second problem, we use the same formula P(A) = n(A)/n(T), which is the probability of an event A occurring. In this case, P(A) = n(A)/n(T) = 3/100 = 0.03. This gives us the result of 0.0458, or 4.58%.

For the third problem, we use the same formula P(A) = n(A)/n(T), which is the probability of an event A occurring. In this case, P(A) = n(A)/n(T) = 2/100 = 0.02 ,then the probability of the next event, which is 89/99, then the probability of the next event, which is 10/98, then the probability of the last event, which is 10/97. This gives us the result of 0.0229, or 2.29%.

Learn more about probability here:

https://brainly.com/question/30034780

#SPJ4

Answer: 5/2x-3

Step-by-step explanation: you would subtract 6 from both sides.

5x = 2y + 6

-6            -6

6-6=0 so they would cross out. and you can't subtract 5x from 6 because they aren't like terms. Your new equation would look like 5x-6 = 2y.

Now you need to get the y alone. To do that you need to divide everything by 2 so that the 2y crosses out. 5x-6 = 2y

                                                                            -----------------

                                                                             2    2    2

I'm trying to visually show it sorry if it looks weird. one you divide everything. (5/2) (-6/2) (2/2) you'll need to clean everything up.

Since you can't simply 5/2 leave it as a fraction. Which makes your answer: 5/2x - 3 = y or y = 5/2x - 3

Answer:

  50%

Step-by-step explanation:

You want the probability of rolling a divisor of 45 on a 6-sided die.

The probability is the ratio of outcomes of interest to the total number of possible outcomes, assuming they are equally likely.

The numbers on a die that are divisors of 45 are 1, 3, 5, which are 3 of the 6 possible outcomes.

  P(divisor of 45) = 3/6 = 1/2 = 0.50 = 50%

The probability of rolling a divisor of 45 is 50%.

__

Additional comment

This problem statement asks for the answer as a percentage. It can also be written as a fraction (1/2) or a decimal (0.50).

The divisors of 45 are 1, 3, 5, 9, 15, 45.

<95141404393>

(1) As a result, the Monitor costs $275 to buy online. (2) There is a 20% price disparity between the two retailers.

10,000 pairs of shoes are ordered by Shoes Unlimited from an overseas vendor. This entails the business issuing a purchase requisition, which serves as a formal promise to buy the specified kind and number of shoes.

(a) To find the price of the TV when purchased online, we can add 25% markup to the manufacturer's price of $220:

Price online = $220 + (0.25 * $220)

Price online = $220 + $55

Price online = $275

So the price of the TV when purchased online is $275.

To find the price of the TV when purchased at the superstore, we can add 45% markup to the manufacturer's price of $220:

Price at superstore = $220 + (0.45 * $220)

Price at superstore = $220 + $99

Price at superstore = $319

So the price of the TV when purchased at the superstore is $319.

(b) The difference between the prices in part (a) is:

Price at superstore - Price online = $319 - $275 = $44

The difference in markup between the two stores is:

Markup at superstore - Markup online = 45% - 25% = 20%

To know more about Purchased visit:

https://brainly.com/question/28717901

#SPJ1

Answer: y = $35 & 3 weeks

Step-by-step explanation:

Answer:

A) 3 week

B) $35

Step-by-step explanation:

$20-$5=15

$20+$15=35

Answer: B?

Step-by-step explanation:

Answer:

Go for B

Step-by-step explanation:

Answer:

To simplify the expression 12d + 7d - 9d, we can combine the like terms (terms with the same variable, in this case, "d"):

12d + 7d - 9d

Combining the coefficients:

(12 + 7 - 9)d

Simplifying the coefficients:

10d

Therefore, 12d + 7d - 9d simplifies to 10d.

Answer:

To simplify the expression 12d + 7d - 9d, we can combine the like terms (terms with the same variable, in this case, 'd').

12d + 7d - 9d can be rewritten as (12 + 7 - 9)d.

Simplifying the coefficients within the parentheses, we have 10d.

Therefore, 12d + 7d - 9d simplifies to 10d.

Question 3: True

Question 4: False

Question 5: True

If the derivative of a function, f'(x), is positive for values of x less than c and negative for values of x greater than c, then it indicates a change in the slope of the function. This change from positive slope to negative slope suggests that the function has a maximum value at x = c.

This is because the function is increasing before x = c and decreasing after x = c, indicating a peak or maximum at x = c.

Question 3: If f'(c) < 0 then f(x) is decreasing and the graph of f(x) is concave down when x = c.

True

When the derivative of a function, f'(x), is negative at a point c, it indicates that the function is decreasing at that point. Additionally, if the second derivative, f''(x), exists and is negative at x = c, it implies that the graph of f(x) is concave down at that point.

Question 4: A local extreme point of a polynomial function f(x) can only occur when f'(x) = 0.

False

A local extreme point of a polynomial function can occur when f'(x) = 0, but it is not the only condition. A local extreme point can also occur when f'(x) does not exist (such as at a sharp corner or cusp) or when f'(x) is undefined. Therefore, f'(x) being equal to zero is not the sole requirement for a local extreme point to exist.

Question 5: If f'(x) > 0 when x < c and f'(x) < 0 when x > c, then f(x) has a maximum value when x = c.

True

If the derivative of a function, f'(x), is positive for values of x less than c and negative for values of x greater than c, then it indicates a change in the slope of the function. This change from positive slope to negative slope suggests that the function has a maximum value at x = c. This is because the function is increasing before x = c and decreasing after x = c, indicating a peak or maximum at x = c.

Learn more about  Polynomial Function at

brainly.com/question/11298461

#SPJ4

Answer:

-5/8, 1/3, -3/2

Step-by-step explanation:

1.

-7/8+1/4

-7/8+2/8

(-7+2)/8

-5/8

2.

-19/3+20/3

1/3

3.

2-7/2

(4-7)/2

-3/2

1-

-7/8+1/4

-7/8+2/8

(-7+2)/8

-5/8

2-

Step 1;

Step 2:

Final answer

Therefore, the probability that the sample will not contain a defective computer

Answer:

8%

Step-by-step explanation:

Hello,

8% of the students take neither History or French

so we have 8*200/100=8*2=16 students  out of French and History

let s say that

a is the number of students taking only History

b is the number of students taking both History and French

c is the number of students taking only French

10% of those who take History also take French

so 0.10(a+b)=b <=> 0.10a+0.10b=b

<=> 0.10a+0.10b-0.10b=b-0.10b=0.9b

<=> 0.10a=0.90b

let's multiply by 10 it comes a = 9b

4 times as many students take History as take French

so a + b = 4 (b + c)

it comes 9b + b = 10b = 4b + 4c

<=> 10b-4b=4b+4c-4b=4c

<=> 6b=4c

<=> 3b=2c

<=> c = 3b/2

and we know that a + b + c = 200 - 16 = 184

so

9b + b + 3b/2 = 184 we can multiply by 2 it comes

20 b + 3b = 184*2

23b = 184*2 = 23 * 8 *2 = 23*16

b = 23*16/23 = 16

so b = 16

c = 3*16/2 = 24

c = 24

a = 9b = 144

a = 144

you can see the Venn diagram below

and then the probability that a student picked at random does History and French is 16/200 = 8%

so the answer is 8%

hope this helps

The margin of error is 2.326 for the supplied values of  c, σ, and n, which are 0.98, 0.78, and 150 respectively.

Margin is the term used to describe the equity that a trader has in their account. Using funds borrowed from a broker to buy stocks is referred to as "marging" or "buying on margin." Instead of a typical brokerage account, you must have a margin account to execute this. Beginning with your gross profit, which is the distinction between revenue and COGS, you may compute profit margin. Find the percentage of revenue that represents gross profit next. Calculate it by dividing your gross profit by your revenue. You can calculate your margin % by multiplying the sum by 100.

Given that,

Population standard deviation =  

 = 0.78

Sample size = n =150

α = 1 - 98% = 1 - 0.98 = 0.02

α= 2 = 0.02/ 2 = 0.01

Z*α = 2 = 0.01 = 2.326     (  Using z table    )  

Margin of error = E =  Z

The margin of error is 2.326 for the supplied values of  c, σ, and n, which are 0.98, 0.78, and 150 respectively.

To know more about margin visit :-

https://brainly.com/question/28856941

#SPJ4

Answer:

ok so

faf

Step-by-step explanation:

Factor 20x2 + 25x – 12x – 15 by grouping.

1. Group terms with common factors.

2. Factor the GCF from each group.

3. Write the polynomial as a product of binomials.

(20x2 – 12x) + (25x– 15)

4x(5x – 3) + 5(5x – 3)

(5x – 3)(

x +  

)

The formula or equation used to determine the slope of a straight line is as follows:

m = (Y₂-Y₁)/(X₂-X₁)

An equation is about two expressions, either arithmetic or algebraic, that are related with a "=" sign that indicates equality of expressions.

Equations can be graphed, they are used to model many problems and theories.

The straight lines are characterized by a finite succession of points, when they have an inclination they adopt a slope value different from 0, and to determine that slope it is necessary to know two points of the line and use the following equation:

m = (Y₂-Y₁)/(X₂-X₁)

Where the points are:

Learn more about equations at:

brainly.com/question/8806877

#SPJ4

If a graph has vertices and edges such that there are no cycles (i.e. it is acyclic) and it is connected, then the graph must be a tree.

To prove this, we first note that a tree is defined as a connected, acyclic graph. Therefore, if we can show that a graph with the given properties is also connected and acyclic, then it must be a tree.

First, let us prove that the graph is connected. Since there are no cycles, every vertex must be part of a path. Moreover, since there are no isolated vertices, every vertex is connected to at least one other vertex. Therefore, if we start at any vertex and follow any path, we will eventually reach all other vertices. Hence, the graph is connected.

Next, let us prove that the graph is acyclic. Suppose, for the sake of contradiction, that the graph contains a cycle. Let v1, v2, ..., vn be the vertices of the cycle in order. Since the graph is connected, there must be a path from v1 to vn that does not include any of the vertices v2, ..., vn-1 (otherwise, we could "short-circuit" the cycle). But this path, together with the edges (v1, v2) and (vn-1, vn), forms a cycle, contradicting our assumption that the graph is acyclic. Therefore, the graph is acyclic.

Since the graph is both connected and acyclic, it must be a tree. Therefore, a graph with vertices and edges that satisfies the given conditions must be a tree.

No, the graph does not necessarily have to be a tree.

The formula v = e + 1 signifies that one more than the number of edges (e) is the number of vertices (v).

This formula is valid for all connected graphs with one extra edge, besides the necessary edges for forming a tree.

Thus, it is possible that the diagram remains with loops and more offshoots, classifying it as a graph that is connected, yet not a tree.

The equation v = e + 1 does not limit the graph's structure beyond its connectedness.

Learn more about vertices at: https://brainly.com/question/3050890

#SPJ4

The degree measure for angle B is 28° and angle D is 62°.

We are given that:

∠ C = 90°

∠ B = ( 2 x + 10 )°

∠ D = ( 5 x + 17 )°

Now, using the angle sum property of a triangle, we get that:

∠B + ∠ C + ∠ D  = 180°

Substituting the values:

2 x + 10 + 90 + 5 x + 17 = 180

7 x + 117 = 180

7 x = 63

x = 63 / 7

x = 9

∠ B = ( 2 x + 10 )

∠ B = 2(9) + 10

∠ B = 18 + 10

∠ B = 28°

∠ D = ( 5 x + 17 )

∠ D = 5 (9) + 17

∠ D = 45 + 17

∠ D = 62°

Therefore, the degree measure for angle B is 28° and angle D is 62°.

Learn more about angle here:

https://brainly.com/question/25716982

#SPJ9

Answer:

4-5x

5x-4

Step-by-step explanation:

Answer:

4g = 8

You would just have to divide 4 on both sides. The 4's would cancel out and now your left with 8÷4 = 2

Your answer would be

g = 2

If the circumference of the circle is 153.86 units, then the radius of that  circle is 24.5 units.

The "Circum-ference" of circle is defined as "total-length" of boundary of the circle. The formula for circumference is = 2 × π × Radius,

The "Radius" of circle is the distance from center of circle to its outer edge. It is half of "diameter" of circle, and it is a measure of size of circle.

We know that, circumference of circle is 153.86 units,

So, the circumference equation becomes,

⇒ 2 × 3.14 × Radius = 153.86,

⇒ 153.86/(2 × 3.14) = Radius,

⇒ Radius ≈ 24.5

So, the radius of the circle is approximately 24.5 units.

Learn more about Circumference here

https://brainly.com/question/16586639

#SPJ4

The given question is incomplete, the complete question is

What is the radius of the circle if it has a circumference of 153.86 units?

By using  the sum or difference formula of cosine to solve cos(525°) we get cos(525°) = -0.465

The formula to find the value of cos(A ± B) is given as,

cos(A + B) = cosA cosB − sinA sinBcos(A − B) = cosA cosB + sinA sinB

Here, A = 450° and B = 75°

We can write 525° as the sum of 450° and 75°.

Therefore,cos(525°) = cos(450° + 75°)

Now, we can apply the formula for cos(A + B) and solve it.

cos(A + B) = cosA cosB − sinA sinBcos(450° + 75°) = cos450° cos75° − sin450° sin75°= 0.707 × 0.259 − 0.707 × 0.966= -0.465

Substituting the values in the above equation, we get

cos(525°) = 0.707 × 0.259 − 0.707 × 0.966= -0.465

Thus, cos(525°) = -0.465.

To know more about cosine visit:

brainly.com/question/29114352

#SPJ11

The point estimate of the difference in the population mean exercised between underclassmen and upperclassmen is 0.16.


To find the point estimate of the difference in the population mean exercised between underclassmen and upperclassmen, you need to subtract the sample mean of underclassmen from the sample mean of upperclassmen.

Sample mean of upperclassmen = 0.76
Sample mean of underclassmen = 0.60

Point estimate = Sample mean of upperclassmen - Sample mean of underclassmen
Point estimate = 0.76 - 0.60
Point estimate = 0.16


The point estimate of the difference in the population mean exercised between underclassmen and upperclassmen is 0.16, which indicates that on average, upperclassmen exercised 0.16 hours more than underclassmen in the past 24 hours.

To know more about Point estimate, visit

https://brainly.com/question/30734674

#SPJ11

Answer:$22.50

Step-by-step explanation:

2.5/100=0.025

900*0.025=22.50

so he made $22.50

Answer:

Step-by-step explanation:

183.5

Answer:

the answer is 183.5

Step-by-step explanation:

Answer:

m∠1 = 147°

Step-by-step explanation:

(5x-2)° and ∠1 are supplementary to each other, which means they add up to 180°. However, we don't know the measure of ∠1. So, we need to solve for x to figure out angle (5x-2)°'s measure. Therefore:

(8x+1)°+(5x-2)°+90°=180°

8x+1+5x-2+90=180

13x+89=180

13x=91

x=7

This means the measure of angle (5x-2)° is 33°, making the measure of ∠1 equal to 147°.