What is the x-intercept of the line with this equation?
−5x + \(\frac{1}{5}\)y = 15
Answers
To find the x-intercept, set y = 0:
-5x + 1/5(0) = 15
-5x + 0 = 15
-5x = 15
Divide both sides by -5:
x = -3.
Therefore, the x-intercept is (-3, 0).
Please mark my answers as the Brainliest if your find my explanations helpful :)
Related Questions
how do you show a number with a decimal side repeating like 23.3333333333…
i’m slow mb guys i didn’t pay attention in 6th grade so 7th and 8th have been
Answers
Answer:
You can write 23.3 with a dot on the three after the decimal point
please help meeeeeeee <33

Answers
9 times 3 is 27 add 7 to that and you get 34
Answer:
f(5) = 22; f(9) = 34
Step-by-step explanation:
f(5) = 3(5) +7
f(5) = 22
f(9) = 3(9) +7
f(9) = 34
find the average rate of change, in simplest form, of the function over the interval 4\le x \le 74≤x≤7.
Answers
Answer:
\(Average\ Rate = \frac{104}{3}\)
Step-by-step explanation:
Given
See attachment for table
Required
Determine the average rate of change over: 4≤x≤7.
Average rate of change is calculated as:
\(Average\ Rate = \frac{f(b) - f(a)}{b - a}\)
Where:
\(a=4\)
\(b = 7\)
So:
\(Average\ Rate = \frac{f(b) - f(a)}{b - a}\)
\(Average\ Rate = \frac{f(7) - f(4)}{7 - 4}\)
\(Average\ Rate = \frac{f(7) - f(4)}{3}\)
From that table:
f(4) =
f(7) =
So:
\(Average\ Rate = \frac{f(7) - f(4)}{3}\)
\(Average\ Rate = \frac{108-4}{3}\)
\(Average\ Rate = \frac{104}{3}\)

Write an algebraic expression for each verbal description.
The product of three and r, increased by the sum of nine, 2r, and one
Answers
Step-by-step explanation:
3r + (9 + 2r + 1)
this can be simplified :
3r + (9 + 2r + 1) = 3r + 2r + 9 + 1 = 5r + 10
Please Answer! Select the correct answer. Waylan created a scatter plot and drew a line of best fit, as shown. What is the equation of the line of best fit that Waylan drew?
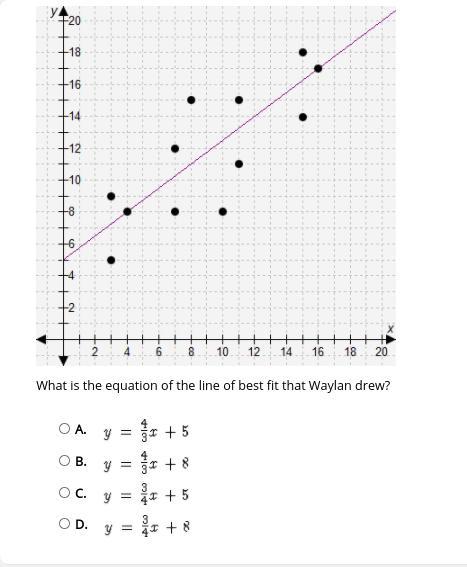
Answers
Answer:
The answer is y=3/4+5 (C)
Step-by-step explanation:
You have to find two exact points on the line then calculate the rise/run (3/4). 3/4 is the slope. Now all that's left is to find the y- intercept (where the line crosses the y-axis) which is 5. Which leaves you with answer choice C.
The equation of the line of best fit that Waylan drew is y=3/4 x+5. Therefore, the correct answer is option C.
From the given graph, the coordinate points are (4, 8) and (16, 17).
Here, slope (m) = (y₂-y₁)/(x₂-x₁)
= (17-8)/(16-4)
= 9/12
= 3/4
Substitute m=3/4 and (x, y)=(4, 8) in y=mx+c, we get
8=3/4 ×4+c
8=3+c
c=5
Substitute m=3/4 and c=5 in y=mx+c, we get
y=3/4 x+5
Therefore, the correct answer is option C.
To learn more about the line of fit visit:
https://brainly.com/question/14279419.
#SPJ4
What is the vertex of the function f(x)= 1/2x^2+3x+3/2?
Answers
Check whether -2 is a zero of the polynomial 3x2 + 4x + 32 .
Answers
Answer:
x = - 2 is not a zero
Step-by-step explanation:
If x = - 2 is a zero of the polynomial then f(- 2) = 0
f(x) = 3x² +4x + 32
f(- 2) = 3(- 2)² + 4(- 2) + 32 = 3(4) - 8 + 32 = 12 - 8 + 32 = 36
Since f(- 2) ≠ 0 , then x = - 2 is not a zero of the polynomial
what is the value of 135,620 written in scientific notation
Answers
Answer:
1.35 x 102 i think
Step-by-step explanation:
if a = b, then does ax = bx and ay = by?
Answers
Answer:
My answer to your question is a "No".
We can know that a and b are the same numbers. If we multiply the same number on each side, the answer will be the same. However, x and y have different values. So, it will show differently.
Let's have an example:
It we say a = 5, x = 6, y = 7.
We know a and b are the same number so, both a and b will be 5. If we multiply a by x, it will be 5 x 6 which is 30. It will show the same answer on multiplying b because as you said a and b are the same. The equation will be 5(a) x 6(x) = 5(b) x 6(x) Let's look at multiplying y. If we multiply a by y, it will be 5 x 7 which is 35. As you said it will show the same answer on multiplying b because a and b are the same. The equation for this will be 5(a) x 7(y) = 5(b) = 7(y). Finally, let's compare the two equations.
5(a) x 6(x) = 5(b) x 6(x) -> 30 = 30
5(a) x 7(y) = 5(b) = 7(y) -> 35 = 35
You can see it more clearly when we compare those to the equations that I made.
Thank you
Yes, if a = b, then ax = bx and ay = by for any value of x.
This is because the equation a = b implies that a and b are equal in value, so any expression involving a can be replaced with an equivalent expression involving b. In other words, any operation performed on a can be performed on b and produce the same result.
For example, if a = b, then multiplying both sides of the equation by x yields ax = bx, and multiplying both sides of the equation by y yields ay = by. This means that any power, root, logarithm, or other mathematical operation performed on a can also be performed on b and produce the same result.
To know more on equal sign
https://brainly.com/question/1640740
#SPJ4
The polynomial P is graphed.
What is the remainder when P(x) is divided by (x+1)?
(image should be attached somewhere)

Answers
Answer:
The remainder will be 3.
Step-by-step explanation:
We can use the Polynomial Remainder Theorem. According to the PRT, if we have a polynomial P(x) divided by a binomial in the form (x - a), then the remainder will be given by P(a).
Our polynomial P(x) is given by the graph, and we are dividing it by the binomial (x + 1).
We can rewrite the binomial as (x - (-1)).
Therefore, a = -1.
Then the remainder will be P(-1).
Looking at the graph, we can see that P(-1) = 3.
Thus, the remainder when P(x) is divided by (x + 1) is 3.
Answer:
The remainder is 3 :)
Step-by-step explanation:
please mark me as brainliest
in one town, 39% of all voters are democrats. if two voters are randomly selected for a survey, find the probability that they are both democrats. round to the nearest thousandth if necessary. group of answer choices
Answers
0.148 is the probability that they are both democrats.
In one town, 39% of all voters are democrats.
If two voters are randomly selected for a survey, the probability that they are both democrats can be calculated as follows.
P(A) = 0.39 is the probability of selecting a democrat in the first draw.
P(B|A) = 0.38
is the probability of selecting a democrat in the second draw if the first draw selects a democrat.
P(B|A') = 0.39 is the probability of selecting a democrat in the second draw if the first draw does not select a democrat.
The probability that both voters are democrats is:
P(A) x P(B|A) = 0.39 x 0.38 = 0.1482
The result is 0.1482, which means the probability that two voters are both democrats in one town is 0.1482 to the nearest thousandth.
For similar question on probability
https://brainly.com/question/25870256
#SPJ11
Are the lines of equations
x = −2 + 2t, y = −6, z = 2 + 6t and
x=−1+t,y=1+t,z=t, t∈ R, perpendicular to each other?
Answers
The given lines of equations are not perpendicular to each other. Therefore, `θ = cos⁻¹(8/(4√10))` which is approximately `28.07°`.Since `θ ≠ 90°`, the given lines of equations are not perpendicular to each other.
Given lines of equations:
x = −2 + 2t, y = −6, z = 2 + 6tx=−1+t,y=1+t,z=t, t∈ R.
Firstly, we need to find the direction vectors of the two given lines.For the first equation,Let `t=1`, then the point on the line is `(-2+2(1), -6, 2+6(1))`=`(0, -6, 8)`.
Let `t=2`, then the point on the line is
\(`(-2+2(2), -6, 2+6(2))`=`(2, -6,\)14)`.T
herefore, direction vector `
\(v1 = (2, -6, 14)-(0, -6, 8)`=`(2, 0, 6)`\)
For the second equation, direction vector \(`v2 = (1, 1, 1)`.\\\)
Let the angle between the direction vectors `v1` and `v2` be `θ`.
Then, we know that `v1 • v2 = |v1||v2| cosθ`, where `•` represents the dot product of the vectors, and `|.|` represents the magnitude of the vector.
Thus, we have:
(2, 0, 6) • (1, 1, 1) = √(2²+0²+6²)√(1²+1²+1²) cosθ
=> 8 = √40√3 cosθ=> cosθ = 8/(4√10)
Therefore,
`θ = cos⁻¹(8/(4√10))`
which is approximately `28.07°`.
Since `θ ≠ 90°`, the given lines of equations are not perpendicular to each other.
To know more about equations visit :-
https://brainly.com/question/29657983
#SPJ11
Write the equation in slope and y intercept. Then write an equation in y=mx+b form.

Answers
Answer:
y=x+1 with slope of 1 and y-intercept of 1.
Step-by-step explanation:
a truck rental company charges $90 plus $20 per hour. identify a function that represents the total charge for renting a truck for a certain number of hours. what is the value of the function for an input of 5, and what does it represent?
Answers
The function that represents the total charge for renting a truck for a certain number of hours is c(h) = $90 + $20(h) and the value of the function for an input of 5, is $190 and this represents the total charge for a rental of 5 hours.
Given,
A truck rental company charges $90 plus $20 per hour.
Here we need to identify a function that represents the total charge for renting a truck for a certain number of hours. And we also need to find the value of the function for an input of 5.
The problem statement tells you the total charge is the sum of $90 and a charge of $20 per hour. That is, for h hours, the total charge is ...
c = 90 + 20h
In functional form, this is
c(h) = 90 +20(h) . . . . . . total charger for a rental of h hours
The function value with an input of 5 is ...
c(5) = 90 +20(5)
c(5) = 90 + 100
c(5) = 290
Since the input is the number of hours of rental, and the function value is the total charge, this represents the total charge for a rental of 5 hours.
To know more about Functions here.
https://brainly.com/question/12431044
#SPJ4
Answer:
f(h)=90+20h
$190
Step-by-step explanation:
Question 2: Products purity and feed flow rate in complete mixing model A binary mixture of gas A which has mole fraction of x = 0.5 and gas B is being fed at a flow rate of q into a membrane module in order to effect seperation of the two gases. The membrane process is to be operated with a feed-side pressure of Ph = 80 cm Hg and permeate-side pressure of p₁ = 20 cm Hg. Gas A has a permeability of P = 400 x 10-10 cm³ (STP) cm/(s cm² cm Hg), and the separation factor between gas A and B is . a 10. If the thickness of the membrane is t = 2 x 10³ cm and the fraction of the feed that = is permeated is 0 = = 0.25, (a) Calculate the permeate composition, yp (b) Calculate the reject composition, xo (c) Calculate the feed flow rate, q, in cm³ (STP)/s if the available area of the membrane is 3 x 108 cm²
Answers
(a) To calculate the permeate composition, yp, we can use the formula:
yp = (P * x * (Ph - p₁) * t * 0) / (q * (Ph - p₁) + P * x * t * 0)
Given:
x = 0.5 (mole fraction of gas A)
Ph = 80 cm Hg (feed-side pressure)
p₁ = 20 cm Hg (permeate-side pressure)
P = 400 x 10^(-10) cm³(STP) cm/(s cm² cm Hg) (permeability)
t = 2 x 10³ cm (membrane thickness)
0 = 0.25 (fraction of feed permeated)
q = feed flow rate (to be determined)
Substituting the given values into the formula:
yp = (400 x 10^(-10) * 0.5 * (80 - 20) * 2 x 10³ * 0) / (q * (80 - 20) + 400 x 10^(-10) * 0.5 * 2 x 10³ * 0)
Since 0 * anything equals zero, we can simplify the formula to:
yp = 0
Therefore, the permeate composition, yp, is zero.
(b) To calculate the reject composition, xo, we can use the formula:
xo = 1 - yp
Since we found in part (a) that yp = 0, we can conclude that:
xo = 1 - 0
xo = 1
Therefore, the reject composition, xo, is 1.
(c) To calculate the feed flow rate, q, we can rearrange the formula from part (a) and solve for q:
q = (P * x * (Ph - p₁) * t * 0) / (yp * (Ph - p₁) + P * x * t * 0)
Substituting the given values into the formula:
q = (400 x 10^(-10) * 0.5 * (80 - 20) * 2 x 10³ * 0) / (0 * (80 - 20) + 400 x 10^(-10) * 0.5 * 2 x 10³ * 0)
Again, since 0 * anything equals zero, we can simplify the formula to:
q = 0
Therefore, the feed flow rate, q, is zero.
In conclusion:
(a) The permeate composition, yp, is zero.
(b) The reject composition, xo, is 1.
(c) The feed flow rate, q, is zero.
Please note that the calculations resulted in a feed flow rate of zero, which may suggest an inconsistency in the given values or equations. Double-checking the values and equations is recommended to ensure accuracy.
To know more about permeate composition, visit :
https://brainly.com/question/15186859
#SPJ11
how prove the following
cos²(120°-A) + cos²A + cos²(120°+A)= 3/2
Answers
Answer:
Sorry if it is not clear enough

Factor the expression.
2x + 8

Answers
Answer:
2x+8
2 is common
2(x+4)=0
Step-by-step explanation:
The terminal side of θ in standard position contains the point (3, 0). Find the exact values of the six trigonometric functions of θ
Answers
\(\textit{we know that }\theta \textit{ contains the point }(\stackrel{x}{3}~~,~~\stackrel{y}{0})\textit{, let's find the hypotenuse} \\\\\\ \textit{using the pythagorean theorem} \\\\ r^2=a^2+b^2\implies r=\sqrt{a^2+b^2}\implies r=\sqrt{3^2+0^2} \qquad \begin{cases} r=hypotenuse\\ a=adjacent\\ b=opposite\\ \end{cases} \\\\\\ r=\sqrt{3^2}\implies r=3\)
\(\rule{34em}{0.25pt}\\\\ sin(\theta)=\cfrac{opposite}{hypotenuse} =\cfrac{y}{r}\implies \cfrac{0}{3}\implies 0 \\\\\\ cos(\theta)=\cfrac{adjacent}{hypotenuse} =\cfrac{x}{r}\implies \cfrac{3}{3}\implies 1 \\\\\\ tan(\theta)=\cfrac{opposite}{adjacent} =\cfrac{y}{x}\implies \cfrac{0}{3}\implies 0\)
\(cot(\theta)=\cfrac{adjacent}{opposite} =\cfrac{x}{y}\implies \cfrac{3}{0}\implies und efined \\\\\\ csc(\theta)=\cfrac{hypotenuse}{opposite} =\cfrac{r}{y}\implies \cfrac{3}{0}\implies und efined \\\\\\ sec(\theta)=\cfrac{hypotenuse}{adjacent} =\cfrac{r}{x}\implies \cfrac{3}{3}\implies 1\)
ind the median and mean of the data set below: 29,8,11,29,29,6,49
Answers
Answer:
Mean= 23
Median= 29
Step-by-step explanation:
If the time is 12:00 A.M. in Greenwich, England, what time is it in Houston, (CST)? 6:00 P.M. 7:00 A.M. 5:00 A.M. 12:00 A.M. 5:00 P.M. If Earth rotated at double its current rotational speed, which of the following would be true? Days would be exactly 24 hours. Years would be shorter than 3651/4 days. Months would last longer than 31 days. Days would be exactly 12 hours.
Answers
the correct answer is "Days would be exactly 12 hours."
If the time is 12:00 A.M. in Greenwich, England, then it is 6:00 P.M. in Houston (CST). Therefore, the correct answer is 6:00 P.M.
Now, let's consider the second question.
If Earth rotated at double its current rotational speed, which of the following would be true?
A day is measured by the time taken by the Earth to complete one rotation around its own axis. The current rotational speed of the Earth is 1 revolution per 24 hours.
Therefore, if the Earth rotates at double its current rotational speed, then one revolution would be completed in 12 hours only. Hence, the days would be exactly 12 hours long.
Therefore, the correct answer is "Days would be exactly 12 hours."
To know more about current rotational
https://brainly.com/question/10718356
#SPJ11
Each histogram represents a set of data with a median of 29.5. Which set of data most likely has a mean that is closest to 29.5?
A graph shows the horizontal axis numbered 9 to 48. The vertical axis is numbered 1 to 5. The graph shows an upward trend from 1 to 33 then a downward trend from 33 to 45.
A graph shows the horizontal axis numbered 15 to 48. The vertical axis is numbered 1 to 5. The graph shows an upward trend from 1 to 30 then a downward trend from 30 to 45.
A graph shows the horizontal axis numbered 12 to 56. The vertical axis is numbered 2 to 8. The graph shows an upward trend from 1 to 32 then a downward trend from 32 to 56.
A graph shows the horizontal axis numbered 15 to 54. The vertical axis is numbered 1 to 5. The graph shows an upward trend from 1 to 24, a downward trend from 24 to 27, an upward trend from 27 to 30, a downward trend from 30 to 39, an upward trend from 39 to 45, a downward trend from 45 to 48, then an upward trend from 48 to 51.
Answers
To determine which set of data most likely has a mean closest to 29.5, we need to analyze the shape and position of the histograms in relation to the value 29.5.
Looking at the histograms described:
The first histogram ranges from 9 to 48, and the upward trend starts from 1 and ends at 33, followed by a downward trend. This histogram suggests that there may be values lower than 29.5, which would bring the mean below 29.5.
The second histogram ranges from 15 to 48, with an upward trend from 1 to 30 and then a downward trend. Similar to the first histogram, it suggests the possibility of values lower than 29.5, indicating a mean below 29.5.
The third histogram ranges from 12 to 56, and the upward trend starts from 1 and ends at 32, followed by a downward trend. This histogram covers a wider range but still suggests the possibility of values below 29.5, indicating a mean below 29.5.
The fourth histogram ranges from 15 to 54 and exhibits multiple trends. While it has fluctuations, it covers a wider range and includes both upward and downward trends. This histogram suggests the possibility of values above and below 29.5, potentially resulting in a mean closer to 29.5.
Based on the descriptions, the fourth histogram, with its more varied trends and wider range, is most likely to have a mean closest to 29.5.
For such more question on histograms
https://brainly.com/question/2962546
#SPJ8
Neil and his mom are volunteering at an emergency relief center. To help prepare in case of a flood, they pack emergency kits with food and water. Neil puts 3 water bottles into each kit they pack. In all, he packs 150 water bottles. Which equation can you use to find the number of emergency kits k Neil packs?
Answers
The number of emergency kits that Neil packed in total for the 150 bottles is gotten as; k = 50 kits.
How to create equations?We are told that Neil puts 3 water bottles into each emergency kit packed.
Now, let the number of emergency kits be k.
Thus;
3k = 150
Division property of equality, we have;
3k/3 = 150/3
k = 50
Thus, the number of emergency kits that Neil packed is gotten as k = 50 kits.
Read more about Equalities at; https://brainly.com/question/25275758
#SPJ1
The pentagons ABCDE and JKL MN are similar.
Find the length x of NJ.

Answers
Answer:
5.4
Step-by-step explanation:
The scale of the 2 shapes is 1:1.8. so if the smaller shape's side is 3, insert 3 into the ratio. Now, multiply 3*1.8, and you get your answer of 5.4
Consider the following two lines: one with parametric equations x(s)=4−2s,y(s)=−2+s,z(s)=1+3s, and the other being the line through (−4,2,17) in the direction v=⟨−2,1,5⟩.a) Find a direction vector for the first line, which is given in parametric form.b) Find parametric equations for the second line, written in terms of the parameter t.c) Show that the two lines intersect at a single point by finding the values of sand tthat result in the same point.d) Find the angle formed where the two lines intersect, noting that this angle will be given by the angle between their respective direction vectors.e) Find an equation for the plane that contains both of the lines described in this problem
Answers
A-The first line has a direction vector of ⟨-2, 1, 3⟩, b-the second line has parametric equations x(t) = -4 - 2t, y(t) = 2 + t, z(t) = 17 + 5t, c-the two lines intersect at the point (1, 3, 10), d-the angle formed is 15.2 degrees, and e- the equation containing both lines is -2x + 7y - 5z = -59.
What is direction vector ?
A direction vector, also known as a directional vector or simply a direction, represents the direction of a line, vector, or a linear path in three-dimensional space. It is a vector that points in the same direction as the line or path it represents.
a) The direction vector for the first line is given by ⟨-2, 1, 3⟩.
b) The parametric equations for the second line, written in terms of the parameter t, are x(t) = -4 - 2t, y(t) = 2 + t, z(t) = 17 + 5t.
c) To find the intersection point, we set the x, y, and z coordinates of both lines equal to each other and solve for s and t:
4 - 2s = -4 - 2t
-2 + s = 2 + t
1 + 3s = 17 + 5t
Solving this system of equations yields s = 3 and t = 1. Therefore, the two lines intersect at the point (1, 3, 10).
d) The angle formed at the intersection point is given by the angle between their respective direction vectors. Using the dot product, the angle θ can be found as cos(θ) = (⟨-2, 1, 3⟩ · ⟨-2, 1, 5⟩) / (|⟨-2, 1, 3⟩| |⟨-2, 1, 5⟩|), which simplifies to cos(θ) = 0.96. Taking the inverse cosine, we find θ ≈ 15.2 degrees.
e) To find the equation of the plane containing both lines, we can use the point-normal form of a plane equation. We choose one of the intersection points (1, 3, 10) and use the cross product of the direction vectors of the two lines as the normal vector. The equation of the plane is given by -2x + 7y - 5z = -59.
learn more about Direction vector here:
https://brainly.com/question/28654681
#SPJ4
Please help will give brainliest if correct

Answers
Answer:
the third option cannot be a function
Step-by-step explanation:
whenever a set or ordered pairs contains an x-value that appears more than once then it cannot form a function
Answer:
The third one
Step-by-step explanation:
which is a characteristic of any function created by the product of two linear factors?
Answers
The characteristic of any function created by the product of two linear factors has two x-intercepts.
According to the question;
We are required to determine which is a characteristic of any function created by the product of two linear factors.
The product of two linear factors results in a quadratic function.
Since a quadratic function is a polynomial of degree two, 2.
In essence, the resulting function created by the product of two linear factors has two x-intercepts.
Read more about of function here
brainly.com/question/11869216
#SPJ4
Given: 5n - 42 = 12n; Prove: n = -6
Statements and reasons
Answers
Answer:
yes n is -6
Step-by-step explanation:
b/c 5(-6)-42=12(-6)
-30-42=-72
-72=-72
This box is packed with cubes that measure one cubic foot.
Enter the volume of the box in cubic feet.

Answers
Answer:
81
Step-by-step explanation:
find area of trapezoids

Answers
Answer
660in²
Step-by-step explanation:
B1=30
B2=36
h=20
1/2*20(30+36)
1/2*20(66)
10(66)
660in²
State if the three numbers can be the measures of the sides of a triangle.
7,5,4
Yes
No
Answers
hope this helps :)
Yes, the three numbers 7, 5, and 4 can be the measures of the sides of a triangle.
What is a triangle?A triangle is a 2-D figure with three sides and three angles.
The sum of the angles is 180 degrees.
We can have an obtuse triangle, an acute triangle, or a right triangle.
We have,
The three numbers 7, 5, and 4 can be the measures of the sides of a triangle.
This is because the sum of the two shorter sides of a triangle must be greater than the longest side.
In this case,
The longest side is 7, and the sum of the two shorter sides is 5 + 4 = 9, which is greater than 7.
Therefore,
The three numbers satisfy the triangle inequality and can be the measures of the sides of a triangle.
Learn more about triangles here:
https://brainly.com/question/25950519
#SPJ3