What kind of sequence is 6 12 24?
Answers
As there is a common ratio between each term so this is a geometric sequence.
How geometric sequence is applied in real life?The idea of geometric progression can be used to determine how much money you will have in your account after a particular number of years if you deposit money in a bank that offers a fixed annual rate of interest.
What is the important property of geometric sequence?In a geometric progression or series, each term is equal to the one before it times the common factor, which is a fixed non-zero multiplier. The number of terms in a geometric series might be either finite or infinite.
Since in 6 12 and 24 the common ratio r=2. That is if we multiply the previous term by 2 we will get the next term so the given sequence is geometric sequence.
To learn more about geometric sequence visit the link:
https://brainly.com/question/24643676
#SPJ4
Related Questions
During a recent rainstorm, 8 centimeters of rain fell in Dubaku's hometown, and 2.36 centimeters of rain fell in Elliot's hometown. During the same storm, 15.19 centimeters of snow fell in Alperen's hometown.
Answers
Answer:
5.64 cm
Step-by-step explanation:
Required:How much more rain fell in Dubaku's town than in Elliot's town?
8 centimeters of rain fell in Dubaku's hometown
2.36 centimeters of rain fell in Elliot's hometown
We are asked to find how much more rain fell in Dubaku's town than in Elliot's town so it is calculated as the difference between the rain in both of there towns and is given by:
Difference in rain= 8-2.36
Difference in rain= 5.64 cm.
Therefore, 5.64 cm of more rain fell in Dubaku's town than in Elliot's town.
Answer:
5.64
Step-by-step explanation:
I NEED HELP PLEASE !

Answers
Answer:
x = 4, -2 1/2
Step-by-step explanation:
factors:
(2x + 5) (x - 4)
set each factor equal to zero:
2x + 5 = 0
2x = -5
x = -5/2
x - 4 = 0
x = 4
Vertical angles are __
a always congruent
b supplementary
c sometimes congruent
Answers
Complete each equation below so that it shows equivalent fractions.

Answers
4/5 would be 8/10 because you’re multiplying by 2
1/6 can be many, but the answer would be 2/12 because you multiplied by two
Answer:
\(\frac{3}{12}, \frac{8}{10},\frac{2}{12}\)
Step-by-step explanation:
→ Find the multiplier in the first one
12 ÷ 4 = 3
→ Multiply onto top
\(\frac{3}{12}\)
→ Find multiplier for second one
10 ÷ 5 = 2
→ Multiply
2 × 4 = 8
a gamblret places a bet on anhorse race. to win she must pick the top thre finishers in order. six horses of equal ability and entereted in the race. assuimg the horses finish in hte randsom ordr, what is he probability the the gambler will win the bet
Answers
The probability that the gambler will win the bet is very low at only 0.83%.
The probability that the gambler will win the bet, we need to first determine the total number of possible outcomes or permutations for the top three finishers out of the six horses. This can be calculated using the formula for permutations:
P(6, 3) = 6! / (6-3)! = 6 x 5 x 4 = 120
This means that there are 120 possible ways that the top three finishers can be chosen out of the six horses. However, the gambler needs to pick the top three finishers in the correct order to win the bet. Therefore, there is only one correct outcome that will result in the gambler winning the bet.
The probability of the correct outcome happening is therefore:
1/120 = 0.0083 or approximately 0.83%
So, the probability that the gambler will win the bet is very low at only 0.83%.
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ11
A cement company is making 8 solid cement spheres Each sphere has a diameter of 2.5
Approximately how much concrete is needed to make all 8 spheres
8.2ft cubed
39.3ft cubed
62.8ft cubed
65.4ft cubed
Answers
Help me with is ixl please

Answers
Answer:
∠EGC
Step-by-step explanation:
Just like for the other problem, you're looking for an angle that's opposite the one you're given and is the same size as the one you're given (meaning they're congruent). If you use you hands to cover the parts of the line AD, you can see how ∠FGB and ∠EGC are opposite of each other and are congruent..
If -9x – 8y = 5 is a true equation, what would be the value of 54x + 48y?
Answers
Answer:
102
Step-by-step explanation:
Use the appropriate formula (and show work) to find a1 given that
S16 = -160 and a16 = -25

Answers
Answer:
a1 = 5
Step-by-step explanation:
1. Substitute S16 = -160 into the second formula.
2. solve as mush as possible
3. a16 is given. substitute.
4. solve

Answer:
\(a_1=5\)
Step-by-step explanation:
Given:
\(S_{16}=-160\)\(a_{16}=-25\)\(\textsf{Use}\quad S_n=\dfrac{n}{2}(a_1+a_n):\)
\(\begin{aligned}S_{16} &=-160\\\implies S_{16}=\dfrac{16}{2}(a_1+a_{16}) &=-160\\\implies \dfrac{16}{2}(a_1+(-25)) & =-160\\8(a_1-25) &=-160\\a_1-25 &=-20\\a_1&=-20+25\\a_1 &=5\end{aligned}\)
For a sample with M = 50 and s = 12, what is the X value corresponding to z =-0.25? a. 46 b.47 c. 53 d. 54
Answers
Step-by-step explanation:
To find the X value corresponding to z = -0.25, we can use the formula:
z = (X - M) / s
where z is the standardized score, X is the raw score we want to find, M is the population mean, and s is the population standard deviation.
Rearranging this formula, we get:
X = z * s + M
Substituting the given values, we get:
X = -0.25 * 12 + 50
X = 47
Therefore, the X value corresponding to z = -0.25 is 47.
Hence, the answer is option B.
The X value corresponding to z = -0.25 is 47 (option b).
To answer this question, we need to use the formula for calculating z-scores, which is:
z = (X - M) / (s)
where z is the z-score, X is the raw score, M is the population mean, and s is the population standard deviation.
In this case, we are given the population mean (M = 50) and the z-score (z = -0.25). We also know that the population standard deviation (s) is 12.
We can rearrange the formula to solve for X:
X = z * s + M
Plugging in the values we have:
X = -0.25 * 12 + 50
X = 47
Therefore, the X value corresponding to z = -0.25 is 47. The answer is (b).
It's important to note that z-scores are a way of standardizing data so that we can compare different sets of data that may have different scales or units. In this case, we are using the z-score to find the corresponding raw score.
To know more about standard deviation visit:
https://brainly.com/question/23907081
#SPJ11
Given that a*b = 2a - 3b, then 2*(-3) =
Answers
Answer:
2*(-3)= -6
Step-by-step explanation:
I do not see how "a*b=2a-3b" would change the fact 2 times negative 3 is -6
For the polynomial function f(x)= -2x² - 4x + 16, find all local and global extrema.
A.) No local extrema exist.
B.) The local and global extrema are: (0, -4), (0, 2) and (-1, 18).
C.) The only extrema point is (-1, 18).
D.) No global extrema exist.
Answers
Answer:
C.) The only extreme point is (-1, 18).
Step-by-step explanation:
You want the local and global extrema of f(x) = -2x² - 4x + 16.
Extreme pointsUnless the domain is restricted to a particular interval, the extrema of a polynomial function will be its turning points. The number of possible turning points is 1 less than the degree of the equation.
An even-degree polynomial equation will always have at least one global extreme on the domain of all real numbers.
The given 2nd-degree equation will have exactly one extreme point: its vertex at (-1, 18).
__
Additional comment
The vertex of ax² +bx +c is located at x=-b/(2a). Here, that is x=-(-4)/(2(-2)) = -1. The value of f(-1) is -2 -(-4) +16 = 18, so the extreme point is (-1, 18). A graphing calculator finds it quickly.

the empirical rule assumes that the distribution of data follows a normal curve. group startstrue or falsetrue, selectedfalse, unselected
Answers
True. The Empirical Rule, also known as the 68-95-99.7 Rule, assumes that the distribution of data follows a normal curve, which is symmetrical and bell-shaped.
The Empirical Rule is a useful tool for understanding the spread of data in a normal distribution. A normal distribution is a symmetrical, bell-shaped distribution that is characterized by its mean, median, and mode. The mean is the average of all the data, the median is the middle value, and the mode is the most frequently occurring value.
According to the Empirical Rule, approximately 68% of the data falls within one standard deviation of the mean. This means that if you have a normal distribution, and you know the mean and standard deviation, you can estimate that 68% of the data will be within one standard deviation above and below the mean. For example, if the mean of a distribution is 100 and the standard deviation is 10, then 68% of the data will be between 90 and 110.
It's important to note that the Empirical Rule is only applicable to data that follows a normal distribution. In other words, if your data is not normally distributed, the Empirical Rule may not provide an accurate representation of the spread of your data.
Learn more about The Empirical Rule here: https://brainly.com/question/10093236
#SPJ4
A. What must the side length of each square represent for the rectangle to correctly represent (0. 3). (0. 5)?
Answers
The side length of each square represent for the rectangle to correctly represent (0, 3) · (0, 5) is 1 units.
A rectangle represented by (0, 3) · (0, 5) has length 3 units and breadth 5 units. To represent that rectangles with minimum number of squares of same size, we calculate the highest common factor.
Highest Common Factor is a mathematical concept used to find the greatest number that divides two or more numbers without leaving a remainder. It is also known as the greatest common divisor (GCD) or the greatest common factor (GCF).
Highest common factor of 3 and 5 is 1 as both are prime numbers. So squares with side length 1 unit best represent the rectangle to correctly represent (0, 3) · (0, 5).
To know more on HCF
https://brainly.com/question/29114178
#SPJ4
4. Jose has $1500 in his bank account. He spends $150 each week. Which equation can be used to
Find the amount of money left in Jose's bank account after x, weeks of spending?
Answers
Answer:
1500/150 = x
Step-by-step explanation:
If he has $1500 in the bank and spends $150 a week, you have to solve how many weeks that is, which is x. So you would have divison. You're answer would be 1500/150 = x, I believe.
Therefore, the equation x weeks = $150x is the answer.
Hoped this helped.
\(BrainiacUser1357\)
A jet airplane reaches 846. Km/h on a certain flight. What distance does it cover in 13.0 min? Set the math up. But don't do any of it. Just leave your answer as a math expression. Also, be sure your answer includes all the correct unit symbols.
Answers
Answer:
Distance = (846 km/h) × (13.0 min × (1 h / 60 min))-----------------
To find the distance you can use the formula:
Distance = Speed × TimeGiven:
Speed = 846 km/hTime = 13.0 minFirst, we need to convert the time from minutes to hours:
13.0 min × (1 h / 60 min)Now, set up the equation using the given values and units:
Distance = (846 km/h) × (13.0 min × (1 h / 60 min))Find the critical numbers of the function and describe the behavior of f at these numbers. (List your answers in increasing order.)
f(x) = x6(x - 2)5
At ____ the function has ---localmax/localmin/ or neither--
At _____the function has ---localmax/localmin/ or neither--
At ______ the function has ---localmax/localmin/ or neither--
Answers
The critical numbers are 0 and 2, and the behavior of f at these numbers is At x = 0, the function has a local minimum.
At x = 2, the function has neither a local maximum nor a local minimum.
To find the critical numbers, we need to take the derivative of the function and set it equal to zero:
f'(x) = 6x^5(x-2)^5 + x^6(5)(x-2)^4(1) = 0
Simplifying this equation, we get:
x(x-2)^4(6x^4 + 5x^2(x-2)) = 0
The critical numbers are where the derivative equals zero, which are x = 0 and x = 2.
To describe the behavior of f at these critical numbers, we need to examine the sign of the derivative around each critical number. If the derivative is positive to the left and negative to the right of a critical number, then the function has a local maximum at that point. If the derivative is negative to the left and positive to the right, then the function has a local minimum at that point. If the derivative does not change sign at a critical number, then the function has neither a local maximum nor a local minimum at that point.
Let's examine each critical number:
At x = 0, we can see that the factor x is negative to the left of 0 and positive to the right of 0. The factor (x-2)^4 is positive everywhere. The factor 6x^4 + 5x^2(x-2) is positive at x = 0 (since the x^2 term is zero), which means the derivative is positive to the left and right of x = 0. Therefore, f has a local minimum at x = 0.
At x = 2, we can see that the factor (x-2)^4 is zero at x = 2, which means the derivative is zero at x = 2. To determine whether f has a local maximum or minimum at x = 2, we need to examine the sign of the derivative on either side of 2. If we plug in a value slightly less than 2 (e.g. x = 1.9), we get a positive derivative. If we plug in a value slightly greater than 2 (e.g. x = 2.1), we also get a positive derivative. Therefore, f has neither a local maximum nor a local minimum at x = 2.
Learn more about critical numbers here
https://brainly.com/question/5984409
#SPJ11
test the series for convergence or divergence. [infinity] 1 n 9n n = 1
Answers
The given series is convergence and converges to 1/8. The given series is: 1/9 + 1/81 + 1/729 + ….+ 1/(9^n)+……..Using the Geometric progression formula, we have: a = 1/9, r = 1/9.∴ sum of the series = a / (1 - r) = (1/9) / [1 - (1/9)] = (1/9) / (8/9) = 1/8. Hence, the series converges to 1/8.
In mathematics, a series is an infinite sequence of numbers added together. When dealing with infinite sequences, convergences, and divergence become essential concepts. In this problem, we will test the series for convergence or divergence.
To do this, we will use the Geometric progression formula :
The given series is:
1/9 + 1/81 + 1/729 + ….+ 1/(9^n)+……..
Using the Geometric progression formula, we have
a = 1/9
r = 1/9.
∴ the sum of the series
= a / (1 - r)
= (1/9) / [1 - (1/9)]
= (1/9) / (8/9)
= 1/8
Therefore, the series converges to 1/8. In conclusion, the given series converges to 1/8.
To know more about the Geometric progression, visit:
brainly.com/question/30447051
#SPJ11
Aiden is replacing the tile in a rectangular kitchen. The length of the kitchen is nine feet shorter than three times its width, w. The perimeter of the kitchen is six times the width. If tiles cost $1.69 a square foot, what is the total cost to tile the kitchen?
Answers
Answer:
Total Cost to tile = $273.28
Let x be the Width; then
3x - 9 = Length; hence
2(W + L) = Perimeter; therefore
6x = 2(x + 3x - 9)
6x = 2(4x - 9) = 8x - 18
6x - 8x = -18
-2x = -18
x = 9
Width = x = 9 ft
Length = 3x - 9 = 3 times 9, minus 9 = 27 - 9 = 18 ft
Area = W times L = 9 ft. times 18 ft. = 162 sq. ft.
Cost of Tile = $1.69 per sq. ft.
Total Cost = $1.69 times 162 = $273.28
To check:
Perimeter = 6(W) = 6x = 6 times 9 ft. = 54 ft.; also
Perimeter = 2(W + L) = 2(9 + 18) = 2(27) = 54 ft
2.5 years = (how many weeks)
Answers
Answer:
130.357 weeks
Step-by-step explanation:
PLEASE HELP ME 40 POINTS!!!
The graph of the linear function f has a positive slope. Match each system of linear equations with the correct number of solutions.
A. No Solution B. Infinitely many solutions C. One Solution
1. y = f(x) ------------> _________
1/4y = 1/4f(x)
2. y = f(x) -----------> _________
y = f (4x)
3. y = f(x) ------------> _________
y = f (x) -4
4. y = f(x) ------------> _________
y = 4f (x)
Answers
Answer:
1. B: the equation is always true
2. C (I'm pretty sure)
3. A: the equation is never true (y does not equal itself minus 4)
4. A: the equation is never true
I need help on this problem. (-5)^5/(-5)^-6

Answers
Answer: (-5)¹¹ of (-5)^11
Step-by-step explanation:
Since the base of the two exponents are teh same at -5, we can subtract the exponents.
5-(-6)=11
Now, we know that the new exponent will be 11, with the base staying the same.
What is the product of 3 2/3 and 9 6/7 how could I show my work to this??
Answers
Answer:
20 3/7
Step-by-step explanation:
change each mixed number into an improper fraction
3 2/3 = (3 · 3 + 2) / 3 or 11/3
9 6/7 = (7 · 9 + 6) / 7 or 69/7
\(\frac{11}{3}\) · \(\frac{69}{7}\) = \(\frac{11}{1}\) · \(\frac{13}{7}\) (you can divide 3 and 69 by 3)
\(\frac{11}{1}\) · \(\frac{13}{7}\) = 11 x 13 = 143/7
1 x 7
change back into mixed number form by dividing 143 by 7
you get 20 remainder 3, so 20 3/7
Answer:
27 4/7
Step-by-step explanation:
3 2/3 x 9 6/7 = 27 12/21 = 27 4/7
midpoint (10,5), endpoint (4, - 1)
Answers
Answer:
M=(7,2)
Step-by-step explanation:
M=(10+4/2,5+-1/2)
M=(7,2)
A recent survey reveals that one of the collie owners interviewed, 54% of them regularly groom their dogs, If there are 50,000 registered poodle owners in the county, how many owners are expected to regular groom their dos
Answers
Answer:
54% × 50000= 27000, the answer is 27000
Which number belongs to the set of integers but does NOT belong to the set of natural numbers? * 10 points Captionless Image A B C D
Answers
awnser: -5
hope this helps
Given:
R is the midpoint of QS
Prove: PRQ = TRS

Answers
Answer:
Step-by-step explanation: Hello!
I don't remember all of the postulates to these, but I hope this will help you! Vertical angles are congruent, so both angles SRT and PRQ are congruent. This also means that line segments PQ and ST are congruent. You also know that angles Q and S are congruent which is given. By the ASA Theorem, when two angles and a side are congruent, then the triangles are congruent.
The polynomial 2x³ + qx² + rx + 2 has a
factor (x - 1) and leaves a remainder of
12 when divided by (x - 2). Find the
constants q and r and hence the other
factors of the polynomial.
Answers
Answer:
q = 1 , r = -5
other factors of polynomial:
(2x - 1) & (x + 2)
Step-by-step explanation:
In the first part, substitute x = 1 make it equal to 0.
Substitute x = 2 and make it equal to 12.
Rearrange both, until you have two linear equations.
Solve the set of simultaneous equation to find q and r .
Second part place the value of q and r to have the full polynomial.
Carry out long division, dividing the polynomial by the factor that's stated in the question (x - 1) and you will have a quadratic equation.
Factorise the quadratic equation to have the two other factors of the polynomial.
Hope this helps:))
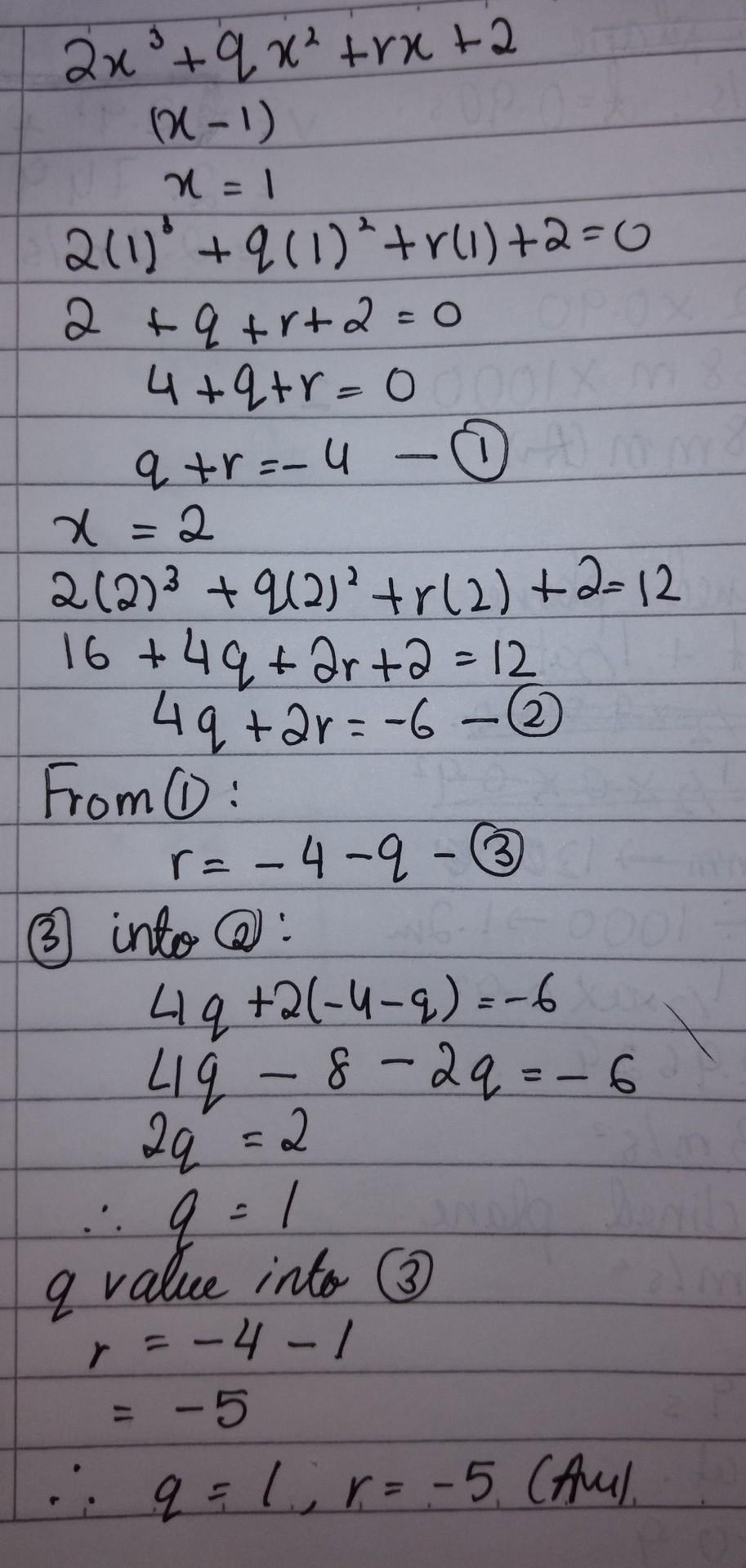

meagan worked 24 hours and earned $84 what is her rate pay
Answers
Answer: 3.50 Per hour
Step-by-step explanation:
Divide 84 by 24
$3.50 per hour
Find the 12th term of the geometric sequence 1, -4, 16,
Answers
Answer:
a₁₂ = - 4194304
Step-by-step explanation:
the nth term of a geometric sequence is
\(a_{n}\) = a₁ \((r)^{n-1}\)
where a₁ is the first term and r the common ratio
here a₁ = 1 and r = \(\frac{-4}{1}\) = - 4 , then
a₁₂ = 1 × \((-4)^{11}\) = - 4194304