Which expression is equivalent to 4^7 x 4^-5
Answers
4^2 (because the powers will be added)
Related Questions
Can someone plz help me with these United rates and explain step by step ?

Answers
Write two numbers that multiply to the value on top and add to the value on bottom.
100 on top
-20 on bottom

Answers
Answer:
-10 and -10
Step-by-step explanation:
Can you use the same number twice?
Solve the equation for x
√√x+5-3-4
Ox=2
Ox=12
Ox=44
Ox=53
help!!

Answers
Hence, OPTION (C) is your answer: X = 44
Step-by-step explanation:
SOLVE FOR X:√x + 5 - 3 = 4
Isolate√x + 5 = 3 + 4
√x + 5 = 7
Squared Both Sides:(√x + 5)2 = (7)2
x + 5 = 49
Rearranged:x - 44 = 0
+44 = 44
X = 44
Add 44 on both sides:Now we conclude!Hence, X = 44
Draw The conclusion: CheckEquation: √x + 5 = 7
Now we Plug in 44 for x√(44) + 5 = 7
Now Simplify√49 = 7
Conclusion checks Solution is:Hence, x = 44
I hope this helps!
Calculator
not allowed
Second chance! Review your workings and see if you can correct your mistake.
Bookwork code: P94
The number line below shows information about a variable, m.
Select all of the following values that m could take:
-2, 4, -3.5, 0, -5, -7
-5 -4 -3 -2 -1 0 1 2 3 4 5
Answers
All of the values that m could take include the following: -3.5, -5, and -7
What is a number line?In Mathematics and Geometry, a number line simply refers to a type of graph that is composed of a graduated straight line, which typically comprises both negative and positive numerical values (numbers) that are located at equal intervals along its length.
This ultimately implies that, all number lines would primarily increase in numerical value towards the right from zero (0) and decrease in numerical value towards the left from zero (0).
From the number line shown in the image attached below, we can logically deduce the inequality:
x ≤ -3
Therefore, the numerical values for x could be equal to -3.5, -5, and -7
Read more on number line here: brainly.com/question/22515080
#SPJ1
Missing information:
The question is incomplete and the complete question is shown in the attached picture.

Write an equation in point-slope form for the line that satisfies the given set of conditions.
slope of 4/5, passes through (10,-3)
Answers
Answer:
y + 3 = \(\frac{4}{5}\) (x - 10)
Step-by-step explanation:
The equation of a line in point- slope form is
y - b = m(x - a)
where m is the slope and (a, b ) a point on the line
Here m = \(\frac{4}{5}\) and (a, b ) = (10, - 3 ) , then
y - (- 3) = \(\frac{4}{5}\) (x - 10) , that is
y + 3 = \(\frac{4}{5}\) (x - 10)
A Friedman number is a whole number which can be written in the form of an expression with same value by using its own digits. The expression can contain the symbols +, −, ×, (, ), and exponentiation, even repeatedly and at any place (e.g. the minus sign could appear on the far left), as well as concatenation of digits (e.g. 1,2 → 12).
For example, 2502 = 2 + 502 and 1024 = (4−2)10 are Friedman numbers.
Which of the following numbers is a Friedman number?
a) 27
b) 36
c) 16
d) 34
e) 125
f) 83
g) 112
Answers
Have an amazing day!! remember your worth it. and I see you trying everyday.
Use the extension of the Cauchy Integral Formula to evaluate the contour integral \[ \int_{C} \frac{5 z^{2}+3 \sqrt{2} z+1}{(z-i)^{3}} d z \] where \( C \) is the circle \( |z|=3 \), positively orShow that
∣
∣
∫
C
R
z
4
+5z
2
+4
z
2
−2
dz
∣
∣
≤
(R
2
−1)(R
2
−4)
2πR
3
+4πR
Hint : Use the triangle inequality. [6]
Answers
The contour integral \(\(\int_{C} \frac{5z^{2}+3\sqrt{2}z+1}{(z-i)^{3}} dz\)\) along the circle |z|=3 evaluates to \(10\pi i\)\), and the inequality \(\(\left|\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz}\right| \leq (R^2-1)(R^2-4) \cdot \frac{2\pi R}{3} + 4\pi R\)\) holds for the given contour \(\(C_R\)\).
To evaluate the contour integral \(\(\int_{C} \frac{5z^{2}+3\sqrt{2}z+1}{(z-i)^{3}} dz\)\) along the circle |z|=3, we can use the extension of the Cauchy Integral Formula for the derivative of a function. The formula states that if f(z) is analytic inside and on a simple closed contour C, except at a point z=a inside C, then the nth derivative of f(z) at a is given by
\(\[f^{(n)}(a) = \frac{n!}{2\pi i}\int_{C} \frac{f(z)}{(z-a)^{n+1}} dz\]\)
In our case, we have \(\(f(z) = 5z^{2}+3\sqrt{2}z+1\)\) and n = 2 (since we want to evaluate the integral of the third derivative). The point a is i, and the contour C is the circle |z|=3.
Using the formula, we can rewrite the integral as:
\(\[\int_{C} \frac{5z^{2}+3\sqrt{2}z+1}{(z-i)^{3}} dz = \frac{2\pi i}{2!}\left(\frac{d^{2}}{dz^{2}}(5z^{2}+3\sqrt{2}z+1)\right)\bigg|_{z=i}\]\)
Taking the second derivative of f(z), we have:
\(\[\frac{d^{2}}{dz^{2}}(5z^{2}+3\sqrt{2}z+1) = 10\]\)
Substituting z=i into the expression, we get:
\(\[\int_{C} \frac{5z^{2}+3\sqrt{2}z+1}{(z-i)^{3}} dz = \frac{2\pi i}{2!}\cdot 10 = 10\pi i\]\)
Therefore, the value of the contour integral is \(\(10\pi i\)\).
Now, let's prove the inequality:
\(\[\left|\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz}\right| \leq (R^2-1)(R^2-4) \cdot \frac{2\pi R}{3} + 4\pi R\]\)
We can rewrite the integral as:
\(\[\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz} = \int_{C_R} (z^4+9z^2-2)dz\]\)
Using the triangle inequality, we have:
\(\[\left|\int_{C_R} (z^4+9z^2-2)dz\right| \leq \int_{C_R} |z^4+9z^2-2||dz|\]\)
For any point z on the contour \(C_R\)\), we have |z| = R. Using this, we can simplify the expression:
\(\[\int_{C_R} |z^4+9z^2-2||dz| = \int_{C_R} |R^4+9R^2-2||dz|\]\)
Since \(\(|R^4+9R^2-2|\)\) is a constant, we can take it outside the integral:
\(\[\int_{C_R} |R^4+9R^2-2||dz| = |R^4+9R^2-2| \int_{C_R} |dz|\]\)
The length of the contour \(\(C_R\)\) is \(\(2\pi R\)\). Substituting this into the integral, we have:
\(\[\int_{C_R} |R^4+9R^2-2||dz| = |R^4+9R^2-2| \cdot 2\pi R\]\)
Thus, we obtain:
\(\[\left|\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz}\right| \leq (R^2-1)(R^2-4) \cdot \frac{2\pi R}{3} + 4\pi R\]\)
which is the desired inequality.
Note: In the given inequality, there seems to be an error in the expression for the integral. It should be \(\(\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz}\)\) instead of \(\(\int_{C_R} \frac{z^4+5z^2+4z^2-2}{dz}\)\).
To know more about contour integral, refer to the link below:
https://brainly.com/question/32644812#
#SPJ11
30 people travelled from London to Manchester for a conference. Of these people 15 travelled by train 9 travelled by plane some travelled by both train and plane 12 did not travel by either train or plane Three people are chosen at random from those who travelled by plane. Find the probability that exactly two of these people also travelled by train.
Answers
The probability that exactly two of the three people selected also travel by train is 4/9
What is the probability of an event occurring?The probability of an event occurring is specified by the ratio of the number of required outcomes to the number of possible outcomes.
The number of people that travelled from London to Manchester for the conference = 30
Number of people that traveled by train = 15
Number of people that travelled by plane = 9
Number of people that did not travel by travel by either train or plane = 12
Number of people chosen from those that traveled by plane = 3
The probability that two of those selected also traveled by train can be found as follows;
The number of people that traveled by either train of plane = 30 - 12 = 18
The number of people that traveled by plane and train = 15 + 9 - 18 = 6
The binomial probability distribution formula that can be used to find the probability of an event, p, occurring exactly r times is as follows;
P(p) = \(_nC_r\cdot p^r\cdot q^{n-r}\)
Where;
n = Number of people chosen = 3
r = Number of people chosen that also traveled by train = 2
p = Probability that a person chosen also traveled by train = 6/9 = 2/3
q = Probability that a person chosen did not travel by train = 3/9 = 1/3
Therefore;
\(P = _3C_2\times \left(\dfrac{2}{3}\right) ^2\times \left(\dfrac{1}{3} \right)^{3-2} = \dfrac{4}{9}\)
The probability that exactly two of the people chosen at random also traveled by train is P = 4/9Learn more about the probability of an event here:
https://brainly.com/question/16237647
#SPJ1
The school band is selling candy to raise $1,800.00 to pay for their trip to a band festival. The band earns $1.50 from every box of candy sold. Kayla solved the inequality 150 x greater-than-or-equal-to 1,800 to find the number of boxes the band must sell to meet their goal. She graphed her solution on a number line and interpreted the solution to mean the band will meet their goal if they sell fewer than 12 boxes.
A number line going from 6 to 16. A closed circle is at 12. Everything to the right of the circle is shaded.
What errors did she make? Select two options.
o She used the wrong coefficient for x in her inequality.
o She should have used the less than or equal to symbol instead of the greater than or equal to symbol.
o She interpreted the graph incorrectly.
o She graphed a solution set that does not match her inequality.
o The graph should have an open circle at 12.
Answers
Answer:
The answers are
A - She used the wrong coefficient for X in her Inequality.
B- NO
C- She interpreted the graph incorrectly.
D-NO
Step-by-step explanation:
Answer:
A and C
Step-by-step explanation:
Took test on edge :)
This problem is so hard!!!!!
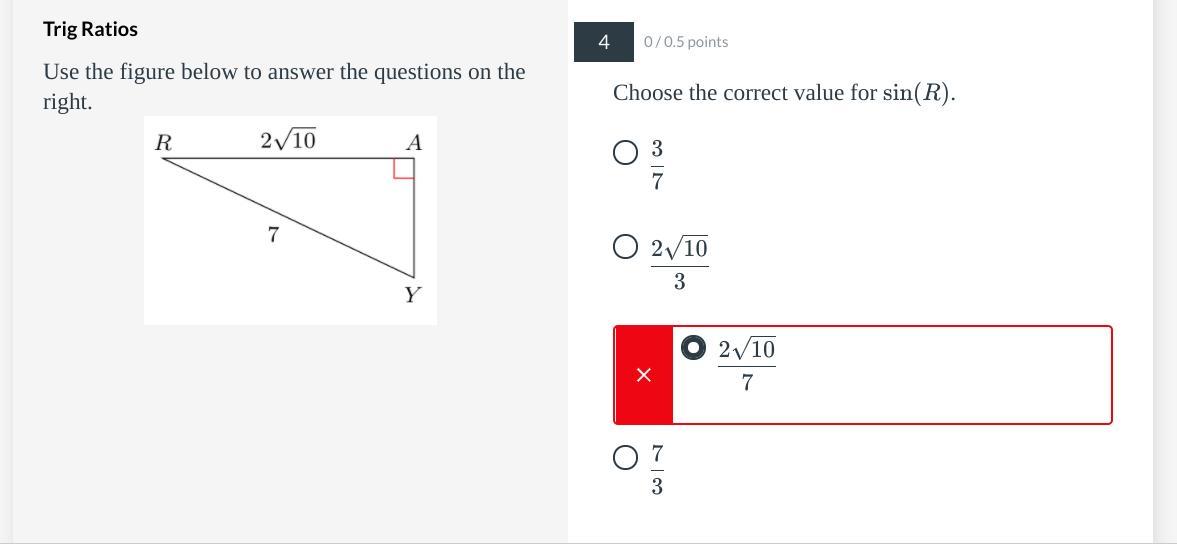
Answers
Answer:
3/7
Step-by-step explanation:
Sin (R) = opposite ÷ hypotenuse,
Find the opposite by using pythagorean theorem.
(AY)^2 + (2√10)^2 = 7^2
(AY)^2 + 40 = 49
(AY)^2 = 9
AY = 3
Sin(R) = 3/7
The final answer Sin(R) = 3/7
Which of the following polynomial functions is graphed below?-70-60-3030-1010-10O A. f(x) = (x - 5)(x - 1)?(x - 1)O B. f(x) - (x - 1)(x - 2)(x - 3)C. f(x) - (x + 5)(x + 1)?(x - 1)OD. () - (x + 4)(x - 2) (x+3)

Answers
(x+4)(x-2)²(x+3) (option D)
Explanation:To determine which polynomial was graphed, we look at the roots of the function on the graph with the options given.
The roots of the equation are the x values that make the function equal to zero.
On the graph, we check the values of the line that crosses the x axis.
Each box on the x axis represents 2 units
we have x = 2 (towards the right of the x-axis).
Note that two lines touched x = 2. This means x =2 appeared twice
we also have x = -4 (towards the left x axis)
Then we have value of x that is before the x = -4. Checking the options, the value of x will be equal to -3
Now we rewrite:
x = 2, x = 2, x = -4, x = -3
x-2 = 0, x-2 = 0, x + 4 = 0, x+3 = 0
The roots of the function:
(x-2)²(x+4)(x+3)
Hence, the polynomial functions graphed below is (x+4)(x-2)²(x+3) (option D)
The formula for the area of a kite is 2. where d, and dz are the lengths of the diagonals. If a kite has an area of 12 square feet and a diagonal with a length of 8 feet, what is the length of the other diagonal? А 4 feet B 48 feet C 3 feet D 12 feet. somebody please help ASAP

Answers
Answer:
C. 3 feet.
Step-by-step explanation:
Please help me ASAP and I have more so PLEASE
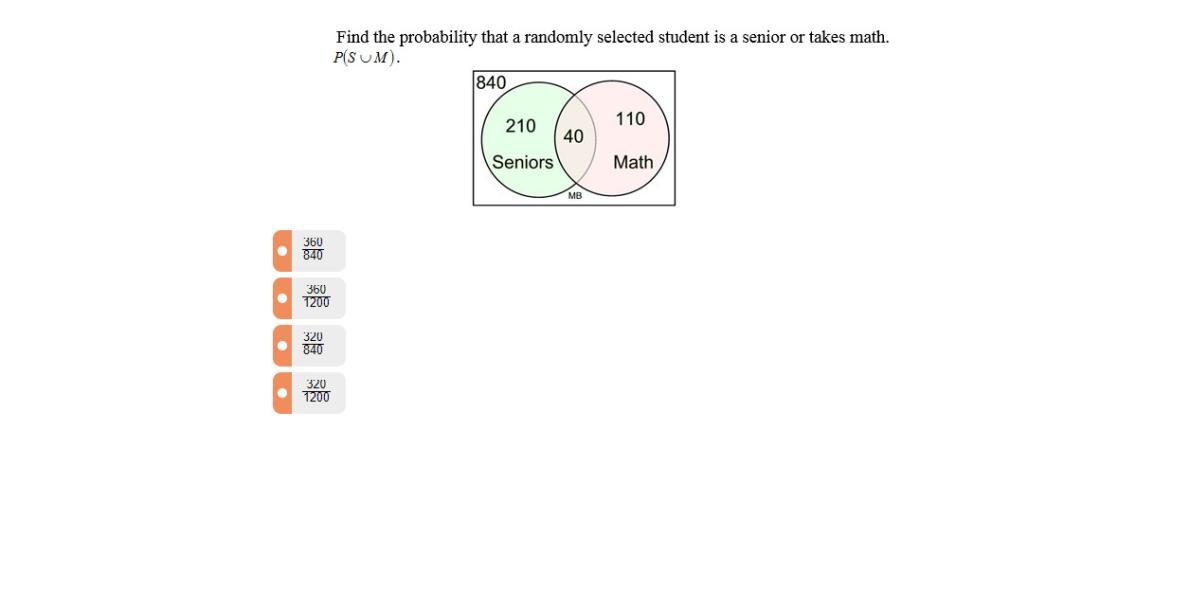
Answers
The answer is B part
As the requirement for the student is either a senior or one who studies maths or both.
So, just add the values within the two venn circles divide it by total no of values.
=> 240+40+110/240+40+110+840
=> 360/1200
Answer:
B
Step-by-step explanation:
840 people aren't senior or don't takes math.
210 + 40 = 250 are seniors
110 + 40 = 150 people take math
In this class has 840 + 210 + 40 + 110 = 1200 people => All
210 + 40 + 110 = 360 people are seniors or take math. => P( S U M)
Probabilty that a randomly selected student is a senior or takes math =>\(\frac{P(S U M)}{All}\)
\(\frac{P(S U M)}{All} = \frac{360}{1200}\)
Hope this helps ^-^
solve 70=-2.5x (one-step equation) what is this answer?
Answers
Answer:
Step by step and hope this helps
70=−2.5x
Step 1: Flip the equation.
−2.5x=70
Step 2: Divide both sides by -2.5.
−2.5x−2.5=70−2.5
x=−28
the expression 18+1.5x is 8 less than 36.5. What is the value of 4x
Answers
In the expression 1.5x + 18 = 36.5 - 8 x is 7, hence 4x is 28.
What are inequalities and their types?Inequality is a relation that compares two numbers or other mathematical expressions in an unequal way.
The symbol a < b indicates that a is smaller than b.
When a > b is used, it indicates that a is bigger than b.
a is less than or equal to b when a notation like a ≤ b.
a is bigger or equal value of an is indicated by the notation a ≥ b.
Given, An expression 18 + 1.5x is 8 less than 36.5.
∴ 1.5x + 18 = 36.5 - 8.
1.5x = 36.5 - 8 - 18.
1.5x = 10.5.
x = 10.5/1.5.
x = 7.
Therefore 4x is equal to 4(7) = 28.
learn more about inequalities here :
https://brainly.com/question/28823603
#SPJ1
To keep in shape, Leah exercises at a track near her home. She requires 84 minutes to do 8 laps running and 10 laps walking. In contrast, she requires 66 minutes to do 2 laps running and 10 laps walking. Assuming she maintains a consistent pace while running and while walking, how long does Leah take to complete a lap? Leah takes minutes to run a lap and minutes to walk a lap.
Answers
Using a system of equations, it is found that:
Leah takes 3 minutes to run a lap and 6 minutes to walk a lap.
What is the system of equations?The variables of the system of equations are presented as follows:
Variable x: time required to complete a lap running.Variable y: time required to complete a lap walking.She requires 84 minutes to do 8 laps running and 10 laps walking, hence the first equation is:
8x + 10y = 84.
She requires 66 minutes to do 2 laps running and 10 laps walking, hence the second equation is:
2x + 10y = 66.
Simplifying by 2, we have that:
x + 5y = 33.
x = 33 - 5y.
Replacing in the first equation, we can find the time it takes to walk a lap, as follows:
8(33 - 5y) + 10y = 84.
264 - 40y + 10y = 84
30y = 180
y = 180/30
y = 6 minutes.
Then the time it takes to run a lap is obtained as follows:
x = 33 - 5y = 33 - 5(6) = 33 - 30 = 3 minutes.
More can be learned about a system of equations at https://brainly.com/question/24342899
#SPJ1
Find the solutions of the equation.
23 <3x-3(-) ≤ 66
a) (-, 11)u[33, [infinity])
b)(-, 11]u[33,[infinity])
c) (11,33)
d) [11, 33]
e) (11, 33]
f) None of the above.
Answers
The solution to the inequality is:
x ∈ (-∞, -21].
The correct option is F.
To solve the given inequality, we'll first simplify the expression:
23 < 3x - 3 ≤ -66
To simplify the inequality,
23 < 3x - 3 ≤ -66
Adding 3 to all parts of the inequality:
23 + 3 < 3x - 3 + 3 ≤ -66 + 3
Simplifying:
26 < 3x ≤ -63
Next, divide all parts of the inequality by 3:
26/3 < 3x/3 ≤ -63/3
Simplifying:
8.67 < x ≤ -21
Therefore, the solution to the inequality is:
x ∈ (-∞, -21]
Learn more about Inequality here:
https://brainly.com/question/20383699
#SPJ4
Select the correct answer. If function f has zeros at -3 and 4, which graph could represent function f?

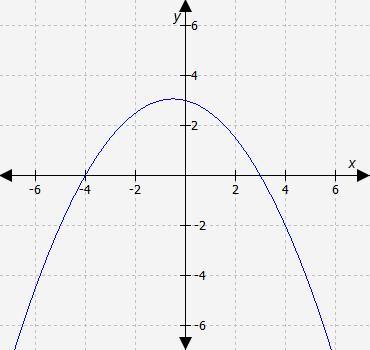


Answers
Answer:
first one
Step-by-step explanation:
The graph intersects x-axis at (4,0) and (-3,0)
If function f has zeros at -3 and 4 then the first graph represents the function f.
When a function has zeros at -3 and 4, it means that the function's value is equal to zero at those x-values.
In other words, when x = -3 and x = 4, the function f(x) becomes 0.
To represent this on a graph, you should look for two points where the graph intersects the x-axis at -3 and 4.
When the graph crosses the x-axis at these points, it indicates that the function has zeros at those x-values.
When we observe the graphs, the first graph has zeros at -3 and 4.
Hence, the first graph could represent the function f with zeros at -3 and 4.
To learn more on Functions click:
https://brainly.com/question/30721594
#SPJ4
18 movie goers bought tickets on-line. This represents 30% of the total people going to the same movie. How many people are seeing the movie?
Answers
Answer:
60
Step-by-step explanation:
\(0.3 \times x =18 \\ x = 18 \div 0.3 \\ x = 60\)
an urn contains 9 balls, 3 of which is(are) red. the selection of a red ball is desired and is therefore considered to be a success. if a person draws 2 balls from the urn, what is the probability of 2 successes?
Answers
Thus, the probability of drawing 2 red balls (2 successes) from the urn is 1/12.
To calculate the probability of 2 successes (drawing 2 red balls) from the urn, we'll use the following terms: total balls, red balls, and combinations.
There are 9 balls in the urn, 3 of which are red.
To find the probability of 2 successes, we need to determine the number of successful combinations and divide that by the total possible combinations.
Successful combinations: Choose 2 red balls from 3, which can be written as C(3,2) = 3! / (2! * (3-2)!) = 3.
Total possible combinations: Choose 2 balls from 9, which can be written as C(9,2) = 9! / (2! * (9-2)!) = 36.
Now, divide the successful combinations by the total possible combinations to find the probability of 2 successes:
Probability = (Successful combinations) / (Total possible combinations) = 3 / 36 = 1/12.
So, the probability of drawing 2 red balls (2 successes) from the urn is 1/12.
Know more about the probability
https://brainly.com/question/24756209
#SPJ11
Use the associative property of addition to rewrite (20 + 11) + 5. Which expression is equivalent? O A. 5(20 + 11) B. (10 + 10 + 10) + 5 O C. 20 + (11 +5) D. (20 - 11) +5
Answers
Answer:
i also needed help on this qustion and the awnser is
Step-by-step explanation:
C.
its the same problem and nothing changed besides the order↑≠≈
Answer:
The answer is C
Step-by-step explanation:
How many more minutes can mate car travel per gallon of gas then jenna's car
Answers
Mate's car can travel approximately 5 more minutes per gallon of gas compared to Jenna's car.
To determine how many more minutes Mate's car can travel per gallon of gas compared to Jenna's car, we would need additional information about the fuel efficiency or miles per gallon (MPG) for each car.
Fuel efficiency is typically measured in terms of miles per gallon, indicating the number of miles a car can travel on a gallon of gas.
To calculate the difference in travel time, we would also need to know the average speed at which the cars are traveling.
Once we have the MPG values for Mate's car and Jenna's car, we can calculate the difference in travel time per gallon of gas by considering their respective fuel efficiencies and average speeds.
If Mate's car has a fuel efficiency of 30 MPG and Jenna's car has a fuel efficiency of 25 MPG, we can calculate the difference in travel time by comparing the distances they can travel on a gallon of gas.
Let's assume both cars are traveling at an average speed of 60 miles per hour.
For Mate's car:
Travel time = Distance / Speed
= (30 miles / 1 gallon) / 60 miles per hour
= 0.5 hours or 30 minutes.
For Jenna's car:
Travel time = Distance / Speed
= (25 miles / 1 gallon) / 60 miles per hour
= 0.4167 hours or approximately 25 minutes.
Without specific information about the MPG values and average speeds of the cars, it is not possible to provide an accurate answer regarding the difference in travel time per gallon of gas between Mate's car and Jenna's car.
For similar questions on Mate's car
https://brainly.com/question/33586007
#SPJ8
20 POINTSSS!!
Which of the following sets of Real Numbers are Counting Numbers?
A. { 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 }
B. { 1, 2, 3, 4 ... }
C. { 0, 1, 2, 3, 4 ...}
D. { ...-2, -1, 0, 1, 2 ... }
Answers
Answer:
option D. {......-2, -1, 0, 1, 2 ......}
What are even and odd numbers from 1 to 100?
Answers
In mathematics, a number is considered to be "even" if it is divisible by 2, and "odd" if it is not divisible by 2. The numbers from 1 to 100 can be divided into two groups: even numbers and odd numbers.
Even numbers are numbers that are divisible by 2, such as 2, 4, 6, 8, and so on. When you divide an even number by 2, the remainder will always be 0. Some examples of even numbers between 1 and 100 include: 2, 4, 6, 8, 10, 12, 14, 16, 18, 20, 22, 24, 26, 28, 30, 32, 34, 36, 38, 40, 42, 44, 46, 48, 50, 52, 54, 56, 58, 60, 62, 64, 66, 68, 70, 72, 74, 76, 78, 80, 82, 84, 86, 88, 90, 92, 94, 96, 98, and 100.
Odd numbers are numbers that are not divisible by 2, such as 1, 3, 5, 7, and so on. When you divide an odd number by 2, the remainder will always be 1. Some examples of odd numbers between 1 and 100 include: 1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29, 31, 33, 35, 37, 39, 41, 43, 45, 47, 49, 51, 53, 55, 57, 59, 61, 63, 65, 67, 69, 71, 73, 75, 77, 79, 81, 83, 85, 87, 89, 91, 93, 95, 97, and 99.
It is important to note that 0 is not a positive or negative number and it is not considered as odd or even.
Knowing the difference between even and odd numbers is an important concept in math, especially when working with patterns, sequences, and operations. Understanding even and odd numbers can help you better understand the properties of numbers and how they can be used in different contexts.
to know more about even numbers refer here
https://brainly.com/question/2289438#
#SPJ11
*
A rectangle is formed by plotting four points, as shown below. What is the length of side
BC?
AL
JB
10
9
8
7
6
A(2, 9)
B(8,9)
C(8,4)
D(2, 4)
5
D
с
3
2
1
0
X
0 1 2 3 4 5 6 7 8 9 10
Done
Answers
Given the graphs of f(x) = X - 8 and g(x) = -4x + 2, find the solution to this system of equations: Y - X = -8 and 4x + y = 2 A) (0, 2) B) (0.-8) C) (2, -6) D)

Answers
Answer
The Answer is C) 2, -6
Step-by-step explanation:
Look at the graph as you can see there is only one solution to that graph and on the line intersected there is one point which that would be 2, -6.
Note: Understand that when asking about the point or something else look for the lines intersected each other then you could find your answer. If there is only one line then it is infinite solutions but if the lines don't intersect then it is a no solution.
Have a good day :)
The number of arcraft departures (in billions) for the years 2000-2006 can be approximated by p(x)= 0.0154x¹-0.2618x³ + 1.33x²-1.54x+9.1, where = 0 corresponds to the year 2000 The departures
Answers
The number of aircraft departures (in billions) from 2000 to 2006 can be approximated by the polynomial function p(x) = 0.0154x - 0.2618x³ + 1.33x² - 1.54x + 9.1, where x = 0 corresponds to the year 2000.
The given polynomial function p(x) represents the number of aircraft departures in billions for the years 2000 to 2006, with x representing the number of years since 2000. The coefficients of the polynomial determine the behavior of the function over the given range.
The function p(x) is a polynomial of degree 3, meaning it is a cubic function. The terms of the polynomial are multiplied by powers of x, representing the increasing influence of each term as the years progress.
The coefficients of the polynomial, 0.0154, -0.2618, 1.33, -1.54, and 9.1, determine the specific shape and behavior of the function. Each coefficient affects the rate of change, concavity, and intercepts of the function. For example, the coefficient of x³ (-0.2618) indicates a negative concavity, while the constant term (9.1) represents the number of aircraft departures in the year 2000 (x = 0).
By evaluating the function p(x) for different values of x within the given range, we can approximate the number of aircraft departures for each corresponding year.
Learn more about polynomial function here
https://brainly.com/question/11298461
#SPJ11
For every 7 push-ups Dulce can do, Sara can do 6. If Ducle did 28 push-ups during gym class, how many push-ups did sara do?
Answers
The number of push-ups did sara did during gym class if for every 7 push-ups Dulce can do, Sara can do 6 is 24 push ups.
How to solve ratio?Number of push ups Dulce can do : number of push ups Sara can do
Let
number of push ups Sara does = x
7 : 6 = 28 : x
7/6 = 28/x
cross product
7 × x = 28 × 6
7x = 168
divide both sides by 7
x = 168/7
x = 24
Therefore, Sara does 24 push ups during the gym class.
Read more on ratio:
https://brainly.com/question/2328454
#SPJ1
If the total bill was $42.50 and you tipped 15%, how much would the TIP be?
Answers
Answer:
About 6.38$
.........................
Can someone help me

Answers
The given table of the values of f(x) is used to complete the table for the function \(y = f \left( \frac{1}{5} \cdot x \right) \), to give;
The following values of x and y as follows;
x: -0.8, -0.2, 0, 0.6, 1.2
y: 7, -2, 3, -4, 5
Please also see the attached table
How can the function, f(x) be scaled to give the function, \(y = f \left( \frac{1}{5} \cdot x \right) \)?The question is with regards to scaling a graph horizontally.
Using the rule of scaling a graph, we have;
Given that a point on the graph of f(x) is (x, y), the corresponding point in the graph of f(C•x) is (C•x, y).
The given modification of the function is presented as follows;
\(y = f \left( \frac{1}{5} \cdot x \right) = f \left( 0.2 \cdot x \right)\)
The points on the graph that completes the table are therefore;
(0.2×(-4), 7) = (-0.8, 7)(0.2×(-1), -2) = (-0.2, -2)(0.2×0, 3) = (0, 3)(0.2×3, (-4)) = (0.6, -4)(0.2×6, 5) = (1.2, 5)The completed table is therefore;
x y
-0.8 7
-0.2. -2
0 3
0.6. -4
1.2. 5
Learn more about scaling of a function here:
https://brainly.com/question/3381225
#SPJ1
