Which of the following quantities are inversely proportional? WILL GIVE BRAINIEST
a
The number of hours worked and the amount of money earned, assuming the worker is paid by the hour
Answers
Answer:
option 1
option 3
option 4
Step-by-step explanation:
Inversely proportional means "one quality increases, the other decreases " or "one quantity decreases ,other increases. " Inverse relantionship, that's it.
option 1:
Since the volume is given as 56 and we know the volume is area of base * height, to keep the volume at constant [56], if height increases, area of base would need to decrease, and vice versa. So Inversely proportional.
option 2:
The time spend in plowing a field with a tractor with increase when we area using more fuel.So more fuel used means more time spent. So these are directly proportional, Not inverse.
option 3:
Since the price is a constant, the amount of length we buy more,the price per one yard HAS to decrease, and vice versa. So ,this is Inverse relantionship.
option 4:
If the number of identical tractors. increase, it will take less time to plow the set 358 acre farm. And vice versa. So this is Inversely proportional as well.
PLEASE MARK ME AS BRAINLIEST!!
Related Questions
This is 8th-grade math and I have to turn this in tomorrow because the and of the term ends tomorrow.

Answers
Answer:
the first one I don't know
the second is 15
third i think is 512
Please help due today

Answers
What is the range of the given function?

Answers
Answer:
you have it on the correct answer
Step-by-step explanation:
range is the y values, and its the lowest negetive to the highest positive
Find the scalar and vector projections of b onto a.
a=⟨−5, 12⟩, b=⟨4, 6⟩
Answers
The scalar projection is 42/13, and the vector projection is 42/169⟨-5,12⟩.
The scalar projection of b onto a can be calculated using the formula \(\frac{a.b}{|a|}\), and the vector projection of b onto a can be calculated using the formula \(\frac{a.b}{|a|^{2} } .a\). The formula for finding the magnitude of a is |a| = √c²+d² if a = ⟨c,d⟩.
a = ⟨−5, 12⟩, b = ⟨4, 6⟩
a⋅b = ⟨−5, 12⟩⟨4, 6⟩
= -20 + 72
= 42
|a| = √[(-5)² + (12)²]
= √25 + 144
= √169
= 13
The scalar projection is
\(\frac{a.b}{|a|}\) = [⟨−5, 12⟩⟨4, 6⟩]/√[(-5)² + (12)²]
= [-20 + 72]/√25 + 144
= 42/√169
= 42/13
The vector projection is
\(\frac{a.b}{|a|^{2} } .a\) = [42/(13)²] ⟨−5, 12⟩
= 42/169⟨−5, 12⟩
To know more about scalar projection, here
https://brainly.com/question/12924145
#SPJ4
Nell participated in a 3-day charity walk. Sheraised $0.50 for each 1/3 of mile that she walked. Thefirst day, Nell walked 12 miles. The second day, shewalked 8 miles. The third day, she walked 16 miles.How much money did Nell raise?
Answers
the Numbers of days = 3
The second statement(She raised $0.50 for each 1/3 of mile that she walked) means
1/3 miles = $0.50
For first day walk we have:
1/3 miles = $0.50
12 miles = 12 x 0.50 x 3 = $18
For Second day walk we have:
1/3 miles = $0.50
8miles = 8 x 0.50 x 3 = $12
For Third day walk we have:
1/3 miles = $0.50
16miles = 16 x 0.50 x 3 = $24
The amount she raised for the three days becomes
$18 + $12 + $24
=$54
Question 8 of 10 A differential equation is: A. any equation involving a differentiable function. B. any equation involving an integral function. C. any equation involving a derivative. D. any equation involving two or more derivatives. E. any equation involving a derivative where the antiderivative is known.
Answers
A differential equation is an equation involving a differentiable function, which is a critical tool in modeling physical phenomena like population growth, radioactive decay, and fluid flow.
A differential equation is an equation that involves a differentiable function. It is an equation in which the variables' derivatives appear. Differential equations are used to model physical phenomena like population growth, radioactive decay, and fluid flow. The order of a differential equation is the highest order of the derivative of the function. A first-order differential equation has the highest order of 1, and a second-order differential equation has the highest order of 2.A differential equation can be classified into three types: Ordinary Differential Equations (ODEs), Partial Differential Equations (PDEs), and Differential Algebraic Equations (DAEs). Ordinary differential equations have a single independent variable and one or more dependent variables that depend on it. Partial differential equations have more than one independent variable and multiple dependent variables that depend on each other. Differential algebraic equations have both derivatives and algebraic equations in them.A differential equation is essential in physics, engineering, and mathematics. It is used to model many natural phenomena and helps in predicting the future. Most differential equations can not be solved analytically, so numerical methods are used to find approximate solutions. In conclusion, A differential equation is an equation involving a differentiable function, which is a critical tool in modeling physical phenomena like population growth, radioactive decay, and fluid flow.
Learn more about diffential equation here,
https://brainly.com/question/28099315
#SPJ11
HELP I NEED HELP ASAP

Answers
Answer:
B
Step-by-step explanation:
HELP ITS MATH AND I SUCK AT MATH WILL GIVE BRAINLIEST :)))))

Answers
Answer:
i believe it's A. f(x) slope is greater than g(x) slope
Step-by-step explanation:
the slope of f(x) is -2, while g(x) is -6. you may think "why isn't -6? 6 is bigger, isn't it?" In this case, they're both negative so the number closer to zero is the greater one
Answer:
A
Step-by-step explanation:
18. Jamison expanded 2,748 using place value. Which number sentence shows 2,748 in expanded form? A (2 x 1,000) + (74 x 100) + (8 x 1) B.(2 x 100) + (7 1,000) + (48 x 1) C. (2 x 100) + (7 x 100) + (4 x 10) + (8 x 1) D. (2 x 1,000) + (7 X 100) + (4 x 10) + (8 x 1)
Answers
Notice that:
\(\begin{gathered} 2748=2000+700+40+8 \\ =2\times1000+7\times100+4\times10+8\times1. \end{gathered}\)Answer: Option D) 2748 in expanded form is:
\((2\times1000)+(7\times100)+(4\times10)+(8\times1).\)A table is sold hire purchase. The sales price consists of deposit of $306 and six monthly instalments of $60 each. How much does a customer pay for the table?
Answers
Answer: $666
Step-by-step explanation: 306+ 60(6)= 666
6. How many times larger is the first number in the pair than the second? a. 34 is times larger than 3³. times larger than 5². times larger than 78. times larger than 17. times larger than 5*. b. 5³ is_____ c. 710 is d. 176 is e. 5 1⁰ is

Answers
3⁴ is 3 times larger than 3³, 5³ is 5 times larger than 5², 7¹⁰ is 49 times larger than 7⁸ and 17⁶ is 289 times larger than 17⁴.
3⁴ / 3³ = (3 × 3 × 3 × 3) / (3 × 3 × 3) = 3
This means that 3⁴ is 3 times larger than 3³.
5³ is 5 times larger than 5².
5³ / 5² = (5 × 5 × 5) / (5× 5) = 5
7¹⁰ is 49 times larger than 7⁸.
7¹⁰/ 7⁸ = 7² =49
17⁶ is 289 times larger than 17⁴.
17⁶ /17⁴ = 289
Hence, 3⁴ is 3 times larger than 3³, 5³ is 5 times larger than 5², 7¹⁰ is 49 times larger than 7⁸ and 17⁶ is 289 times larger than 17⁴.
To learn more on Number system click:
https://brainly.com/question/22046046
#SPJ1
Which set of ordered pairs represents a function?
o {(6,5), (-5,4), (5, 7), (6, -8)}
o {(2, 1), (5,-6), (8,-5), (4, -6)}
{(4,-5), (-7,3), (-7, -8), (-5, -4)}
o {(6,-1), (-7,0), (6,3), (-2,4)}
Answers
factor this trinomial completely -10x^2+36x+16
Answers
Answer:
-2(5x+2)(x-4)
Step-by-step explanation:
(⊙o⊙) <-- it wants Brainliest
Answer:
-2(5x+2)(x-4)
Step-by-step explanation:
Yani buys a certain brand of cereal that cost you $10 per box. Yani changes to a super-saving brand of the same size. The equation shows the price, y, as a function of the number of boxes, x, for the new brand. Y= 7x
Part A: How many more dollars is the price of a box if Yanis original of cereal than the price of a box of super-saving cereal? Show your work.
Part B: how much money does Yani save each month with the change In cereal brand if he buys 5 cereal boxes each month? Show your work.
Answers
Answer:
part A= 3
Part B= 15$
Step-by-step explanation:
Part A= 10-7=3
Part B = 5*10 =50 7*5= 35 50-35= 15$
Point I is on line segment HJ. Given HI = x, IJ = 2x + 9, and
HJ = 4x, determine the numerical length of IJ.
Answers
Answer:27
Step-by-step explanation:
The numerical length of IJ is 27 units.
What is a line segment?A line segment in geometry is a section of a line that has two clearly defined endpoints and contains every point on the line that lies within its confines.
Given that Point, I is on line segment HJ. Given HI = x, IJ = 2x + 9, and
HJ = 4x.
The total length of the line will be written as below:-
HJ = HI + IJ
4x = x + 2x + 9
4x = 3x + 9
4x - 3x = 9
x = 9
The length of the segment IJ will be:-
IJ = 2x + 9
IJ = ( 2 x 9 ) + 9
IJ = 18 + 9
IJ = 27
Therefore, the numerical length of IJ is 27 units.
To know more about line segments follow
https://brainly.com/question/2437195
#SPJ2
How much cement is needed to build a sidewalk that is 50m long, 8m wide, and 0.5m thick? Responses 200 m³ 66.7 m³ 667 m³ 2000 m³
Answers
Hi! I'm Pearl, from your math class, you're the one who always uses ♡ this ♡
during class with Leah, right?
Anyways, here is your answer:
200 meters^3
Volume = 50×8×0.5 = 200 meters^3
Correct me if I'm wrong! :)
Answer: 200m³
Step-by-step explanation: 50m x 8m x 0.5m
Haaaaaaaaaaaaaaaaaaai
Answers
Identify A, B, and C
-6x2-5x+3=-8X2-4x
Answers
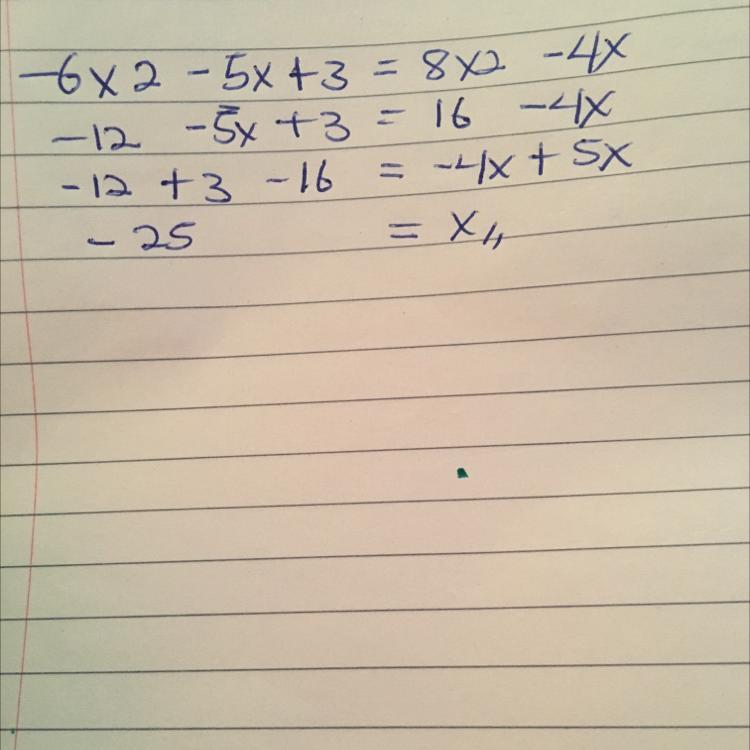
A study of 244 advertising firms revealed their income after taxes: Income after Taxes Under $1 million $1 million to $20 million $20 million or more Number of Firms 128 62 54 W picture Click here for the Excel Data File Clear BI U 8 iste : c Income after Taxes Under $1 million $1 million to $20 million $20 million or more B Number of Firms 128 62 Check my w picture Click here for the Excel Data File a. What is the probability an advertising firm selected at random has under $1 million in income after taxes? (Round your answer to 2 decimal places.) Probability b-1. What is the probability an advertising firm selected at random has either an income between $1 million and $20 million, or an Income of $20 million or more? (Round your answer to 2 decimal places.) Probability nt ences b-2. What rule of probability was applied? Rule of complements only O Special rule of addition only Either
Answers
a. The probability that an advertising firm chosen at random has under probability $1 million in income after taxes is 0.52.
Number of advertising firms having income less than $1 million = 128Number of firms = 244Formula used:P(A) = (Number of favourable outcomes)/(Total number of outcomes)The total number of advertising firms = 244P(A) = Number of firms having income less than $1 million/Total number of firms=128/244=0.52b-1. The probability that an advertising firm chosen at random has either an income between $1 million and $20 million, or an Income of $20 million or more is 0.48. (Round your answer to 2 decimal places.)Explanation:Given information:Number of advertising firms having income between $1 million and $20 million = 62Number of advertising firms having income of $20 million or more = 54Total number of advertising firms = 244Formula used:
P(A or B) = P(A) + P(B) - P(A and B)Probability of advertising firms having income between $1 million and $20 million:P(A) = 62/244Probability of advertising firms having income of $20 million or more:P(B) = 54/244Probability of advertising firms having income between $1 million and $20 million and an income of $20 million or more:P(A and B) = 0Using the formula:P(A or B) = P(A) + P(B) - P(A and B)P(A or B) = 62/244 + 54/244 - 0=116/244=0.48Therefore, the probability that an advertising firm chosen at random has either an income between $1 million and $20 million, or an Income of $20 million or more is 0.48.b-2. Rule of addition was applied.
To know more about probability visit:
https://brainly.com/question/11234923
#SPJ11
Make a number line and mark the points that represent the following values of x. X ≤ 1 2/3 and x <- 3/4
Answers
Answer:
can't you do Russian math homework on your own
Step-by-step explanation:
Consider a two-period binomial model with risk-neutral prob- ability distribution p=0.6, q=0.4. Let V2 be the payoff for a derivative with: Va(ww.) = { s 1 if w1 = H, W2 = H or w1 = T, W2 =T 0 otherwise Find the price of this derivative.
Answers
To price the derivative using the two-period binomial model, we need to calculate the expected payoff of the derivative using the risk-neutral probabilities.
The possible outcomes for the two-period binomial model are H and T, there are four possible states of the world: HH, HT, TH, and TT.
To calculate the expected payoff we need to calculate the probability of each state occurring. The probability of HH occurring is pp=0.60.6=0.36, the probability of HT and TH occurring is pq+qp=0.60.4+0.40.6=0.48, and the probability of TT occurring is qq=0.40.4=0.16.
Next, we can calculate the expected payoff in HH and TT states, the derivative pays off 1, and in the HT and TH states, the derivative pays off 0. The expected payoff of the derivative in the HH and TT states is 10.36=0.36, and the expected payoff in the HT and TH states is 00.48=0.
We need to discount the expected payoffs back to time 0 using the risk-neutral probabilities.
The probability of that state occurring multiplied by the discount factor, which is 1/(1+r), where r is the risk-free interest rate.
Since this is a risk-neutral model, the risk-free interest rate is equal to 1. Therefore, the risk-neutral probability of each state occurring is
HH: 0.36/(1+1) = 0.18
HT/TH: 0.48/(1+1) = 0.24
TT: 0.16/(1+1) = 0.08
Finally, we can calculate the price of the derivative
Price = 0.181 + 0.240 + 0.240 + 0.081 = 0.26
Therefore, the price of the derivative is 0.26.
For similar question on two-period binomial model
https://brainly.com/question/9362207
#SPJ11
Karthik borrows 1200 from junaid for 2 years for 20% rate of interest how much karthik should play to compound annually
Answers
Answer:
1728
Step-by-step explanation:
1200 borrowed for 2 years at 20% compounded annually ;
Using the compound interest formula :
A = P(1 + r/n)^nt
P = 1200 = principal
Time, t = 2 years
Rate, r = 20% = 0.2
A = 1200(1 + 0.2)^2
A = 1200(1.2)^2
A = 1200 * 1.44
A = 1728
Final amount to be repayed = 1728
Write equations for the horizontal and vertical lines passing through the point (5,2).
Answers
The required equation of the vertical line is x=5 and the horizontal line is y=2 when passing through the point (5,2).
What is equation?A straight line's general equation is y = mx + c, where m is the gradient and y = c is the value at which the line intersects the y-axis. This value c is known as the y-axis intercept. A linear equation has the algebraic form y=mx+b, where m and b are constants, x is the independent variable, and y is the dependent variable. A linear equation is stated in statistical terms as y=a+bx, where a and b are constants. The equation of a two-dimensional line is ax+by=c; it is fair to predict that the equation of a three-dimensional line is ax+by+cz=d; reasonable, but incorrect—it turns out that this is the equation of a plane.
Here,
As the coordinate is (5,2),
The vertical line is x=5
The horizontal line is y=2
When passing through the point (5,2), the vertical line's required equation is x=5, and the horizontal line's required equation is y=2.
To know more about equation,
https://brainly.com/question/10413253
#SPJ4
If the Weight of Candy Is proportional to the price and 12 ounces of candy is $3.00,dollars how much is 20 ounces of candy?
Answers
Answer:
$5.00
Step-by-step explanation:
if you divide $3.00 by 12 you get $0.25 which is what your paying per ounce so all you would have to do once you get how much your paying per ounce is multiply your per ounce number ($0.25) by your total amount of ounces (20) and you then get your answer $5.00. Hope this makes sense!
What is the slope of the line that passes through the points (0, –7) and (–4, 3)?
Answers
-5/2 is the slope of thosr points
Select all the correct answers.
Which expressions are equivalent to log4 (²) ?

Answers
Answer:
A: -1 + 2 log4^x
C: log4 (1/4) + log4 x^2
Step-by-step explanation:
Apply logarithm properties:
log4 (1/4x^2) = log4 (1/4) + log4 x^2
Evaluate: log4 (1/4)
log4 (1/4) = -1
Substitute the value back:
-1 + lg4 x^2
Apply logarithm properties:
-1 + 2 log4 ^x
Draw a conclusion:
The expressions equivalent to: log4 (1/4x^2) are:
Answer Choices: A, and C
A= -1 + 2 log4^x
C= log4 (1/4) + log4 x^2
Hope this helps!
The radius of a circle is 10 cm. Find its circumference in terms of pi
Answers
Answer:
20 pi is the correct answer
Which is an equation in point-slope form for the given point and slope? point: (–3, 7); slope: 4
Answers
The equation of line that passes a point (–3, 7) and has slope of 4, in the point-slope form is y - 7 = 4 (x + 3)
A linear equation can be expressed in three forms:
- slope intercept form : y = mx + c
- point-slope form: y - y₁ = m (x - x₁)
- standard form: Ax + By + C = 0
Where:
m = slope
(x₁, y₁) = point on the line
A, B, C are constant
In this problem, the point is (–3, 7) and the slope is 4. Hence,
x₁ = –3
y₁ = 7
m = 4
Plug these parameters into the equation:
y - y₁ = m (x - x₁)
y - 7 = 4 (x - (-3))
y - 7 = 4 (x + 3)
Learn more about point-slope form here:
https://brainly.com/question/24907633
#SPJ4
Given the definitions of f(x) and g(x) below, find the value of g(f(-3)).

Answers
Answer:
-11
Step-by-step explanation:
f(x) = 5x + 11
g(x) = x^2 + 4x - 11
g(f(-3)) = ?
f(-3) = 5(-3) + 11
= -15 + 11 = -4
g(f(-3)) = g(-4) = -(4)^2 + 4(-4) - 11
= 16 - 16 - 11 = -11
A function assigns the value of each element of one set to the other specific element of another set. The value of g(f(-3)) is -11.
What is a Function?A function assigns the value of each element of one set to the other specific element of another set.
Given f(x)=5x+11 and g(x)=x²+4x-11, therefore, we can write the value of g(f(x)) as,
g(f(x)) = (5x + 11)² + 4(5x + 11) - 11
= 25x² + 121 + 110x + 20x + 44 - 11
= 25x² + 130x + 154
Now, substitute the value of x as -3 in the function g(f(x)), therefore, we can write,
g(f(-3)) = 25x² + 130x + 154
= 25(3)² + 130(-3) + 154
= 225 - 390 + 154
= -11
Hence, the value of g(f(-3)) is -11.
Learn more about Function here:
https://brainly.com/question/5245372
#SPJ2
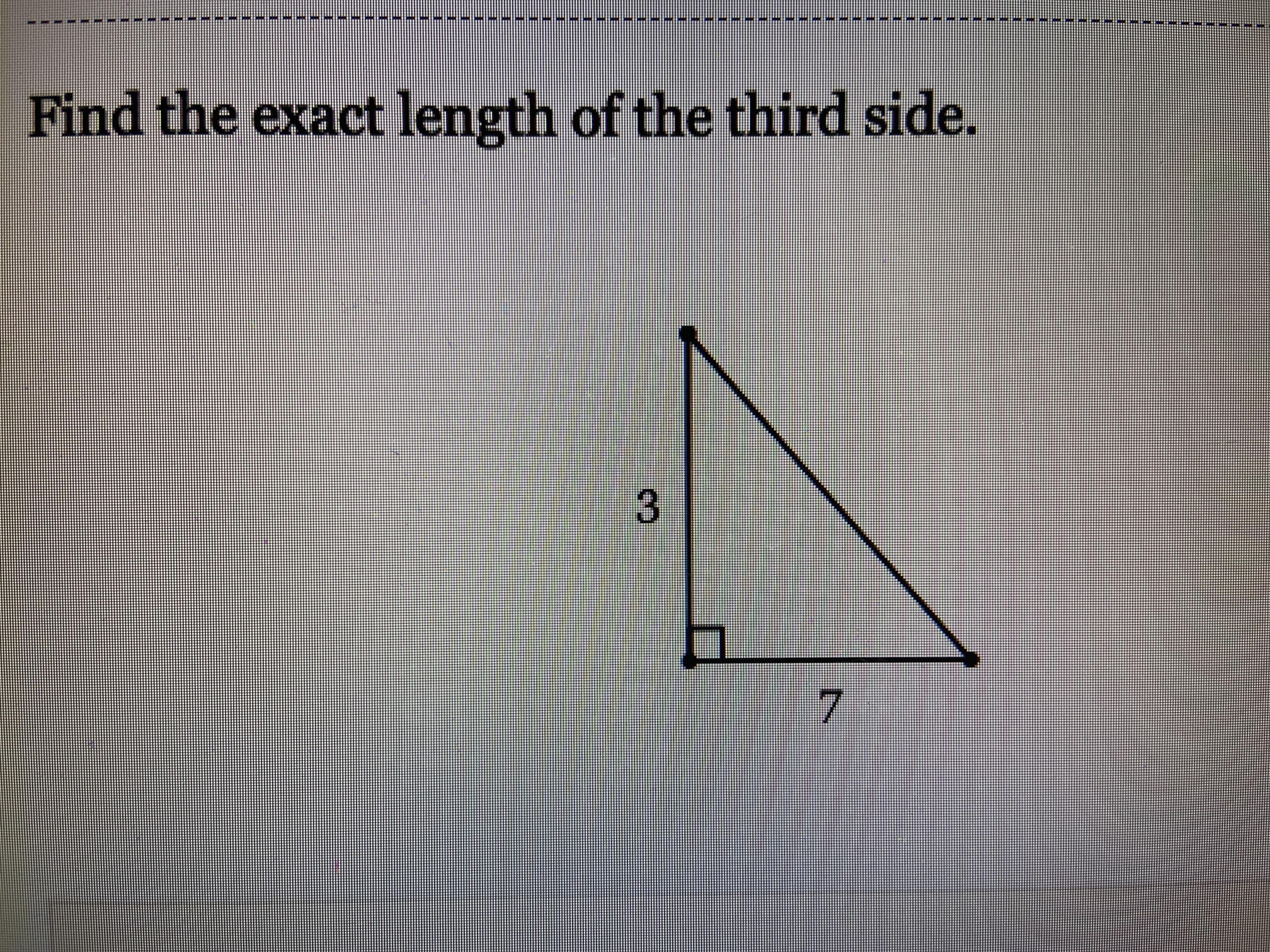
I need help, i don’t understand how to do it
Answers
It will be 7^2 + 3^2 = 58
square root 58 and you will get 7.62
Hope this helps and i am sorry if my answer was wrong .